Hostname: page-component-78c5997874-4rdpn Total loading time: 0 Render date: 2024-11-17T16:13:04.876Z Has data issue: false hasContentIssue false
  • English
  • Français

A Note on Chirally Cosmetic Surgery on Cable Knots

Published online by Cambridge University Press:  29 April 2020

Tetsuya Ito
Tetsuya Ito*
Affiliation:
Department of Mathematics, Kyoto University, Kyoto606-8502, JAPAN
*
e-mail: tetitoh@math.kyoto-u.ac.jp
Get access Rights & Permissions [Opens in a new window]

Abstract

We show that a 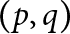$(p,q)$-cable of a non-trivial knot K does not admit chirally cosmetic surgeries for 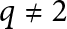$q\neq 2$, or $q=2$ with additional assumptions. In particular, we show that a $(p,q)$-cable of a non-trivial knot K does not admit chirally cosmetic surgeries as long as the set of JSJ pieces of the knot exterior does not contain the 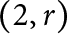$(2,r)$-torus exterior for any r. We also show that an iterated torus knot other than the $(2,p)$-torus knot does not admit chirally cosmetic surgery.

Keywords

Chirally cosmetic surgerycable knots
Type
Article
Information
Canadian Mathematical Bulletin , Volume 64 , Issue 1 , March 2021 , pp. 163 - 173
Check for updates
Copyright
© Canadian Mathematical Society 2020

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

The author has been partially supported by JSPS KAKENHI Grant Number 19K03490, 16H02145.

References

Boyer, S. and Lines, D., Surgery formulae for Casson’s invariant and extensions to homology lens spaces. J. Reine Angew. Math. 405 (1990), 181–220.Google Scholar
Bleiler, S., Hodgson, C., and Weeks, J., Cosmetic surgery on knots. In: Proceedings of the Kirbyfest (Berkeley, CA, 1998), Geom. Topol. Monogr., 2, Geom. Topol. Publ., Coventry. 1999, pp. 23–34. https://doi.org/10.2140/gtm.1999.2.23Google Scholar
Gordon, C., Dehn surgery and satellite knots. Trans. Amer. Math. Soc, 275 (1983), 687–708. https://doi.org/10.2307/1999046CrossRefGoogle Scholar
Hatcher, A., Notes on basic 3-manifold topology. https://pi.math.cornell.edu/hatcher/3M/3Mdownloads.htmlGoogle Scholar
Hom, J., A note on cabling and L-space surgeries. Algebr. Geom. Topol. 11 (2011), 219–223. https://doi.org/10.2140/agt.2011.11.219CrossRefGoogle Scholar
Ichihara, K., Ito, T., and Saito, T., Chirally cosmetic surgeries and Casson invariants. Tokyo J. Math., to appear.Google Scholar
Ichihara, K. and Jong, I. D., Cosmetic banding on knots and links, with an appendix by H. Masai. Osaka J. Math. 55 (2018), 731–745.Google Scholar
Ito, T., On LMO invariant constraints for cosmetic surgery and other surgery problems for knots in S 3. Comm. Anal. Geom. 28 (2020), 321–349.CrossRefGoogle Scholar
Kirby, R. (ed.), Problems in low-dimensional topology. AMS/IP Stud. Adv. Math., 2.2, Geometric topology (Athens, GA, 1993). Amer. Math. Soc., Providence, RI, 1997, pp. 35–473.Google Scholar
Ozsváth, P. and Szabó, Z., On knot Floer homology and lens space surgeries. Topology 44 (2005), 1281–1300. https://doi.org/10.1016/j.top.2005.05.001CrossRefGoogle Scholar
Ozsváth, P. and Szabó, Z., Knot Floer homology and rational surgeries. Algebr. Geom. Topol. 11 (2011), no. 1, 1–68. https://doi.org/10.2140/agt.2011.11.1CrossRefGoogle Scholar
Rong, Y. W., Some knots not determined by their complements. In: Quantum topology, Ser. Knots Everything, 3, World Sci. Publ., River Edge, NJ, 1993, pp. 339–353.CrossRefGoogle Scholar
Scharlemann, M., Producing reducible 3-manifolds by surgery on a knot. Topology 29 (1990), 481–500. https://doi.org/10.1016/0040-9383(90)90017-ECrossRefGoogle Scholar
Tao, R., Cable knots do not admit cosmetic surgeries. J. Knot Theory Ramifications 28 (2019), 1950034. https://doi.org/10.1142/S0218216519500342CrossRefGoogle Scholar
Walker, K., An extension of Casson’s invariant. Annals of Mathematics Studies, 126, Princeton University Press, Princeton, NJ, 1992. https://doi.org/10.1515/9781400882465Google Scholar