Hostname: page-component-6bf8c574d5-7jkgd Total loading time: 0 Render date: 2025-02-18T09:42:02.815Z Has data issue: false hasContentIssue false
  • English
  • Français

On the greatest common divisor of n and the nth Fibonacci number, II

Part of: Elementary number theory Multiplicative number theory Sequences and sets

Published online by Cambridge University Press:  06 October 2022

Abhishek Jha Open the ORCID record for Abhishek Jha [Opens in a new window]  and
Carlo Sanna Open the ORCID record for Carlo Sanna [Opens in a new window]
Abhishek Jha
Affiliation:
Indraprastha Institute of Information Technology, Okhla Industrial Estate, Phase-3, New Delhi, India e-mail: abhishek20553@iiitd.ac.in
Carlo Sanna*
Affiliation:
Department of Mathematical Sciences, Politecnico di Torino, Corso Duca degli Abruzzi 24, Torino, Italy
*
e-mail: carlo.sanna@polito.it
Get access Rights & Permissions [Opens in a new window]

Abstract

Let 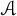$\mathcal {A}$ be the set of all integers of the form 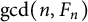$\gcd (n, F_n)$ , where n is a positive integer and 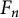$F_n$ denotes the nth Fibonacci number. Leonetti and Sanna proved that 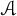$\mathcal {A}$ has natural density equal to zero, and asked for a more precise upper bound. We prove that

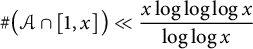$$ \begin{align*} \#\big(\mathcal{A} \cap [1, x]\big) \ll \frac{x \log \log \log x}{\log \log x} \end{align*} $$
for all sufficiently large x. In fact, we prove that a similar bound also holds when the sequence of Fibonacci numbers is replaced by a general nondegenerate Lucas sequence.

Keywords

Fibonacci numbersgreatest common divisorLucas sequencerank of appearanceupper bound

MSC classification

Primary: 11B39: Fibonacci and Lucas numbers and polynomials and generalizations
Secondary: 11A05: Multiplicative structure; Euclidean algorithm; greatest common divisors 11N25: Distribution of integers with specified multiplicative constraints
Type
Article
Information
Canadian Mathematical Bulletin , Volume 66 , Issue 2 , June 2023 , pp. 617 - 625
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press on behalf of The Canadian Mathematical Society

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ailon, N. and Rudnick, Z., Torsion points on curves and common divisors of ${a}^k-1$ and ${b}^k-1$ . Acta Arith. 113(2004), no. 1, 31–38.CrossRefGoogle Scholar
Alba González, J. J., Luca, F., Pomerance, C., and Shparlinski, I. E., On numbers $n$ dividing the $n$ th term of a linear recurrence . Proc. Edinb. Math. Soc. (2) 55(2012), no. 2, 271–289.CrossRefGoogle Scholar
André-Jeannin, R., Divisibility of generalized Fibonacci and Lucas numbers by their subscripts . Fibonacci Quart. 29(1991), no. 4, 364–366.Google Scholar
Bilu, Y., Hanrot, G., and Voutier, P. M., Existence of primitive divisors of Lucas and Lehmer numbers . J. Reine Angew. Math. 539(2001), 75–122, with an appendix by M. Mignotte.Google Scholar
Bugeaud, Y., Corvaja, P., and Zannier, U., An upper bound for the G.C.D. of ${a}^n-1$ and ${b}^n-1$ . Math. Z. 243(2003), no. 1, 79–84.CrossRefGoogle Scholar
Cubre, P. and Rouse, J., Divisibility properties of the Fibonacci entry point . Proc. Amer. Math. Soc. 142(2014), no. 11, 3771–3785.CrossRefGoogle Scholar
Halberstam, H. and Richert, H.-E., Sieve methods, London Mathematical Society Monographs, 4, Academic Press [Harcourt Brace Jovanovich], London–New York, 1974.Google Scholar
Jha, A., On terms in a dynamical divisibility sequence having a fixed G.C.D. with their indices . New York J. Math. 28(2022), 1152–1171.Google Scholar
Jha, A. and Nath, A., The distribution of G.C.D.s of shifted primes and Lucas sequences. Preprint, 2022. arXiv:2207.00825Google Scholar
Jha, A. and Sanna, C., Greatest common divisors of shifted primes and Fibonacci numbers . Res. Number Theory 8(2022), no. 4, Article no. 65.CrossRefGoogle Scholar
Kim, S., The density of the terms in an elliptic divisibility sequence having a fixed G.C.D. with their indices . J. Number Theory 207(2020), 22–41, with an appendix by M. Ram Murty.CrossRefGoogle Scholar
Leonetti, P. and Sanna, C., On the greatest common divisor of $n$ and the $n$ th Fibonacci number . Rocky Mountain J. Math. 48(2018), no. 4, 1191–1199.CrossRefGoogle Scholar
Luca, F. and Tron, E., The distribution of self-Fibonacci divisors . In: Advances in the theory of numbers, Fields Institute Communication, 77, Fields Institute for Research in Mathematical Sciences, Toronto, ON, 2015, pp. 149–158.CrossRefGoogle Scholar
Mastrostefano, D., An upper bound for the moments of a GCD related to Lucas sequences . Rocky Mountain J. Math. 49(2019), no. 3, 887–902.CrossRefGoogle Scholar
Mastrostefano, D. and Sanna, C., On numbers $n$ with polynomial image coprime with the $n$ th term of a linear recurrence . Bull. Aust. Math. Soc. 99(2019), no. 1, 23–33.CrossRefGoogle Scholar
Moree, P., On primes $p$ for which $d$ divides ${ord}_p(g)$ . Funct. Approx. Comment. Math. 33(2005), 85–95.CrossRefGoogle Scholar
OEIS Foundation Inc., The On-Line Encyclopedia of Integer Sequences, 2022, published electronically at https://oeis.org Google Scholar
Pollack, P., Numbers which are orders only of cyclic groups . Proc. Amer. Math. Soc. 150(2022), no. 2, 515–524.CrossRefGoogle Scholar
Pomerance, C., On the distribution of amicable numbers . J. Reine Angew. Math. 293(1977), no. 294, 217–222.Google Scholar
Renault, M., The period, rank, and order of the $\left(a,b\right)$ – Fibonacci sequence $\mathit{\mathsf{\operatorname{mod}}}\;m$ . Math. Mag. 86(2013), no. 5, 372–380.CrossRefGoogle Scholar
Ribenboim, P., My numbers, my friends: popular lectures on number theory, Springer, New York, 2000.CrossRefGoogle Scholar
Sanna, C., On numbers $n$ dividing the $n$ th term of a Lucas sequence . Int. J. Number Theory 13(2017), no. 3, 725–734.CrossRefGoogle Scholar
Sanna, C., The moments of the logarithm of a G.C.D. related to Lucas sequences . J. Number Theory 191(2018), 305–315.CrossRefGoogle Scholar
Sanna, C., On numbers $n$ relatively prime to the $n$ th term of a linear recurrence . Bull. Malays. Math. Sci. Soc. 42(2019), no. 2, 827–833.CrossRefGoogle Scholar
Sanna, C., On the divisibility of the rank of appearance of a Lucas sequence . Int. J. Number Theory 18(2022), no. 10, 2145–2156.CrossRefGoogle Scholar
Sanna, C. and Tron, E., The density of numbers $n$ having a prescribed G.C.D. with the $n$ th Fibonacci number . Indag. Math. (N.S.) 29(2018), no. 3, 972–980.CrossRefGoogle Scholar
Silverman, J. H., Generalized greatest common divisors, divisibility sequences, and Vojta’s conjecture for blowups . Monatsh. Math. 145(2005), no. 4, 333–350.CrossRefGoogle Scholar
Somer, L., Divisibility of terms in Lucas sequences by their subscripts . In: Applications of Fibonacci numbers (St. Andrews, 1992). Vol. 5, Kluwer Academic Publishers, Dordrecht, 1993, pp. 515–525.CrossRefGoogle Scholar
Tenenbaum, G., Introduction to analytic and probabilistic number theory, 3rd ed., Graduate Studies in Mathematics, 163, American Mathematical Society, Providence, RI, 2015, translated from the 2008 French edition by Patrick D. F. Ion.CrossRefGoogle Scholar
Tron, E., The greatest common divisor of linear recurrences . Rend. Semin. Mat. Univ. Politec. Torino 78(2020), no. 1, 103–124.Google Scholar