No CrossRef data available.
Article contents
A Pólya–Vinogradov inequality for short character sums
Published online by Cambridge University Press: 02 December 2020
Abstract
In this paper, we obtain a variation of the Pólya–Vinogradov inequality with the sum restricted to a certain height. Assume 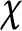 $\chi $
to be a primitive character modulo q,
$\chi $
to be a primitive character modulo q, 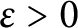 $ \epsilon>0$
and
$ \epsilon>0$
and 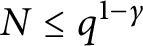 $N\le q^{1-\gamma }$
, with
$N\le q^{1-\gamma }$
, with 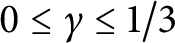 $0\le \gamma \le 1/3$
. We prove that
$0\le \gamma \le 1/3$
. We prove that 
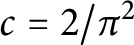 $c=2/\pi ^2$
if
$c=2/\pi ^2$
if 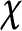 $\chi $
is even and
$\chi $
is even and 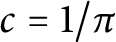 $c=1/\pi $
if
$c=1/\pi $
if 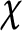 $\chi $
is odd. The result is based on the work of Hildebrand and Kerr.
$\chi $
is odd. The result is based on the work of Hildebrand and Kerr.
MSC classification
- Type
- Article
- Information
- Copyright
- © Canadian Mathematical Society 2020



