Hostname: page-component-f554764f5-rj9fg Total loading time: 0 Render date: 2025-04-13T20:45:22.051Z Has data issue: false hasContentIssue false
  • English
  • Français

An Analogue of the Multinomial Theorem

Published online by Cambridge University Press:  20 November 2018

T. V. Narayana
T. V. Narayana*
Affiliation:
University of Alberta and National Institute of Arthritis and Metabolic Diseases
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let xi, yi (i=1,2,…, t) and n be non-negative integers. A function (n; x1, …, xt) may be defined recursively as follows: let(0;0,…, 0)=1 and

1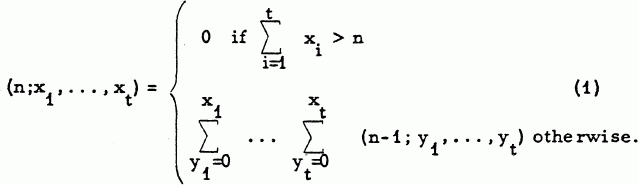

Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 5 , Issue 1 , January 1962 , pp. 43 - 50
Copyright
Copyright © Canadian Mathematical Society 1962

References

1. Feller, W., An introduction to probability theory and its applications, Vol. 1 (New York 1957).Google Scholar
2. Narayana, T. V., Sur les treillis formas par les partitions d'un entier; leurs applications a la théorie des probabilities. CR., vol. 240, pp. 1188-89 (1955).Google Scholar
3. Narayana, T. V. and Fulton, G. E., A note on the compositions of an integer. Can. Math. Bull. Vol. 1, No. 3, pp. 169-173, Sept. 1958.Google Scholar
4. Brainerd, B. and Narayana, T. V., A Note on Simple Binomial Sampling Plans. Annals of Math. Statistics, 32, (1961), 906-8.Google Scholar
Narayana, T. V. and Mohanty, S. G., Some properties of compositions and their applications to probability and statistics I, II (submitted to Biometrische Zeitschrift).Google Scholar