No CrossRef data available.
Article contents
On Deformations of the Complex Structure on the Moduli Space of Spatial Polygons
Published online by Cambridge University Press: 20 November 2018
Abstract
For an integer 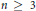 $n\,\ge \,3$, let
$n\,\ge \,3$, let 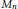 ${{M}_{n}}$ be the moduli space of spatial polygons with
${{M}_{n}}$ be the moduli space of spatial polygons with  $n$ edges. We consider the case of odd
$n$ edges. We consider the case of odd  $n$. Then
$n$. Then 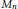 ${{M}_{n}}$ is a Fano manifold of complex dimension
${{M}_{n}}$ is a Fano manifold of complex dimension 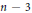 $n\,-\,3$. Let
$n\,-\,3$. Let 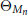 ${{\Theta }_{{{M}_{n}}}}$ be the sheaf of germs of holomorphic sections of the tangent bundle
${{\Theta }_{{{M}_{n}}}}$ be the sheaf of germs of holomorphic sections of the tangent bundle 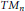 $T{{M}_{n}}$. In this paper, we prove
$T{{M}_{n}}$. In this paper, we prove  ${{H}^{q}}\left( {{M}_{n}},\,{{\Theta }_{{{M}_{n}}}} \right)\,=\,0$ for all
${{H}^{q}}\left( {{M}_{n}},\,{{\Theta }_{{{M}_{n}}}} \right)\,=\,0$ for all 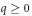 $q\,\ge \,0$ and all odd
$q\,\ge \,0$ and all odd  $n$. In particular, we see that the moduli space of deformations of the complex structure on
$n$. In particular, we see that the moduli space of deformations of the complex structure on 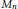 ${{M}_{n}}$ consists of a point. Thus the complex structure on
${{M}_{n}}$ consists of a point. Thus the complex structure on 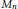 ${{M}_{n}}$ is locally rigid.
${{M}_{n}}$ is locally rigid.
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 2002


