Hostname: page-component-78c5997874-t5tsf Total loading time: 0 Render date: 2024-11-16T13:10:45.018Z Has data issue: false hasContentIssue false
  • English
  • Français

On the Gras Conjecture for Imaginary Quadratic Fields

Published online by Cambridge University Press:  20 November 2018

Hassan Oukhaba  and
Stéphane Viguié
Hassan Oukhaba
Affiliation:
Laboratoire de mathématique, 16 Route de Gray, 25030 Besançon cedex, France e-mail: houkhaba@univ-fcomte.fr; sviguie@univ-fcomte.fr
Stéphane Viguié
Affiliation:
Laboratoire de mathématique, 16 Route de Gray, 25030 Besançon cedex, France e-mail: houkhaba@univ-fcomte.fr; sviguie@univ-fcomte.fr
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

In this paper we extend K. Rubin's methods to prove the Gras conjecture for abelian extensions of a given imaginary quadratic field 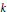$k$ and prime numbers 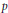$p$ that divide the number of roots of unity in 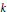$k$.

Keywords

11R2711R2911G16elliptic unitsStark unitsGras conjectureEuler systems
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 56 , Issue 1 , 01 March 2013 , pp. 148 - 160
Copyright
Copyright © Canadian Mathematical Society 2013

References

[1] Gillard, R., Remarques sur les unités cyclotomiques et les unités elliptiques. J. Number Theory 11 (1979), no. 1, 21–48. http://dx.doi.org/10.1016/0022-314X(79)90018-0 Google Scholar
[2] Oukhaba, H. and Viguié, S., The Gras conjecture in function fields by Euler systems. Bull. London Math. Soc. 43 (2011), no. 3, 523–535. http://dx.doi.org/10.1112/blms/bdq119 Google Scholar
[3] Robert, G., Unités de Stark comme unités elliptiques. Prépublication de l’institut Fourier, no. 143, 1989.Google Scholar
[4] Oukhaba, H. and Viguié, S., Concernant la relation de distribution satisfaite par la fonction ‘ associée à un réseau complexe. Invent. Math. 100 (1990), no. 2, 231–257. http://dx.doi.org/10.1007/BF01231186 Google Scholar
[5] Oukhaba, H. and Viguié, S., Unités de Stark et racine 12-i`eme canonique. Prépublication de l’institut Fourier, no. 181, 1991.Google Scholar
[6] Oukhaba, H. and Viguié, S., La racine 12-iéme canoniqueΔ(L)[L: L]/Δ(L). In: Séminaire de Théorie des Nombres, Paris, 1989–90, Progr. Math., 102, Birköuser Boston, Boston, MA, 1992, pp. 209–232.Google Scholar
[7] Rubin, K., Global units and ideal class groups. Invent. Math. 89 (1987), no. 3, 511–526. http://dx.doi.org/10.1007/BF01388983 Google Scholar
[8] Rubin, K., The “main conjectures” of Iwasawa theory for imaginary quadratic fields. Invent. Math. 103 (1991), no. 1, 25–68. http://dx.doi.org/10.1007/BF01239508 Google Scholar
[9] Rubin, K., More “main conjectures” for imaginary quadratic fields. In: Elliptic curves and related topics, CRM Proc. Lecture Notes, 4, American Mathematical Society, Providence, RI, 1994, pp. 23–28.Google Scholar
[10] Sinnott, W., On the Stickelberger ideal and the circular units of an abelian field. Invent. Math. 62 (1980/81), no. 2, 181–234. http://dx.doi.org/10.1007/BF01389158 Google Scholar
[11] Stark, H. M., L-functions at s = 1 . IV. First derivatives at s = 0. Adv. in Math. 35 (1980), no. 3, 197–235. http://dx.doi.org/10.1016/0001-8708(80)90049-3 Google Scholar
[12] Tate, J., Les conjectures de Stark sur les fonctions L d’Artin en s= 0. Lecture notes edited by Dominique Bernardi and Norbert Schappacher, Progress in Mathematics, 47, Birkhäuser Boston, Inc., Boston, MA, 1984.Google Scholar
[13] Viguié, S., Index-modules and applications. Manuscripta Math. Published online April 6, 2011. http://dx.doi.org/10.1007/s00229-011-0452-y Google Scholar