Hostname: page-component-78c5997874-m6dg7 Total loading time: 0 Render date: 2024-11-19T09:20:02.044Z Has data issue: false hasContentIssue false
  • English
  • Français

Sets of Uniqueness for Univalent Functions

Published online by Cambridge University Press:  20 November 2018

Marius Overholt
Marius Overholt*
Affiliation:
Department of Mathematics and Statistics University of Tromsø N-9037 Tromsø Norway, email: marius@math.uit.no
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We observe that any set of uniqueness for the Dirichlet space 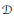$\mathcal{D}$ is a set of uniqueness for the class 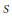$S$ of normalized univalent holomorphic functions.

Keywords

30C5530C15
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 43 , Issue 1 , 01 March 2000 , pp. 105 - 107
Copyright
Copyright © Canadian Mathematical Society 2000

References

[1] Bogdan, K., On the zeros of functions with finite Dirichlet integral. Kodai Math. J. 19 (1996), 7–16.Google Scholar
[2] Caughran, J. G., Two results concerning the zeros of functions with finite Dirichlet integral. Canad. J. Math. 21 (1969), 312–316.Google Scholar
[3] Duren, P. L., Univalent Functions. Springer-Verlag, New York, 1983.Google Scholar
[4] Duren, P. L., Problem 6.83. In: Research Problems in Complex Analysis (Eds. K. F. Barth, D. A. Brannan and W. K. Hayman), Bull. Lond.Math. Soc. 16 (1984), 490–517.Google Scholar
[5] Lappan, P., Points Where Univalent Functions May Coincide. Complex Variables 5 (1985), 17–20.Google Scholar
[6] Nagel, A., Rudin, W. and Shapiro, J. H., Tangential boundary behavior of functions in Dirichlet-type spaces. Ann. of Math. 116 (1982), 331–360.Google Scholar
[7] Obrock, A., Problem 2.2. In: Problems in Complex Function Theory (Ed. Ch. Pommerenke), Bull. Lond. Math. Soc. 4 (1972), 354–366.Google Scholar
[8] Shapiro, H. S. and Shields, A. L., On the zeros of functions with finite Dirichlet integral and some related function spaces. Math. Z. 80 (1962), 217–229.Google Scholar
[9] Stegbuchner, H., Carleson sets and fixed-points of schlicht functions. Romanian-Finnish Seminar in Complex Analysis (Proc., Bucharest, 1976), 373–388, Lecture Notes in Math. 743, Springer-Verlag, Berlin, 1979.Google Scholar