Hostname: page-component-cd9895bd7-dzt6s Total loading time: 0 Render date: 2024-12-27T07:54:14.127Z Has data issue: false hasContentIssue false
  • English
  • Français

Square-Reduced Residue Systems (Mod r) and Related Arithmetical Functions

Published online by Cambridge University Press:  20 November 2018

R. Sivaramakrishnan
R. Sivaramakrishnan*
Affiliation:
Department of Mathematics, University of Calicut, Kerala 673635, India
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We define a square-reduced residue system (mod r) as the set of integers a (mod r) such that the greatest common divisor of a and r, denoted by (a, r), is a perfect square ≥ 1 and contained in a residue system (mod r). This leads to a Class-division of integers (mod r) based on the 'square-free' divisors of r. The number of elements in a square-reduced residue system (mod r) is denoted by b(r). It is shown that

(1)

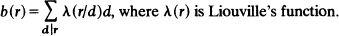
(2)

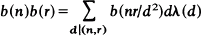
In view of (2), b(r) is said to be 'specially multiplicative'. The exponential sum associated with a square-reduced residue system (mod r) is defined by

where the summation is over a square-reduced residue system (mod r).

B(n, r) belongs to a new class of multiplicative functions known as 'Quasi-symmetric functions' and

(3)

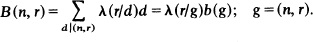
As an application, the sum is considered in terms of the Cauchy-composition of even functions (mod r). It is found to be multiplicative in r. The evaluation of the above sum gives an identity involving Pillai's arithmetic function

Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 22 , Issue 2 , 01 June 1979 , pp. 207 - 220
Copyright
Copyright © Canadian Mathematical Society 1979

References

1. Cohen, Eckford, Arithmetical functions associated with the unitary divisors of an integer, Math. Zeitschr 74 (1960)66-80.Google Scholar
2. Cohen, Eckford, A class of arithmetical functions, Proc. Nat. Acad. Sa, 41 (1955)939-944.Google Scholar
3. Cohen, Eckford, Representations of Even Functions (mod r) I Arithmetical Identities, Duke Math. J., 25 (1958)401-422.Google Scholar
4. Cohen, Eckford, Representations of Even Functions (mod r) II Cauchy Products, Duke Math. J., 26 (1959)165-182.Google Scholar
5. Hardy, G. H. and Wright, E. M., An Introduction to the Theory of Numbers, ELBS and Oxford University Press (1971 Edition).Google Scholar
6. Menon, P.Kesava, On the Sum Σ(a, n)=1 (a - 1, n), J. Ind. Math. Soc. (New Series) 29 (1963)155-163.Google Scholar
7. Sivaramakrishnan, R., Contributions to the study of multiplicative arithmetic functions, Ph.D Thesis University of Kerala (1971).Google Scholar
8. Sivaramakrishnan, R., On a class of multiplicative arithmetic functions, J. Reine. Angew. Math., 280 (1976)157-162.Google Scholar
9. Sivaramakrishnan, R., Multiplicative Even Functions (mod r) I Structural properties, J. Reine. Angew. Math. 302 (1978).Google Scholar
10. Pillai, S. S., On an arithmetic function, J. Annamalai University II No. 2 243-248.Google Scholar
11. Venkataraman, C. S., Modular Multiplicative Functions, J. Madras University XIX (1950)69-78.Google Scholar
12. Venkataraman, C. S., A new Identical Equation for multiplicative functions of two arguments and its applications to Ramanujan's Sum CM(N), Proc. Ind. Acad. Sa, XXIV No. 6 Sec. A (1946) 518-529.Google Scholar
13. Venkataraman, C. S., On Von Sterneck Ramanujan Function, J. Ind. Math. Soc. (New Series) 13 65-72 (1949).Google Scholar