Hostname: page-component-cd9895bd7-dk4vv Total loading time: 0 Render date: 2024-12-22T14:19:36.141Z Has data issue: false hasContentIssue false
  • English
  • Français

Glicci ideals

Part of: Special varieties Theory of modules and ideals

Published online by Cambridge University Press:  28 June 2013

Juan Migliore  and
Uwe Nagel
Juan Migliore
Affiliation:
Department of Mathematics, University of Notre Dame, Notre Dame, IN 46556, USA email migliore.1@nd.edu
Uwe Nagel
Affiliation:
Department of Mathematics, University of Kentucky, 715 Patterson Office Tower, Lexington, KY 40506-0027, USA email uwe.nagel@uky.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

A central problem in liaison theory is to decide whether every arithmetically Cohen–Macaulay subscheme of projective $n$-space can be linked by a finite number of arithmetically Gorenstein schemes to a complete intersection. We show that this can indeed be achieved if the given scheme is also generically Gorenstein and we allow the links to take place in an 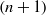$(n+ 1)$-dimensional projective space. For example, this result applies to all reduced arithmetically Cohen–Macaulay subschemes. We also show that every union of fat points in projective 3-space can be linked in the same space to a union of simple points in finitely many steps, and hence to a complete intersection in projective 4-space.

Keywords

linkageliaisonGorenstein subschemearithmetically Cohen–Macaulay subschemereduced schemefat pointsglicci

MSC classification

Secondary: 13C40: Linkage, complete intersections and determinantal ideals 14M05: Varieties defined by ring conditions (factorial, Cohen-Macaulay, seminormal) 14M06: Linkage
Type
Research Article
Information
Compositio Mathematica , Volume 149 , Issue 9 , September 2013 , pp. 1583 - 1591
Copyright
© The Author(s) 2013 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Bruns, W. and Herzog, J., Cohen–Macaulay rings, Cambridge Studies in Advanced Mathematics, vol. 39 (Cambridge University Press, Cambridge, 1998).CrossRefGoogle Scholar
Casanellas, M., Drozd, E. and Hartshorne, R., Gorenstein liaison and ACM sheaves, J. Reine Angew. Math. 584 (2005), 149–171.CrossRefGoogle Scholar
Gorla, E., A generalized Gaeta’s theorem, Compositio Math. 144 (2008), 689–704.CrossRefGoogle Scholar
Hartshorne, R., Some examples of Gorenstein liaison in codimension three, Collect. Math. 53 (2002), 21–48.Google Scholar
Hartshorne, R., Sabadini, I. and Schlesinger, E., Codimension 3 arithmetically Gorenstein subschemes of projective $N$-space, Ann. Inst. Fourier (Grenoble) 58 (2008), 2037–2073.Google Scholar
Huneke, C. and Ulrich, B., The structure of linkage, Ann. of Math. (2) 126 (1987), 221–275.CrossRefGoogle Scholar
Huneke, C. and Ulrich, B., Liaison of monomial ideals, Bull. Lond. Math. Soc. 39 (2007), 384–392.CrossRefGoogle Scholar
Kleppe, J., Migliore, J., Miró-Roig, R. M., Nagel, U. and Peterson, C., Gorenstein liaison, complete intersection liaison invariants and unobstructedness, Mem. Amer. Math. Soc. 154 (2001), no. 732.Google Scholar
Migliore, J., Introduction to liaison theory and deficiency modules, Progress in Mathematics, vol. 165 (Birkhäuser, Boston, 1998).Google Scholar
Migliore, J. and Nagel, U., Lifting monomial ideals, Comm. Algebra 28 (2000), 5679–5701.CrossRefGoogle Scholar
Migliore, J. and Nagel, U., Monomial ideals and the Gorenstein liaison class of a complete intersection, Compositio Math. 133 (2002), 25–36.CrossRefGoogle Scholar
Migliore, J. and Nagel, U., Reduced arithmetically Gorenstein schemes and simplicial polytopes with maximal Betti numbers, Adv. Math. 180 (2003), 1–63.CrossRefGoogle Scholar
Nagel, U. and Römer, T., Glicci simplicial complexes, J. Pure Appl. Algebra 212 (2008), 2250–2258.Google Scholar
Polini, C. and Ulrich, B., Linkage and reduction numbers, Math. Ann. 310 (1998), 631–651.CrossRefGoogle Scholar
Rao, P., Liaison among curves in ${ \mathbb{P} }^{3} $, Invent. Math. 50 (1979), 205–217.CrossRefGoogle Scholar