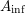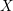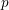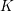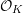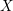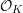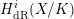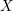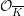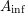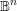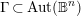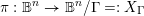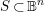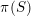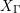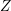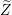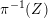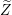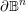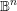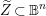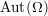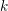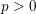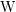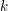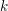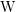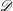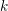For a proper, smooth scheme X$X$ over a p$p$-adic field K$K$, we show that any proper, flat, semistable {\mathcal{O}}_{K}${\mathcal{O}}_{K}$-model {\mathcal{X}}${\mathcal{X}}$ of X$X$ whose logarithmic de Rham cohomology is torsion free determines the same {\mathcal{O}}_{K}${\mathcal{O}}_{K}$-lattice inside H_{\text{dR}}^{i}(X/K)$H_{\text{dR}}^{i}(X/K)$ and, moreover, that this lattice is functorial in X$X$. For this, we extend the results of Bhatt–Morrow–Scholze on the construction and the analysis of an A_{\text{inf}}$A_{\text{inf}}$-valued cohomology theory of p$p$-adic formal, proper, smooth {\mathcal{O}}_{\overline{K}}${\mathcal{O}}_{\overline{K}}$-schemes \mathfrak{X}$\mathfrak{X}$ to the semistable case. The relation of the A_{\text{inf}}$A_{\text{inf}}$-cohomology to the p$p$-adic étale and the logarithmic crystalline cohomologies allows us to reprove the semistable conjecture of Fontaine–Jannsen.

We prove the analogue of the Ax–Lindemann–Weierstrass theorem for not necessarily arithmetic lattices of the automorphism group of the complex unit ball 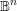\mathbb{B}^{n}$\mathbb{B}^{n}$ using methods of several complex variables, algebraic geometry and Kähler geometry. Consider a torsion-free lattice 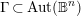\unicode[STIX]{x1D6E4}\,\subset \,\text{Aut}(\mathbb{B}^{n})$\unicode[STIX]{x1D6E4}\,\subset \,\text{Aut}(\mathbb{B}^{n})$ and the associated uniformization map 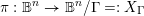\unicode[STIX]{x1D70B}:\mathbb{B}^{n}\rightarrow \mathbb{B}^{n}/\unicode[STIX]{x1D6E4}=:X_{\unicode[STIX]{x1D6E4}}$\unicode[STIX]{x1D70B}:\mathbb{B}^{n}\rightarrow \mathbb{B}^{n}/\unicode[STIX]{x1D6E4}=:X_{\unicode[STIX]{x1D6E4}}$. Given an algebraic subset 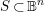S\,\subset \,\mathbb{B}^{n}$S\,\subset \,\mathbb{B}^{n}$ and writing Z$Z$ for the Zariski closure of 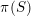\unicode[STIX]{x1D70B}(S)$\unicode[STIX]{x1D70B}(S)$ in 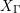X_{\unicode[STIX]{x1D6E4}}$X_{\unicode[STIX]{x1D6E4}}$ (which is equipped with a canonical quasi-projective structure), in some precise sense we realize 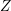Z$Z$ as a variety uniruled by images of algebraic subsets under the uniformization map, and study the asymptotic geometry of an irreducible component 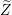\widetilde{Z}$\widetilde{Z}$ of 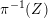\unicode[STIX]{x1D70B}^{-1}(Z)$\unicode[STIX]{x1D70B}^{-1}(Z)$ as 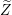\widetilde{Z}$\widetilde{Z}$ exits the boundary 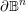\unicode[STIX]{x2202}\mathbb{B}^{n}$\unicode[STIX]{x2202}\mathbb{B}^{n}$ by exploiting the strict pseudoconvexity of 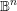\mathbb{B}^{n}$\mathbb{B}^{n}$, culminating in the proof that 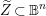\widetilde{Z}\,\subset \,\mathbb{B}^{n}$\widetilde{Z}\,\subset \,\mathbb{B}^{n}$ is totally geodesic. Our methodology sets the stage for tackling problems in functional transcendence theory for arbitrary lattices of 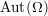\text{ Aut}(\unicode[STIX]{x1D6FA})$\text{ Aut}(\unicode[STIX]{x1D6FA})$ for (possibly reducible) bounded symmetric domains \unicode[STIX]{x1D6FA}$\unicode[STIX]{x1D6FA}$.

We describe generators of disguised residual intersections in any commutative Noetherian ring. It is shown that, over Cohen–Macaulay rings, the disguised residual intersections and algebraic residual intersections are the same, for ideals with sliding depth. This coincidence provides structural results for algebraic residual intersections in a quite general setting. It is shown how the DG-algebra structure of Koszul homologies affects the determination of generators of residual intersections. It is shown that the Buchsbaum–Eisenbud family of complexes can be derived from the Koszul–Čech spectral sequence. This interpretation of Buchsbaum–Eisenbud families has a crucial rule to establish the above results.

Let k$k$ be a perfect field of characteristic p>0$p>0$ and let \operatorname{W}$\operatorname{W}$ be the ring of Witt vectors of k$k$. In this article, we give a new proof of the Frobenius descent for convergent isocrystals on a variety over k$k$ relative to \operatorname{W}$\operatorname{W}$. This proof allows us to deduce an analogue of the de Rham complexes comparison theorem of Berthelot [\mathscr{D}$\mathscr{D}$-modules arithmétiques. II. Descente par Frobenius, Mém. Soc. Math. Fr. (N.S.) 81 (2000)] without assuming a lifting of the Frobenius morphism. As an application, we prove a version of Berthelot’s conjecture on the preservation of convergent isocrystals under the higher direct image by a smooth proper morphism of k$k$-varieties.

Gallagher’s theorem is a sharpening and extension of the Littlewood conjecture that holds for almost all tuples of real numbers. We provide a fibre refinement, solving a problem posed by Beresnevich, Haynes and Velani in 2015. Hitherto, this was only known on the plane, as previous approaches relied heavily on the theory of continued fractions. Using reduced successive minima in lieu of continued fractions, we develop the structural theory of Bohr sets of arbitrary rank, in the context of diophantine approximation. In addition, we generalise the theory and result to the inhomogeneous setting. To deal with this inhomogeneity, we employ diophantine transference inequalities in lieu of the three distance theorem.