Hostname: page-component-586b7cd67f-gb8f7 Total loading time: 0 Render date: 2024-11-24T19:31:00.020Z Has data issue: false hasContentIssue false
  • English
  • Français

A generalization of the Jarník–Besicovitch theorem by continued fractions

Published online by Cambridge University Press:  11 February 2015

BAO-WEI WANG ,
JUN WU  and
JIAN XU
BAO-WEI WANG
Affiliation:
School of Mathematics and Statistics, Huazhong University of Science and Technology, 430074 Wuhan, China email bwei_wang@hust.edu.cn, jun.wu@mail.hust.edu.cn, arielxj@hotmail.com
JUN WU
Affiliation:
School of Mathematics and Statistics, Huazhong University of Science and Technology, 430074 Wuhan, China email bwei_wang@hust.edu.cn, jun.wu@mail.hust.edu.cn, arielxj@hotmail.com
JIAN XU
Affiliation:
School of Mathematics and Statistics, Huazhong University of Science and Technology, 430074 Wuhan, China email bwei_wang@hust.edu.cn, jun.wu@mail.hust.edu.cn, arielxj@hotmail.com
Get access Rights & Permissions [Opens in a new window]

Abstract

We apply the tools of continued fractions to tackle the Diophantine approximation, including the classic Jarník–Besicovitch theorem, localized Jarník–Besicovitch theorem and its several generalizations. As is well known, the classic Jarník–Besicovitch sets, expressed in terms of continued fractions, can be written as

$$\begin{eqnarray}\{x\in [0,1):a_{n+1}(x)\geq e^{{\it\tau}(\log |T^{\prime }x|+\cdots +\log |T^{\prime }(T^{n-1}x)|)}~\text{for infinitely many}~n\in \mathbb{N}\},\end{eqnarray}$$
where $T$ is the Gauss map and $a_{n}(x)$ is the $n$th partial quotient of $x$. In this paper, we consider the size of the generalized Jarník–Besicovitch set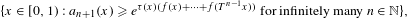
$$\begin{eqnarray}\{x\in [0,1):a_{n+1}(x)\geq e^{{\it\tau}(x)(f(x)+\cdots +f(T^{n-1}x))}~\text{for infinitely many}~n\in \mathbb{N}\},\end{eqnarray}$$
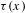 where ${\it\tau}(x)$ and 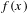$f(x)$ are positive functions defined on 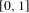$[0,1]$.

Type
Research Article
Information
Ergodic Theory and Dynamical Systems , Volume 36 , Issue 4 , June 2016 , pp. 1278 - 1306
Copyright
© Cambridge University Press, 2015 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Adamczewski, B. and Bugeaud, Y.. On the Littlewood conjecture in simultaneous Diophantine approximation. J. Lond. Math. Soc. 73 (2006), 355–366.Google Scholar
Barral, J. and Seuret, S.. A localized Jarnik-Bescovitch theorem. Adv. Math. 226 (2011), 3191–3215.Google Scholar
Besicovitch, A. S.. Sets of fractional dimension (IV): On rational approximation to real numbers. J. Lond. Math. Soc. 9 (1934), 126–131.CrossRefGoogle Scholar
Bosma, W., Dajani, K. and Kraaikamp, C.. Entropy quotients and correct digits in number-theoretic expansions. Dynamics & Stochastics: Festschrift in Honor of M. S. Keane (IMS Lecture Notes Monograph Series, 48). Eds. Denteneer, D., den Hollander, F. and Verbitskiy, E.. Institute of Mathematical Statistics, Beachwood, OH, 2006, pp. 176–188.Google Scholar
Bugeaud, Y.. Sets of exact approximation order by rational numbers. Math. Ann. 327 (2003), 171–190.CrossRefGoogle Scholar
Bugeaud, Y.. Approximation by Algebraic Numbers (Cambridge Tracts in Mathematics, 160). Cambridge University Press, Cambridge, 2004.CrossRefGoogle Scholar
Bugeaud, Y.. Sets of exact approximation order by rational numbers II. Unif. Distrib. Theory 3 (2008), 9–20.Google Scholar
Bugeaud, Y. and Moreira, C. G.. Sets of exact approximation order by rational numbers III. Acta Arith. 146 (2011), 177–193.CrossRefGoogle Scholar
Bumby, R. T.. Hausdorff dimensions of Cantor sets. J. Reine Angew. Math. 331 (1982), 192–206.Google Scholar
Cesaratto, E. and Vallée, B.. Hausdorff dimension of real numbers with bounded digits averages. Acta Arith. 125 (2006), 115–162.CrossRefGoogle Scholar
Cornfeld, I., Fomin, S. and Sinai, Y.. Ergodic Theory. Springer, New York, 1982.CrossRefGoogle Scholar
Falconer, K. J.. Fractal Geometry, Mathematical Foundations and Applications. Wiley, Chichester, 1990.Google Scholar
Good, I. J.. The fractional dimensional theory of continued fractions. Proc. Cambridge Philos. Soc. 37 (1941), 199–228.Google Scholar
Hanus, P., Mauldin, R. D. and Urbański, M.. Thermodynamic formalism and multifractal analysis of conformal infinite iterated function systems. Acta Math. Hungar. 96 (2002), 27–98.Google Scholar
Hardy, G. and Wright, E.. An Introduction to the Theory of Numbers, 5th edn. Clarendon Press, Oxford, 1979.Google Scholar
Hensley, D.. A polynomial time algorithm for the Hausdorff dimension of continued fraction Cantor sets. J. Number Theory 58 (1996), 9–45.CrossRefGoogle Scholar
Hirst, K. E.. A problem in the fractional dimension theory of continued fractions. Q. J. Math. Oxford Ser. (2) 21 (1970), 29–35.Google Scholar
Iosifescu, M. and Kraaikamp, C.. Metrical Theory of Continued Fractions (Mathematics and its Applications, 547). Kluwer Academic Publishers, Dordrecht, 2002.Google Scholar
Jarník, I.. Zur metrischen Theorie der diopahantischen Approximationen. Proc. Mat. Fyz. 36 (1928), 91–106.Google Scholar
Jarník, I.. Diophantische Approximationen und Hausdorffsches Mass. Mat. Sb. 36 (1929), 371–381.Google Scholar
Jarník, I.. Über die simultanen diophantischen Approximationen. Math. Z. 33 (1931), 503–543.CrossRefGoogle Scholar
Jenkinson, O. and Pollicott, M.. Computing the dimension of dynamically defined sets: E 2 and bounded continued fractions. Ergod. Th. & Dynam. Sys. 21(5) (2001), 1429–1445.CrossRefGoogle Scholar
Kesseböhmer, M. and Zhu, S.. Dimension sets for infinite IFSs: Texan conjecture. J. Number Theory 116 (2006), 230–246.Google Scholar
Khintchine, A. Ya.. Continued Fractions. P. Noordhoff, Groningen, 1963.Google Scholar
Li, B., Wang, B. W., Wu, J. and Xu, J.. The shrinking target problem in the dynamical system of continued fractions. Proc. Lond. Math. Soc. (3) 108(1) (2014), 159–186.Google Scholar
Lúczak, T.. On the fractional dimension of sets of continued fractions. Mathematika 44 (1997), 50–53.Google Scholar
Mauldin, R. D. and Urbański, M.. Dimensions and measures in infinite iterated function systems. Proc. London Math. Soc. (3) 73 (1996), 105–154.Google Scholar
Mauldin, R. D. and Urbański, M.. Conformal iterated function systems with applications to the geometry of continued fractions. Trans. Amer. Math. Soc. 351(12) (1999), 4995–5025.Google Scholar
Mauldin, R. D. and Urbański, M.. Graph directed Markov systems. Geometry and Dynamics of Limit Sets (Cambridge Tracts in Mathematics, 148). Cambridge University Press, Cambridge, 2003.Google Scholar
Pollington, A. and Velani, S.. On a problem in simultaneous Diophantine approximation: Littlewood’s conjecture. Acta Math. 185 (2000), 287–306.Google Scholar
Schmidt, W. M.. Diophantine Approximation (Lecture Notes in Mathematics, 785). Springer, Berlin, 1980.Google Scholar
Schweiger, F.. Ergodic Theory of Fibred Systems and Metirc Number Theory. Clarendon Press, Oxford, 1995.Google Scholar
Walters, P.. An Introduction to Ergodic Theory (GTM, 79). Springer, New York, 1982.Google Scholar
Wang, B. W. and Wu, J.. Hausdorff dimension of certain sets arising in continued fraction expansions. Adv. Math. 218 (2008), 1319–1339.Google Scholar
Wang, B. W. and Wu, J.. A problem of Hirst on continued fraction with sequences of partial quotients. Bull. Lond. Math. Soc. 40 (2008), 18–22.Google Scholar
Wu, J.. Continued fraction and decimal expansions of an irrational number. Adv. Math. 206 (2006), 684–694.Google Scholar
Wu, J. and Xu, J.. The distribution of the largest digit in continued fraction expansions. Math. Proc. Cambridge Philos. Soc. 146 (2009), 207–212.Google Scholar