Hostname: page-component-586b7cd67f-r5fsc Total loading time: 0 Render date: 2024-11-22T22:23:25.006Z Has data issue: false hasContentIssue false
  • English
  • Français

On twisted group 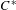$C^{\ast }$ -algebras associated with FC-hypercentral groups and other related groups

Published online by Cambridge University Press:  01 June 2015

ERIK BÉDOS  and
TRON OMLAND
ERIK BÉDOS
Affiliation:
Institute of Mathematics, University of Oslo, P.B. 1053 Blindern, 0316 Oslo, Norway email bedos@math.uio.no
TRON OMLAND
Affiliation:
School of Mathematical and Statistical Sciences, Arizona State University, PO Box 871804, Tempe, AZ 85287-1804, USA email omland@asu.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

We show that the twisted group $C^{\ast }$ -algebra associated with a discrete FC-hypercentral group is simple (respectively, has a unique tracial state) if and only if Kleppner’s condition is satisfied. This generalizes a result of Packer for countable nilpotent groups. We also consider a larger class of groups, for which we can show that the corresponding reduced twisted group $C^{\ast }$ -algebras have a unique tracial state if and only if Kleppner’s condition holds.

Type
Research Article
Information
Ergodic Theory and Dynamical Systems , Volume 36 , Issue 6 , September 2016 , pp. 1743 - 1756
Copyright
© Cambridge University Press, 2015 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Bédos, E.. Discrete groups and simple C ∗ -algebras. Math. Proc. Cambridge Philos. Soc. 109 (1991), 521–537.Google Scholar
Bédos, E.. On the uniqueness of the trace of some simple C ∗ -algebras. J. Operator Theory 30 (1993), 149–160.Google Scholar
Bédos, E.. Notes on hypertraces and C ∗ -algebras. J. Operator Theory 34 (1995), 285–306.Google Scholar
Bédos, E. and Conti, R.. On maximal ideals in certain reduced twisted $C^{\ast }$ -crossed products. Math. Proc. Cambridge Philos. Soc. Available on CJO2015, doi:10.1017/S0305004115000031.Google Scholar
Bekka, M., Cowling, M. and de la Harpe, P.. Some groups whose reduced C ∗ -algebra is simple. Publ. Math. Inst. Hautes Études Sci. 80 (1994), 117–134.Google Scholar
Brown, N. P. and Ozawa, N.. C ∗ -algebras and Finite-Dimensional Approximations (Graduate Studies in Mathematics, 88) . American Mathematical Society, Providence, RI, 2008.Google Scholar
Carey, A. L. and Moran, W.. Characters on nilpotent groups. Math. Proc. Cambridge Philos. Soc. 96 (1984), 123–137.Google Scholar
Chou, C.. Elementary amenable groups. Illinois J. Math. 24 (1980), 396–407.Google Scholar
Echterhoff, S.. On maximal prime ideals in certain group C ∗ -algebras and crossed product algebras. J. Operator Theory 23 (1990), 317–338.Google Scholar
Exel, R.. Exact groups and Fell bundles. Math. Ann. 323 (2002), 259–266.Google Scholar
Gromov, M.. Groups of polynomial growth and expanding maps. Publ. Math. Inst. Hautes Études Sci. 53 (1981), 53–73.CrossRefGoogle Scholar
Haagerup, U. and Zsido, L.. Sur la propriété de Dixmier pour les C ∗ -algèbres. C. R. Acad. Sci. Paris 298 (1984), 173–177.Google Scholar
de la Harpe, P.. On simplicity of reduced group C ∗ -algebras. Bull. Lond. Math. Soc. 39 (2007), 1–26.Google Scholar
Isaacs, I. M.. Character Theory of Finite Groups. Academic Press, New York, 1976.Google Scholar
Jaworski, W.. Countable amenable identity excluding groups. Canad. Math. Bull. 47 (2004), 215–228.Google Scholar
Kleppner, A.. The structure of some induced representations. Duke Math. J. 29 (1962), 555–572.CrossRefGoogle Scholar
McLain, D. H.. Remarks on the upper central series of a group. Proc. Glasgow Math. Assoc. 3 (1956), 38–44.Google Scholar
Moore, C. C. and Rosenberg, J.. Groups with T1 primitive ideal space. J. Funct. Anal. 22 (1976), 204–224.Google Scholar
Murphy, G. J.. Uniqueness of the trace and simplicity. Proc. Amer. Math. Soc. 128 (2000), 3563–3570.Google Scholar
Omland, T.. Primeness and primitivity conditions for twisted group C ∗ -algebras. Math. Scand. 114 (2014), 299–319.Google Scholar
Ozawa, N.. Dixmier approximation and symmetric amenability for C ∗ -algebras. J. Math. Sci. Univ. Tokyo 20 (2013), 349–374.Google Scholar
Packer, J. A.. Twisted group C ∗ -algebras corresponding to nilpotent discrete groups. Math. Scand. 64 (1989), 109–122.CrossRefGoogle Scholar
Paterson, A.. Amenability (Mathematical Surveys and Monographs, 29) . American Mathematical Society, Providence, RI, 1988.Google Scholar
Promislow, S. D.. A class of groups producing simple, unique trace C ∗ -algebras. Math. Proc. Cambridge Philos. Soc. 114 (1993), 223–233.Google Scholar
Robinson, D. J. S.. Finiteness Conditions and Generalized Soluble Groups. Part 1. Springer, New York, 1972.Google Scholar
Slawny, J.. On factor representations and the C ∗ -algebra of canonical commutation relations. Comm. Math. Phys. 24 (1972), 151–170.Google Scholar
Zeller-Meier, G.. Produits croisés d’une C ∗ -algèbre par un groupe d’automorphismes. J. Math. Pures Appl. (9) 47 (1968), 101–239.Google Scholar