Hostname: page-component-84b7d79bbc-5lx2p Total loading time: 0 Render date: 2024-07-29T02:59:59.348Z Has data issue: false hasContentIssue false
  • English
  • Français

Metric properties of ε-trajectories of dynamical systems with stochastic behaviour

Published online by Cambridge University Press:  19 September 2008

M. L. Blank
M. L. Blank
Affiliation:
All-Union Research Centre of Cardiology, AMS, 3 Cherepkovsky Street 15, Moscow 121552, USSR
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

A highly developed branch of the modern theory of dynamical systems is the study of deterministic ones with statistical properties in behaviour. During the last decade such systems were discovered in various domains of physics, chemistry, biology and technology. It is due to their complexity, that only the simplest of such systems have been analytically investigated (Lorenz system, Rikitake dynamo, billiard systems), and that is why numerical methods are widely used, especially in applied investigations. In numerical modelling we have no true trajectory of a dynamical system f, but an approximation = (x1, x2,…) such that the sequence of distances 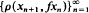is small in some sense. For the case of round off errors in computer modelling, such a sequence is uniformly small, i.e. there exists some ε > 0, such that supnρ(xn+1, fxn)<ε. The sequence in this case is called an ε-trajectory of the dynamical system f[1]. In a series of investigations [1–14] a study was made of the properties and applications of ε-trajectories.

Type
Research Article
Information
Ergodic Theory and Dynamical Systems , Volume 8 , Issue 3 , September 1988 , pp. 365 - 378
Copyright
Copyright © Cambridge University Press 1988

References

REFERENCES

[1]Anosov, D. V.. Geodesic flows on closed Riemannian manifolds with negative curvature. Tr. Institute of math. AN SSSR 90 (1967).Google Scholar
[2]Sinai, Y. G.. Gibbs measures in ergodic theory. Uspekhi Mat. Nauk 27(4) (1972), 21–64.Google Scholar
[3]Sinai, Y. G.. Stochasticity of dynamical systems. In: Nonlinear Waves Nauka: Moscow, 1979.Google Scholar
[4]Bowen, R.. Methods of Symbolic Dynamics Mir: Moscow, 1979.Google Scholar
[5]Nitecki, Z.. Introduction to Differential Dynamics Mir: Moscow, 1975.Google Scholar
[6]Sigmund, K.. Generic properties of invariant measures for Axiom A diffeomorphisms. Inventiones Math. 11 (1970), 99–109.CrossRefGoogle Scholar
[7]Sigmund, K.. On dynamical systems with the specification property. Trans. Amer. Math. Soc. 190 (1978), 285–299.CrossRefGoogle Scholar
[8]Komuro, M.. Lorenz attractors do not have the pseudo-orbit tracing property. J. Math. Soc. Jap. 37(3) (1985), 479–514.Google Scholar
[9]Kato, K.. Pseudo-orbit and stability of flows. Mem. Fac. Sci. Kochi Univ. (Math.) 5 (1984), 49–62.Google Scholar
[10]Kato, K. & Morimoto, A.. Topological Ω stability of Axiom A flows with no Ω-explosions. J Diff. Eq. 34 (1979), 464–481.Google Scholar
[11]Morimoto, A.. The method of pseudo-orbit tracing and stability of dynamical systems. Seminar Note, 1979, 39.Google Scholar
[12]Walters, P.. On pseudo-orbit tracing property and its relationship to stability. Lect. Notes Math. 668 (1978), 291–294.Google Scholar
[13]Boyarsky, A.. On the significance of absolutely continuous invariant measures. Physica 11D (1984), 130–146.Google Scholar
[14]Boyarsky, A.. Computer orbits. Preprint Concordia Univ., 1984.Google Scholar
[15]Kifer, Yu. I.. Small random perturbations of some smooth dynamical systems. Izv. AN SSSR, Ser. Mat. 38(5) (1974), 1091–1115.Google Scholar
[16]Keller, G.. Stochastic stability in some chaotic dynamical systems. Monatsh. Math. 94 (1982), 313–333.CrossRefGoogle Scholar
[17]Parry, W.. Intrinsic Markov chains. Trans. Amer. Math. Soc. 112(1) (1964), 55–66.CrossRefGoogle Scholar
[18]Blank, M. L.. Small perturbations and stabilization of unstable trajectories. Dept. VINITI, 2201–85, 1985, 38 pp.Google Scholar
[19]Blank, M. L.. Ergodic properties of discretizations of dynamical systems. Dokl. AN SSSR 278(4) (1984), 779–782.Google Scholar
[20]Doob, J. L.. Stochastic Processes IL: Moscow, 1956.Google Scholar
[21]Yosida, K.. Functional Analysis Mir: Moscow, 1979.Google Scholar
[22]Blank, M. L.. On the conjugacy of a certain class of homeomorphisms to the class of piecewise monotonic maps. Russian Math. Surveys 40(1) (1985), 211–212.Google Scholar