Research Article
Andrei Nikolaevich Kolmogorov
-
- Published online by Cambridge University Press:
- 19 September 2008, pp. 493-499
-
- Article
-
- You have access
- Export citation
Minimal flows of finite almost periodic rank
-
- Published online by Cambridge University Press:
- 19 September 2008, pp. 155-172
-
- Article
-
- You have access
- Export citation
Dynamics induced on the ends of non-compact manifold
-
- Published online by Cambridge University Press:
- 19 September 2008, pp. 1-15
-
- Article
-
- You have access
- Export citation
A method for constructing attractors
-
- Published online by Cambridge University Press:
- 19 September 2008, pp. 331-349
-
- Article
-
- You have access
- Export citation
Other
Charles C. Conley, 1933–1984
-
- Published online by Cambridge University Press:
- 10 December 2009, pp. 1-7
-
- Article
-
- You have access
- Export citation
Research Article
Duality for automorphisms on a compact C*-dynamical system
-
- Published online by Cambridge University Press:
- 19 September 2008, pp. 173-189
-
- Article
-
- You have access
- Export citation
Joint ergodicity for group actions
-
- Published online by Cambridge University Press:
- 19 September 2008, pp. 351-364
-
- Article
-
- You have access
- Export citation
Other
Some comments on ‘The gradient structure of a flow: I’
-
- Published online by Cambridge University Press:
- 10 December 2009, pp. 9-10
-
- Article
-
- You have access
- Export citation
Research Article
The speed interval: a rotation algorithm for endomorphisms of the circle
-
- Published online by Cambridge University Press:
- 19 September 2008, pp. 17-33
-
- Article
-
- You have access
- Export citation
About A. N. Kolmogorov's work on the entropy of dynamical systems
-
- Published online by Cambridge University Press:
- 19 September 2008, pp. 501-502
-
- Article
-
- You have access
- Export citation
Metric properties of ε-trajectories of dynamical systems with stochastic behaviour
-
- Published online by Cambridge University Press:
- 19 September 2008, pp. 365-378
-
- Article
-
- You have access
- Export citation
Infinite limits in the iteration of entire functions
-
- Published online by Cambridge University Press:
- 19 September 2008, pp. 503-507
-
- Article
-
- You have access
- Export citation
Some compact invariant sets for hyperbolic linear automorphisms of torii
-
- Published online by Cambridge University Press:
- 19 September 2008, pp. 191-204
-
- Article
-
- You have access
- Export citation
The gradient structure of a flow: I
-
- Published online by Cambridge University Press:
- 10 December 2009, pp. 11-26
-
- Article
-
- You have access
- Export citation
Points génériques de Champernowne sur certains systèmes codes; application aux θ-shifts
-
- Published online by Cambridge University Press:
- 19 September 2008, pp. 35-51
-
- Article
-
- You have access
- Export citation
An invariant for rigid rank-1 transformations
-
- Published online by Cambridge University Press:
- 19 September 2008, pp. 53-72
-
- Article
-
- You have access
- Export citation
Rank two interval exchange transformations*
-
- Published online by Cambridge University Press:
- 19 September 2008, pp. 379-394
-
- Article
-
- You have access
- Export citation
A priori bounds for periodic solutions of a class of Hamiltonian systems
-
- Published online by Cambridge University Press:
- 10 December 2009, pp. 27-31
-
- Article
-
- You have access
- Export citation
The attracting centre of a continuous self-map of the interval
-
- Published online by Cambridge University Press:
- 19 September 2008, pp. 205-213
-
- Article
-
- You have access
- Export citation
Rotation shadowing properties of circle and annulus maps
-
- Published online by Cambridge University Press:
- 19 September 2008, pp. 509-521
-
- Article
-
- You have access
- Export citation

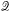 , μ*,
, μ*, 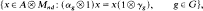
 to be the subspace
to be the subspace
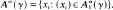 .
.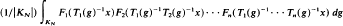
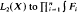 . The necessary and sufficient conditions are that each of the following representations are ergodic:
. The necessary and sufficient conditions are that each of the following representations are ergodic:  = (
= (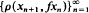 is small in some sense. For the case of round off errors in computer modelling, such a sequence is uniformly small, i.e. there exists some ε > 0, such that sup
is small in some sense. For the case of round off errors in computer modelling, such a sequence is uniformly small, i.e. there exists some ε > 0, such that sup −Γ is either empty or countably infinite, where
−Γ is either empty or countably infinite, where 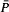 denotes the closure of the set
denotes the closure of the set 