Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Wu, Xinxing
Oprocha, Piotr
and
Chen, Guanrong
2016.
On various definitions of shadowing with average error in tracing.
Nonlinearity,
Vol. 29,
Issue. 7,
p.
1942.
Oprocha, Piotr
and
Wu, Xinxing
2017.
On averaged tracing of periodic average pseudo orbits.
Discrete & Continuous Dynamical Systems - A,
Vol. 37,
Issue. 9,
p.
4943.
Brian, Will
and
Oprocha, Piotr
2018.
Ultrafilters and Ramsey-type shadowing phenomena in topological dynamics.
Israel Journal of Mathematics,
Vol. 227,
Issue. 1,
p.
423.
Mitchell, Joel
2019.
Orbital shadowing, ω-limit sets and minimality.
Topology and its Applications,
Vol. 268,
Issue. ,
p.
106903.
Good, Chris
Mitchell, Joel
and
Thomas, Joe
2020.
Preservation of shadowing in discrete dynamical systems.
Journal of Mathematical Analysis and Applications,
Vol. 485,
Issue. 1,
p.
123767.
Good, Chris
Meddaugh, Jonathan
and
Mitchell, Joel
2020.
Shadowing, internal chain transitivity and α-limit sets.
Journal of Mathematical Analysis and Applications,
Vol. 491,
Issue. 1,
p.
124291.
Dong, Meihua
Jung, Woochul
and
Morales, Carlos
2020.
Eventually Shadowable Points.
Qualitative Theory of Dynamical Systems,
Vol. 19,
Issue. 1,
Darabi, Ali
2021.
A note on Fs-shadowing property.
Topology and its Applications,
Vol. 298,
Issue. ,
p.
107710.
Meddaugh, Jonathan
2022.
Shadowing as a structural property of the space of dynamical systems.
Discrete & Continuous Dynamical Systems,
Vol. 42,
Issue. 5,
p.
2439.
Mitchell, Joel
2022.
When is the beginning the end? On full trajectories, limit sets and internal chain transitivity.
Topology and its Applications,
Vol. 306,
Issue. ,
p.
107934.
Kamarudin, Nor Syahmina
and
Dzul-Kifli, Syahida Che
2024.
The implication of eventually shadowing property on a ℤd-action to the equivalence of chain recurrent set and omega limit set.
Vol. 3150,
Issue. ,
p.
020013.
Gonçalves, Daniel
and
Uggioni, Bruno B.
2024.
Shadowing for Local Homeomorphisms, with Applications to Edge Shift Spaces of Infinite Graphs.
Journal of Dynamics and Differential Equations,
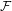 ${\mathcal{F}}$ with the Ramsey property an arbitrary sequence of points can be
${\mathcal{F}}$ with the Ramsey property an arbitrary sequence of points can be  ${\it\varepsilon}$-traced over a set from
${\it\varepsilon}$-traced over a set from  ${\mathcal{F}}$.
${\mathcal{F}}$.

