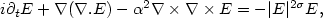Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Colin, M.
and
Colin, T.
2006.
A numerical model for the Raman amplification for laser-plasma interaction.
Journal of Computational and Applied Mathematics,
Vol. 193,
Issue. 2,
p.
535.
Masmoudi, Nader
and
Nakanishi, Kenji
2008.
Energy convergence for singular limits of Zakharov type systems.
Inventiones mathematicae,
Vol. 172,
Issue. 3,
p.
535.
Simpson, G.
Sulem, C.
and
Sulem, P. L.
2009.
Arrest of Langmuir wave collapse by quantum effects.
Physical Review E,
Vol. 80,
Issue. 5,
Han, Houde
Shih, Yintzer
and
Yin, Dongsheng
2017.
Tailored Finite Point Methods for Solving Singularly Perturbed Eigenvalue Problems with Higher Eigenvalues.
Journal of Scientific Computing,
Vol. 73,
Issue. 1,
p.
242.
Qu, Shiduo
Li, Wenlei
and
Shi, Shaoyun
2021.
Renormalization Group Approach to SDEs with Nonlinear Diffusion Terms.
Mediterranean Journal of Mathematics,
Vol. 18,
Issue. 5,