Hostname: page-component-78c5997874-fbnjt Total loading time: 0 Render date: 2024-11-15T12:15:11.216Z Has data issue: false hasContentIssue false
  • English
  • Français

A Fourier-Neumann series and its application to the reduction of triple cosine series

Published online by Cambridge University Press:  18 May 2009

C. J. Tranter  and
J. C. Cooke
C. J. Tranter
Affiliation:
Royal Military College of Science, Shrivenham
J. C. Cooke
Affiliation:
University of Bristol
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

The Jacobi expansion

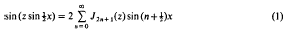
is well known and easily obtained from the generating function of the Besselcoefficients. The sum of the series on the right of equation (1) when sin (n+½)x is replaced by cos (n+½)x cannot be found in this way but it can be expressed in terms of a definite integral as shown below. The result so obtained is useful in reducing certain triple cosine series to dual series and so simplifying the solution given by one of us for such series in an earlier paper [1].

Type
Research Article
Information
Glasgow Mathematical Journal , Volume 14 , Issue 2 , September 1973 , pp. 198 - 201
Copyright
Copyright © Glasgow Mathematical Journal Trust 1973

References

REFERENCES

1.Tranter, C. J., Some triple trigonometrical series, Glasgow Math. J. 10 (1969), 121–125.CrossRefGoogle Scholar
2.Magnus, W. and Oberhettinger, F. (translated by Wermer, J.), Special functions of mathematical physics (New York, 1949).Google Scholar
3.Watson, G. N., Theory of Bessel functions (Cambridge, 1944).Google Scholar