Article contents
Limit theorems for the single server queue with traffic intensity one
Published online by Cambridge University Press: 14 July 2016
Extract
We consider a single server queueing system with inter-arrival times {un , n ≧ 1}, and service times {υn , n ≧ 1} and the queue-discipline, ‘first-come, first-served’. It is assumed that {un } and {υn } are two independent renewal processes, and 0 > E(un ) =a < ∞, 0 < E(υn ) = b < ∞. The traffic intensity is P ρ b/a(0 > ρ > ∞). This paper is concerned with the case ρ = 1, where it is known that the various queueing processes such as the queue-length Q(t) and waiting time W(t) diverge to + ∞ in distribution as t → ∞. Borovkov [1], [2] and Brody [3] have obtained limit distributions for Q(t) and W(t) with appropriate location and scale parameters in the cases P ≧ 1. Here we investigate random variables related to the busy and idle periods in the system. To explain our approach, we consider the random variables Xn = υn – un (n ≧ 1). Let S 0 ≡ 0, Sn = X 1 + X 2 + ··· + Xn (n ≧ 1), and define the sequence {Nk , k ≧ 0} as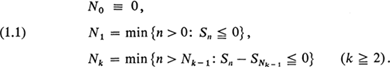
Information
- Type
- Research Papers
- Information
- Copyright
- Copyright © Applied Probability Trust
References
- 9
- Cited by

