Hostname: page-component-cd9895bd7-p9bg8 Total loading time: 0 Render date: 2024-12-22T11:15:50.046Z Has data issue: false hasContentIssue false
  • English
  • Français

Some results for repairable systems with general repair

Published online by Cambridge University Press:  14 July 2016

Masaaki Kijima
Masaaki Kijima*
Affiliation:
Tokyo Institute of Technology
*
∗Postal address: Department of Information Sciences, Tokyo Institute of Technology, Meguro-ku, Tokyo 152, Japan.
Get access Rights & Permissions [Opens in a new window]

Abstract

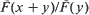In this paper, we develop general repair models for a repairable system by using the idea of the virtual age process of the system. If the system has the virtual age Vn –1 = y immediately after the (n – l)th repair, the nth failure-time Xn is assumed to have the survival function where 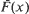is the survival function of the failure-time of a new system. A general repair is represented as a sequence of random variables An taking a value between 0 and 1, where An denotes the degree of the nth repair. For the extremal values 0 and 1, An = 1 means a minimal repair and An= 0 a perfect repair. Two models are constructed depending on how the repair affects the virtual age process: Vn = Vn– 1+ AnXn as Model 1 and Vn = An(Vn– 1 + Xn) as Model II. Various monotonicity properties of the process with respect to stochastic orderings of general repairs are obtained. Using a result, an upper bound for E[Sn] when a general repair is used is derived.

Keywords

MINIMAL REPAIRSTOCHASTIC ORDERING
Type
Research Papers
Information
Journal of Applied Probability , Volume 26 , Issue 1 , March 1989 , pp. 89 - 102
Copyright
Copyright © Applied Probability Trust 1989 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

[1] Abramowitz, M. and Stegun, I. A. (1965) Handbook of Mathematical Functions. Dover, New York.Google Scholar
[2] Barlow, R. E. and Proschan, F. (1965) Mathematical Theory of Reliability. Wiley, New York.Google Scholar
[3] Block, H. W., Borges, W. S. and Savits, T. H. (1985) Age-dependent minimal repair. J. Appl. Prob. 22, 370–385.CrossRefGoogle Scholar
[4] Brown, M. and Proschan, F. (1983) Imperfect repair. J. Appl. Prob. 20, 851–859.CrossRefGoogle Scholar
[5] Crow, E. L. (1974), 379–410 in Reliability and Biometry , ed. Proschan, F. and Serfling, R. SIAM, Philadelphia.Google Scholar
[6] Kijima, M. and Sumita, U. (1986) A useful generalization of renewal theory: counting process governed by nonnegative Markovian increments. J. Appl. Prob. 23, 71–88.Google Scholar
[7] Kijima, M., Morimura, H. and Suzuki, Y. (1988) Periodical replacement problem without assuming minimal repair. Europ. J. Operat. Res. 37, 194–203.Google Scholar
[8] Shaked, M. and Shanthikumar, J. G. (1986) Multivariate imperfect repair. Operat. Res. 34, 437–448.CrossRefGoogle Scholar
[9] Stoyan, D. (1983) Comparison Methods for Queues and Other Stochastic Models. Wiley, New York.Google Scholar
[10] Uematsu, K. and Nishida, T. (1987) One unit system with a failure rate depending upon the degree of repair. Math. Japonica , 32, 139–147.Google Scholar