Hostname: page-component-586b7cd67f-t7fkt Total loading time: 0 Render date: 2024-11-24T22:35:43.485Z Has data issue: false hasContentIssue false
  • English
  • Français

Comparative study on single-incident and dual-incident shock wave/turbulent boundary layer interactions with identical total deflection angle

Published online by Cambridge University Press:  05 April 2022

Xin Li ,
Yue Zhang ,
Huijun Tan Open the ORCID record for Huijun Tan [Opens in a new window] ,
Yi Jin  and
Chao Li
Xin Li
Affiliation:
College of Energy and Power Engineering, Nanjing University of Aeronautics and Astronautics, Nanjing 210016, PR China
Yue Zhang*
Affiliation:
College of Energy and Power Engineering, Nanjing University of Aeronautics and Astronautics, Nanjing 210016, PR China
Huijun Tan*
Affiliation:
College of Energy and Power Engineering, Nanjing University of Aeronautics and Astronautics, Nanjing 210016, PR China
Yi Jin
Affiliation:
College of Energy and Power Engineering, Nanjing University of Aeronautics and Astronautics, Nanjing 210016, PR China
Chao Li
Affiliation:
College of Energy and Power Engineering, Nanjing University of Aeronautics and Astronautics, Nanjing 210016, PR China
*
†Email addresses for correspondence: y.zhang@nuaa.edu.cn, thj@263.net
†Email addresses for correspondence: y.zhang@nuaa.edu.cn, thj@263.net
Get access Rights & Permissions [Opens in a new window]

Abstract

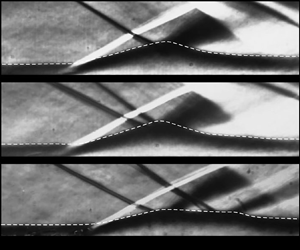
Interactions between the boundary layer and two successive incident shock waves (ISWs) often occur in the supersonic mixed-compression inlets. However, the flow mechanism involved in such interactions has been studied rarely. In this study, we investigate experimentally and analytically the turbulent boundary layer separation flow induced by the single ISW and dual ISWs at the identical total deflection angles in a Mach 2.73 flow. Schlieren photography, wall pressure measurement and surface oil-flow visualisation are employed to diagnose the flow field. Experiments with the impingement points of the two ISWs intersecting on the bottom wall exhibit a separated flow with a triangle-like separation bubble, namely the first kind of dual-ISW/turbulent boundary layer interaction (ISWTBLI). Comparative studies show that various flow features of this kind of dual-ISWTBLI, including the extent of the separation region, pressure distribution and surface-flow topological structures, are nearly identical to those of the single-ISWTBLI with an identical total deflection angle. As the distance between the two ISWs increases, the shape of the separation region in the dual-ISWTBLI changes from triangle-like to quadrilateral-like, and the height of the separation region decreases accordingly, forming the second kind of dual-ISWTBLI. Furthermore, an inviscid model is developed for the dual-ISWTBLI to describe the complex shock wave system and elucidate the cause of a quadrilateral-like separation bubble in the second kind of dual-ISWTBLI. Moreover, based on a previous work by Souverein et al. (J. Fluid Mech., vol. 714, 2013, pp. 505–535) on the single-ISWTBLI, a modified scaling method is established for the first kind of dual-ISWTBLI.

JFM classification

Compressible Flows: Supersonic flow Compressible Flows: Shock waves Boundary Layers: Boundary layer separation
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 940 , 10 June 2022 , A7
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Adler, M.C. & Gaitonde, D.V. 2018 Dynamic linear response of a shock/turbulent-boundary-layer interaction using constrained perturbations. J. Fluid Mech. 840, 291–341.CrossRefGoogle Scholar
Anderson, J.D. 2010 Fundamentals of Aerodynamics. McGraw-Hill.Google Scholar
Babinsky, H. & Harvey, J.K. 2011 Shock Wave–Boundary-Layer Interactions. Cambridge University Press.CrossRefGoogle Scholar
Babinsky, H. & Ogawa, H. 2008 SBLI control for wings and inlets. Shock Waves 18 (2), 89–96.CrossRefGoogle Scholar
Babinsky, H., Oorebeek, J. & Cottingham, T. 2013 Corner effects in reflecting oblique shock-wave/boundary-layer interactions. AIAA Paper 2013-859.CrossRefGoogle Scholar
Benek, J., Suchyta, C. & Babinsky, H. 2013 The effect of tunnel size on incident shock boundary layer interaction experiments. AIAA Paper 2013-862.CrossRefGoogle Scholar
Bermejo-Moreno, I., Campo, L., Larsson, J., Bodart, J., Helmer, D. & Eaton, J.K. 2014 Confinement effects in shock wave/turbulent boundary layer interactions through wall-modelled large-eddy simulations. J. Fluid Mech. 758, 5–62.CrossRefGoogle Scholar
Bookey, P., Wyckham, C. & Smits, A. 2005 Experimental investigations of Mach 3 shock-wave turbulent boundary layer interactions. AIAA Paper 2005-4899.CrossRefGoogle Scholar
Brooks, J., Gupta, A., Marineau, E.C., Martín, M.P., Smith, M. & Marineau, E. 2017 Mach 10 PIV flow field measurements of a turbulent boundary layer and shock turbulent boundary layer interaction. AIAA Paper 2017-3325.CrossRefGoogle Scholar
Carrière, P., Sirieix, M. & Solignac, J.L. 1969 Similarity properties of the laminar or turbulent separation phenomena in a non-uniform supersonic flow. In Applied Mechanics, pp. 145–157. Springer.CrossRefGoogle Scholar
Chapman, D.R., Kuehn, D.M. & Larson, H.K. 1957 Investigation of separated flows in supersonic and subsonic streams with emphasis on the effect of transition. NACA Tech. Rep. 1356.Google Scholar
Charwat, A.F. 1970 Supersonic flows with imbedded separated regions. Adv. Heat Transfer 6, 1–132.CrossRefGoogle Scholar
Clemens, N.T. & Narayanaswamy, V. 2014 Low-frequency unsteadiness of shock wave/turbulent boundary layer interactions. Annu. Rev. Fluid Mech. 46, 469–492.CrossRefGoogle Scholar
Daub, D., Willems, S. & Gülhan, A. 2016 Experimental results on unsteady shock-wave/boundary-layer interaction induced by an impinging shock. CEAS Space J. 8 (1), 3–12.CrossRefGoogle Scholar
Dolling, D.S. 2001 Fifty years of shock-wave/boundary-layer interaction research: what next? AIAA J. 39 (8), 1517–1531.CrossRefGoogle Scholar
van Driest, E.R. 1951 Turbulent boundary layer in compressible fluids. J. Aeronaut. Sci. 18 (3), 145–160.CrossRefGoogle Scholar
Dupont, P., Haddad, C. & Debiève, J.F. 2006 Space and time organization in a shock-induced separated boundary layer. J. Fluid Mech. 559, 255–277.CrossRefGoogle Scholar
Dussauge, J.P. & Piponniau, S. 2008 Shock/boundary-layer interactions: possible sources of unsteadiness. J. Fluids Struct. 24 (8), 1166–1175.CrossRefGoogle Scholar
Délery, J. & Dussauge, J.P. 2009 Some physical aspects of shock wave/boundary layer interactions. Shock Waves 19 (6), 453–468.CrossRefGoogle Scholar
Délery, J., Marvin, J.G. & Reshotko, E. 1986 Shock-wave boundary layer interactions. Tech. Rep. AGARD-AG-280. North Atlantic Treaty Organization Advisory Group for Aerospace Research and Development.Google Scholar
Edney, B. 1968 Anomalous heat transfer and pressure distributions on blunt bodies at hypersonic speeds in the presence of an impinging shock. Tech. Rep. FFA Report 115. Aeronautical Research Institute of Sweden, Stockholm.CrossRefGoogle Scholar
Erdos, J. & Pallone, A. 1962 Shock-boundary layer interaction and flow separation. In Proceedings of the 1962 Heat Transfer and Fluid Mechanics Institute, vol. 15, pp. 239–254. Stanford University Press.Google Scholar
Ferri, A. 1940 Experimental results with airfoils tested in the high-speed tunnel at Guidonia. NACA Tech. Rep. 946.Google Scholar
Gaitonde, D.V. 2015 Progress in shock wave/boundary layer interactions. Prog. Aerosp. Sci. 72, 80–99.CrossRefGoogle Scholar
Ganapathisubramani, B., Clemens, N.T. & Dolling, D.S. 2007 Effects of upstream boundary layer on the unsteadiness of shock-induced separation. J. Fluid Mech. 585, 369–394.CrossRefGoogle Scholar
Green, J.E. 1970 Interactions between shock waves and turbulent boundary layers. Prog. Aerosp. Sci. 11, 235–340.CrossRefGoogle Scholar
Grossman, I.J. & Bruce, P.J.K. 2018 Confinement effects on regular–irregular transition in shock-wave–boundary-layer interactions. J. Fluid Mech. 853, 171–204.CrossRefGoogle Scholar
Helm, C.M. 2021 Direct and large-eddy simulation data and analysis of shock-separated flows. PhD thesis, University of Maryland.Google Scholar
Helm, C.M. & Martín, M.P. 2021 Scaling of hypersonic shock/turbulent boundary layer interactions. Phys. Rev. Fluids 6 (7), 074607.CrossRefGoogle Scholar
Henderson, L.F. 1967 The reflexion of a shock wave at a rigid wall in the presence of a boundary layer. J. Fluid Mech. 30 (4), 699–722.CrossRefGoogle Scholar
Hong, Y., Li, Z. & Yang, J. 2021 Scaling of interaction lengths for hypersonic shock wave/turbulent boundary layer interactions. Chin. J. Aeronaut. 34 (5), 504–509.CrossRefGoogle Scholar
Huang, H.X., Tan, H.J., Sun, S. & Sheng, F.J. 2017 Unthrottled flows with complex background waves in curved isolators. AIAA J. 55 (9), 2942–2955.CrossRefGoogle Scholar
Kendall, A. & Koochesfahani, M. 2008 A method for estimating wall friction in turbulent wall-bounded flows. Exp. Fluids 44 (5), 773–780.CrossRefGoogle Scholar
Krishnan, L., Sandham, N.D. & Steelant, J. 2009 Shock-wave/boundary-layer interactions in a model scramjet intake. AIAA J. 47 (7), 1680–1691.CrossRefGoogle Scholar
Law, C.H. 1976 Supersonic shock wave turbulent boundary-layer interactions. AIAA J. 14 (6), 730–734.CrossRefGoogle Scholar
Li, H., Chpoun, A. & Ben-Dor, G. 1999 Analytical and experimental investigations of the reflection of asymmetric shock waves in steady flows. J. Fluid Mech. 390, 25–43.CrossRefGoogle Scholar
Li, X., Tan, H.J., Zhang, Y., Huang, H.X., Guo, Y.J. & Lin, Z.K. 2020 Flow patterns of dual-incident shock waves/turbulent boundary layer interaction. J. Vis. 23 (6), 931–935.CrossRefGoogle Scholar
Matheis, J. & Hickel, S. 2015 On the transition between regular and irregular shock patterns of shock-wave/boundary-layer interactions. J. Fluid Mech. 776, 200–234.CrossRefGoogle Scholar
Perry, A.E. & Hornung, H. 1984 Some aspects of three-dimensional separation. II. Vortex skeletons. Z. Flugwiss. Weltraumforsch. 8, 155–160.Google Scholar
Piponniau, S., Dussauge, J.P., Debiève, J.F. & Dupont, P. 2009 A simple model for low-frequency unsteadiness in shock-induced separation. J. Fluid Mech. 629, 87–108.CrossRefGoogle Scholar
Pirozzoli, S. & Grasso, F. 2006 Direct numerical simulation of impinging shock wave/turbulent boundary layer interaction at ${M}=2.25$. Phys. Fluids 18 (6), 065113.CrossRefGoogle Scholar
Priebe, S. & Martín, M.P. 2012 Low-frequency unsteadiness in shock wave-turbulent boundary layer interaction. J. Fluid Mech. 699, 1–49.CrossRefGoogle Scholar
Priebe, S., Tu, J.H., Rowley, C.W. & Martín, M.P. 2016 Low-frequency dynamics in a shock-induced separated flow. J. Fluid Mech. 807, 441–477.CrossRefGoogle Scholar
Ramesh, M.D. & Tannehill, J.C. 2004 Correlations to predict the streamwise influence regions in supersonic turbulent flows. J. Aircraft 41 (2), 274–283.CrossRefGoogle Scholar
Reda, D.C. & Murphy, J.D. 1973 Shock wave/turbulent boundary-layer interactions in rectangular channels. AIAA J. 11 (2), 139–140.CrossRefGoogle Scholar
Sanders, B.W. & Weir, L.J. 2008 Aerodynamic design of a dual-flow Mach 7 hypersonic inlet system for a turbine-based combined-cycle hypersonic propulsion system. NASA/CR Tech. Rep. 215214.Google Scholar
Settles, G.S. 1976 An experimental study of compressible turbulent boundary layer separation at high Reynolds numbers. PhD thesis, Princeton University.CrossRefGoogle Scholar
Settles, G.S., Bogdonoff, S.M. & Vas, I.E. 1976 Incipient separation of a supersonic turbulent boundary layer at high Reynolds numbers. AIAA J. 14 (1), 50–56.CrossRefGoogle Scholar
Souverein, L.J., Bakker, P.G. & Dupont, P. 2013 A scaling analysis for turbulent shock-wave/boundary-layer interactions. J. Fluid Mech. 714, 505–535.CrossRefGoogle Scholar
Souverein, L.J., Dupont, P., Debieve, J.F., Dussauge, J.P., van Oudheusden, B.W. & Scarano, F. 2010 Effect of interaction strength on unsteadiness in shock-wave-induced separations. AIAA J. 48 (7), 1480–1493.CrossRefGoogle Scholar
Souverein, L.J., van Oudheusden, B.W., Scarano, F. & Dupont, P. 2009 Application of a dual-plane particle image velocimetry (dual-PIV) technique for the unsteadiness characterization of a shock wave turbulent boundary layer interaction. Meas. Sci. Technol. 20 (7), 074003.CrossRefGoogle Scholar
Spaid, F.W. & Frishett, J.C. 1972 Incipient separation of a supersonic, turbulent boundary layer, including effects of heat transfer. AIAA J. 10 (7), 915–922.CrossRefGoogle Scholar
Tan, H.J., Sun, S. & Huang, H.X. 2012 Behavior of shock trains in a hypersonic inlet/isolator model with complex background waves. Exp. Fluids 53 (6), 1647–1661.CrossRefGoogle Scholar
Thomke, G.J. & Roshko, A. 1969 Incipient separation of a turbulent boundary layer at high Reynolds number in two-dimensional supersonic flow over a compression corner. Tech. Rep. DAC-59819. NASA Ames Research Center.Google Scholar
Touber, E. & Sandham, N.D. 2009 Large-eddy simulation of low-frequency unsteadiness in a turbulent shock-induced separation bubble. Theor. Comput. Fluid Dyn. 23 (2), 79–107.CrossRefGoogle Scholar
Touber, E. & Sandham, N.D. 2011 Low-order stochastic modelling of low-frequency motions in reflected shock-wave/boundary-layer interactions. J. Fluid Mech. 671 (3), 417–465.CrossRefGoogle Scholar
Viswanath, P.R. 1988 Shock-wave–turbulent-boundary-layer interaction and its control: a survey of recent developments. Sadhana 12 (1), 45–104.CrossRefGoogle Scholar
Wang, B., Sandham, N.D., Hu, Z. & Liu, W. 2015 Numerical study of oblique shock-wave/boundary-layer interaction considering sidewall effects. J. Fluid Mech. 767, 526–561.CrossRefGoogle Scholar
White, F.M. 2006 Viscous Fluid Flow. McGraw-Hill.Google Scholar
Wu, M. & Martín, M.P. 2008 Analysis of shock motion in shockwave and turbulent boundary layer interaction using direct numerical simulation data. J. Fluid Mech. 594, 71–83.CrossRefGoogle Scholar
Xiang, X. & Babinsky, H. 2019 Corner effects for oblique shock wave/turbulent boundary layer interactions in rectangular channels. J. Fluid Mech. 862, 1060–1083.CrossRefGoogle Scholar
Zhang, H., Li, X., Tan, H., Sun, S., Wu, J. & Zhang, Y. 2021 Experimental investigation of dual swept shock wave/boundary layer interactions. J. Vis. 24 (6), 1115–1122.CrossRefGoogle Scholar
Zheltovodov, A. 2006 Some advances in research of shock wave turbulent boundary layer interactions. AIAA Paper 2006-496.CrossRefGoogle Scholar