On the basis of a two-point similarity analysis, the well-known power-law variations for the mean kinetic energy dissipation rate $\overline{\epsilon }$ and the longitudinal velocity variance 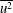$\overline{u^2}$ on the axis of a round jet are derived. In particular, the prefactor for $\overline{\epsilon } \propto (x-x_0)^{-4}$, where 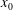$x_0$ is a virtual origin, follows immediately from the variation of the mean velocity, the constancy of the local turbulent intensity and the ratio between the axial and transverse velocity variance. Second, the limit at small separations of the two-point budget equation yields an exact relation illustrating the equilibrium between the skewness of the longitudinal velocity derivative 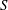$S$ and the destruction coefficient $G$ of enstrophy. By comparing the latter relation with that for homogeneous isotropic decaying turbulence, it is shown that the approach towards the asymptotic state at infinite Reynolds number of 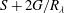$S+2G/R_{\lambda }$ in the jet differs from that in purely decaying turbulence, although $S+2G/R_{\lambda } \propto R_{\lambda }^{-1}$ in each case. This suggests that, at finite Reynolds numbers, the transport equation for $\overline{\epsilon }$ imposes a fundamental constraint on the balance between 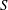$S$ and $G$ that depends on the type of large-scale forcing and may thus differ from flow to flow. This questions the conjecture that $S$ and 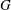$G$ follow a universal evolution with 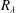$R_{\lambda }$; instead, 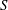$S$ and $G$ must be tested separately in each flow. The implication for the constant 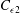$C_{\epsilon 2}$ in the $k-\overline{\epsilon }$ model is also discussed.