Article contents
Erosion of a sharp density interface by a turbulent jet at moderate Froude and Reynolds numbers
Published online by Cambridge University Press: 25 January 2018
Abstract
Using water–salt water laboratory experiments, we investigate the mechanism of erosion by a turbulent jet impinging onto a density interface, for moderate Reynolds and Froude numbers. The Froude number is defined by 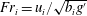 $Fr_{i}=u_{i}/\sqrt{b_{i}g^{\prime }}$, with
$Fr_{i}=u_{i}/\sqrt{b_{i}g^{\prime }}$, with 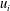 $u_{i}$ and
$u_{i}$ and 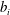 $b_{i}$, the typical velocity and width of the jet at the interface, and
$b_{i}$, the typical velocity and width of the jet at the interface, and 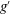 $g^{\prime }$ the reduced gravitational acceleration. The Froude number
$g^{\prime }$ the reduced gravitational acceleration. The Froude number 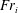 $Fr_{i}$ characterizes the competition between inertial forces against the restoring buoyancy force. Contrary to previous observations reporting baroclinic destabilization of the interface, we show that the entrainment, in the range of parameters explored here, is driven by interfacial gravity waves. The waves are generated by the barotropic excitation coming from the turbulent fluctuations of the jet; they are then amplified by a mechanism of wave-induced stress; and they finally break and induce entrainment and mixing. Based on those physical observations, we introduce a scaling model for the entrainment rate, which varies continuously from
$Fr_{i}$ characterizes the competition between inertial forces against the restoring buoyancy force. Contrary to previous observations reporting baroclinic destabilization of the interface, we show that the entrainment, in the range of parameters explored here, is driven by interfacial gravity waves. The waves are generated by the barotropic excitation coming from the turbulent fluctuations of the jet; they are then amplified by a mechanism of wave-induced stress; and they finally break and induce entrainment and mixing. Based on those physical observations, we introduce a scaling model for the entrainment rate, which varies continuously from 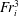 $Fr_{i}^{3}$ to an
$Fr_{i}^{3}$ to an  $Fr_{i}$ power law from small to large Froude numbers, in agreement with the present and some of the previous laboratory data.
$Fr_{i}$ power law from small to large Froude numbers, in agreement with the present and some of the previous laboratory data.
JFM classification
- Type
- JFM Papers
- Information
- Copyright
- © 2018 Cambridge University Press
References
- 8
- Cited by



