Hostname: page-component-586b7cd67f-vdxz6 Total loading time: 0 Render date: 2024-12-01T04:31:32.409Z Has data issue: false hasContentIssue false
  • English
  • Français

Experimental study on radial gravity currents flowing in a vegetated channel

Published online by Cambridge University Press:  06 January 2022

D. Petrolo Open the ORCID record for D. Petrolo [Opens in a new window] ,
M. Ungarish Open the ORCID record for M. Ungarish [Opens in a new window] ,
L. Chiapponi Open the ORCID record for L. Chiapponi [Opens in a new window]  and
S. Longo Open the ORCID record for S. Longo [Opens in a new window]
D. Petrolo*
Affiliation:
Department of Engineering and Architecture, Università degli Studi di Parma, Parco Area delle Scienze 181/A, 43124Parma, Italy
M. Ungarish
Affiliation:
Department of Computer Science, Technion, Israel Institute of Technology, Haifa32000, Israel
L. Chiapponi
Affiliation:
Department of Engineering and Architecture, Università degli Studi di Parma, Parco Area delle Scienze 181/A, 43124Parma, Italy
S. Longo
Affiliation:
Department of Engineering and Architecture, Università degli Studi di Parma, Parco Area delle Scienze 181/A, 43124Parma, Italy
*
†Email address for correspondence: diana.petrolo@unipr.it
Get access Rights & Permissions [Opens in a new window]

Abstract

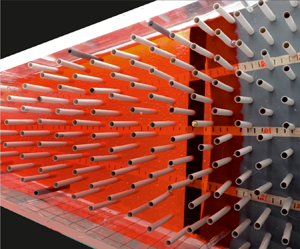
We present an experimental study of gravity currents in a cylindrical geometry, in the presence of vegetation. Forty tests were performed with a brine advancing in a fresh water ambient fluid, in lock release, and with a constant and time-varying flow rate. The tank is a circular sector of angle $30^\circ$ with radius equal to 180 cm. Two different densities of the vegetation were simulated by vertical plastic rods with diameter $D=1.6\ \textrm{cm}$. We marked the height of the current as a function of radius and time and the position of the front as a function of time. The results indicate a self-similar structure, with lateral profiles that after an initial adjustment collapse to a single curve in scaled variables. The propagation of the front is well described by a power law function of time. The existence of self-similarity on an experimental basis corroborates a simple theoretical model with the following assumptions: (i) the dominant balance is between buoyancy and drag, parameterized by a power law of the current velocity $\sim |u|^{\lambda-1}u$; (ii) the current advances in shallow-water conditions; and (iii) ambient-fluid dynamics is negligible. In order to evaluate the value of ${\lambda}$ (the only tuning parameter of the theoretical model), we performed two additional series of measurements. We found that $\lambda$ increased from 1 to 2 while the Reynolds number increased from 100 to approximately $6\times10^3$, and the drag coefficient and the transition from $\lambda=1$ to $\lambda=2$ are quantitatively affected by D, but the structure of the model is not.

JFM classification

Geophysical and Geological Flows: Gravity currents Mathematical Foundations: Topological fluid dynamics Flow Control: Drag reduction
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 933 , 25 February 2022 , A46
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Belcher, S.E., Harman, I.N. & Finnigan, J.J. 2012 The wind in the willows: flows in forest canopies in complex terrain. Annu. Rev. Fluid Mech. 44, 479–504.CrossRefGoogle Scholar
Benjamin, T.B. 1968 Gravity currents and related phenomena. J. Fluid Mech. 31 (2), 209–248.CrossRefGoogle Scholar
Cenedese, C., Nokes, R. & Hyatt, J. 2018 Lock-exchange gravity currents over rough bottoms. Environ. Fluid Mech. 18 (1), 59–73.CrossRefGoogle Scholar
Chiapponi, L., Ungarish, M., Longo, S., Di Federico, V. & Addona, F. 2018 Critical regime of gravity currents flowing in non-rectangular channels with density stratification. J. Fluid Mech. 840, 579–612.CrossRefGoogle Scholar
Chiapponi, L., Ungarish, M., Petrolo, D., Di Federico, V. & Longo, S. 2019 Non-Boussinesq gravity currents and surface waves generated by lock release in a circular-section channel: theoretical and experimental investigation. J. Fluid Mech. 869, 610–633.CrossRefGoogle Scholar
Chowdhury, M.R. & Testik, F.Y. 2014 A review of gravity currents formed by submerged single-port discharges in inland and coastal waters. Environ. Fluid Mech. 14 (2), 265–293.CrossRefGoogle Scholar
De Falco, M.C., Adduce, C., Negretti, M.E. & Hopfinger, E.J. 2021 On the dynamics of quasi-steady gravity currents flowing up a slope. Adv. Water Resour. 147, 103791.CrossRefGoogle Scholar
Di Federico, V., Archetti, R. & Longo, S. 2012 Spreading of axisymmetric non-Newtonian power-law gravity currents in porous media. J. Non-Newtonian Fluid Mech. 189, 31–39.CrossRefGoogle Scholar
Di Federico, V., Longo, S., King, S.E., Chiapponi, L., Petrolo, D. & Ciriello, V. 2017 Gravity-driven flow of Herschel–Bulkley fluid in a fracture and in a 2D porous medium. J. Fluid Mech. 821, 59–84.CrossRefGoogle Scholar
Ergun, S. 1952 Fluid flow through packed columns. Chem. Engng Prog. 48, 89–94.Google Scholar
Finnigan, J. 2000 Turbulence in plant canopies. Annu. Rev. Fluid Mech. 32 (1), 519–571.CrossRefGoogle Scholar
Hatcher, L., Hogg, A.J. & Woods, A.W. 2000 The effects of drag on turbulent gravity currents. J. Fluid Mech. 416, 297–314.CrossRefGoogle Scholar
Hogg, A.J., Ungarish, M. & Huppert, H.E. 2000 Particle-driven gravity currents: asymptotic and box model solutions. Eur. J. Mech. (B/Fluids) 19 (1), 139–165.CrossRefGoogle Scholar
Huppert, H.E. 2006 Gravity currents: a personal perspective. J. Fluid Mech. 554, 299.CrossRefGoogle Scholar
Lane-Serff, G.F. 1993 On drag-limited gravity currents. Deep-Sea Res. (I) 40 (8), 1699–1702.CrossRefGoogle Scholar
Lauber, G. & Hager, W.H. 1998 Experiments to dambreak wave: horizontal channel. J. Hydraul Res. 36 (3), 291–307.CrossRefGoogle Scholar
Lippert, M.C. & Woods, A.W. 2020 Experiments on the sedimentation front in steady particle-driven gravity currents. J. Fluid Mech. 889, A20.CrossRefGoogle Scholar
Longo, S. 2022 Principles and Applications of Dimensional Analysis and Similarity. Springer.Google Scholar
Longo, S., Ungarish, M., Di Federico, V., Chiapponi, L. & Addona, F. 2016 Gravity currents in a linearly stratified ambient fluid created by lock release and influx in semi-circular and rectangular channels. Phys. Fluids 28 (9), 096602.CrossRefGoogle Scholar
Longo, S., Ungarish, M., Di Federico, V., Chiapponi, L. & Maranzoni, A. 2015 The propagation of gravity currents in a circular cross-section channel: experiments and theory. J. Fluid Mech. 764, 513.CrossRefGoogle Scholar
Longo, S., Ungarish, M., Di Federico, V., Chiapponi, L. & Petrolo, D. 2018 Gravity currents produced by lock-release: theory and experiments concerning the effect of a free top in non-Boussinesq systems. Adv. Water Resour. 121, 456–471.CrossRefGoogle Scholar
Macdonald, I.F., El-Sayed, M.S., Mow, K. & Dullien, F.A.L. 1979 Flow through porous media-the Ergun equation revisited. Ind. Engng Chem. Fundam. 18 (3), 199–208.CrossRefGoogle Scholar
Martin, A., Negretti, M.E., Ungarish, M. & Zemach, T. 2020 Propagation of a continuously supplied gravity current head down bottom slopes. Phys. Rev. Fluids 5 (5), 054801.CrossRefGoogle Scholar
Maxworthy, T. 1983 Gravity currents with variable inflow. J. Fluid. Mech. 128, 247–257.CrossRefGoogle Scholar
Naftchali, A.K., Khozeymehnezhad, H., Akbarpour, A. & Varjavand, P. 2016 Experimental study on the effects of artificial vegetation density on forehead of saline current flow. Ain Shams Engng J. 7 (2), 799–809.CrossRefGoogle Scholar
Nepf, H.M. 2012 Flow and transport in regions with aquatic vegetation. Annu. Rev. Fluid Mech. 44, 123–142.CrossRefGoogle Scholar
Ottolenghi, L., Adduce, C., Inghilesi, R., Roman, F. & Armenio, V. 2016 Mixing in lock-release gravity currents propagating up a slope. Phys. Fluids 28 (5), 056604.CrossRefGoogle Scholar
Ozan, A.Y., Constantinescu, G. & Hogg, A.J. 2015 Lock-exchange gravity currents propagating in a channel containing an array of obstacles. J. Fluid Mech. 765, 544–575.CrossRefGoogle Scholar
Sayag, R. & Worster, G.M. 2013 Axisymmetric gravity currents of power-law fluids over a rigid horizontal surface. J. Fluid Mech. 716, R5.CrossRefGoogle Scholar
Sciortino, G., Adduce, C. & Lombardi, V. 2018 A new front condition for non-Boussinesq gravity currents. J. Hydraul Res. 56 (4), 517–525.CrossRefGoogle Scholar
Sher, D. & Woods, A.W. 2017 Mixing in continuous gravity currents. J. Fluid Mech. 818, R4.CrossRefGoogle Scholar
Simpson, J.E. 1999 Gravity Currents: In the Environment and the Laboratory. Cambridge University Press.Google Scholar
Sparks, R.S.J., Bonnecaze, R.T., Huppert, H.E., Lister, J.R., Hallworth, M.A., Mader, H. & Phillips, J. 1993 Sediment-laden gravity currents with reversing buoyancy. Earth Planet. Sci. Lett. 114 (2–3), 243–257.CrossRefGoogle Scholar
Stone, B.M. & Shen, H.T. 2002 Hydraulic resistance of flow in channels with cylindrical roughness. J. Hydraul. Engng ASCE 128 (5), 500–506.CrossRefGoogle Scholar
Tanino, Y., Nepf, H.M. & Kulis, P.S. 2005 Gravity currents in aquatic canopies. Water Resour. Res. 41, W12402.CrossRefGoogle Scholar
Testik, F.Y. & Ungarish, M. 2016 On the self-similar propagation of gravity currents through an array of emergent vegetation-like obstacles. Phys. Fluids 28 (5), 056605.CrossRefGoogle Scholar
Testik, F.Y. & Yilmaz, N.A. 2015 Anatomy and propagation dynamics of continuous-flux release bottom gravity currents through emergent aquatic vegetation. Phys. Fluids 27 (5), 056603.CrossRefGoogle Scholar
Tsakiri, M., Prinos, P. & Koftis, T. 2016 Numerical simulation of turbulent exchange flow in aquatic canopies. J. Hydraul Res. 54 (2), 131–144.CrossRefGoogle Scholar
Ungarish, M. 2009 An Introduction to Gravity Currents and Intrusions. CRC Press.CrossRefGoogle Scholar
Ungarish, M. 2018 Thin-layer models for gravity currents in channels of general cross-section area, a review. Environ. Fluid Mech. 18 (1), 283–333.CrossRefGoogle Scholar
Ungarish, M. 2020 Gravity Currents and Intrusions. World Scientific.CrossRefGoogle Scholar
Vargas-Luna, A., Crosato, A., Calvani, G. & Uijttewaal, W.S.J. 2016 Representing plants as rigid cylinders in experiments and models. Adv. Water Resour. 93, 205–222.CrossRefGoogle Scholar
Whitaker, S. 1996 The Forchheimer equation: a theoretical development. Transp. Porous Med. 25 (1), 27–61.CrossRefGoogle Scholar
Zemach, T., Chiapponi, L., Petrolo, D., Ungarish, M., Longo, S. & Di Federico, V. 2017 On the propagation of particulate gravity currents in circular and semi-circular channels partially filled with homogeneous or stratified ambient fluid. Phys. Fluids 29 (10), 106605.CrossRefGoogle Scholar
Zgheib, N., Bonometti, T. & Balachandar, S. 2015 Dynamics of non-circular finite release gravity currents. J. Fluid Mech. 783, 344.CrossRefGoogle Scholar
Zhang, X. & Nepf, H.M. 2008 Density-driven exchange flow between open water and an aquatic canopy. Water Resour. Res. 44, W08417.CrossRefGoogle Scholar