Hostname: page-component-cd9895bd7-hc48f Total loading time: 0 Render date: 2024-12-29T11:10:26.240Z Has data issue: false hasContentIssue false
  • English
  • Français

On the contact region of a diffusion-limited evaporating drop: a local analysis

Published online by Cambridge University Press:  18 December 2013

S. J. S. Morris
S. J. S. Morris*
Affiliation:
Department of Mechanical Engineering, University of California, Berkeley, CA 94720, USA
*
†Email address for correspondence: morris@berkeley.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

Motivated by experiments showing that a sessile drop of volatile perfectly wetting liquid initially advances over the substrate, but then reverses, we formulate the problem describing the contact region at reversal. Assuming a separation of scales, so that the radial extent of this region is small compared with the instantaneous radius $a$ of the apparent contact line, we show that the time scale characterizing the contact region is small compared with that on which the bulk drop is evolving. As a result, the contact region is governed by a boundary-value problem, rather than an initial-value problem: the contact region has no memory, and all its properties are determined by conditions at the instant of reversal. We conclude that the apparent contact angle 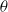$\theta $ is a function of the instantaneous drop radius $a$, as found in the experiments. We then non-dimensionalize the boundary-value problem, and find that its solution depends on one parameter 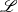$\mathscr{L}$, a dimensionless surface tension. According to this formulation, the apparent contact angle is well-defined: at the outer edge of the contact region, the film slope approaches a limit that is independent of the curvature of bulk drop. In this, it differs from the dynamic contact angle observed during spreading of non-volatile drops. Next, we analyse the boundary-value problem assuming 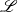$\mathscr{L}$ to be small. Though, for arbitrary 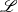$\mathscr{L}$, determining $\theta $ requires solving the steady diffusion equation for the vapour, there is, for small 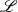$\mathscr{L}$, a further separation of scales within the contact region. As a result, 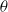$\theta $ is now determined by solving an ordinary differential equation. We predict that 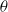$\theta $ varies as 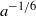${a}^{- 1/ 6} $, as found experimentally for small drops ($a\lt 1~\mathrm{mm} $). For these drops, predicted and measured angles agree to within 10–30 %. Because the discrepancy increases with $a$, but 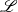$\mathscr{L}$ is a decreasing function of $a$, we infer that some process occurring outside the contact region is required to explain the observed behaviour of larger drops having 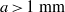$a\gt 1~\mathrm{mm} $.

JFM classification

Interfacial Flows (free surface): Capillary flows Interfacial Flows (free surface): Contact lines Interfacial Flows (free surface): Thin films
Type
Papers
Information
Journal of Fluid Mechanics , Volume 739 , 25 January 2014 , pp. 308 - 337
Copyright
©2013 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Berezhnoi, A. N. & Semenov, A. V. 1997 Binary Diffusion Coefficients of Liquid Vapours in Gases. Begell House.Google Scholar
Beverley, K. J., Clint, J. H. & Fletcher, P. D. I. 1999 Phys. Chem. Chem. Phys. 1, 149–153.CrossRefGoogle Scholar
Bonn, D., Eggers, J., Indekeu, J., Meunier, J. & Rolley, E. 2009 Rev. Mod. Phys. 81, 739–806.CrossRefGoogle Scholar
Carruth, G. F. & Kobayashi, R. 1973 J. Chem. Engng Data 18, 115–126.CrossRefGoogle Scholar
Cazabat, A. M. & Guéna, G. 2010 Soft Matt. 6, 2591–2612.CrossRefGoogle Scholar
Chae, K., Elvati, P. & Violi, A. 2011 J. Phys. Chem. B 115, 500–506.Google Scholar
Chapman, S. & Cowling, T. G. 1970 The Mathemtical Theory of Non-Uniform Gases, 3rd edn. Cambridge.Google Scholar
Deegan, R. D., Bakajin, O., Dupont, T. F., Huber, G., Nagel, S. R. & Witten, T. A. 2000 Phys. Rev. E62, 756–765.Google Scholar
Doumenc, F. & Guerrier, B. 2010 Langmuir 26, 13959–13967.Google Scholar
Eggers, J. & Pismen, L. M. 2010 Phys. Fluids 22, 112101.CrossRefGoogle Scholar
Flaningam, O. L. 1986 J. Chem. Engng Data 31, 268–272.Google Scholar
Gee, M. L., Healy, T. W. & White, L. R. 1989 J. Colloid Interface Sci. 131, 18–23.Google Scholar
Gibbs, J. W. 1875 Trans. Conn. Acad. 3, 108–248; Collected Works, 1, p.160.Google Scholar
Guéna, G. 2007 Discussions sur l’évaporation d’une gouttelette mouillante. Thesis number tel-00292745 at ‘tel.archives-ouvertes.fr’.Google Scholar
Guéna, G., Allançon, P. & Cazabat, A.-M. 2007a Colloids Surf. A 300, 307–314.Google Scholar
Guéna, G., Poulard, C. & Cazabat, A.-M. 2007b. J. Colloid Interface Sci. 312, 164–171.Google Scholar
Israelachvili, J. 1991 Intermolecular and Surface Forces, 2nd edn. Academic.Google Scholar
Kelly-Zion, P. L., Batra, J. & Pursell, C. J. 2013 Intl J. Heat Mass Transfer 64, 278–285.Google Scholar
Landau, L. D. & Lifshitz, E. M. 1960 Electrodynamics of Continuous Media. Pergamon.Google Scholar
Levinson, P., Valignat, M. P., Fraysse, N., Cazabat, A. M. & Heslot, F. 1993 Thin Solid Films 234, 482–485.Google Scholar
Lindley, D. D. & Hershey, H. C. 1990 Fluid Phase Equilibria 55, 109–124.Google Scholar
Maczek, A. O. S. & Edwards, C. J. C. 1979 Viscosity and binary diffusion coefficients of some gaseous hydrocarbons, fluorocarbons and siloxanes. In Symposium on Transport Properties of Fluids and Fluid Mixtures, National Engineering Laboratory, East Kilbride, Glasgow.Google Scholar
Morris, S. J. S. 2001 J. Fluid Mech. 432, 1–30.Google Scholar
Njante, J.-P. 2012 Diffusion-controlled evaporating completely wetting meniscus in a channel. Ph.D. dissertation. University of California, Berkeley.Google Scholar
Park, T., Rettich, T. R., Battino, R. & Wilhelm, E. 1987 Mater. Chem. Phys. 15, 397–410.Google Scholar
Poulard, C., Guéna, G., Cazabat, A.-M., Boudaoud, A. & Ben Amar, M. 2005 Langmuir 21, 8226–8233.Google Scholar
Tee, L. S., Gotoh, S. & Stewart, W. E. 1966 Ind. Engng Chem. Fundam. 5, 356–363.Google Scholar
Thomson, W. 1872 Proc. R. Soc. Edin. 7, 63–68.Google Scholar
Truong, J. G. & Wayner, P. C. 1987 J. Chem. Phys. 87, 4180–4188.Google Scholar
Valignat, M. P., Fraysse, N., Cazabat, A.-M., Heslot, F. & Levinson, P. 1993 Thin Solid Films 234, 475–477.Google Scholar