Article contents
On the wall-bounded model of fingering double diffusive convection
Published online by Cambridge University Press: 20 October 2023
Abstract
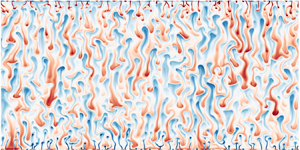
The present work aims at clarifying the effects of a solid boundary on the salt fingers in the wall-bounded double diffusive convection turbulence driven by the salinity and temperature differences between the top and bottom plates. The fluid properties are the same as the seawater, and two-dimensional direct numerical simulations are conducted over a wide range of the thermal and salinity Rayleigh numbers which measure the strength of driving salinity difference and stabilising temperature difference. We find that the bulk density ratio  $\varLambda _b$, defined by the mean temperature and salinity gradients at the bulk, controls the flow morphology. As
$\varLambda _b$, defined by the mean temperature and salinity gradients at the bulk, controls the flow morphology. As  $\varLambda _b$ exceeds unity, the bulk flow shifts from wide convection rolls to slender salt fingers. Two different regimes are further identified for the cases of salt-finger type. One is the confined salt-finger regime where the characteristic height of salt fingers is comparable to the bulk height and the influences of the solid boundary are noticeable. The other is the free salt-finger regime where the salt fingers are much shorter than the bulk height. In this latter regime, the transport properties versus
$\varLambda _b$ exceeds unity, the bulk flow shifts from wide convection rolls to slender salt fingers. Two different regimes are further identified for the cases of salt-finger type. One is the confined salt-finger regime where the characteristic height of salt fingers is comparable to the bulk height and the influences of the solid boundary are noticeable. The other is the free salt-finger regime where the salt fingers are much shorter than the bulk height. In this latter regime, the transport properties versus  $\varLambda _b$ are in quantitative agreement with those obtained in the fully periodic domain (e.g. Traxler et al., J. Fluid Mech., vol. 677, 2011, pp. 530–553). For a limited range of density ratio at the highest salinity Rayleigh number considered here, multiple states can be obtained from different initial conditions. The large-scale secondary instability and spontaneous formation of staircase from finger layers are not observed in the current study.
$\varLambda _b$ are in quantitative agreement with those obtained in the fully periodic domain (e.g. Traxler et al., J. Fluid Mech., vol. 677, 2011, pp. 530–553). For a limited range of density ratio at the highest salinity Rayleigh number considered here, multiple states can be obtained from different initial conditions. The large-scale secondary instability and spontaneous formation of staircase from finger layers are not observed in the current study.
JFM classification
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2023. Published by Cambridge University Press
References
- 4
- Cited by


