Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Alidoost, Mohammadali
and
Reza Pishevar, Ahmad
2018.
On Importance of the Surface Charge Transport Equation in Numerical Simulation of Drop Deformation in a Direct Current Field.
Journal of Fluids Engineering,
Vol. 140,
Issue. 12,
Mori, Yoichiro
and
Young, Y.-N.
2018.
From electrodiffusion theory to the electrohydrodynamics of leaky dielectrics through the weak electrolyte limit.
Journal of Fluid Mechanics,
Vol. 855,
Issue. ,
p.
67.
Lanauze, Javier A.
Sengupta, Rajarshi
Bleier, Blake J.
Yezer, Benjamin A.
Khair, Aditya S.
and
Walker, Lynn M.
2018.
Colloidal stability dictates drop breakup under electric fields.
Soft Matter,
Vol. 14,
Issue. 46,
p.
9351.
Poddar, Antarip
Mandal, Shubhadeep
Bandopadhyay, Aditya
and
Chakraborty, Suman
2018.
Sedimentation of a surfactant-laden drop under the influence of an electric field.
Journal of Fluid Mechanics,
Vol. 849,
Issue. ,
p.
277.
Behera, Nalinikanta
Mandal, Shubhadeep
and
Chakraborty, Suman
2019.
Electrohydrodynamic settling of drop in uniform electric field: beyond Stokes flow regime.
Journal of Fluid Mechanics,
Vol. 881,
Issue. ,
p.
498.
Poddar, Antarip
Mandal, Shubhadeep
Bandopadhyay, Aditya
and
Chakraborty, Suman
2019.
Electrical switching of a surfactant coated drop in Poiseuille flow.
Journal of Fluid Mechanics,
Vol. 870,
Issue. ,
p.
27.
Abbasi, Muhammad Salman
Song, Ryungeun
Kim, Sung-Min
Kim, Hyoungsoo
and
Lee, Jinkee
2019.
Mono-emulsion droplet stretching under direct current electric field.
Soft Matter,
Vol. 15,
Issue. 11,
p.
2328.
Abbasi, Muhammad Salman
Song, Ryungeun
and
Lee, Jinkee
2019.
Breakups of an encapsulated surfactant-laden aqueous droplet under a DC electric field.
Soft Matter,
Vol. 15,
Issue. 43,
p.
8905.
Abbasi, Muhammad Salman
Song, Ryungeun
Kim, Hyoungsoo
and
Lee, Jinkee
2019.
Multimodal breakup of a double emulsion droplet under an electric field.
Soft Matter,
Vol. 15,
Issue. 10,
p.
2292.
Wang, Qiming
Ma, Manman
and
Siegel, Michael
2019.
Deformation and stability of a viscous electrolyte drop in a uniform electric field.
Physical Review Fluids,
Vol. 4,
Issue. 5,
Vlahovska, Petia M.
2019.
Electrohydrodynamics of Drops and Vesicles.
Annual Review of Fluid Mechanics,
Vol. 51,
Issue. 1,
p.
305.
Abbasi, Muhammad
Song, Ryungeun
Cho, Seongsu
and
Lee, Jinkee
2020.
Electro-Hydrodynamics of Emulsion Droplets: Physical Insights to Applications.
Micromachines,
Vol. 11,
Issue. 10,
p.
942.
Luo, Kang
Wu, Jian
Yi, Hong-Liang
and
Tan, He-Ping
2020.
Numerical analysis of two-phase electrohydrodynamic flows in the presence of surface charge convection.
Physics of Fluids,
Vol. 32,
Issue. 12,
Nie, Qichun
Huang, Yongan
Yin, Zhouping
and
Fang, Haisheng
2020.
Large deformation of a conductive nanodroplet in a strong electric field.
Physics of Fluids,
Vol. 32,
Issue. 2,
Sengupta, Rajarshi
Walker, Lynn M.
and
Khair, Aditya S.
2020.
Deformation of a conducting drop in a randomly fluctuating electric field.
Physical Review Fluids,
Vol. 5,
Issue. 6,
Liu, Chunyan
Fan, Yu
and
Lin, Ping
2020.
Numerical investigation of a fractional diffusion model on circular comb-inward structure.
Applied Mathematics Letters,
Vol. 100,
Issue. ,
p.
106053.
Gawande, Neha
Mayya, Y. S.
and
Thaokar, Rochish
2020.
Jet and progeny formation in the Rayleigh breakup of a charged viscous drop.
Journal of Fluid Mechanics,
Vol. 884,
Issue. ,
Behera, Nalinikanta
and
Chakraborty, Suman
2020.
Effect of charge convection on gravitational settling of drop in uniform electric field.
Physics of Fluids,
Vol. 32,
Issue. 11,
Sorgentone, Chiara
Kach, Jeremy I.
Khair, Aditya S.
Walker, Lynn M.
and
Vlahovska, Petia M.
2021.
Numerical and asymptotic analysis of the three-dimensional electrohydrodynamic interactions of drop pairs.
Journal of Fluid Mechanics,
Vol. 914,
Issue. ,
Das, Santanu Kumar
Dalal, Amaresh
and
Tomar, Gaurav
2021.
Electrohydrodynamic-induced interactions between droplets.
Journal of Fluid Mechanics,
Vol. 915,
Issue. ,
 $Ca_{E}-Re_{E}$ parameter space, where
$Ca_{E}-Re_{E}$ parameter space, where 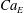 $Ca_{E}$ is the electric capillary number (ratio of the electric to capillary stresses); and
$Ca_{E}$ is the electric capillary number (ratio of the electric to capillary stresses); and  $Re_{E}$ is the electric Reynolds number (ratio of charge relaxation to flow time scales), which characterizes the strength of surface charge convection along the interface. For so-called ‘prolate A’ drops, where the surface charge is convected towards the ‘poles’ of the drop, we demonstrate that increasing
$Re_{E}$ is the electric Reynolds number (ratio of charge relaxation to flow time scales), which characterizes the strength of surface charge convection along the interface. For so-called ‘prolate A’ drops, where the surface charge is convected towards the ‘poles’ of the drop, we demonstrate that increasing 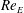 $Re_{E}$ reduces the critical capillary number for breakup. Moreover, surface charge convection is the cause of an abrupt transition in the breakup mode of a drop from end pinching, where the drop elongates and develops bulbs at its ends that eventually detach, to a breakup mode characterized by the formation of conical ends. On the contrary, the deformation of ‘prolate B’ drops, where the surface charge is convected away from the poles, is essentially unaffected by the magnitude of
$Re_{E}$ reduces the critical capillary number for breakup. Moreover, surface charge convection is the cause of an abrupt transition in the breakup mode of a drop from end pinching, where the drop elongates and develops bulbs at its ends that eventually detach, to a breakup mode characterized by the formation of conical ends. On the contrary, the deformation of ‘prolate B’ drops, where the surface charge is convected away from the poles, is essentially unaffected by the magnitude of 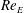 $Re_{E}$.
$Re_{E}$.