1. Introduction
Natural flyers and swimmers exhibit innovative flapping manoeuvres by exploiting the flexibility of their wings or fins in order to maximise the aerodynamic forces. These biological motions have significant potential to be mimicked and adapted in the design of bio-inspired miniature surveillance devices, such as micro aerial vehicles (MAVs) and autonomous underwater vehicles (AUVs). An appropriate understanding of the underlying flow physics is essential to achieve an efficient design. Studies on rigid flapping systems for different kinematics have already established that the key to optimal load generation lies in the ensuing vortex-dominated unsteady flow field surrounding them (Shyy, Berg & Ljungqvist Reference Shyy, Berg and Ljungqvist1999; Triantafyllou, Triantafyllou & Yue Reference Triantafyllou, Triantafyllou and Yue2000; Triantafyllou, Techet & Hover Reference Triantafyllou, Techet and Hover2004; Shyy et al. Reference Shyy, Aono, Chimakurthi, Trizila, Kang, Cesnik and Liu2010). Most of the flapping appendages (wings and fins) of biological flyers and swimmers typically possess both spanwise and chordwise flexibility of various degrees, and accordingly their deformation happens during flapping (Ennos Reference Ennos1988; Wootton Reference Wootton1999; Daniel & Combes Reference Daniel and Combes2002; Combes & Daniel Reference Combes and Daniel2003). Both experimental and numerical studies have demonstrated that elastic deformation of wings/fins has significant impact on their aerodynamic performances (Heathcote & Gursul Reference Heathcote and Gursul2007a; Heathcote, Wang & Gursul Reference Heathcote, Wang and Gursul2008; Vanella et al. Reference Vanella, Fitzgerald, Preidikman, Balaras and Balachandran2009; Mazaheri & Ebrahimi Reference Mazaheri and Ebrahimi2010; Kang et al. Reference Kang, Aono, Cesnik and Shyy2011; Ramananarivo, Godoy-Diana & Thiria Reference Ramananarivo, Godoy-Diana and Thiria2011). Results of rigid foils with a deformable tail at a certain flexibility level showing an enhanced efficiency of net power extraction through increased lift generation were presented by Heathcote & Gursul (Reference Heathcote and Gursul2007a), Ramananarivo et al. (Reference Ramananarivo, Godoy-Diana and Thiria2011) and Wu et al. (Reference Wu, Wu, Tian, Zhao and Li2015).
The existing literature so far has focused mainly on investigating the effects of flexibility from the purview of aerodynamic loads and propulsive efficiency. Despite the knowledge that flexibility may play a key role in achieving efficient flapping flights, the associated flow-field interaction mechanisms are not very clear. The role played by the near-field vortices, and the interactions between the unsteady flow and the flexible body, especially need a careful attention. Note that in natural flapping systems, the flexural rigidity in the spanwise direction is orders of magnitude higher than in the chordwise direction (Combes & Daniel Reference Combes and Daniel2003), hence the latter is expected to have a more prominent effect on the flow field and the dynamics. The present study investigates the effects of chordwise flexibility in modifying the near-field interactions with associated wake patterns as well as the related nonlinear dynamical states. A rigid flapping foil with a flexible aft-tail configuration has been considered, which provides a combined approach of exploiting the active control of the rigid foil and the passive deformation of the tail. Also, due to the fact that natural flyers often possess a relatively rigid wing root, the chosen configuration can be considered as a canonical model of a flapping system with varying levels of flexibility.
The wake transition behind rigid flapping foils has been studied comprehensively in the recent literature for different kinematics, such as pure heaving (Lai & Platzer Reference Lai and Platzer1999; Lewin & Haj-Hariri Reference Lewin and Haj-Hariri2003; Ashraf, Young & Lai Reference Ashraf, Young and Lai2012; Badrinath, Bose & Sarkar Reference Badrinath, Bose and Sarkar2017; Majumdar, Bose & Sarkar Reference Majumdar, Bose and Sarkar2020a,Reference Majumdar, Bose and Sarkarb) and pure pitching (Koochesfahani Reference Koochesfahani1989; Godoy-Diana, Aider & Wesfreid Reference Godoy-Diana, Aider and Wesfreid2008; Schnipper, Andersen & Bohr Reference Schnipper, Andersen and Bohr2009; Shinde & Arakeri Reference Shinde and Arakeri2013), as well as simultaneous heaving–pitching (Lentink et al. Reference Lentink, Van Heijst, Muijres and Van Leeuwen2010; Bose & Sarkar Reference Bose and Sarkar2018; Bose, Gupta & Sarkar Reference Bose, Gupta and Sarkar2021; Majumdar, Bose & Sarkar Reference Majumdar, Bose and Sarkar2022). The dynamic plunge velocity (![]() $\kappa h$) or Strouhal number (
$\kappa h$) or Strouhal number (![]() $St$) was used as the control parameter to study the transition; here,
$St$) was used as the control parameter to study the transition; here, ![]() $St=2f_s A/U_{\infty }$, where
$St=2f_s A/U_{\infty }$, where ![]() $A$ and
$A$ and ![]() $f_s$ are the plunge/heave amplitude and frequency, respectively;
$f_s$ are the plunge/heave amplitude and frequency, respectively; ![]() $h=A/c$ is the non-dimensional heave amplitude; and
$h=A/c$ is the non-dimensional heave amplitude; and ![]() $\kappa =2{\rm \pi} f_s c/U_{\infty }$ is the reduced frequency, with
$\kappa =2{\rm \pi} f_s c/U_{\infty }$ is the reduced frequency, with ![]() $c$ being the chord length of the foil. It is well established that the transition from Kármán to reverse Kármán vortex street, with increasing
$c$ being the chord length of the foil. It is well established that the transition from Kármán to reverse Kármán vortex street, with increasing ![]() $\kappa h$ or
$\kappa h$ or ![]() $St$, is accompanied closely by drag-to-thrust transition in the wake (Koochesfahani Reference Koochesfahani1989; Jones, Dohring & Platzer Reference Jones, Dohring and Platzer1998; Lai & Platzer Reference Lai and Platzer1999). At higher
$St$, is accompanied closely by drag-to-thrust transition in the wake (Koochesfahani Reference Koochesfahani1989; Jones, Dohring & Platzer Reference Jones, Dohring and Platzer1998; Lai & Platzer Reference Lai and Platzer1999). At higher ![]() $\kappa h$ or
$\kappa h$ or ![]() $St$ ranges, deflected jets through a symmetry-breaking bifurcation emerge, which is associated with increased thrust and a non-zero lift (Jones et al. Reference Jones, Dohring and Platzer1998; Lai & Platzer Reference Lai and Platzer1999; Godoy-Diana et al. Reference Godoy-Diana, Aider and Wesfreid2008). Note that in the periodic regime, a deflected jet remains stable as it does not undergo any temporal change in its deflection direction, which is dictated by the starting condition of the aerofoil motion. However, with further increase in
$St$ ranges, deflected jets through a symmetry-breaking bifurcation emerge, which is associated with increased thrust and a non-zero lift (Jones et al. Reference Jones, Dohring and Platzer1998; Lai & Platzer Reference Lai and Platzer1999; Godoy-Diana et al. Reference Godoy-Diana, Aider and Wesfreid2008). Note that in the periodic regime, a deflected jet remains stable as it does not undergo any temporal change in its deflection direction, which is dictated by the starting condition of the aerofoil motion. However, with further increase in ![]() $\kappa h$ or
$\kappa h$ or ![]() $St$, the periodicity of the wake is lost gradually. A variety of aperiodic states such as quasi-periodicity and intermittency emerge in this regime, through different local bifurcation routes (Badrinath et al. Reference Badrinath, Bose and Sarkar2017; Bose & Sarkar Reference Bose and Sarkar2018; Majumdar et al. Reference Majumdar, Bose and Sarkar2020a; Bose et al. Reference Bose, Gupta and Sarkar2021). As a result, spontaneous and repeated reversals in the deflection direction of the reverse Kármán street take place with time, a phenomenon known as jet-switching (Jones et al. Reference Jones, Dohring and Platzer1998; Heathcote & Gursul Reference Heathcote and Gursul2007b; Shinde & Arakeri Reference Shinde and Arakeri2013; Majumdar et al. Reference Majumdar, Bose and Sarkar2020b; Bose et al. Reference Bose, Gupta and Sarkar2021). Majumdar et al. (Reference Majumdar, Bose and Sarkar2020b) have shown that quasi-periodic movement of shed vortices acts as the primary trigger for facilitating jet-switching behind a purely heaving foil. In this regime, alternate opposite-sense ‘vortex pairing’ in the near wake was reported to be the underlying mechanism for triggering jet-switching. However, shedding of strong leading-edge vortices (LEVs) did not take place, and any active interactions with the trailing-edge vortices (TEVs) were also not observed. Hence the LEVs did not participate directly in triggering the switching. In contrast, for a simultaneous heaving–pitching aerofoil, the primary LEV was seen to play the key role behind jet-switching (Bose et al. Reference Bose, Gupta and Sarkar2021). In the quasi-periodic regime (
$St$, the periodicity of the wake is lost gradually. A variety of aperiodic states such as quasi-periodicity and intermittency emerge in this regime, through different local bifurcation routes (Badrinath et al. Reference Badrinath, Bose and Sarkar2017; Bose & Sarkar Reference Bose and Sarkar2018; Majumdar et al. Reference Majumdar, Bose and Sarkar2020a; Bose et al. Reference Bose, Gupta and Sarkar2021). As a result, spontaneous and repeated reversals in the deflection direction of the reverse Kármán street take place with time, a phenomenon known as jet-switching (Jones et al. Reference Jones, Dohring and Platzer1998; Heathcote & Gursul Reference Heathcote and Gursul2007b; Shinde & Arakeri Reference Shinde and Arakeri2013; Majumdar et al. Reference Majumdar, Bose and Sarkar2020b; Bose et al. Reference Bose, Gupta and Sarkar2021). Majumdar et al. (Reference Majumdar, Bose and Sarkar2020b) have shown that quasi-periodic movement of shed vortices acts as the primary trigger for facilitating jet-switching behind a purely heaving foil. In this regime, alternate opposite-sense ‘vortex pairing’ in the near wake was reported to be the underlying mechanism for triggering jet-switching. However, shedding of strong leading-edge vortices (LEVs) did not take place, and any active interactions with the trailing-edge vortices (TEVs) were also not observed. Hence the LEVs did not participate directly in triggering the switching. In contrast, for a simultaneous heaving–pitching aerofoil, the primary LEV was seen to play the key role behind jet-switching (Bose et al. Reference Bose, Gupta and Sarkar2021). In the quasi-periodic regime (![]() $\kappa h = 1.6$), Bose et al. (Reference Bose, Gupta and Sarkar2021) observed a spatial reversal in the deflection direction from near- to far-wake, resulting in an arc-shaped pattern in the wake. This particular phenomenon occurred in the far-wake region, and was referred to as far-wake switching. This was triggered by a quasi-periodic disturbance travelling from the LEV, and a subsequent breakdown of a secondary vortex street. A complete reversal in the trailing-edge couple's deflection direction was reported by the same authors at
$\kappa h = 1.6$), Bose et al. (Reference Bose, Gupta and Sarkar2021) observed a spatial reversal in the deflection direction from near- to far-wake, resulting in an arc-shaped pattern in the wake. This particular phenomenon occurred in the far-wake region, and was referred to as far-wake switching. This was triggered by a quasi-periodic disturbance travelling from the LEV, and a subsequent breakdown of a secondary vortex street. A complete reversal in the trailing-edge couple's deflection direction was reported by the same authors at ![]() $\kappa h=1.7$, and this was seen to be associated with the dynamical state of intermittency. The LEV played a crucial role behind the intermittent aperiodic interactions in the near field, enabling the dominant trailing-edge couple to reverse its deflection direction completely. Cleaver, Wang & Gursul (Reference Cleaver, Wang and Gursul2013) conducted experiments with both an NACA0012 aerofoil and a flat plate at Reynolds number
$\kappa h=1.7$, and this was seen to be associated with the dynamical state of intermittency. The LEV played a crucial role behind the intermittent aperiodic interactions in the near field, enabling the dominant trailing-edge couple to reverse its deflection direction completely. Cleaver, Wang & Gursul (Reference Cleaver, Wang and Gursul2013) conducted experiments with both an NACA0012 aerofoil and a flat plate at Reynolds number ![]() $10\,000$, and found that for
$10\,000$, and found that for ![]() $0^\circ$ angle-of-attack, NACA0012 produces a stable wake, whereas the flat plate exhibits jet-switching. It was reported that the jet-switching was triggered primarily by the LEV shedding. Note that most of the above studies considered rigid foils, and the role of flexibility behind the transitional wake dynamics did not receive any detailed attention in the literature. In light of the above, the present study focuses on exploring the effect of flexibility on the near-field interactions and jet-switching. None of the previous studies have reported the role of the system's flexibility on the inhibition of jet-switching, interlinking it with the nonlinear dynamical signature of the wake.
$0^\circ$ angle-of-attack, NACA0012 produces a stable wake, whereas the flat plate exhibits jet-switching. It was reported that the jet-switching was triggered primarily by the LEV shedding. Note that most of the above studies considered rigid foils, and the role of flexibility behind the transitional wake dynamics did not receive any detailed attention in the literature. In light of the above, the present study focuses on exploring the effect of flexibility on the near-field interactions and jet-switching. None of the previous studies have reported the role of the system's flexibility on the inhibition of jet-switching, interlinking it with the nonlinear dynamical signature of the wake.
The chordwise deformation in the wings/fins introduces additional passive pitching, changing the effective angle-of-attack quite significantly. This can alter considerably the transition scenario in the trailing wake. Existing studies that look into the wake-stabilising effects of flexibility are limited, with the notable exceptions of Marais et al. (Reference Marais, Thiria, Wesfreid and Godoy-Diana2012) and Shinde & Arakeri (Reference Shinde and Arakeri2014). Marais et al. (Reference Marais, Thiria, Wesfreid and Godoy-Diana2012) investigated the wake-transition of a chordwise flexible foil due to passive pitching, and showed that flexibility could inhibit the symmetry-breaking of a reverse Kármán wake and neutralise the deflection of a jet through strong fluid–structure interaction effects. It was also shown that flexibility could delay the onset of deflection by increasing the separation distance between successively shed TEVs. This is experimental evidence in support of the role of flexibility in altering the wake patterns behind a flapping foil. Heathcote & Gursul (Reference Heathcote and Gursul2007a,Reference Heathcote and Gursulb) observed experimentally the presence of jet-switching for a heaving rigid teardrop-shaped foil with a chordwise flexible tail in the quiescent flow condition. The authors observed a quasi-periodic switching pattern, in contrast to the aperiodic pattern reported earlier (Jones et al. Reference Jones, Dohring and Platzer1998). Heathcote & Gursul (Reference Heathcote and Gursul2007a,Reference Heathcote and Gursulb) did not find any important effect of the flexibility on the jet-switching behaviour in the range of flexibility parameters considered in their study. On the other hand, Shinde & Arakeri (Reference Shinde and Arakeri2014) showed that in the quiescent flow condition, a flexible tail of appropriate stiffness could suppress jet-switching by generating a narrow jet, whereas a meandering jet was produced in the absence of flexibility. However, the underlying near-field interactions behind the suppression of switching need more attention and are yet to be clearly understood.
The above studies point out that flexibility can play a crucial role in dictating and modifying the qualitative wake patterns. These observations provide an additional motivation to us to revisit our recently observed dynamical transition routes (Badrinath et al. Reference Badrinath, Bose and Sarkar2017; Bose & Sarkar Reference Bose and Sarkar2018; Majumdar et al. Reference Majumdar, Bose and Sarkar2020a; Bose et al. Reference Bose, Gupta and Sarkar2021) in the light of introducing flexibility. Specifically, the role of a chordwise flexible aft-tail in changing the wake patterns of a heaving foil by affecting the near-field interactions among the primary wake vortices is focused upon. The effect of additional passive pitching of a flexible tail structure on the nonlinear dynamical signature of the wake is also investigated, which has not been reported in the literature to the best of our knowledge. The primary contents of the present study are: (i) studying the role played by a chordwise flexible aft-tail in altering the qualitative patterns of the trailing wake (such as jet-switching) in comparison to a rigid configuration; (ii) investigating the associated underlying nonlinear dynamical states and bifurcations in the flow field; (iii) understanding the role of deformation of the flexible tail in influencing the leading-edge separation behaviour and the subsequent near-field interactions. To achieve these goals, the flow-field transition for a rigid tail configuration with an increasing dynamic plunge velocity (![]() $\kappa h$) is studied first. Thereafter, the efficacy of a flexible tail in reinstating the periodicity of the wake by eliminating the jet-switching phenomena is analysed by varying the degree of flexibility. The degree of flexibility is changed in terms of the bending rigidity for a fixed aft-tail length, and subsequently, it is changed by altering the length for a fixed bending rigidity. The underlying nonlinear dynamics behind jet-switching and its regularisation is examined thoroughly using robust time series analysis tools. At this stage, the scope of two-dimensional (2-D) studies in relation to realistic three-dimensional (3-D) engineering applications should also be discussed. Deng, Sun & Shao (Reference Deng, Sun and Shao2015) and Sun, Deng & Shao (Reference Sun, Deng and Shao2018) presented the boundary of the 2-D to 3-D transition as a function of non-dimensional stroke amplitude and Strouhal number for the rigid flapping aerofoils using Floquet stability analysis. The kinematic parameters for the present study are chosen in such a way that they lie well inside the 2-D regime. Furthermore, the present study considers so-called high-amplitude/low-frequency flapping, which has been shown to possess coherent LEVs intact in 3-D even at high Strouhal numbers, showing good agreement with the 2-D results (Ashraf et al. Reference Ashraf, Young and Lai2012). However, one should be cautious that the 2-D simulations might fail at high Strouhal numbers for the other class of problems (low-amplitude and high-frequency cases), that may encounter significant spanwise perturbation in the wake (Visbal Reference Visbal2009). Also, in relation to realistic 3-D designs, 2-D results alone may be inadequate for low-aspect-ratio flappers due to the significant tip-vortex effects (Gursul & Cleaver Reference Gursul and Cleaver2019).
$\kappa h$) is studied first. Thereafter, the efficacy of a flexible tail in reinstating the periodicity of the wake by eliminating the jet-switching phenomena is analysed by varying the degree of flexibility. The degree of flexibility is changed in terms of the bending rigidity for a fixed aft-tail length, and subsequently, it is changed by altering the length for a fixed bending rigidity. The underlying nonlinear dynamics behind jet-switching and its regularisation is examined thoroughly using robust time series analysis tools. At this stage, the scope of two-dimensional (2-D) studies in relation to realistic three-dimensional (3-D) engineering applications should also be discussed. Deng, Sun & Shao (Reference Deng, Sun and Shao2015) and Sun, Deng & Shao (Reference Sun, Deng and Shao2018) presented the boundary of the 2-D to 3-D transition as a function of non-dimensional stroke amplitude and Strouhal number for the rigid flapping aerofoils using Floquet stability analysis. The kinematic parameters for the present study are chosen in such a way that they lie well inside the 2-D regime. Furthermore, the present study considers so-called high-amplitude/low-frequency flapping, which has been shown to possess coherent LEVs intact in 3-D even at high Strouhal numbers, showing good agreement with the 2-D results (Ashraf et al. Reference Ashraf, Young and Lai2012). However, one should be cautious that the 2-D simulations might fail at high Strouhal numbers for the other class of problems (low-amplitude and high-frequency cases), that may encounter significant spanwise perturbation in the wake (Visbal Reference Visbal2009). Also, in relation to realistic 3-D designs, 2-D results alone may be inadequate for low-aspect-ratio flappers due to the significant tip-vortex effects (Gursul & Cleaver Reference Gursul and Cleaver2019).
The rest of the paper is arranged as follows. In § 2, the structural model and the flow solver are discussed along with convergence tests and validation studies. Section 3 reports the dynamical transition and the presence of the jet-switching phenomenon for the rigid aft-tail configuration. The effect of tail flexibility in altering the same is presented in §§ 4.1 and 4.2. A comparison of the wake for different flexibility levels of the tail in terms of suppression of jet-switching is presented in § 4.3. The effect of the aft-tail length on the flow-field dynamics and its associated mechanism in stabilising the wake is presented in § 4.6. Finally, the salient outcomes of this study and the conclusions are drawn in § 5.
2. Numerical methodology
A rigid elliptic foil of major axis length ![]() $c$, with a chordwise flexible aft-tail of length
$c$, with a chordwise flexible aft-tail of length ![]() $0.3c$, is considered to model a 2-D flexible wing configuration, as shown in figure 1(a). The thickness to chord ratio of the elliptic section (marked ‘I’ in figure 1a) is
$0.3c$, is considered to model a 2-D flexible wing configuration, as shown in figure 1(a). The thickness to chord ratio of the elliptic section (marked ‘I’ in figure 1a) is ![]() $0.12$. The Reynolds number is chosen as
$0.12$. The Reynolds number is chosen as ![]() $Re = 300$. The flow field around the flapping wing is governed by the incompressible Navier–Stokes (N–S) equations. A discrete forcing IBM-based in-house N–S solver is used to simulate the flow field (Majumdar et al. Reference Majumdar, Bose and Sarkar2020a). Computationally intensive parts of the flow solver have been parallelised using the OpenMP technique to enhance the computational speed; see Shah, Majumdar & Sarkar (Reference Shah, Majumdar and Sarkar2019). Necessary numerical details of the structural motion, flow solver and fluid–structure coupling are given in the following subsections.
$Re = 300$. The flow field around the flapping wing is governed by the incompressible Navier–Stokes (N–S) equations. A discrete forcing IBM-based in-house N–S solver is used to simulate the flow field (Majumdar et al. Reference Majumdar, Bose and Sarkar2020a). Computationally intensive parts of the flow solver have been parallelised using the OpenMP technique to enhance the computational speed; see Shah, Majumdar & Sarkar (Reference Shah, Majumdar and Sarkar2019). Necessary numerical details of the structural motion, flow solver and fluid–structure coupling are given in the following subsections.

Figure 1. (a) A schematic view of the wing configuration; the ‘I’ and ‘II’ sections are the rigid foil and flexible tail portions, respectively. (b) The computational domain and the boundary conditions (not to scale).
2.1. Structural governing equations
A sinusoidal heaving motion: ![]() $y_c(t)=h\sin (\kappa t)$ is imposed at the centre of the elliptic foil (figure 1b). Here,
$y_c(t)=h\sin (\kappa t)$ is imposed at the centre of the elliptic foil (figure 1b). Here, ![]() $y_c(t)$ is the instantaneous position of the centre of a foil in the non-dimensional form,
$y_c(t)$ is the instantaneous position of the centre of a foil in the non-dimensional form, ![]() $t$ being the time non-dimensionalised by
$t$ being the time non-dimensionalised by ![]() $c/U_{\infty }$ (where
$c/U_{\infty }$ (where ![]() $U_{\infty }$ is the free-stream velocity);
$U_{\infty }$ is the free-stream velocity); ![]() $h$ and
$h$ and ![]() $\kappa$ denote the non-dimensional heaving amplitude and frequency, respectively. All the quantities throughout this paper are non-dimensionalised, considering
$\kappa$ denote the non-dimensional heaving amplitude and frequency, respectively. All the quantities throughout this paper are non-dimensionalised, considering ![]() $c$ and
$c$ and ![]() $U_{\infty }$ as the reference length and velocity scales, respectively. The flexible tail portion (marked ‘II’ in figure 1a) is modelled as an inextensible filament that is allowed to undergo a passive oscillation. In the non-dimensional form, the governing equation of the tail motion is given by
$U_{\infty }$ as the reference length and velocity scales, respectively. The flexible tail portion (marked ‘II’ in figure 1a) is modelled as an inextensible filament that is allowed to undergo a passive oscillation. In the non-dimensional form, the governing equation of the tail motion is given by
where, ![]() $s$ denotes the arc length,
$s$ denotes the arc length, ![]() $\boldsymbol {X}=(X(s,t),Y(s,t))$ is the position of the filament,
$\boldsymbol {X}=(X(s,t),Y(s,t))$ is the position of the filament, ![]() $T_s$ is the tension coefficient along the filament,
$T_s$ is the tension coefficient along the filament, ![]() $\gamma$ is the bending rigidity, and
$\gamma$ is the bending rigidity, and ![]() $\boldsymbol {F}$ indicates the fluid force acting on the filament. These non-dimensional quantities are obtained by using characteristic scales
$\boldsymbol {F}$ indicates the fluid force acting on the filament. These non-dimensional quantities are obtained by using characteristic scales ![]() $\rho _f U_{\infty }^2$ for the fluid force,
$\rho _f U_{\infty }^2$ for the fluid force, ![]() $\rho _f U_{\infty }^2 c$ for the tension coefficient, and
$\rho _f U_{\infty }^2 c$ for the tension coefficient, and ![]() $\rho _f U_{\infty }^2 c^3$ for the bending rigidity. The mass ratio is defined as
$\rho _f U_{\infty }^2 c^3$ for the bending rigidity. The mass ratio is defined as ![]() $\beta ={\rho _s t_h}/{\rho _f c}$, where
$\beta ={\rho _s t_h}/{\rho _f c}$, where ![]() $\rho _s$ and
$\rho _s$ and ![]() $\rho _f$ are the solid and fluid densities, respectively;
$\rho _f$ are the solid and fluid densities, respectively; ![]() $t_h$ (
$t_h$ (![]() $= 0.02c$) is the thickness of the tail structure;
$= 0.02c$) is the thickness of the tail structure; ![]() $Fr={{g} c}/{U_{\infty }^2}$ is the Froude number, with
$Fr={{g} c}/{U_{\infty }^2}$ is the Froude number, with ![]() ${g}=|\boldsymbol {g}|$ being gravitational force. It is to be noted that the gravity term is included here in the structural governing equations alone, solely for the validation of the present solver against the available literature. However, it is not considered for the present fluid–structure interaction (FSI) simulations throughout the study, following the work of Zhu, He & Zhang (Reference Zhu, He and Zhang2014). In order to maintain a constant length of the tail, the inextensibility condition is implemented as
${g}=|\boldsymbol {g}|$ being gravitational force. It is to be noted that the gravity term is included here in the structural governing equations alone, solely for the validation of the present solver against the available literature. However, it is not considered for the present fluid–structure interaction (FSI) simulations throughout the study, following the work of Zhu, He & Zhang (Reference Zhu, He and Zhang2014). In order to maintain a constant length of the tail, the inextensibility condition is implemented as
In (2.1), ![]() $\gamma$ is assumed to be constant. Here,
$\gamma$ is assumed to be constant. Here, ![]() $T_s$ is a function of both
$T_s$ is a function of both ![]() $s$ and
$s$ and ![]() $t$, hence
$t$, hence ![]() $T_s$ is determined by substituting the inextensibility constraint (2.2) in (2.1). This results in a Poisson equation for
$T_s$ is determined by substituting the inextensibility constraint (2.2) in (2.1). This results in a Poisson equation for ![]() $T_s$:
$T_s$:
where ![]() $\boldsymbol {F}_b=-({\partial ^2}/{\partial s^2})(\gamma ({\partial ^2\boldsymbol {X}}/{\partial s^2}))$ denotes the bending force. For more details of the formulation of the above equations, one can refer to the work of Huang, Shin & Sung (Reference Huang, Shin and Sung2007). Note that the non-dimensional reference scales used in this work are different to what was used by Huang et al. (Reference Huang, Shin and Sung2007).
$\boldsymbol {F}_b=-({\partial ^2}/{\partial s^2})(\gamma ({\partial ^2\boldsymbol {X}}/{\partial s^2}))$ denotes the bending force. For more details of the formulation of the above equations, one can refer to the work of Huang, Shin & Sung (Reference Huang, Shin and Sung2007). Note that the non-dimensional reference scales used in this work are different to what was used by Huang et al. (Reference Huang, Shin and Sung2007).
At ![]() $t = 0$, the leading edge of the elliptic foil lies at the origin, with the tail at rest in the horizontal position. The boundary conditions for the aft-tail are as follows: at the free end
$t = 0$, the leading edge of the elliptic foil lies at the origin, with the tail at rest in the horizontal position. The boundary conditions for the aft-tail are as follows: at the free end ![]() $(s=1.3c)$,
$(s=1.3c)$,
and at the fixed end ![]() $(s=c)$,
$(s=c)$,
where ![]() $\boldsymbol {X}_0=(c,y_c(t))$ is the position of the leading edge of the aft-tail. Equations (2.1) and (2.3) are solved using a finite difference technique (Huang et al. Reference Huang, Shin and Sung2007). In order to perform the finite difference discretisation for the structural equations, the flexible filament is divided into
$\boldsymbol {X}_0=(c,y_c(t))$ is the position of the leading edge of the aft-tail. Equations (2.1) and (2.3) are solved using a finite difference technique (Huang et al. Reference Huang, Shin and Sung2007). In order to perform the finite difference discretisation for the structural equations, the flexible filament is divided into ![]() $N$ solid elements, each having width
$N$ solid elements, each having width ![]() $\Delta s$ and thickness
$\Delta s$ and thickness ![]() $t_h$, as shown in figure 2.
$t_h$, as shown in figure 2.

Figure 2. (a) Schematic sketch of the flexible filament structure along with the background Eulerian mesh. (b) Spatial discretisation strategy of the filament.
2.2. Flow solver
In the present IBM solver framework, a momentum forcing ![]() $\boldsymbol {f}$ is applied throughout the solid domain to enforce the no-slip, no-penetration boundary condition exactly on the solid boundary (Majumdar et al. Reference Majumdar, Bose and Sarkar2020a). In order to ensure rigorous mass conservation across the immersed boundary, a source/sink term
$\boldsymbol {f}$ is applied throughout the solid domain to enforce the no-slip, no-penetration boundary condition exactly on the solid boundary (Majumdar et al. Reference Majumdar, Bose and Sarkar2020a). In order to ensure rigorous mass conservation across the immersed boundary, a source/sink term ![]() $q$ is added to the continuity equation. Hence the flow governing equations take the form
$q$ is added to the continuity equation. Hence the flow governing equations take the form
Here, ![]() $\boldsymbol {u}$ denotes the flow velocity vector non-dimensionalised by
$\boldsymbol {u}$ denotes the flow velocity vector non-dimensionalised by ![]() $U_\infty$,
$U_\infty$, ![]() $p$ is the pressure non-dimensionalised by
$p$ is the pressure non-dimensionalised by ![]() $\rho _f U_\infty ^2$, and the Reynolds number is
$\rho _f U_\infty ^2$, and the Reynolds number is ![]() $Re = {U_\infty c}/{\nu }$. Equations (2.6) and (2.7) are solved on a background Eulerian grid using a finite-volume-based semi-implicit fractional step method. The diffusion term is discretised using the second-order Crank–Nicolson method, and Adams–Bashforth discretisation is used for the convection term. At every time step, the velocity is corrected using a pseudo-pressure-correction term. Note that for a grid cell entirely in the fluid domain, which has none of the cell faces inside the solid domain,
$Re = {U_\infty c}/{\nu }$. Equations (2.6) and (2.7) are solved on a background Eulerian grid using a finite-volume-based semi-implicit fractional step method. The diffusion term is discretised using the second-order Crank–Nicolson method, and Adams–Bashforth discretisation is used for the convection term. At every time step, the velocity is corrected using a pseudo-pressure-correction term. Note that for a grid cell entirely in the fluid domain, which has none of the cell faces inside the solid domain, ![]() $\boldsymbol {f}$ and
$\boldsymbol {f}$ and ![]() $q$ are considered to be zero. For grid locations inside the solid domain, they take non-zero values. Further details of the flow solver can be found in Kim, Kim & Choi (Reference Kim, Kim and Choi2001), Lee & Choi (Reference Lee and Choi2015) and Majumdar et al. (Reference Majumdar, Bose and Sarkar2020a).
$q$ are considered to be zero. For grid locations inside the solid domain, they take non-zero values. Further details of the flow solver can be found in Kim, Kim & Choi (Reference Kim, Kim and Choi2001), Lee & Choi (Reference Lee and Choi2015) and Majumdar et al. (Reference Majumdar, Bose and Sarkar2020a).
The fluid force acting on each solid element is calculated using (Lee et al. Reference Lee, Kim, Choi and Yang2011)
where ![]() $\Delta V_i$ denotes the control volume of each solid element. In figure 2,
$\Delta V_i$ denotes the control volume of each solid element. In figure 2, ![]() $\boldsymbol {X}_i$ shows the centre of a typical solid element, and the region bounded by
$\boldsymbol {X}_i$ shows the centre of a typical solid element, and the region bounded by ![]() $PQRS$ indicates the corresponding control volume. The present formulation requires the size of the Eulerian grid near the solid domain to be much smaller than a solid element, ensuring a sufficient number of fluid cells within a solid element (figure 2a), minimising the error involved in computing the volume integrals. The overall lift and drag coefficients,
$PQRS$ indicates the corresponding control volume. The present formulation requires the size of the Eulerian grid near the solid domain to be much smaller than a solid element, ensuring a sufficient number of fluid cells within a solid element (figure 2a), minimising the error involved in computing the volume integrals. The overall lift and drag coefficients, ![]() $C_L$ and
$C_L$ and ![]() $C_D$, respectively, are evaluated by performing the integration given in (2.8) over the entire solid domain. Further details can be found in the studies of Lee & Choi (Reference Lee and Choi2015) and Majumdar et al. (Reference Majumdar, Bose and Sarkar2020a).
$C_D$, respectively, are evaluated by performing the integration given in (2.8) over the entire solid domain. Further details can be found in the studies of Lee & Choi (Reference Lee and Choi2015) and Majumdar et al. (Reference Majumdar, Bose and Sarkar2020a).
The present FSI framework consists of a partitioned weak coupling strategy. In this approach, the flow governing equations are solved to get the flow field around the body at every time step, and the aerodynamic loads acting on the body are evaluated. These loads are then supplied to the structural solver to compute the position/shape of the body for the next time step, and the flow field is then solved with the modified position/shape of the structure. Thus at every time step, the flow and the structural solvers exchange information in a staggered manner.
2.3. Convergence study
Schematic representations of the rectangular computational domain and the non-uniform structured Cartesian mesh used in this study are shown in figures 1(b) and 3, respectively. The mesh size is uniform in the near-body region and then increases gradually towards the outer boundaries. The size of the flow domain is chosen to be sufficiently large so that the boundary effects are redundant. A Dirichlet-type boundary condition is applied at the inlet, a slip boundary condition is implemented at the upper and lower boundaries, and a Neumann-type boundary condition is employed at the outlet. A total of ![]() $1520$ Lagrangian markers are used to represent the solid surface. The optimum size of the discretised element for the flexible tail structure to solve the structural equations has been selected after performing a convergence study with the following parametric combination:
$1520$ Lagrangian markers are used to represent the solid surface. The optimum size of the discretised element for the flexible tail structure to solve the structural equations has been selected after performing a convergence study with the following parametric combination: ![]() $h=0.25$,
$h=0.25$, ![]() $\kappa = 4.0$,
$\kappa = 4.0$, ![]() $\gamma =0.1$,
$\gamma =0.1$, ![]() $\beta =1.0$,
$\beta =1.0$, ![]() $Fr=0.0$,
$Fr=0.0$, ![]() $\boldsymbol {g}/{g}=(0,0)$ and
$\boldsymbol {g}/{g}=(0,0)$ and ![]() $Re=300$. Three different sizes of the structural element,
$Re=300$. Three different sizes of the structural element, ![]() $\Delta s = 0.05,0.02,0.005$, have been considered. The structural element length independence test is performed with
$\Delta s = 0.05,0.02,0.005$, have been considered. The structural element length independence test is performed with ![]() $\Delta x=\Delta y=0.005$ and
$\Delta x=\Delta y=0.005$ and ![]() $\Delta t = 0.0004$. The corresponding time histories of the free-end tip deflection of the flexible aft-tail (
$\Delta t = 0.0004$. The corresponding time histories of the free-end tip deflection of the flexible aft-tail (![]() $Y_{te}$) are compared in figure 4. The results obtained for
$Y_{te}$) are compared in figure 4. The results obtained for ![]() $\Delta s = 0.02$ and
$\Delta s = 0.02$ and ![]() $0.005$ are seen to be in very good agreement with each other. Therefore, the element size for the discretisation of the flexible tail structure is considered to be
$0.005$ are seen to be in very good agreement with each other. Therefore, the element size for the discretisation of the flexible tail structure is considered to be ![]() $0.02$ for the rest of the simulations in the present study.
$0.02$ for the rest of the simulations in the present study.

Figure 3. Meshing strategy: (a) Cartesian grid, (b) zoomed section of the background grid around the foil with a flexible tail, and (c) uniform mesh grid near the body.

Figure 4. Convergence study for the number of elements considered for the trailing edge.
The flow domain is discretised into a mesh consisting of ![]() $N_x \times N_y$ grid points, where
$N_x \times N_y$ grid points, where ![]() $N_x$ and
$N_x$ and ![]() $N_y$ indicate the numbers of Cartesian grid points along the streamwise and transverse directions, respectively. Four different meshes, Grid-1, Grid-2, Grid-3 and Grid-4, respectively having minimum grid sizes
$N_y$ indicate the numbers of Cartesian grid points along the streamwise and transverse directions, respectively. Four different meshes, Grid-1, Grid-2, Grid-3 and Grid-4, respectively having minimum grid sizes ![]() $\Delta x=\Delta y=0.01$,
$\Delta x=\Delta y=0.01$, ![]() $\Delta x=\Delta y=0.008$,
$\Delta x=\Delta y=0.008$, ![]() $\Delta x=\Delta y=0.005$ and
$\Delta x=\Delta y=0.005$ and ![]() $\Delta x=\Delta y=0.002$, are considered. The corresponding total numbers of grid points
$\Delta x=\Delta y=0.002$, are considered. The corresponding total numbers of grid points ![]() $N_x \times N_y$ are
$N_x \times N_y$ are ![]() $546 \times 748$,
$546 \times 748$, ![]() $614 \times 840$,
$614 \times 840$, ![]() $800 \times 1080$ and
$800 \times 1080$ and ![]() $1440 \times 1860$. The structural element size and time step are kept at
$1440 \times 1860$. The structural element size and time step are kept at ![]() $\Delta s = 0.02$ and
$\Delta s = 0.02$ and ![]() $\Delta t = 0.0004$, respectively. The aerodynamic load coefficients obtained from these four grids are compared in figure 5. Time evolutions of
$\Delta t = 0.0004$, respectively. The aerodynamic load coefficients obtained from these four grids are compared in figure 5. Time evolutions of ![]() $C_L$ and
$C_L$ and ![]() $C_D$ obtained from Grid-3 match closely with those obtained from Grid-4. Therefore, Grid-3 is chosen for all further simulations.
$C_D$ obtained from Grid-3 match closely with those obtained from Grid-4. Therefore, Grid-3 is chosen for all further simulations.

Figure 5. Grid convergence study: (a) lift coefficient, and (b) drag coefficient.
To test the time convergence, four different time steps are considered: ![]() $\Delta t=0.001$,
$\Delta t=0.001$, ![]() $0.0007$,
$0.0007$, ![]() $0.0004$ and
$0.0004$ and ![]() $0.0001$ at
$0.0001$ at ![]() $\Delta s = 0.02$ and
$\Delta s = 0.02$ and ![]() $\Delta x=\Delta y=0.005$. The corresponding
$\Delta x=\Delta y=0.005$. The corresponding ![]() $C_L$ and
$C_L$ and ![]() $C_D$ time histories are compared in figures 6(a) and 6(b), respectively. The results obtained for
$C_D$ time histories are compared in figures 6(a) and 6(b), respectively. The results obtained for ![]() $\Delta t=0.0004$ and
$\Delta t=0.0004$ and ![]() $0.0001$ are seen to be in very good agreement with each other. Therefore, the time step
$0.0001$ are seen to be in very good agreement with each other. Therefore, the time step ![]() $\Delta t=0.0004$ is selected for the rest of the computations.
$\Delta t=0.0004$ is selected for the rest of the computations.

Figure 6. Time step convergence study: (a) lift coefficient, and (b) drag coefficient.
2.4. Validation of the FSI solver
An extensive qualitative and quantitative validation of the flow solver has been presented by the authors in their recent study (Majumdar et al. Reference Majumdar, Bose and Sarkar2020a), and therefore is not repeated here for the sake of brevity. Instead, the validation results for the structural response and the coupled FSI response are presented in two stages: first, the structural solver is validated, and next, the validation of the coupled FSI solver is presented using structural displacement as well as flow-field and aerodynamic loads. For validating the structural solver alone, a flexible filament hanging under the gravitational force is considered in the absence of any ambient fluid. The time history of the free-end displacement of the filament (![]() $Y_{te}$) obtained from the present solver is compared with the results presented by Huang et al. (Reference Huang, Shin and Sung2007) in figure 7(a). A close match between these results confirms the capability of the structural solver.
$Y_{te}$) obtained from the present solver is compared with the results presented by Huang et al. (Reference Huang, Shin and Sung2007) in figure 7(a). A close match between these results confirms the capability of the structural solver.

Figure 7. Validation of the present solver set-up in terms of the free-end tip displacement: (a) for a flexible filament hanging under the gravitational force in the absence of ambient fluid at ![]() $\gamma = 0.01$,
$\gamma = 0.01$, ![]() $Fr = 10$,
$Fr = 10$, ![]() $\boldsymbol {g}/{g}=(1,0)$,
$\boldsymbol {g}/{g}=(1,0)$, ![]() $L = 1$ and
$L = 1$ and ![]() $N = 100$; and (b) for the FSI behaviour of a flexible filament in the presence of upstream flow at
$N = 100$; and (b) for the FSI behaviour of a flexible filament in the presence of upstream flow at ![]() $Re=200$,
$Re=200$, ![]() $\gamma =0.0015$,
$\gamma =0.0015$, ![]() $\beta =1.5$,
$\beta =1.5$, ![]() $Fr=0.5$,
$Fr=0.5$, ![]() $\boldsymbol {g}/{g}=(1,0)$,
$\boldsymbol {g}/{g}=(1,0)$, ![]() $L=1.0$ and
$L=1.0$ and ![]() $N=100$. Parametric symbols follow the definitions given by Huang et al. Reference Huang, Shin and Sung2007.
$N=100$. Parametric symbols follow the definitions given by Huang et al. Reference Huang, Shin and Sung2007.
The coupled FSI solver is validated first by simulating the interaction of a flexible filament with the surrounding free stream at ![]() $Re=200$. Figure 7(b) shows a comparison of the time history of
$Re=200$. Figure 7(b) shows a comparison of the time history of ![]() $Y_{te}$ obtained from present solver with the results from the works of Huang et al. (Reference Huang, Shin and Sung2007) and Lee & Choi (Reference Lee and Choi2015). The
$Y_{te}$ obtained from present solver with the results from the works of Huang et al. (Reference Huang, Shin and Sung2007) and Lee & Choi (Reference Lee and Choi2015). The ![]() $C_L$ and
$C_L$ and ![]() $C_D$ time histories are also compared with the results presented by Lee & Choi (Reference Lee and Choi2015) in figures 8(a) and 8(b), respectively. It is evident from figures 7(b) and 8 that the present FSI results are in very good agreement with these earlier published data, establishing the validity of the current FSI solver. A qualitative comparison of the instantaneous flow-field data with that presented by Lee & Choi (Reference Lee and Choi2015) is also given in figure 9. A close match between the two further confirms the efficacy of the present in-house FSI solver.
$C_D$ time histories are also compared with the results presented by Lee & Choi (Reference Lee and Choi2015) in figures 8(a) and 8(b), respectively. It is evident from figures 7(b) and 8 that the present FSI results are in very good agreement with these earlier published data, establishing the validity of the current FSI solver. A qualitative comparison of the instantaneous flow-field data with that presented by Lee & Choi (Reference Lee and Choi2015) is also given in figure 9. A close match between the two further confirms the efficacy of the present in-house FSI solver.

Figure 8. Validation of the present solver set-up in terms of the aerodynamic loads: (a) lift coefficient and (b) drag coefficient time histories of a flexible filament undergoing FSI with the surrounding free stream at ![]() $Re=200$,
$Re=200$, ![]() $\gamma =0.0015$,
$\gamma =0.0015$, ![]() $\beta =1.5$,
$\beta =1.5$, ![]() $Fr=0.5$,
$Fr=0.5$, ![]() $\boldsymbol {g}/{g}=(1,0)$,
$\boldsymbol {g}/{g}=(1,0)$, ![]() $L=1.0$ and
$L=1.0$ and ![]() $N=100$.
$N=100$.

Figure 9. Comparison of vorticity fields between that from Lee & Choi (Reference Lee and Choi2015) (a–d) and the present simulation (e–h) of a flexible filament undergoing FSI with the surrounding free stream at ![]() $Re=200$,
$Re=200$, ![]() $\gamma =0.0015$,
$\gamma =0.0015$, ![]() $\beta =1.5$,
$\beta =1.5$, ![]() $Fr=0.5$,
$Fr=0.5$, ![]() $\boldsymbol {g}/{g}=(1,0)$,
$\boldsymbol {g}/{g}=(1,0)$, ![]() $L=1.0$ and
$L=1.0$ and ![]() $N=100$. Permission for reproducing the figures from Lee & Choi (Reference Lee and Choi2015) has been obtained from the publisher.
$N=100$. Permission for reproducing the figures from Lee & Choi (Reference Lee and Choi2015) has been obtained from the publisher.
To add further quantitative validation, the flow past a NACA0015 aerofoil with a flexible aft-tail, a configuration similar to the present study, is simulated and compared with the computational results of Wu et al. (Reference Wu, Wu, Tian, Zhao and Li2015). The Reynolds number is ![]() $Re=1100$, and the foil undergoes an active pitching and an induced heaving motion. Results from the present simulations are compared with Wu et al. (Reference Wu, Wu, Tian, Zhao and Li2015), in terms of instantaneous lift coefficient
$Re=1100$, and the foil undergoes an active pitching and an induced heaving motion. Results from the present simulations are compared with Wu et al. (Reference Wu, Wu, Tian, Zhao and Li2015), in terms of instantaneous lift coefficient ![]() $C_L$ and its root-mean-square values,
$C_L$ and its root-mean-square values, ![]() $(C_L)_{rms}$, in figures 10(a) and 10(b), respectively. The present simulations corroborate well with Wu et al. (Reference Wu, Wu, Tian, Zhao and Li2015), providing further quantitative validation in the concerned Reynolds number regime.
$(C_L)_{rms}$, in figures 10(a) and 10(b), respectively. The present simulations corroborate well with Wu et al. (Reference Wu, Wu, Tian, Zhao and Li2015), providing further quantitative validation in the concerned Reynolds number regime.

Figure 10. (a) Comparison of lift coefficient ![]() $C_L$ for
$C_L$ for ![]() $\theta _m=20^{\circ }$,
$\theta _m=20^{\circ }$, ![]() $m_t^*=5$,
$m_t^*=5$, ![]() $\omega ^*=0.4$ and
$\omega ^*=0.4$ and ![]() $f^*=0.15$. (b) Root-mean-square values of lift coefficient
$f^*=0.15$. (b) Root-mean-square values of lift coefficient ![]() $(C_L)_{rms}$ with the work of Wu et al. (Reference Wu, Wu, Tian, Zhao and Li2015) at
$(C_L)_{rms}$ with the work of Wu et al. (Reference Wu, Wu, Tian, Zhao and Li2015) at ![]() $Re=1100$. Parametric symbols follow the definitions given by Wu et al. (Reference Wu, Wu, Tian, Zhao and Li2015).
$Re=1100$. Parametric symbols follow the definitions given by Wu et al. (Reference Wu, Wu, Tian, Zhao and Li2015).
The present solver has also been used to compare with the experimental results of Heathcote & Gursul (Reference Heathcote and Gursul2007b), where jet-switching was studied in detail. Heathcote & Gursul (Reference Heathcote and Gursul2007b) considered a rigid teardrop foil with a flexible tail having length of two times the chord of the rigid foil, under quiescent flow conditions. The same configuration with the same level of flexibility has been used in the present validation simulations under quiescent flow conditions. Due to the limitations of the numerical schemes and algorithms used in the current IBM solver, the simulations were run at a lower ![]() $Re_f= f_s c^2/\nu =300$, whereas in the experiments, the value of
$Re_f= f_s c^2/\nu =300$, whereas in the experiments, the value of ![]() $Re_f$ was
$Re_f$ was ![]() $16\,200$. This comparison is presented to establish the capability of the FSI solver in capturing the jet-switching phenomenon. Figure 11 shows the qualitative comparison of the wake patterns. It is seen from figures 11(a,c) and figures 11(b,d) that the jet-switching phenomenon is captured properly under the quiescent flow. For quantitative comparison, the vortex core locations of the counter-clockwise and clockwise vortices are shown in figures 12(a) and 12(b) together with the results of Heathcote & Gursul (Reference Heathcote and Gursul2007b), and an overall agreement is observed in the time window considered. The temporal evolution of the flow field corresponding to figure 11 is presented in supplementary movie 5 available at https://doi.org/10.1017/jfm.2022.591.
$16\,200$. This comparison is presented to establish the capability of the FSI solver in capturing the jet-switching phenomenon. Figure 11 shows the qualitative comparison of the wake patterns. It is seen from figures 11(a,c) and figures 11(b,d) that the jet-switching phenomenon is captured properly under the quiescent flow. For quantitative comparison, the vortex core locations of the counter-clockwise and clockwise vortices are shown in figures 12(a) and 12(b) together with the results of Heathcote & Gursul (Reference Heathcote and Gursul2007b), and an overall agreement is observed in the time window considered. The temporal evolution of the flow field corresponding to figure 11 is presented in supplementary movie 5 available at https://doi.org/10.1017/jfm.2022.591.

Figure 11. Comparison of the velocity vector field for two representative flapping cycles, showing the jet-switching phenomenon under quiescent flow conditions: (a,c) experimental results reported by Heathcote & Gursul (Reference Heathcote and Gursul2007b), and (b,d) simulation results; ![]() $h=0.194$ and
$h=0.194$ and ![]() $b/c=1.13\times 10^{-3}$. Note that the parametric symbols follow the definitions given by Heathcote & Gursul (Reference Heathcote and Gursul2007b), equivalent to
$b/c=1.13\times 10^{-3}$. Note that the parametric symbols follow the definitions given by Heathcote & Gursul (Reference Heathcote and Gursul2007b), equivalent to ![]() $\gamma =0.496$. Permission for reproducing the figures from Heathcote & Gursul (Reference Heathcote and Gursul2007b) has been obtained from the publisher.
$\gamma =0.496$. Permission for reproducing the figures from Heathcote & Gursul (Reference Heathcote and Gursul2007b) has been obtained from the publisher.

Figure 12. Comparison of vortex core location in chord lengths obtained from the present simulation and the experimental work of Heathcote & Gursul (Reference Heathcote and Gursul2007b); ![]() $h=0.194$ and
$h=0.194$ and ![]() $b/c=1.13\times 10^{-3}$. Note that the parametric symbols follow the definitions given by Heathcote & Gursul (Reference Heathcote and Gursul2007b), equivalent to
$b/c=1.13\times 10^{-3}$. Note that the parametric symbols follow the definitions given by Heathcote & Gursul (Reference Heathcote and Gursul2007b), equivalent to ![]() $\gamma =0.496$: (a) counter-clockwise vortex, and (b) clockwise vortex.
$\gamma =0.496$: (a) counter-clockwise vortex, and (b) clockwise vortex.
3. Flow-field transition in the wake with a rigid aft-tail
The rigid system is considered as the base configuration in this study, and the associated flow field and nonlinear dynamical states are used as a benchmark to compare with the results of the flexible configurations. The rigid case results are presented in this section. In the next section, two different flexible tail configurations with ![]() $\gamma = 0.1$ and
$\gamma = 0.1$ and ![]() $0.01$ (referred to as flexibility levels I and II, respectively) are discussed. To follow the qualitative changes in the flow field for the case of a rigid aft-tail,
$0.01$ (referred to as flexibility levels I and II, respectively) are discussed. To follow the qualitative changes in the flow field for the case of a rigid aft-tail, ![]() $\kappa h$ is varied as the control parameter. The results are presented for three typically chosen parametric values to show the representative wake patterns of importance: three heave amplitudes,
$\kappa h$ is varied as the control parameter. The results are presented for three typically chosen parametric values to show the representative wake patterns of importance: three heave amplitudes, ![]() $h = 0.25,0.3,0.375$, have been considered at a fixed reduced frequency
$h = 0.25,0.3,0.375$, have been considered at a fixed reduced frequency ![]() $\kappa = 4.0$, resulting in
$\kappa = 4.0$, resulting in ![]() $\kappa h = 1.0,1.2,1.5$. The rigid aft-tail configuration represents the limiting case of bending rigidity,
$\kappa h = 1.0,1.2,1.5$. The rigid aft-tail configuration represents the limiting case of bending rigidity, ![]() $\gamma \to \infty$. The flow Reynolds number is chosen to be constant at
$\gamma \to \infty$. The flow Reynolds number is chosen to be constant at ![]() $Re = 300$. The other system parameters that remain constant for all the simulations are
$Re = 300$. The other system parameters that remain constant for all the simulations are ![]() $\beta = 1.0$,
$\beta = 1.0$, ![]() $Fr = 0.0$ and
$Fr = 0.0$ and ![]() $\boldsymbol {g}/{g} = (0,0)$. Table 1 summarises the parameter space considered in the present study. In addition, to understand the effect of the aft-tail length (
$\boldsymbol {g}/{g} = (0,0)$. Table 1 summarises the parameter space considered in the present study. In addition, to understand the effect of the aft-tail length (![]() $L_t$) in retaining the periodicity in wake and inhibiting jet-switching,
$L_t$) in retaining the periodicity in wake and inhibiting jet-switching, ![]() $L_t$ is changed by keeping the bending rigidity fixed at
$L_t$ is changed by keeping the bending rigidity fixed at ![]() $\gamma = 0.1$, and the results are presented for a typical value
$\gamma = 0.1$, and the results are presented for a typical value ![]() $L_t = 0.38c$ in this study. For the rigid aft-tail configuration, the flow field exhibits a mildly deflected reverse Kármán wake at
$L_t = 0.38c$ in this study. For the rigid aft-tail configuration, the flow field exhibits a mildly deflected reverse Kármán wake at ![]() $\kappa h=1.0$, associated with periodic dynamics. At
$\kappa h=1.0$, associated with periodic dynamics. At ![]() $\kappa h = 1.2$, mild jet-switching is observed in the wake, which turns into vigorous jet-switching involving much higher deflection angles at
$\kappa h = 1.2$, mild jet-switching is observed in the wake, which turns into vigorous jet-switching involving much higher deflection angles at ![]() $\kappa h = 1.5$.
$\kappa h = 1.5$.
Table 1. Parameter space.

The upward or downward deflection of the trailing wake is characterized based on the overall wake deflection angle ![]() $\varTheta$ (Godoy-Diana et al. Reference Godoy-Diana, Marais, Aider and Wesfreid2009; Majumdar et al. Reference Majumdar, Bose and Sarkar2020b). It is defined as the angle between the mean position of heaving motion (denoted by the dashed-dotted line) and the average wake deflection direction (denoted by the dashed line); see figure 13(a). Upward and downward deflected wakes are indicated by the positive and negative values of
$\varTheta$ (Godoy-Diana et al. Reference Godoy-Diana, Marais, Aider and Wesfreid2009; Majumdar et al. Reference Majumdar, Bose and Sarkar2020b). It is defined as the angle between the mean position of heaving motion (denoted by the dashed-dotted line) and the average wake deflection direction (denoted by the dashed line); see figure 13(a). Upward and downward deflected wakes are indicated by the positive and negative values of ![]() $\varTheta$, respectively. To understand the mechanism of jet-switching, a system of the first three vortices present in the wake at the end of a flapping cycle is considered following the works of Wei & Zheng (Reference Wei and Zheng2014) and Majumdar et al. (Reference Majumdar, Bose and Sarkar2020b); see figure 13(b). Here, vortices
$\varTheta$, respectively. To understand the mechanism of jet-switching, a system of the first three vortices present in the wake at the end of a flapping cycle is considered following the works of Wei & Zheng (Reference Wei and Zheng2014) and Majumdar et al. (Reference Majumdar, Bose and Sarkar2020b); see figure 13(b). Here, vortices ![]() $\boldsymbol {A}$,
$\boldsymbol {A}$, ![]() $\boldsymbol {B}$ and
$\boldsymbol {B}$ and ![]() $\boldsymbol {C}$ refer to the first counter-clockwise (CCW), first clockwise (CW) and second CCW vortex, respectively. As the flow evolves, if the distance between vortices
$\boldsymbol {C}$ refer to the first counter-clockwise (CCW), first clockwise (CW) and second CCW vortex, respectively. As the flow evolves, if the distance between vortices ![]() $\boldsymbol {A}$ and
$\boldsymbol {A}$ and ![]() $\boldsymbol {B}$ becomes less than that between
$\boldsymbol {B}$ becomes less than that between ![]() $\boldsymbol {B}$ and
$\boldsymbol {B}$ and ![]() $\boldsymbol {C}$, then vortex couple
$\boldsymbol {C}$, then vortex couple ![]() $\boldsymbol {A}\unicode{x2013}\boldsymbol {B}$ forms the dominant upward deflecting couple (Majumdar et al. Reference Majumdar, Bose and Sarkar2020b). It eventually deflects the entire wake in the upward direction in the subsequent cycles, which is the direction of its self-induced dipole velocity. The opposite scenario takes place during the formation of
$\boldsymbol {A}\unicode{x2013}\boldsymbol {B}$ forms the dominant upward deflecting couple (Majumdar et al. Reference Majumdar, Bose and Sarkar2020b). It eventually deflects the entire wake in the upward direction in the subsequent cycles, which is the direction of its self-induced dipole velocity. The opposite scenario takes place during the formation of ![]() $\boldsymbol {B}\unicode{x2013}\boldsymbol {C}$, the dominant downward deflecting couple, which induces the wake to deflect downwards. They will be referred to as ‘symmetry-breaking couples’ here. Eventually, the mutual competition between these two symmetry-breaking couples dictates the overall deflection direction of the wake (Godoy-Diana et al. Reference Godoy-Diana, Marais, Aider and Wesfreid2009; Zheng & Wei Reference Zheng and Wei2012). The dominance of either
$\boldsymbol {B}\unicode{x2013}\boldsymbol {C}$, the dominant downward deflecting couple, which induces the wake to deflect downwards. They will be referred to as ‘symmetry-breaking couples’ here. Eventually, the mutual competition between these two symmetry-breaking couples dictates the overall deflection direction of the wake (Godoy-Diana et al. Reference Godoy-Diana, Marais, Aider and Wesfreid2009; Zheng & Wei Reference Zheng and Wei2012). The dominance of either ![]() $\boldsymbol {A}\unicode{x2013}\boldsymbol {B}$ or
$\boldsymbol {A}\unicode{x2013}\boldsymbol {B}$ or ![]() $\boldsymbol {B}\unicode{x2013}\boldsymbol {C}$ is illustrated here using the ratio of their self-induced dipole velocities. It is defined as
$\boldsymbol {B}\unicode{x2013}\boldsymbol {C}$ is illustrated here using the ratio of their self-induced dipole velocities. It is defined as ![]() $U_{dipole}$-ratio
$U_{dipole}$-ratio ![]() $=U_{dipole, AB}/U_{dipole, BC}$, where
$=U_{dipole, AB}/U_{dipole, BC}$, where ![]() $U_{ dipole}$ is the self-induced dipole velocity evaluated as (Anderson Reference Anderson2010)
$U_{ dipole}$ is the self-induced dipole velocity evaluated as (Anderson Reference Anderson2010)
Here, ![]() $\xi$ denotes the distance between the two partners of a vortex couple, and
$\xi$ denotes the distance between the two partners of a vortex couple, and ![]() $\varGamma _{ avg}$ is the average of the absolute circulation values of the two partners. Also,
$\varGamma _{ avg}$ is the average of the absolute circulation values of the two partners. Also, ![]() $U_{ dipole}$-ratio can be evaluated as the ratio of two quantities,
$U_{ dipole}$-ratio can be evaluated as the ratio of two quantities, ![]() $\varGamma _{ avg}$-ratio (defined as
$\varGamma _{ avg}$-ratio (defined as ![]() $\varGamma _{ avg, AB} / \varGamma _{ avg, BC}$) and
$\varGamma _{ avg, AB} / \varGamma _{ avg, BC}$) and ![]() $\xi$-ratio (defined as
$\xi$-ratio (defined as ![]() $\xi _{ AB} / \xi _{ BC}$). Throughout this paper, terms ‘AB’ or ‘BC’ added in the suffix of any quantity/measure designate that particular quantity/measure to be associated with couple
$\xi _{ AB} / \xi _{ BC}$). Throughout this paper, terms ‘AB’ or ‘BC’ added in the suffix of any quantity/measure designate that particular quantity/measure to be associated with couple ![]() $\boldsymbol {A}\unicode{x2013}\boldsymbol {B}$ or
$\boldsymbol {A}\unicode{x2013}\boldsymbol {B}$ or ![]() $\boldsymbol {B}\unicode{x2013}\boldsymbol {C}$, respectively. For more discussion on the above-mentioned quantities, please refer to Godoy-Diana et al. (Reference Godoy-Diana, Marais, Aider and Wesfreid2009), Zheng & Wei (Reference Zheng and Wei2012), Wei & Zheng (Reference Wei and Zheng2014) and Majumdar et al. (Reference Majumdar, Bose and Sarkar2020b). The computational methodology for
$\boldsymbol {B}\unicode{x2013}\boldsymbol {C}$, respectively. For more discussion on the above-mentioned quantities, please refer to Godoy-Diana et al. (Reference Godoy-Diana, Marais, Aider and Wesfreid2009), Zheng & Wei (Reference Zheng and Wei2012), Wei & Zheng (Reference Wei and Zheng2014) and Majumdar et al. (Reference Majumdar, Bose and Sarkar2020b). The computational methodology for ![]() $\varGamma$ and
$\varGamma$ and ![]() $\xi$ is depicted in § 1 of the supplementary material for the sake of brevity. The qualitatively different wake behaviours are also associated with different nonlinear dynamical states, as will be established in the following subsections using a series of nonlinear time series analysis tools; the detailed description of these tools can be found in § 2 of the supplementary material and is also available in our earlier studies (Badrinath et al. Reference Badrinath, Bose and Sarkar2017; Bose & Sarkar Reference Bose and Sarkar2018).
$\xi$ is depicted in § 1 of the supplementary material for the sake of brevity. The qualitatively different wake behaviours are also associated with different nonlinear dynamical states, as will be established in the following subsections using a series of nonlinear time series analysis tools; the detailed description of these tools can be found in § 2 of the supplementary material and is also available in our earlier studies (Badrinath et al. Reference Badrinath, Bose and Sarkar2017; Bose & Sarkar Reference Bose and Sarkar2018).

Figure 13. (a) Definition of the deflection angle ![]() $(\varTheta )$. (b) Schematic representation of the system of the first three vortices in the trailing wake used in the present analysis.
$(\varTheta )$. (b) Schematic representation of the system of the first three vortices in the trailing wake used in the present analysis.
3.1.  $\kappa h=1.0$: reverse Kármán wake with mild deflection (periodic dynamics)
$\kappa h=1.0$: reverse Kármán wake with mild deflection (periodic dynamics)
At this ![]() $\kappa h$, the trailing wake exhibits a reverse Kármán vortex street with a small downward deflection with
$\kappa h$, the trailing wake exhibits a reverse Kármán vortex street with a small downward deflection with ![]() $\varTheta \approx -0.1^\circ$, as shown in figure 14 in terms of the instantaneous vorticity and velocity magnitude contours. Time variation of
$\varTheta \approx -0.1^\circ$, as shown in figure 14 in terms of the instantaneous vorticity and velocity magnitude contours. Time variation of ![]() $\varTheta$ is presented in figure 15(a). A mild dominance of downward deflecting couple
$\varTheta$ is presented in figure 15(a). A mild dominance of downward deflecting couple ![]() $\boldsymbol {B}\unicode{x2013}\boldsymbol {C}$, indicated by the
$\boldsymbol {B}\unicode{x2013}\boldsymbol {C}$, indicated by the ![]() $U_{ dipole}$-ratio less than unity (figure 15b), is able to direct the wake towards the downward direction. In figure 14, the vortex structures repeat in the consecutive flapping cycles due to the periodic nature of the flow field, resulting in a periodic
$U_{ dipole}$-ratio less than unity (figure 15b), is able to direct the wake towards the downward direction. In figure 14, the vortex structures repeat in the consecutive flapping cycles due to the periodic nature of the flow field, resulting in a periodic ![]() $C_D$ time history as shown in figure 16(a). The closed-loop behaviour of the phase portrait in the reconstructed phase space (figure 16b), the dominant frequency peak corresponding to twice the heaving frequency accompanied by super harmonics in the frequency spectra (figure 16c), and the wavelet spectra with a narrow frequency band (figure 16d), all confirm the periodic dynamics.
$C_D$ time history as shown in figure 16(a). The closed-loop behaviour of the phase portrait in the reconstructed phase space (figure 16b), the dominant frequency peak corresponding to twice the heaving frequency accompanied by super harmonics in the frequency spectra (figure 16c), and the wavelet spectra with a narrow frequency band (figure 16d), all confirm the periodic dynamics.

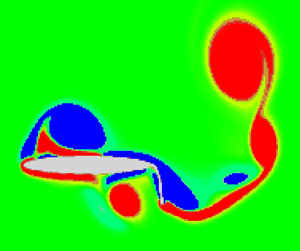
Figure 14. For ![]() $\kappa h=1.0$, rigid tail: instantaneous vorticity (top) and velocity magnitude (bottom) contours depicting a reverse Kármán wake with mild downward deflection. Note that the same contour levels have been used throughout the paper for all the vorticity and velocity contour plots, and are therefore not repeated hereafter.
$\kappa h=1.0$, rigid tail: instantaneous vorticity (top) and velocity magnitude (bottom) contours depicting a reverse Kármán wake with mild downward deflection. Note that the same contour levels have been used throughout the paper for all the vorticity and velocity contour plots, and are therefore not repeated hereafter.

Figure 15. For ![]() $\kappa h=1.0$, rigid tail: (a) wake deflection angle, (b) quantitative measures associated with the vortex system. Dominant effect of the downward deflecting vortex couple
$\kappa h=1.0$, rigid tail: (a) wake deflection angle, (b) quantitative measures associated with the vortex system. Dominant effect of the downward deflecting vortex couple ![]() $\boldsymbol {B}\unicode{x2013}\boldsymbol {C}$ results in negative deflecting angle.
$\boldsymbol {B}\unicode{x2013}\boldsymbol {C}$ results in negative deflecting angle.

Figure 16. For ![]() $\kappa h=1.0$, rigid tail, time series analysis of
$\kappa h=1.0$, rigid tail, time series analysis of ![]() $C_D$ indicates periodic dynamics: (a) time history, (b) reconstructed phase portrait, (c) frequency spectra (where PSD denotes power spectral density), and (d) wavelet transform.
$C_D$ indicates periodic dynamics: (a) time history, (b) reconstructed phase portrait, (c) frequency spectra (where PSD denotes power spectral density), and (d) wavelet transform.
Due to flow periodicity, the same vortex couple ![]() $\boldsymbol {B}\unicode{x2013}\boldsymbol {C}$ remains dominant throughout, leading to a downward deflected wake for all time; see figure 15(a). The minor variation in the
$\boldsymbol {B}\unicode{x2013}\boldsymbol {C}$ remains dominant throughout, leading to a downward deflected wake for all time; see figure 15(a). The minor variation in the ![]() $\varTheta$ time history can be attributed to the small changes observed in the
$\varTheta$ time history can be attributed to the small changes observed in the ![]() $\xi$-ratio, while the
$\xi$-ratio, while the ![]() $\varGamma _{ avg}$-ratio remains almost constant; see figure 15(b). These results are also quite similar to our earlier results for a rigid heaving system without any aft-tail (Majumdar et al. Reference Majumdar, Bose and Sarkar2020b).
$\varGamma _{ avg}$-ratio remains almost constant; see figure 15(b). These results are also quite similar to our earlier results for a rigid heaving system without any aft-tail (Majumdar et al. Reference Majumdar, Bose and Sarkar2020b).
3.2.  $\kappa h=1.2$: mild jet-switching (quasi-periodic dynamics)
$\kappa h=1.2$: mild jet-switching (quasi-periodic dynamics)
With an increase in ![]() $\kappa h$, the magnitude of the wake deflection angle increases, and a jet-switching behaviour is seen to take place around
$\kappa h$, the magnitude of the wake deflection angle increases, and a jet-switching behaviour is seen to take place around ![]() $\kappa h = 1.2$; see figure 17. Figure 18(a) shows that
$\kappa h = 1.2$; see figure 17. Figure 18(a) shows that ![]() $\varTheta$ changes its sign from positive to negative and vice versa, multiple times. The modulating oscillation in the
$\varTheta$ changes its sign from positive to negative and vice versa, multiple times. The modulating oscillation in the ![]() $C_D$ time history (figure 19a) represents the quasi-periodic behaviour of the flow field, which can be confirmed from the corresponding reconstructed phase space showing a toroidal portrait; see figure 19(b). The presence of quasi-periodicity can be proven further by the frequency spectra, which comprise two incommensurate frequencies,
$C_D$ time history (figure 19a) represents the quasi-periodic behaviour of the flow field, which can be confirmed from the corresponding reconstructed phase space showing a toroidal portrait; see figure 19(b). The presence of quasi-periodicity can be proven further by the frequency spectra, which comprise two incommensurate frequencies, ![]() $f_1=1.27$ and
$f_1=1.27$ and ![]() $f_2=0.61$, along with other non-harmonic peaks that are in a linear combination of these two; see figure 19(c). These incommensurate frequency bands are also visible in the wavelet spectra (figure 19d). The quasi-periodic wake interactions trigger jet-switching by inducing small changes in vortex strengths and vortex core locations in different cycles (Majumdar et al. Reference Majumdar, Bose and Sarkar2020b). As a result, the distances between the wake vortices change, leading to alternate dominance of upward and downward deflecting couples through the ‘vortex pairing’ process. The alternate dominance of the symmetry-breaking couples is depicted in terms of the variation in
$f_2=0.61$, along with other non-harmonic peaks that are in a linear combination of these two; see figure 19(c). These incommensurate frequency bands are also visible in the wavelet spectra (figure 19d). The quasi-periodic wake interactions trigger jet-switching by inducing small changes in vortex strengths and vortex core locations in different cycles (Majumdar et al. Reference Majumdar, Bose and Sarkar2020b). As a result, the distances between the wake vortices change, leading to alternate dominance of upward and downward deflecting couples through the ‘vortex pairing’ process. The alternate dominance of the symmetry-breaking couples is depicted in terms of the variation in ![]() $U_{ dipole}$-ratio in figure 18(b). The dominant symmetry-breaking couple, while convecting downstream, pulls the fluid behind it, forcing the mean jet to deflect in the direction of its self-induced velocity (Godoy-Diana et al. Reference Godoy-Diana, Marais, Aider and Wesfreid2009). The subsequent vortices follow the path dictated by the dominant couple, resulting in a deflected street. As the relative dominance shifts alternately between the upward deflecting
$U_{ dipole}$-ratio in figure 18(b). The dominant symmetry-breaking couple, while convecting downstream, pulls the fluid behind it, forcing the mean jet to deflect in the direction of its self-induced velocity (Godoy-Diana et al. Reference Godoy-Diana, Marais, Aider and Wesfreid2009). The subsequent vortices follow the path dictated by the dominant couple, resulting in a deflected street. As the relative dominance shifts alternately between the upward deflecting ![]() $\boldsymbol {A}\unicode{x2013}\boldsymbol {B}$ and downward deflecting
$\boldsymbol {A}\unicode{x2013}\boldsymbol {B}$ and downward deflecting ![]() $\boldsymbol {B}\unicode{x2013}\boldsymbol {C}$, they force the vortex street to switch the deflection direction alternately.
$\boldsymbol {B}\unicode{x2013}\boldsymbol {C}$, they force the vortex street to switch the deflection direction alternately.

Figure 17. For ![]() $\kappa h=1.2$, rigid tail: instantaneous vorticity (top) and velocity magnitude (bottom) contours showing mild jet-switching.
$\kappa h=1.2$, rigid tail: instantaneous vorticity (top) and velocity magnitude (bottom) contours showing mild jet-switching.

Figure 18. For ![]() $\kappa h=1.2$, rigid tail: (a) wake deflection angle, (b) quantitative measures associated with the vortex system. Alternate dominance of the upward and downward deflecting vortex couples leads to jet-switching.
$\kappa h=1.2$, rigid tail: (a) wake deflection angle, (b) quantitative measures associated with the vortex system. Alternate dominance of the upward and downward deflecting vortex couples leads to jet-switching.

Figure 19. For ![]() $\kappa h=1.2$, rigid tail, time series analysis of
$\kappa h=1.2$, rigid tail, time series analysis of ![]() $C_D$ indicates quasi-periodic dynamics: (a) time history, (b) reconstructed phase portrait, (c) frequency spectra, and (d) wavelet transform.
$C_D$ indicates quasi-periodic dynamics: (a) time history, (b) reconstructed phase portrait, (c) frequency spectra, and (d) wavelet transform.
3.3.  $\kappa h=1.5$: prominent jet-switching with higher deflection angles (dynamical state of intermittency)
$\kappa h=1.5$: prominent jet-switching with higher deflection angles (dynamical state of intermittency)
As ![]() $\kappa h$ is increased further to
$\kappa h$ is increased further to ![]() $1.5$, the jet-switching phenomenon becomes more prominent and the vortex street fluctuates with higher deflection angles compared to the previous case; see figure 20. The rapidity of switching also increases notably in this regime. The augmentation of jet-switching is triggered by strong intermittent perturbations from the primary LEV and its subsequent interactions with the TEV (as is discussed later in this subsection). As a result, the quasi-periodic pattern gets intermittently laden with irregular windows of chaotic bursts. In the nonlinear dynamics literature, such temporal patterns in which the system alternates irregularly between the states of quasi-periodicity and chaos are known as type-II intermittency (Hilborn et al. Reference Hilborn2000). The
$1.5$, the jet-switching phenomenon becomes more prominent and the vortex street fluctuates with higher deflection angles compared to the previous case; see figure 20. The rapidity of switching also increases notably in this regime. The augmentation of jet-switching is triggered by strong intermittent perturbations from the primary LEV and its subsequent interactions with the TEV (as is discussed later in this subsection). As a result, the quasi-periodic pattern gets intermittently laden with irregular windows of chaotic bursts. In the nonlinear dynamics literature, such temporal patterns in which the system alternates irregularly between the states of quasi-periodicity and chaos are known as type-II intermittency (Hilborn et al. Reference Hilborn2000). The ![]() $C_D$ time history in figure 21(a) shows two such typical chaotic windows (marked by rectangular boxes
$C_D$ time history in figure 21(a) shows two such typical chaotic windows (marked by rectangular boxes ![]() $B_1$ and
$B_1$ and ![]() $B_2$) in between the quasi-periodic modulations. Note that the reconstructed phase space (figure 21b) and the frequency spectra (figure 21c) are unable to capture the properties of these intermittent behaviours, and reflect an average quasi-periodic behaviour instead. However, the temporal evolution of the frequency content presented in the wavelet spectra (figure 21d) captures clearly the intermittency behaviour through the sporadic bursts of broadband frequencies. The recurrence plot in figure 22 also identifies the two different dynamical states (quasi-periodic and chaotic) present in the system. The quasi-periodic state is characterised by the unequally spaced lines parallel to the main diagonal, whereas the short and broken lines along with isolated points (marked by windows
$B_2$) in between the quasi-periodic modulations. Note that the reconstructed phase space (figure 21b) and the frequency spectra (figure 21c) are unable to capture the properties of these intermittent behaviours, and reflect an average quasi-periodic behaviour instead. However, the temporal evolution of the frequency content presented in the wavelet spectra (figure 21d) captures clearly the intermittency behaviour through the sporadic bursts of broadband frequencies. The recurrence plot in figure 22 also identifies the two different dynamical states (quasi-periodic and chaotic) present in the system. The quasi-periodic state is characterised by the unequally spaced lines parallel to the main diagonal, whereas the short and broken lines along with isolated points (marked by windows ![]() $B_1$ and
$B_1$ and ![]() $B_2$ in figure 22) are representative of the chaotic state.
$B_2$ in figure 22) are representative of the chaotic state.

Figure 20. Rigid tail configuration; variation in the wake deflection angle.

Figure 21. For ![]() $\kappa h=1.5$, rigid tail, time series analysis of
$\kappa h=1.5$, rigid tail, time series analysis of ![]() $C_D$ during type-II intermittency: (a) time history, (b) reconstructed phase portrait, (c) frequency spectra, and (d) wavelet transform.
$C_D$ during type-II intermittency: (a) time history, (b) reconstructed phase portrait, (c) frequency spectra, and (d) wavelet transform.

Figure 22. For ![]() $\kappa h=1.5$, rigid tail: recurrence plot of
$\kappa h=1.5$, rigid tail: recurrence plot of ![]() $C_D$ (depicting intermittency).
$C_D$ (depicting intermittency).
Recall that in the quasi-periodic regime, jet-switching started through the process of alternate dominance of upward and downward deflecting couples. The marginal shifts in the positions of the wake vortices was instrumental in flipping this dominance, and the LEVs had no direct contribution in jet-switching. However, during the state of intermittency, LEVs become important in the switching process through LEV–TEV interactions as the chaotic bursts provide strong perturbations in the near-field region. This results in the formation of strong symmetry-breaking couples at the trailing edge. A similar near-field behaviour was also observed by Bose et al. (Reference Bose, Gupta and Sarkar2021) for a no-tail configuration. For the present case, figure 23 shows the evolution of the primary LEVs (![]() $\boldsymbol {L1}\unicode{x2013}\boldsymbol {L16}$) tracked for eight consecutive flapping cycles during the chaotic window
$\boldsymbol {L1}\unicode{x2013}\boldsymbol {L16}$) tracked for eight consecutive flapping cycles during the chaotic window ![]() $B_1$. The snapshots are presented for the same phases of the cycles, and they are chosen during the ends of upstrokes and downstrokes. The primary LEVs are named differently for different flapping cycles in figure 23 as their strengths and locations differ from one cycle to another during the chaotic window. Towards the end of the upstrokes, aperiodic leading-edge separation is observed that leads to shedding of vortex couples of different strengths at different cycles, either from the leading edge (
$B_1$. The snapshots are presented for the same phases of the cycles, and they are chosen during the ends of upstrokes and downstrokes. The primary LEVs are named differently for different flapping cycles in figure 23 as their strengths and locations differ from one cycle to another during the chaotic window. Towards the end of the upstrokes, aperiodic leading-edge separation is observed that leads to shedding of vortex couples of different strengths at different cycles, either from the leading edge (![]() $\boldsymbol {C1}$) or from the mid-surface (
$\boldsymbol {C1}$) or from the mid-surface (![]() $\boldsymbol {C2}$). Shedding of leading-edge couples from the lower surface is hindered during a few cycles by the circumnavigation of another couple on the upper surface (e.g. see
$\boldsymbol {C2}$). Shedding of leading-edge couples from the lower surface is hindered during a few cycles by the circumnavigation of another couple on the upper surface (e.g. see ![]() $\boldsymbol {L5}$ at
$\boldsymbol {L5}$ at ![]() $t/T = 113.25$ in figure 23a). This aperiodic growth and convection of the LEVs affect their subsequent interactions with the TEVs considerably.
$t/T = 113.25$ in figure 23a). This aperiodic growth and convection of the LEVs affect their subsequent interactions with the TEVs considerably.

Figure 23. For ![]() $\kappa h=1.5$, rigid tail: LEV behaviour during the aperiodic window
$\kappa h=1.5$, rigid tail: LEV behaviour during the aperiodic window ![]() $B_1$ towards the end of (a) upstrokes and (b) downstrokes.
$B_1$ towards the end of (a) upstrokes and (b) downstrokes.
In order to understand the role of intermittency in enhancing jet-switching, the chronology of vortex interactions from five consecutive cycles (![]() $112{\rm th}$ to
$112{\rm th}$ to ![]() $116{\rm th}$) during chaotic window
$116{\rm th}$) during chaotic window ![]() $B_1$ is presented in figure 24. In the
$B_1$ is presented in figure 24. In the ![]() $112{\rm th}$ cycle, leading-edge couple
$112{\rm th}$ cycle, leading-edge couple ![]() $\boldsymbol {C1}$ interacts with the nascent TEVs,
$\boldsymbol {C1}$ interacts with the nascent TEVs, ![]() $\boldsymbol {T1}$ and
$\boldsymbol {T1}$ and ![]() $\boldsymbol {T2}$, which were produced during consecutive half-strokes. Consequently,
$\boldsymbol {T2}$, which were produced during consecutive half-strokes. Consequently, ![]() $\boldsymbol {T1}$ is not able to convect away but it stays close to
$\boldsymbol {T1}$ is not able to convect away but it stays close to ![]() $\boldsymbol {T2}$, which ensures a local dominance of downward deflecting couple
$\boldsymbol {T2}$, which ensures a local dominance of downward deflecting couple ![]() $\boldsymbol {T2}\unicode{x2013}\boldsymbol {T1}$ (similar to couple
$\boldsymbol {T2}\unicode{x2013}\boldsymbol {T1}$ (similar to couple ![]() $\boldsymbol {B}\unicode{x2013}\boldsymbol {C}$ of figure 13b). A similar set of events takes place during the
$\boldsymbol {B}\unicode{x2013}\boldsymbol {C}$ of figure 13b). A similar set of events takes place during the ![]() $115{\rm th}$ cycle as well, where
$115{\rm th}$ cycle as well, where ![]() $\boldsymbol {C2}$ influences the shedding of
$\boldsymbol {C2}$ influences the shedding of ![]() $\boldsymbol {T3}$ and
$\boldsymbol {T3}$ and ![]() $\boldsymbol {T4}$, resulting eventually in the dominance of downward deflecting
$\boldsymbol {T4}$, resulting eventually in the dominance of downward deflecting ![]() $\boldsymbol {T4}\unicode{x2013}\boldsymbol {T3}$ (at
$\boldsymbol {T4}\unicode{x2013}\boldsymbol {T3}$ (at ![]() $t/T = 116$ in figure 24t). Thus during the chaotic bursts in the intermittency regime, the primary LEV structures (say,
$t/T = 116$ in figure 24t). Thus during the chaotic bursts in the intermittency regime, the primary LEV structures (say, ![]() $\boldsymbol {C1}$ or
$\boldsymbol {C1}$ or ![]() $\boldsymbol {C2}$) influence directly the shedding and convection of the TEVs (say,
$\boldsymbol {C2}$) influence directly the shedding and convection of the TEVs (say, ![]() $\boldsymbol {T1}$ and
$\boldsymbol {T1}$ and ![]() $\boldsymbol {T2}$, or
$\boldsymbol {T2}$, or ![]() $\boldsymbol {T3}$ and
$\boldsymbol {T3}$ and ![]() $\boldsymbol {T4}$) through LEV–TEV interactions. This provides an additional impetus towards the formation of the symmetry-breaking couples, resulting in higher deflection angles and enhancement of the jet-switching process. Our previous studies (Majumdar et al. Reference Majumdar, Bose and Sarkar2020b; Bose et al. Reference Bose, Gupta and Sarkar2021) and the present findings confirm that the LEV shedding and its subsequent interactions with the TEV are instrumental in the formation of the symmetry-breaking couples at the trailing edge, leading to vigorous jet-switching.
$\boldsymbol {T4}$) through LEV–TEV interactions. This provides an additional impetus towards the formation of the symmetry-breaking couples, resulting in higher deflection angles and enhancement of the jet-switching process. Our previous studies (Majumdar et al. Reference Majumdar, Bose and Sarkar2020b; Bose et al. Reference Bose, Gupta and Sarkar2021) and the present findings confirm that the LEV shedding and its subsequent interactions with the TEV are instrumental in the formation of the symmetry-breaking couples at the trailing edge, leading to vigorous jet-switching.

Figure 24. For ![]() $\kappa h=1.5$, rigid tail: instantaneous vorticity contours during
$\kappa h=1.5$, rigid tail: instantaneous vorticity contours during ![]() $112{\rm th}$ to
$112{\rm th}$ to ![]() $116{\rm th}$ cycles.
$116{\rm th}$ cycles.
Note that the deflection angles reported in the present study, during the prominent jet-switching at ![]() $\kappa h = 1.5$, are smaller comparatively (
$\kappa h = 1.5$, are smaller comparatively (![]() $<15^{\circ }$) than that reported in the experimental results of Heathcote & Gursul (Reference Heathcote and Gursul2007b). This can be attributed possibly to the lower
$<15^{\circ }$) than that reported in the experimental results of Heathcote & Gursul (Reference Heathcote and Gursul2007b). This can be attributed possibly to the lower ![]() $Re$ regime considered here, which is at least two orders of magnitude lower than that of the above-mentioned experimental study. Liang et al. (Reference Liang, Ou, Premasuthan, Jameson and Wang2011) and Zheng & Wei (Reference Zheng and Wei2012) have shown that the wake deflection angle varies considerably with
$Re$ regime considered here, which is at least two orders of magnitude lower than that of the above-mentioned experimental study. Liang et al. (Reference Liang, Ou, Premasuthan, Jameson and Wang2011) and Zheng & Wei (Reference Zheng and Wei2012) have shown that the wake deflection angle varies considerably with ![]() $Re$, and a decrease in
$Re$, and a decrease in ![]() $Re$ results in a decrease in deflection angle as well. Besides, in the experiments with rigid and flexible teardrop foils under quiescent flow, Heathcote & Gursul (Reference Heathcote and Gursul2007b) observed the deflection angle to be higher than uniform inflow cases. Thus use of uniform free stream in the present simulations could also be another possible reason for encountering smaller angles compared to Heathcote & Gursul (Reference Heathcote and Gursul2007b).
$Re$ results in a decrease in deflection angle as well. Besides, in the experiments with rigid and flexible teardrop foils under quiescent flow, Heathcote & Gursul (Reference Heathcote and Gursul2007b) observed the deflection angle to be higher than uniform inflow cases. Thus use of uniform free stream in the present simulations could also be another possible reason for encountering smaller angles compared to Heathcote & Gursul (Reference Heathcote and Gursul2007b).
4. Effect of tail flexibility on the transitional dynamics
The flexible tail undergoes coupled fluid–structure interactions with the surrounding flow field, altering the features of LEV separation, TEV shedding, and LEV–TEV interactions. The flow-field behaviour triggering quasi-periodicity and intermittency gets changed, which affects eventually the formation of the dominant symmetry-breaking couples. These changes in the near field interfere with the jet-switching phenomenon and re-establish the symmetry in the wake. The underlying flow dynamics is examined in this section for ![]() $\kappa h=1.5$ for two different flexibility levels of the aft-tail, referred to as flexibility levels I and II here. Recall that at this
$\kappa h=1.5$ for two different flexibility levels of the aft-tail, referred to as flexibility levels I and II here. Recall that at this ![]() $\kappa h$, a vigorous jet-switching associated with a dynamical state of intermittency was observed for the rigid tail configuration. It is observed here that the flexible deformation of the tail inhibits jet-switching for both flexibility levels I and II, which leads to quasi-periodic and periodic wake dynamics, respectively. This is in contrast to the state of intermittency observed for the rigid tail case. The underlying flow dynamics responsible for suppressing jet-switching and establishing symmetry in the wake are addressed in the following. To the best of the authors’ knowledge, the role of flexibility behind the suppression of jet-switching with interlinked dynamical characteristics is being discussed for the first time in the present study.
$\kappa h$, a vigorous jet-switching associated with a dynamical state of intermittency was observed for the rigid tail configuration. It is observed here that the flexible deformation of the tail inhibits jet-switching for both flexibility levels I and II, which leads to quasi-periodic and periodic wake dynamics, respectively. This is in contrast to the state of intermittency observed for the rigid tail case. The underlying flow dynamics responsible for suppressing jet-switching and establishing symmetry in the wake are addressed in the following. To the best of the authors’ knowledge, the role of flexibility behind the suppression of jet-switching with interlinked dynamical characteristics is being discussed for the first time in the present study.
4.1. Flexibility level I: deflected wake pattern associated with quasi-periodic dynamics
In contrast to the rigid tail configuration, the tail with flexibility level I (![]() $\gamma =0.1$) exhibits a downward deflected reverse Kármán street without any switching (figure 25a). In this case, the
$\gamma =0.1$) exhibits a downward deflected reverse Kármán street without any switching (figure 25a). In this case, the ![]() $C_D$ time history reflects distinct quasi-periodic dynamics without any trace of intermittency (figure 26a). The corresponding reconstructed phase space depicts a well-developed toroidal portrait without any irregularities, which confirms the state to be pure quasi-periodic (figure 26b). The corresponding frequency spectrum is comprised of two incommensurate frequencies (
$C_D$ time history reflects distinct quasi-periodic dynamics without any trace of intermittency (figure 26a). The corresponding reconstructed phase space depicts a well-developed toroidal portrait without any irregularities, which confirms the state to be pure quasi-periodic (figure 26b). The corresponding frequency spectrum is comprised of two incommensurate frequencies (![]() $f_1=1.27$ and
$f_1=1.27$ and ![]() $f_2=0.87$), with other peaks being their linear combinations, which is again indicative of quasi-periodicity (figure 26c). The existence of these two incommensurate frequencies in the form of two narrow bands is also evident from the scalogram of the
$f_2=0.87$), with other peaks being their linear combinations, which is again indicative of quasi-periodicity (figure 26c). The existence of these two incommensurate frequencies in the form of two narrow bands is also evident from the scalogram of the ![]() $C_D$ time history in figure 26(d). Quasi-periodicity is also visible from the recurrence plot presented in figure 27, showing unequally spaced lines parallel to the main diagonal. To establish the presence of quasi-periodicity through a direct flow-field quantity, correlation coefficients
$C_D$ time history in figure 26(d). Quasi-periodicity is also visible from the recurrence plot presented in figure 27, showing unequally spaced lines parallel to the main diagonal. To establish the presence of quasi-periodicity through a direct flow-field quantity, correlation coefficients ![]() $\rho (t)$ of the vorticity field are estimated. For details of the formulation of
$\rho (t)$ of the vorticity field are estimated. For details of the formulation of ![]() $\rho (t)$, please refer to our earlier study, Badrinath et al. (Reference Badrinath, Bose and Sarkar2017). The variation of the maximum
$\rho (t)$, please refer to our earlier study, Badrinath et al. (Reference Badrinath, Bose and Sarkar2017). The variation of the maximum ![]() $\rho (t)$ between
$\rho (t)$ between ![]() $0.5$ to
$0.5$ to ![]() $1$ confirms the fact that the flow structures do not exactly repeat, nor are they completely different in consecutive cycles (figure 25b), which points to a quasi-periodic scenario. The details of the flow-field behaviour responsible for this change in the dynamics, in comparison to the rigid case, will be discussed in §§ 4.3 and 4.4.
$1$ confirms the fact that the flow structures do not exactly repeat, nor are they completely different in consecutive cycles (figure 25b), which points to a quasi-periodic scenario. The details of the flow-field behaviour responsible for this change in the dynamics, in comparison to the rigid case, will be discussed in §§ 4.3 and 4.4.

Figure 25. For ![]() $\kappa h=1.5$, flexibility level I: (a) downward deflected vortex street, and (b) vorticity correlation.
$\kappa h=1.5$, flexibility level I: (a) downward deflected vortex street, and (b) vorticity correlation.

Figure 26. For ![]() $\kappa h=1.5$, flexibility level I, time series analysis of
$\kappa h=1.5$, flexibility level I, time series analysis of ![]() $C_D$ indicates quasi-periodic dynamics: (a) time history, (b) reconstructed phase portrait, (c) frequency spectra, and (d) wavelet transform.
$C_D$ indicates quasi-periodic dynamics: (a) time history, (b) reconstructed phase portrait, (c) frequency spectra, and (d) wavelet transform.

Figure 27. For ![]() $\kappa h=1.5$, flexibility level I: recurrence plot of
$\kappa h=1.5$, flexibility level I: recurrence plot of ![]() $C_D$.
$C_D$.
4.2. Flexibility level II: symmetric reverse Kármán wake with periodic dynamics
As the flexibility increases to level II, the trailing wake shows a reverse Kármán street without any switching or even any strong deflection (figure 28a). Contrary to both the cases of rigid and flexibility level I, the flow-field aperiodicity disappears in level II, and the wake vortices repeat almost exactly in consecutive cycles. Detailed flow-field comparisons are presented in §§ 4.3 and 4.4. The corresponding ![]() $C_D$ time history has no modulations in its amplitude (figure 29a), and instead of a toroidal shape, two distinct closed loops in the reconstructed phase space are seen. The latter represent a period-2 signature; see figure 29(b). The frequency spectra (figure 29c) and the wavelet spectra (figure 29d) highlight a dominant frequency peak along with the subharmonics and superharmonics only. The uniformly spaced continuous lines parallel to the main diagonal in the recurrence plot as shown in figure 30 are characteristic of a periodic signal too. Also, the maximum correlation coefficient (
$C_D$ time history has no modulations in its amplitude (figure 29a), and instead of a toroidal shape, two distinct closed loops in the reconstructed phase space are seen. The latter represent a period-2 signature; see figure 29(b). The frequency spectra (figure 29c) and the wavelet spectra (figure 29d) highlight a dominant frequency peak along with the subharmonics and superharmonics only. The uniformly spaced continuous lines parallel to the main diagonal in the recurrence plot as shown in figure 30 are characteristic of a periodic signal too. Also, the maximum correlation coefficient (![]() $\rho$) of the vorticity field remains unity, which establishes the periodicity of the flow field (figure 28b).
$\rho$) of the vorticity field remains unity, which establishes the periodicity of the flow field (figure 28b).

Figure 28. For ![]() $\kappa h=1.5$, flexibility level II: (a) mildly deflected wake, and (b) vorticity correlation.
$\kappa h=1.5$, flexibility level II: (a) mildly deflected wake, and (b) vorticity correlation.

Figure 29. For ![]() $\kappa h=1.5$, flexibility level II, time series analysis of
$\kappa h=1.5$, flexibility level II, time series analysis of ![]() $C_D$ indicates periodic dynamics: (a) time history, (b) reconstructed phase portrait, (c) frequency spectra, and (d) wavelet transform.
$C_D$ indicates periodic dynamics: (a) time history, (b) reconstructed phase portrait, (c) frequency spectra, and (d) wavelet transform.

Figure 30. For ![]() $\kappa h=1.5$, flexibility level II: recurrence plot of
$\kappa h=1.5$, flexibility level II: recurrence plot of ![]() $C_D$.
$C_D$.
4.3. Difference in the wake deflection characteristics between the rigid and flexible configurations
It is evident that the flow topology as well as the dynamical states undergoes a significant change as flexibility is introduced in the system, and as the flexibility increases, the trailing wake becomes more organised. The vigorous jet-switching at ![]() $\kappa h = 1.5$ seen for the rigid configuration is suppressed completely, and the trailing wake returns to a periodic reverse Kármán street at the higher flexibility level.
$\kappa h = 1.5$ seen for the rigid configuration is suppressed completely, and the trailing wake returns to a periodic reverse Kármán street at the higher flexibility level.
The instantaneous vorticity and velocity magnitude contours depicting the deflection angles of the jet at a few chosen cycles corresponding to the rigid and flexible tail configurations are compared in figure 31. The continuous temporal evolution of the flow fields for the respective cases are presented in supplementary movies 1, 2 and 3. Here, vigorous jet-switching with high deflection angles is observed for the rigid case. A downward deflected wake with ![]() $\varTheta =-2.6$ is seen at
$\varTheta =-2.6$ is seen at ![]() $t/T=104.0$, the deflection direction changes with time and the shed vortices start to move upwards, giving an upward deflected wake with
$t/T=104.0$, the deflection direction changes with time and the shed vortices start to move upwards, giving an upward deflected wake with ![]() $\varTheta =4.2$ at
$\varTheta =4.2$ at ![]() $t/T=110.0$ (figures 31a–d). Such switchings between downward and upward directions continue to take place for the rigid case (see at
$t/T=110.0$ (figures 31a–d). Such switchings between downward and upward directions continue to take place for the rigid case (see at ![]() $t/T=119.0$ with
$t/T=119.0$ with ![]() $\varTheta =-6.6$, and at
$\varTheta =-6.6$, and at ![]() $t/T=126.0$ with
$t/T=126.0$ with ![]() $\varTheta =3.4$) . However, this gets inhibited with the introduction of flexibility, and at flexibility level I, the wake deflection direction no longer switches. It remains downward deflected for all time, with small time-dependent changes in its deflection angle (see figures 31e–h). With flexibility increasing to level II, the wake gets more regularised. It reorganises into a periodic reverse Kármán pattern with a very small deflection angle (figures 31i–l). The jet-switching and its inhibition at different flexibility levels reflect directly on the aerodynamic loads as well. This has been presented in terms of period-average lift coefficient in § 3 of the supplementary material.
$\varTheta =3.4$) . However, this gets inhibited with the introduction of flexibility, and at flexibility level I, the wake deflection direction no longer switches. It remains downward deflected for all time, with small time-dependent changes in its deflection angle (see figures 31e–h). With flexibility increasing to level II, the wake gets more regularised. It reorganises into a periodic reverse Kármán pattern with a very small deflection angle (figures 31i–l). The jet-switching and its inhibition at different flexibility levels reflect directly on the aerodynamic loads as well. This has been presented in terms of period-average lift coefficient in § 3 of the supplementary material.

Figure 31. For ![]() $\kappa h=1.5$, comparison of instantaneous vorticity fields and corresponding velocity magnitude contours for (a–d) rigid tail, (e–h) flexibility level I, and (i–l) flexibility level II.
$\kappa h=1.5$, comparison of instantaneous vorticity fields and corresponding velocity magnitude contours for (a–d) rigid tail, (e–h) flexibility level I, and (i–l) flexibility level II.
The flip in the relative dominance of the upward and downward deflecting couples is responsible for the manifestation of jet-switching, and the ![]() $\xi$-ratio is the primary factor dictating the dominance of the symmetry-breaking couples (Majumdar et al. Reference Majumdar, Bose and Sarkar2020b). Three representative cycles, at
$\xi$-ratio is the primary factor dictating the dominance of the symmetry-breaking couples (Majumdar et al. Reference Majumdar, Bose and Sarkar2020b). Three representative cycles, at ![]() $t/T=108, 116,126$, are chosen to compare the
$t/T=108, 116,126$, are chosen to compare the ![]() $\xi$-ratios for rigid and flexible configurations in figure 32. The complete time evolution of the
$\xi$-ratios for rigid and flexible configurations in figure 32. The complete time evolution of the ![]() $\xi$-ratio is presented later, in figure 33(c). For the rigid tail, the
$\xi$-ratio is presented later, in figure 33(c). For the rigid tail, the ![]() $\xi$-ratio of
$\xi$-ratio of ![]() $\boldsymbol {A}\unicode{x2013}\boldsymbol {B}$ to
$\boldsymbol {A}\unicode{x2013}\boldsymbol {B}$ to ![]() $\boldsymbol {B}\unicode{x2013}\boldsymbol {C}$ is seen to be less than unity (
$\boldsymbol {B}\unicode{x2013}\boldsymbol {C}$ is seen to be less than unity (![]() $\xi _{AB}/\xi _{BC}=0.82$) at
$\xi _{AB}/\xi _{BC}=0.82$) at ![]() $t/T = 108$. Hence
$t/T = 108$. Hence ![]() $\boldsymbol {A}\unicode{x2013}\boldsymbol {B}$ forms the dominant couple, and this induces an upward jet that maintains the upward deflected wake subsequently (figures 32a,d,g). However, at
$\boldsymbol {A}\unicode{x2013}\boldsymbol {B}$ forms the dominant couple, and this induces an upward jet that maintains the upward deflected wake subsequently (figures 32a,d,g). However, at ![]() $t/T=116.0$, the
$t/T=116.0$, the ![]() $\xi$-ratio becomes
$\xi$-ratio becomes ![]() $1.24$, and the dominance is transferred to
$1.24$, and the dominance is transferred to ![]() $\boldsymbol {B}\unicode{x2013}\boldsymbol {C}$, which induces a downward jet causing the subsequently shed vortices to follow a downward deflected path (see at
$\boldsymbol {B}\unicode{x2013}\boldsymbol {C}$, which induces a downward jet causing the subsequently shed vortices to follow a downward deflected path (see at ![]() $t/T=116.0$ in figures 32a,d,g). At
$t/T=116.0$ in figures 32a,d,g). At ![]() $t/T=126.0$, the
$t/T=126.0$, the ![]() $\xi$-ratio again becomes less than 1, indicating the dominance of upward deflecting
$\xi$-ratio again becomes less than 1, indicating the dominance of upward deflecting ![]() $\boldsymbol {A}\unicode{x2013}\boldsymbol {B}$ that gives way to another switching. The same was confirmed from the velocity magnitude contour plots in figures 31(a–d) as well.
$\boldsymbol {A}\unicode{x2013}\boldsymbol {B}$ that gives way to another switching. The same was confirmed from the velocity magnitude contour plots in figures 31(a–d) as well.

Figure 32. For ![]() $\kappa h=1.5$, comparison of trailing-wake patterns showing the
$\kappa h=1.5$, comparison of trailing-wake patterns showing the ![]() $\xi -$ratio for (a,d,g) rigid tail, (b,e,h) flexibility level I, and (c, f,i) flexibility level II.
$\xi -$ratio for (a,d,g) rigid tail, (b,e,h) flexibility level I, and (c, f,i) flexibility level II.

Figure 33. For ![]() $\kappa h=1.5$, variation of: (a) deflection angle, (b) dipole velocity ratio, (c) distance ratio, and (d) average circulation ratio, for different aft-tail configurations.
$\kappa h=1.5$, variation of: (a) deflection angle, (b) dipole velocity ratio, (c) distance ratio, and (d) average circulation ratio, for different aft-tail configurations.
The corresponding ![]() $\xi$-ratio for flexibility level I remains more than unity at all times with a varying magnitude, causing the wake to remain downward deflected, with the angle of deflection varying with time (figures 32b,e,h). Since the
$\xi$-ratio for flexibility level I remains more than unity at all times with a varying magnitude, causing the wake to remain downward deflected, with the angle of deflection varying with time (figures 32b,e,h). Since the ![]() $\xi$-ratio remains always greater than 1,
$\xi$-ratio remains always greater than 1, ![]() $\boldsymbol {B}\unicode{x2013}\boldsymbol {C}$ is able to maintain its continuous dominance to pull the wake downwards at every cycle; see figures 32(b,e,h). As an outcome, the phenomenon of jet-switching gets suppressed. At flexibility level II, the
$\boldsymbol {B}\unicode{x2013}\boldsymbol {C}$ is able to maintain its continuous dominance to pull the wake downwards at every cycle; see figures 32(b,e,h). As an outcome, the phenomenon of jet-switching gets suppressed. At flexibility level II, the ![]() $\xi$-ratio remains almost constant and quite close to unity (
$\xi$-ratio remains almost constant and quite close to unity (![]() $\approx 0.9$), facilitating the upward deflecting couple
$\approx 0.9$), facilitating the upward deflecting couple ![]() $\boldsymbol {A}\unicode{x2013}\boldsymbol {B}$ to maintain a small dominance forever. This results in an almost symmetric (with a negligible upward deflection) stable reverse Kármán street, as shown in figures 32(c, f,i).
$\boldsymbol {A}\unicode{x2013}\boldsymbol {B}$ to maintain a small dominance forever. This results in an almost symmetric (with a negligible upward deflection) stable reverse Kármán street, as shown in figures 32(c, f,i).
The results can also be visualised quantitatively through a comparison of the time histories of different measures, as shown in figure 33. For the rigid tail, ![]() $\varTheta$ crosses the zero mark multiple times, indicating multiple switchings; see figure 33(a). For flexibility level I,
$\varTheta$ crosses the zero mark multiple times, indicating multiple switchings; see figure 33(a). For flexibility level I, ![]() $\varTheta$ varies with time but never changes its sign; hence jet-switching is absent and the wake stays downward deflected for all time. For flexibility level II,
$\varTheta$ varies with time but never changes its sign; hence jet-switching is absent and the wake stays downward deflected for all time. For flexibility level II, ![]() $\varTheta$ remains nearly constant with time with a very small positive value close to zero, representative of a vortex street having a mild upward deflection. The temporal evolution of
$\varTheta$ remains nearly constant with time with a very small positive value close to zero, representative of a vortex street having a mild upward deflection. The temporal evolution of ![]() $U_{{dipole}}$-ratio,
$U_{{dipole}}$-ratio, ![]() $\xi$-ratio and
$\xi$-ratio and ![]() $\varGamma _{{avg}}$-ratio are shown in figures 33(b), 33(c) and 33(d), respectively. The
$\varGamma _{{avg}}$-ratio are shown in figures 33(b), 33(c) and 33(d), respectively. The ![]() $\xi$-ratio, and therefore the
$\xi$-ratio, and therefore the ![]() $U_{{dipole}}$-ratio, undergoes significant modulations around unity for the rigid case, leading to frequent shifts in the dominance of the symmetry-breaking couples that get manifested as vigorous switching. Note that the variation of the
$U_{{dipole}}$-ratio, undergoes significant modulations around unity for the rigid case, leading to frequent shifts in the dominance of the symmetry-breaking couples that get manifested as vigorous switching. Note that the variation of the ![]() $\varGamma _{{avg}}$-ratio is relatively lesser, signifying its unimportant role in jet-switching. For flexibility level I, the fluctuations in
$\varGamma _{{avg}}$-ratio is relatively lesser, signifying its unimportant role in jet-switching. For flexibility level I, the fluctuations in ![]() $\xi$-ratio and
$\xi$-ratio and ![]() $U_{{dipole}}$-ratio are seen to stay always above and below unity, respectively, as no change takes place in the deflection direction. With the increase in flexibility to level II, the fluctuations in these measures come down significantly as the wake gets reorganised into an almost symmetric reverse Kármán pattern.
$U_{{dipole}}$-ratio are seen to stay always above and below unity, respectively, as no change takes place in the deflection direction. With the increase in flexibility to level II, the fluctuations in these measures come down significantly as the wake gets reorganised into an almost symmetric reverse Kármán pattern.
4.4. Underlying vortex interaction mechanisms
From the above results, flexibility emerges as a crucial system parameter that can be manipulated to alter the dynamics as well as suppress the phenomenon of jet-switching. The near-field vortex interactions that lead to this suppression are examined in this subsection. It is important to understand the mechanism of change in the dominance and formation of the symmetry-breaking couples under the flexible tail configurations. In this regard, the chronology of vortex interactions during a typical flapping cycle is presented for flexibility levels I and II in figure 34. For flexibility level I, LEV ![]() $\boldsymbol {L1}^*$ initially sheds as a couple having a strong CCW and a fragile CW component at the start of the upstroke (see at
$\boldsymbol {L1}^*$ initially sheds as a couple having a strong CCW and a fragile CW component at the start of the upstroke (see at ![]() $t/T=110.125$ in figure 34a). Subsequently, the weaker CW component gets convected away, and the stronger CCW counterpart reattaches on the lower surface around the mid-chord, and gives rise to couple
$t/T=110.125$ in figure 34a). Subsequently, the weaker CW component gets convected away, and the stronger CCW counterpart reattaches on the lower surface around the mid-chord, and gives rise to couple ![]() $\boldsymbol {C1}^*$ (see at
$\boldsymbol {C1}^*$ (see at ![]() $t/T=110.375$ in figure 34a). In the next half-cycle,
$t/T=110.375$ in figure 34a). In the next half-cycle, ![]() $\boldsymbol {C1}^*$ is shed from the lower surface and interacts with the CW TEV
$\boldsymbol {C1}^*$ is shed from the lower surface and interacts with the CW TEV ![]() $\boldsymbol {T1}^*$, forming eventually a dominant downward deflected symmetry-breaking couple. The CW LEV
$\boldsymbol {T1}^*$, forming eventually a dominant downward deflected symmetry-breaking couple. The CW LEV ![]() $\boldsymbol {L2}^*$ does not shed from the foil (see
$\boldsymbol {L2}^*$ does not shed from the foil (see ![]() $t/T=110.625$ to
$t/T=110.625$ to ![]() $110.75$ in figure 34b), but remains attached to the upper surface near the leading-edge and gets flattened gradually over the body (
$110.75$ in figure 34b), but remains attached to the upper surface near the leading-edge and gets flattened gradually over the body (![]() $t/T=110.875$ to
$t/T=110.875$ to ![]() $111.0$ in figure 34b). Note that
$111.0$ in figure 34b). Note that ![]() $\boldsymbol {L2}^*$ does not participate in any interactions with the TEV. Therefore, the LEV–TEV interaction takes place asymmetrically and only at the lower surface of the foil. The asymmetric LEV–TEV interactions prevent a full reversal of the deflection direction, as only a downward deflected symmetry-breaking couple forms at every cycle. As the associated dynamical state is quasi-periodic, the strength of this couple varies from one cycle to another, resulting in a time-varying
$\boldsymbol {L2}^*$ does not participate in any interactions with the TEV. Therefore, the LEV–TEV interaction takes place asymmetrically and only at the lower surface of the foil. The asymmetric LEV–TEV interactions prevent a full reversal of the deflection direction, as only a downward deflected symmetry-breaking couple forms at every cycle. As the associated dynamical state is quasi-periodic, the strength of this couple varies from one cycle to another, resulting in a time-varying ![]() $\varTheta$ behaviour (figure 33a).
$\varTheta$ behaviour (figure 33a).

Figure 34. For ![]() $\kappa h = 1.5$, comparison of near-field vortex interactions at the
$\kappa h = 1.5$, comparison of near-field vortex interactions at the ![]() $111{\rm th}$ cycle: (a) first half-cycle, (b) second half-cycle; each left-hand column shows flexibility level I, and each right-hand column shows flexibility level II.
$111{\rm th}$ cycle: (a) first half-cycle, (b) second half-cycle; each left-hand column shows flexibility level I, and each right-hand column shows flexibility level II.
As the flexibility increases to level II, the flow becomes more regularised, and the vortex interactions become symmetric between the upper and lower surfaces. Unlike level I, in this case an isolated CCW LEV, ![]() $\boldsymbol {L1}^**$, is formed, which stays close to the lower surface of the foil. However, similar to flexibility level I,
$\boldsymbol {L1}^**$, is formed, which stays close to the lower surface of the foil. However, similar to flexibility level I, ![]() $\boldsymbol {L1}^**$ forms couple
$\boldsymbol {L1}^**$ forms couple ![]() $\boldsymbol {C1}^**$ with a shear layer vortex while convecting towards the trailing edge. The strength of
$\boldsymbol {C1}^**$ with a shear layer vortex while convecting towards the trailing edge. The strength of ![]() $\boldsymbol {C1}^**$ is considerably lower than that of
$\boldsymbol {C1}^**$ is considerably lower than that of ![]() $\boldsymbol {C1}^*$ in the level I case, and it remains very close to the surface while moving downstream. Subsequently,
$\boldsymbol {C1}^*$ in the level I case, and it remains very close to the surface while moving downstream. Subsequently, ![]() $\boldsymbol {C1}^**$ interacts with the highly deformed tail structure and gets trapped near it, preventing the couple from interacting directly with the TEV
$\boldsymbol {C1}^**$ interacts with the highly deformed tail structure and gets trapped near it, preventing the couple from interacting directly with the TEV ![]() $\boldsymbol {T2}^**$. Similar events occur on the upper surface in the following half-cycle as well, where LEV
$\boldsymbol {T2}^**$. Similar events occur on the upper surface in the following half-cycle as well, where LEV ![]() $\boldsymbol {L2}^**$ forms couple
$\boldsymbol {L2}^**$ forms couple ![]() $\boldsymbol {C2}^**$. Similar to
$\boldsymbol {C2}^**$. Similar to ![]() $\boldsymbol {C1}^**$,
$\boldsymbol {C1}^**$, ![]() $\boldsymbol {C2}^**$ also does not get a chance to interact with the TEV due to its interaction with the highly deformed tail. The large bending of the flexible aft-tail inhibits the LEVs from interacting with the shed TEVs directly, thus preventing the formation of the symmetry-breaking couples that are the main agencies behind jet-switching. As a result, the near-wake vortices get well organised and finally settle into a near symmetric reverse Kármán wake through the merging of the same-signed vortices.
$\boldsymbol {C2}^**$ also does not get a chance to interact with the TEV due to its interaction with the highly deformed tail. The large bending of the flexible aft-tail inhibits the LEVs from interacting with the shed TEVs directly, thus preventing the formation of the symmetry-breaking couples that are the main agencies behind jet-switching. As a result, the near-wake vortices get well organised and finally settle into a near symmetric reverse Kármán wake through the merging of the same-signed vortices.
4.5. Flexible deformation of the aft-tail leading to suppression of jet-switching
Deformation of the flexible tail alters the interactions of the near-field vortices that decides the fate of the overall far-wake pattern. The deflection envelope and the phase plot of the tail-tip are shown in figure 35. The flexible tail acts as an inextensible cantilever beam clamped at the trailing edge of the foil. At flexibility level I, the tail is seen to bend predominantly in its first mode shape, as presented in figure 35(a). At flexibility level II, it exhibits mixed-mode oscillations involving both first and second modes with a significantly higher amplitude (figure 35c). In both these cases, the tip of the tail exhibits a ‘figure-of-eight’ motion (figures 35b,d). The phase portrait corresponding to level I in figure 35(b) is clearly indicative of a quasi-periodic dynamics, and this is triggered by the structure's coupled interactions with the quasi-periodic flow dynamics (presented in § 4.1). The flexibility level II case shows two distinct loops, representative of a period-2 dynamics (§ 4.2). Note that the flexible bending of the tail is influenced primarily by the trailing-edge separation in both the cases. However, the mild bending seen for level I does not prevent the LEV and the secondary vortices from interacting with the TEV. This gives way to the formation of the primary symmetry-breaking couple. At level II, the tail bends severely and even goes in the backward direction, taking an arch-like shape at the extreme positions. This highly deformed shape traps the LEVs and prevents the LEV–TEV interactions. Therefore, the resulting wake shape is dictated by the shedding of the strong TEVs, and the spatial symmetry of the wake is more or less retained.

Figure 35. For ![]() $\kappa h=1.5$, deflection envelopes and phase portraits for the tip displacement of the flexible tail for flexibility level I (a,b) and flexibility level II (c,d). (a) Mode shape structure for flexibility level I. (b) Phase plot of the free end of the trailing edge for flexibility level I. (c) Mode shape structure for flexibility level II. (d) Phase plot of the free end of the trailing edge for flexibility level II.
$\kappa h=1.5$, deflection envelopes and phase portraits for the tip displacement of the flexible tail for flexibility level I (a,b) and flexibility level II (c,d). (a) Mode shape structure for flexibility level I. (b) Phase plot of the free end of the trailing edge for flexibility level I. (c) Mode shape structure for flexibility level II. (d) Phase plot of the free end of the trailing edge for flexibility level II.
The pressure coefficient (![]() $C_P$) contours are compared in figure 36 for the three different tail configurations at the end of upstroke and downstroke of a typical cycle (
$C_P$) contours are compared in figure 36 for the three different tail configurations at the end of upstroke and downstroke of a typical cycle (![]() $t/T=110.25$ and
$t/T=110.25$ and ![]() $110.75$, respectively). For the rigid tail case, a large low-pressure zone is seen to be present near the leading edge. This is followed by a high-pressure zone extended up to the trailing end of the tail along the lower surface, at the beginning of the upstroke. This creates a strong adverse pressure gradient. Also, a reverse flow is observed close to the leading edge; see figure 36(a), where the velocity field is marked by the arrows. A strong CCW LEV (
$110.75$, respectively). For the rigid tail case, a large low-pressure zone is seen to be present near the leading edge. This is followed by a high-pressure zone extended up to the trailing end of the tail along the lower surface, at the beginning of the upstroke. This creates a strong adverse pressure gradient. Also, a reverse flow is observed close to the leading edge; see figure 36(a), where the velocity field is marked by the arrows. A strong CCW LEV (![]() $\boldsymbol {L2}$) gets shed due to the strong adverse pressure gradient, and, it interacts with the TEV subsequently to result in the formation of a symmetry-breaking couple. A similar strong low-pressure zone followed by a high-pressure zone on the upper surface of the foil is observed during the end of the downstroke (see figure 36d). Therefore, separation of the LEVs (
$\boldsymbol {L2}$) gets shed due to the strong adverse pressure gradient, and, it interacts with the TEV subsequently to result in the formation of a symmetry-breaking couple. A similar strong low-pressure zone followed by a high-pressure zone on the upper surface of the foil is observed during the end of the downstroke (see figure 36d). Therefore, separation of the LEVs (![]() $\boldsymbol {L2}$ and
$\boldsymbol {L2}$ and ![]() $\boldsymbol {L10}$, corresponding to figure 23) takes place from both upper and lower surfaces. This in turn results in the formation of upward and downward deflected symmetry-breaking couples. As discussed in the previous subsection, these symmetry-breaking couples are responsible for triggering jet-switching in case of the rigid configuration. In contrast, for flexibility level I, separation of the CCW LEV (
$\boldsymbol {L10}$, corresponding to figure 23) takes place from both upper and lower surfaces. This in turn results in the formation of upward and downward deflected symmetry-breaking couples. As discussed in the previous subsection, these symmetry-breaking couples are responsible for triggering jet-switching in case of the rigid configuration. In contrast, for flexibility level I, separation of the CCW LEV (![]() $\boldsymbol {L1}^*$) takes place only during the upstroke. Due to a high adverse pressure gradient, counter-clockwise LEVs are formed and separated at the lower surface during the upstroke. But the flow on the upper surface remains smooth, as indicated by the unidirectional vector field (figure 36(b) at
$\boldsymbol {L1}^*$) takes place only during the upstroke. Due to a high adverse pressure gradient, counter-clockwise LEVs are formed and separated at the lower surface during the upstroke. But the flow on the upper surface remains smooth, as indicated by the unidirectional vector field (figure 36(b) at ![]() $t/T=110.25$). During the downstroke, a reverse flow is observed along both the surfaces, and the CW LEV (
$t/T=110.25$). During the downstroke, a reverse flow is observed along both the surfaces, and the CW LEV (![]() $\boldsymbol {L2}^*$) in the upper surface does not get separated but circumnavigates the leading edge to traverse in the lower surface. So LEV–TEV interaction occurs only along the lower surface in every cycle. Hence the trailing wake remains deflected in the same direction always – though, depending on the strengths of the LEV and the TEV, the magnitude of the deflection angle changes as was presented in § 4.1. For level II, the low- and high-pressure zones get reduced significantly due to the severe deformation of the tail; see figures 36(c, f). Furthermore, the downstream low-pressure zone comes much closer to the foil, increasing the suction. This pulls the upstream fluid at a faster rate as indicated by the longer lengths of the arrows. Therefore, the LEVs
$\boldsymbol {L2}^*$) in the upper surface does not get separated but circumnavigates the leading edge to traverse in the lower surface. So LEV–TEV interaction occurs only along the lower surface in every cycle. Hence the trailing wake remains deflected in the same direction always – though, depending on the strengths of the LEV and the TEV, the magnitude of the deflection angle changes as was presented in § 4.1. For level II, the low- and high-pressure zones get reduced significantly due to the severe deformation of the tail; see figures 36(c, f). Furthermore, the downstream low-pressure zone comes much closer to the foil, increasing the suction. This pulls the upstream fluid at a faster rate as indicated by the longer lengths of the arrows. Therefore, the LEVs ![]() $(\boldsymbol {L1}^**$ and
$(\boldsymbol {L1}^**$ and ![]() $\boldsymbol {L2}^**)$ do not get sufficient time to grow and separate. Instead, the LEVs stay close to the body, roll over the surface, and get trapped eventually in the vicinity of the flexible tail. No shedding of them happens during the upstroke or the downstroke. The flow remains almost periodic, without the formation of any symmetry-breaking couples, thereby inhibiting the jet-switching process.
$\boldsymbol {L2}^**)$ do not get sufficient time to grow and separate. Instead, the LEVs stay close to the body, roll over the surface, and get trapped eventually in the vicinity of the flexible tail. No shedding of them happens during the upstroke or the downstroke. The flow remains almost periodic, without the formation of any symmetry-breaking couples, thereby inhibiting the jet-switching process.

Figure 36. For ![]() $\kappa h=1.5$, comparison of pressure coefficient
$\kappa h=1.5$, comparison of pressure coefficient ![]() $(C_P)$ contours during the end of (a–c) upstroke (
$(C_P)$ contours during the end of (a–c) upstroke (![]() $t/T=110.25$) and (d–f) downstroke (
$t/T=110.25$) and (d–f) downstroke (![]() $t/T=110.75$), for (a,d) rigid tail, (b,e) flexibility level I, and (c, f) flexibility level II. Lengths of arrows indicate the magnitude of the velocity vector field.
$t/T=110.75$), for (a,d) rigid tail, (b,e) flexibility level I, and (c, f) flexibility level II. Lengths of arrows indicate the magnitude of the velocity vector field.
4.6. Effect of the aft-tail length  $(L_t)$ on the flow-field dynamics
$(L_t)$ on the flow-field dynamics
The results presented so far have been able to identify tail flexibility as a potential instrument for inhibiting jet-switching, by following the flow-field interactions systematically and interlinking them with the associated nonlinear dynamical states. In the earlier subsections, interactions of the near-field vortices have been investigated, and the corresponding nonlinear dynamical states were established using different qualitative and quantitative flow-field measures, and time series tools. The flow mechanisms of flexibility influencing directly the formation of symmetry-breaking couples, which dictate the wake deflection characteristics and play a pivotal role in triggering jet-switching (Godoy-Diana et al. Reference Godoy-Diana, Marais, Aider and Wesfreid2009; Zheng & Wei Reference Zheng and Wei2012; Majumdar et al. Reference Majumdar, Bose and Sarkar2020b), were also highlighted. It is worth noting that in the previous subsections, the length of the flexible tail was kept constant, and the non-dimensional bending rigidity has been varied to increase the flexibility. However, one can change the degree of flexibility by changing the aft-tail length as well, keeping the non-dimensional bending rigidity fixed (which is not a function of the length in any implicit manner). The effect of aft-tail length on the wake dynamics is not clear from the existing literature and is of interest to the present subsection. The present results obtained so far in this direction indicate that the tail length, being another crucial parameter to govern the flexibility level of the system, can play an important role in regularising a non-periodic wake. Therefore, both the non-dimensional bending rigidity and the aft-tail length can be considered as alternative choices for benefiting the design of engineering devices, ensuring a periodic wake to maximise the aerodynamic efficiency.
Here, results are presented for a typical case of aft-tail length of ![]() $L_t=0.38c$, for
$L_t=0.38c$, for ![]() $\gamma = 0.1$ and
$\gamma = 0.1$ and ![]() $\kappa h=1.5$. As was shown in § 4.1, the wake deflected downwards with quasi-periodic dynamics for
$\kappa h=1.5$. As was shown in § 4.1, the wake deflected downwards with quasi-periodic dynamics for ![]() $L_t=0.3c$. But as
$L_t=0.3c$. But as ![]() $L_t$ is increased to
$L_t$ is increased to ![]() $0.38c$, the wake turns periodic, as can be confirmed by the
$0.38c$, the wake turns periodic, as can be confirmed by the ![]() $C_D$ time history shown in figure 37(a), which represents a completely periodic response with no amplitude modulation. The closed loop in the corresponding reconstructed phase space, dominant frequency peak with subharmonics and superharmonics in the frequency spectra, and narrow bands of harmonic frequencies in the wavelet spectra, further establish the periodic dynamics; see figures 37(b), 37(c) and 37(d), respectively. The instantaneous vorticity contours for three consecutive cycles (
$C_D$ time history shown in figure 37(a), which represents a completely periodic response with no amplitude modulation. The closed loop in the corresponding reconstructed phase space, dominant frequency peak with subharmonics and superharmonics in the frequency spectra, and narrow bands of harmonic frequencies in the wavelet spectra, further establish the periodic dynamics; see figures 37(b), 37(c) and 37(d), respectively. The instantaneous vorticity contours for three consecutive cycles (![]() $111{\rm th}$–
$111{\rm th}$–![]() $113{\rm th}$) are presented in figure 38. The flow field is seen to be repeating exactly from one cycle to another, confirming the periodicity of the wake. In contrast to the tail length
$113{\rm th}$) are presented in figure 38. The flow field is seen to be repeating exactly from one cycle to another, confirming the periodicity of the wake. In contrast to the tail length ![]() $L_t=0.3c$, the vortices are regularised, and the wake can be characterised by a reverse Kármán street with very mild upward deflection (
$L_t=0.3c$, the vortices are regularised, and the wake can be characterised by a reverse Kármán street with very mild upward deflection (![]() $\varTheta =1.65^{\circ }$) at
$\varTheta =1.65^{\circ }$) at ![]() $L_t=0.38$; see figures 39(a,b). The periodic nature of the flow field is confirmed further quantitatively from the vorticity correlation plot where the maximum value of
$L_t=0.38$; see figures 39(a,b). The periodic nature of the flow field is confirmed further quantitatively from the vorticity correlation plot where the maximum value of ![]() ${\rho }$ reaches unity in every cycle; see figure 39(c). Here,
${\rho }$ reaches unity in every cycle; see figure 39(c). Here, ![]() $\varTheta$ remains nearly constant with time, with a small positive value representative of the vortex street having a mild deflection (figure 40a). A slight dominance of upward deflecting couple
$\varTheta$ remains nearly constant with time, with a small positive value representative of the vortex street having a mild deflection (figure 40a). A slight dominance of upward deflecting couple ![]() $\boldsymbol {A}\unicode{x2013}\boldsymbol {B}$ can be visualised through the temporal evolution of
$\boldsymbol {A}\unicode{x2013}\boldsymbol {B}$ can be visualised through the temporal evolution of ![]() $U_{{dipole}}$-ratio,
$U_{{dipole}}$-ratio, ![]() $\xi$-ratio and
$\xi$-ratio and ![]() $\varGamma _{{avg}}$-ratio presented in figure 40(b). The
$\varGamma _{{avg}}$-ratio presented in figure 40(b). The ![]() $\xi$-ratio and
$\xi$-ratio and ![]() $U_{{dipole}}$-ratio are always below and above unity, respectively, and remain constant in every cycle. Therefore, the couple
$U_{{dipole}}$-ratio are always below and above unity, respectively, and remain constant in every cycle. Therefore, the couple ![]() $\boldsymbol {A}\unicode{x2013}\boldsymbol {B}$ always remains dominant, resulting in a slightly upward-looking wake. The continuous temporal evolution of the flow field for the present case is presented in supplementary movie 4.
$\boldsymbol {A}\unicode{x2013}\boldsymbol {B}$ always remains dominant, resulting in a slightly upward-looking wake. The continuous temporal evolution of the flow field for the present case is presented in supplementary movie 4.

Figure 37. Changed tail length: ![]() $\kappa h=1.5$,
$\kappa h=1.5$, ![]() $\gamma = 0.1$, aft-tail length
$\gamma = 0.1$, aft-tail length ![]() $L_t=0.38c$. Time series analysis of
$L_t=0.38c$. Time series analysis of ![]() $C_D$ indicates periodic dynamics: (a) time history, (b) reconstructed phase portrait, (c) frequency spectra, and (d) wavelet transform.
$C_D$ indicates periodic dynamics: (a) time history, (b) reconstructed phase portrait, (c) frequency spectra, and (d) wavelet transform.

Figure 38. Changed tail length: ![]() $\kappa h=1.5$,
$\kappa h=1.5$, ![]() $\gamma = 0.1$, aft-tail length
$\gamma = 0.1$, aft-tail length ![]() $L_t=0.38c$. Instantaneous vorticity contours during
$L_t=0.38c$. Instantaneous vorticity contours during ![]() $111{\rm th}$–
$111{\rm th}$–![]() $113{\rm th}$ cycles.
$113{\rm th}$ cycles.

Figure 39. Changed tail length: ![]() $\kappa h=1.5$,
$\kappa h=1.5$, ![]() $\gamma = 0.1$, aft-tail length
$\gamma = 0.1$, aft-tail length ![]() $L_t=0.38c$. (a) Instantaneous vorticity and (b) velocity magnitude, contours depicting reverse Kármán wake with mild upward deflection; and (c) vorticity correlation.
$L_t=0.38c$. (a) Instantaneous vorticity and (b) velocity magnitude, contours depicting reverse Kármán wake with mild upward deflection; and (c) vorticity correlation.

Figure 40. Changed tail length: ![]() $\kappa h=1.5$,
$\kappa h=1.5$, ![]() $\gamma = 0.1$, aft-tail length
$\gamma = 0.1$, aft-tail length ![]() $L_t=0.38c$. (a) Wake deflection angle and (b) quantitative measures associated with the vortex system. Dominant effect of the upward deflecting vortex couple
$L_t=0.38c$. (a) Wake deflection angle and (b) quantitative measures associated with the vortex system. Dominant effect of the upward deflecting vortex couple ![]() $\boldsymbol {A}\unicode{x2013}\boldsymbol {B}$ results in a positive deflection angle.
$\boldsymbol {A}\unicode{x2013}\boldsymbol {B}$ results in a positive deflection angle.
The deflection envelope and the corresponding phase plot of the tail-tip are presented in figure 41. The tail bends with a little asymmetry and shows mostly a first-mode oscillation profile. The mechanism by which the tail influences the near field can be understood better from the ![]() $C_P$ contour during the end of upstroke and downstroke as shown in figure 42. The downstream low-pressure zone created by the deflection of the tail sucks the upstream fluid at a faster rate, as indicated by the longer lengths of the arrows. Hence the LEVs do not get sufficient time to grow and separate, instead getting trapped in the vicinity of the tail, similar to flexibility level II as was shown in § 4.5. The flow remains periodic without the formation of any symmetry-breaking couples, thereby inhibiting jet-switching and maintaining periodicity. This also evidently underlines the role of the length of the tail in influencing the dynamical transitions of the system. Therefore, an appropriate selection of the aft-tail length and bending rigidity inhibits the aperiodicity in the flow field. To the best of the authors’ knowledge, the effect of aft-tail length in inhibiting the jet-switching and regularising the flow field has not yet been taken up adequately in the existing literature. Note that design of flapping devices based on natural flyers/swimmers would like to avoid aperiodicity to propel efficiently by exploiting the combination of the length of flapping appendages and the wing/fin morphology. The present results contribute towards that by adding insights on the role of flexibility in suppressing the switching and inhibiting aperiodicity in the wake. Currently, the authors are studying the effect of a flexible aft-tail in delaying the onset of aperiodicity at higher values of
$C_P$ contour during the end of upstroke and downstroke as shown in figure 42. The downstream low-pressure zone created by the deflection of the tail sucks the upstream fluid at a faster rate, as indicated by the longer lengths of the arrows. Hence the LEVs do not get sufficient time to grow and separate, instead getting trapped in the vicinity of the tail, similar to flexibility level II as was shown in § 4.5. The flow remains periodic without the formation of any symmetry-breaking couples, thereby inhibiting jet-switching and maintaining periodicity. This also evidently underlines the role of the length of the tail in influencing the dynamical transitions of the system. Therefore, an appropriate selection of the aft-tail length and bending rigidity inhibits the aperiodicity in the flow field. To the best of the authors’ knowledge, the effect of aft-tail length in inhibiting the jet-switching and regularising the flow field has not yet been taken up adequately in the existing literature. Note that design of flapping devices based on natural flyers/swimmers would like to avoid aperiodicity to propel efficiently by exploiting the combination of the length of flapping appendages and the wing/fin morphology. The present results contribute towards that by adding insights on the role of flexibility in suppressing the switching and inhibiting aperiodicity in the wake. Currently, the authors are studying the effect of a flexible aft-tail in delaying the onset of aperiodicity at higher values of ![]() $\kappa h$.
$\kappa h$.

Figure 41. Changed tail length: ![]() $\kappa h=1.5$,
$\kappa h=1.5$, ![]() $\gamma = 0.1$, aft-tail length
$\gamma = 0.1$, aft-tail length ![]() $L_t=0.38c$, (a) Deflection envelopes, mode shape structure. (b) Phase plot of the tail-tip of the flexible tail.
$L_t=0.38c$, (a) Deflection envelopes, mode shape structure. (b) Phase plot of the tail-tip of the flexible tail.

Figure 42. Changed tail length: ![]() $\kappa h=1.5$,
$\kappa h=1.5$, ![]() $\gamma = 0.1$, aft-tail length
$\gamma = 0.1$, aft-tail length ![]() $L_t=0.38c$. Pressure coefficient
$L_t=0.38c$. Pressure coefficient ![]() $(C_P)$ contours during the end of (a) upstroke (
$(C_P)$ contours during the end of (a) upstroke (![]() $t/T=110.25$), and (b) downstroke (
$t/T=110.25$), and (b) downstroke (![]() $t/T=110.75$).
$t/T=110.75$).
5. Conclusions
The present study analyses the role of tail flexibility in suppressing the jet-switching phenomenon in the wake of a heaving foil, and interlinks the underlying flow-field interaction mechanisms with the corresponding nonlinear dynamical signatures of the wake. With a fully rigid aft-tail, mild jet-switching is observed at ![]() $\kappa h=1.2$, due to quasi-periodic movement of the wake vortices. Vigorous and frequent jet-switching with large deflection is observed at
$\kappa h=1.2$, due to quasi-periodic movement of the wake vortices. Vigorous and frequent jet-switching with large deflection is observed at ![]() $\kappa h=1.5$, due to sporadic bursts of aperiodic interactions of near-field vortices, as the dynamics changes from quasi-periodicity to the state of intermittency. During the intermittent bursts, the primary LEV interacts directly with the TEV that gives way to the formation of strong symmetry-breaking couples, which trigger the switching phenomenon. The LEV–TEV interactions take place from both upper and lower surfaces of the body, thus forming alternate (upward and downward deflecting) symmetry-breaking couples.
$\kappa h=1.5$, due to sporadic bursts of aperiodic interactions of near-field vortices, as the dynamics changes from quasi-periodicity to the state of intermittency. During the intermittent bursts, the primary LEV interacts directly with the TEV that gives way to the formation of strong symmetry-breaking couples, which trigger the switching phenomenon. The LEV–TEV interactions take place from both upper and lower surfaces of the body, thus forming alternate (upward and downward deflecting) symmetry-breaking couples.
Two different flexibility levels of the aft-tail have been considered in the present study. In the presence of flexibility, the intermittent dynamics and the associated vigorous jet-switching are suppressed through a regularisation of the flow field. At the lower flexibility level, a downward deflected reverse Kármán wake with varying deflection angle is observed. In this case, the flexible tail modifies the near field in such a way that the LEV–TEV interactions take place only along the lower surface in each cycle, thereby producing only one type of symmetry-breaking couple (downward deflecting). This makes the resulting wake stay deflected in the downward direction for all time. At the higher flexibility of the aft-tail, the flow field gets further regularised, and the wake settles into a periodic reverse Kármán street with a mild deflection. In this case, a highly deformed tail prevents LEV–TEV interactions completely by trapping the LEV structures within its vicinity. This inhibits the formation of any strong symmetry-breaking couples, and as a result, jet-switching gets suppressed. Similar regularisation in the wake can be achieved by altering the aft-tail length. For higher aft-tail length at a moderate rigidity level, the wake was seen to settle into an almost perfect reverse Kármán street. Thus the regularisation of the non-periodic flow field can be achieved in both ways.
Supplementary material and movies
Supplementary material and movies are available at https://doi.org/10.1017/jfm.2022.591.
Declaration of interests
The authors report no conflict of interest.