Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Klewicki, J.
Philip, J.
Marusic, I.
Chauhan, K.
and
Morrill-Winter, C.
2014.
Self-similarity in the inertial region of wall turbulence.
Physical Review E,
Vol. 90,
Issue. 6,
Lee, Myoungkyu
and
Moser, Robert D.
2015.
Direct numerical simulation of turbulent channel flow up to .
Journal of Fluid Mechanics,
Vol. 774,
Issue. ,
p.
395.
Vallikivi, M.
Hultmark, M.
and
Smits, A. J.
2015.
Turbulent boundary layer statistics at very high Reynolds number.
Journal of Fluid Mechanics,
Vol. 779,
Issue. ,
p.
371.
Rodríguez-López, Eduardo
Bruce, Paul J. K.
and
Buxton, Oliver R. H.
2015.
A robust post-processing method to determine skin friction in turbulent boundary layers from the velocity profile.
Experiments in Fluids,
Vol. 56,
Issue. 4,
Klewicki, J.
and
Oberlack, M.
2015.
Finite Reynolds number properties of a turbulent channel flow similarity solution.
Physics of Fluids,
Vol. 27,
Issue. 9,
Borisenkov, Youry
Kholmyansky, Michael
Krylov, Slava
Liberzon, Alex
and
Tsinober, Arkady
2015.
Multiarray Micromachined Probe for Turbulence Measurements Assembled of Suspended Hot-Film Sensors.
Journal of Microelectromechanical Systems,
Vol. 24,
Issue. 5,
p.
1503.
Schaefer, John A.
West, Thomas
Hosder, Serhat
Rumsey, Christopher L.
Carlson, Jan-Renee
and
Kleb, William L.
2015.
Uncertainty Quantification of Turbulence Model Closure Coefficients for Transonic Wall-Bounded Flows.
Hohman, T.C.
Buren, T. Van
Martinelli, L.
and
Smits, A.J.
2015.
Generating an artificially thickened boundary layer to simulate the neutral atmospheric boundary layer.
Journal of Wind Engineering and Industrial Aerodynamics,
Vol. 145,
Issue. ,
p.
1.
Trettel, Andrew
and
Larsson, Johan
2016.
Mean velocity scaling for compressible wall turbulence with heat transfer.
Physics of Fluids,
Vol. 28,
Issue. 2,
Ostilla-Mónico, Rodolfo
Verzicco, Roberto
Grossmann, Siegfried
and
Lohse, Detlef
2016.
The near-wall region of highly turbulent Taylor–Couette flow.
Journal of Fluid Mechanics,
Vol. 788,
Issue. ,
p.
95.
Örlü, Ramis
Segalini, Antonio
Klewicki, Joseph
and
Alfredsson, P. Henrik
2016.
High-order generalisation of the diagnostic scaling for turbulent boundary layers.
Journal of Turbulence,
Vol. 17,
Issue. 7,
p.
664.
Schaefer, John A.
Hosder, Serhat
Mani, Mortaza
Cary, Andrew W.
and
Krakos, Joshua
2016.
The Effect of Grid Topology and Flow Solver on Turbulence Model Closure Coefficient Uncertainties for a Transonic Airfoil.
Bailey, S. C. C.
and
Witte, B. M.
2016.
On the universality of local dissipation scales in turbulent channel flow.
Journal of Fluid Mechanics,
Vol. 786,
Issue. ,
p.
234.
Cameron, S. M.
Nikora, V. I.
and
Stewart, M. T.
2017.
Very-large-scale motions in rough-bed open-channel flow.
Journal of Fluid Mechanics,
Vol. 814,
Issue. ,
p.
416.
Mouri, Hideaki
Morinaga, Takeshi
Yagi, Toshimasa
and
Mori, Kazuyasu
2017.
Logarithmic scaling for fluctuations of a scalar concentration in wall turbulence.
Physical Review E,
Vol. 96,
Issue. 6,
Ansorge, Cedrick
2017.
Analyses of Turbulence in the Neutrally and Stably Stratified Planetary Boundary Layer.
p.
75.
Schaefer, John
Hosder, Serhat
West, Thomas
Rumsey, Christopher
Carlson, Jan-Renee
and
Kleb, William
2017.
Uncertainty Quantification of Turbulence Model Closure Coefficients for Transonic Wall-Bounded Flows.
AIAA Journal,
Vol. 55,
Issue. 1,
p.
195.
Schaefer, John A.
Cary, Andrew W.
Mani, Mortaza
and
Spalart, Philippe R.
2017.
Uncertainty Quantification and Sensitivity Analysis of SA Turbulence Model Coefficients in Two and Three Dimensions.
Fernandez, P.
Nguyen, N.C.
and
Peraire, J.
2017.
The hybridized Discontinuous Galerkin method for Implicit Large-Eddy Simulation of transitional turbulent flows.
Journal of Computational Physics,
Vol. 336,
Issue. ,
p.
308.
Camassa, Roberto
Ogrosky, H. Reed
and
Olander, Jeffrey
2017.
Viscous film-flow coating the interior of a vertical tube. Part 2. Air-driven flow.
Journal of Fluid Mechanics,
Vol. 825,
Issue. ,
p.
1056.
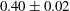 $0.40 \pm 0.02$. A more precise estimate will require improved instrumentation.
$0.40 \pm 0.02$. A more precise estimate will require improved instrumentation.

