Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Wang, Shizhao
Shi, Beiji
Li, Yuhang
and
He, Guowei
2016.
A large eddy simulation of flows around an underwater vehicle model using an immersed boundary method.
Theoretical and Applied Mechanics Letters,
Vol. 6,
Issue. 6,
p.
302.
Posa, Antonio
Lippolis, Antonio
and
Balaras, Elias
2016.
Investigation of Separation Phenomena in a Radial Pump at Reduced Flow Rate by Large-Eddy Simulation.
Journal of Fluids Engineering,
Vol. 138,
Issue. 12,
Posa, Antonio
Parker, Colin M.
Leftwich, Megan C.
and
Balaras, Elias
2016.
Wake structure of a single vertical axis wind turbine.
International Journal of Heat and Fluid Flow,
Vol. 61,
Issue. ,
p.
75.
Posa, Antonio
and
Balaras, Elias
2018.
Large Eddy Simulation of an isolated vertical axis wind turbine.
Journal of Wind Engineering and Industrial Aerodynamics,
Vol. 172,
Issue. ,
p.
139.
Jin, Zhihui
Wang, Peng
Xia, Hui
Ding, Yongle
and
Han, Peng
2018.
Validation of Numerical Simulation on the Flow Field of Submarine with Various Types of stern appendages.
p.
1.
Kumar, Praveen
and
Mahesh, Krishnan
2018.
Large-eddy simulation of flow over an axisymmetric body of revolution.
Journal of Fluid Mechanics,
Vol. 853,
Issue. ,
p.
537.
Yane, T.
and
Subaschandar, N.
2018.
Mean velocity behaviour in the near wake of long slender cylinder with a sharp trailing edge at a high Reynolds number.
Journal of Turbulence,
Vol. 19,
Issue. 10,
p.
910.
Weiss, Pierre-Élie
and
Deck, Sébastien
2018.
On the coupling of a zonal body-fitted/immersed boundary method with ZDES: Application to the interactions on a realistic space launcher afterbody flow.
Computers & Fluids,
Vol. 176,
Issue. ,
p.
338.
Posa, Antonio
and
Balaras, Elias
2018.
Large-Eddy Simulations of a notional submarine in towed and self-propelled configurations.
Computers & Fluids,
Vol. 165,
Issue. ,
p.
116.
Jones, Matthew C.
and
Paterson, Eric G.
2018.
Effect of Hull–Form Variation on the Stratified Near Wake of a Self–Propelled Body.
p.
1.
Posa, Antonio
and
Lippolis, Antonio
2018.
A LES investigation of off-design performance of a centrifugal pump with variable-geometry diffuser.
International Journal of Heat and Fluid Flow,
Vol. 70,
Issue. ,
p.
299.
Jones, Matthew C.
and
Paterson, Eric G.
2018.
Influence of Propulsion Type on the Stratified Near Wake of an Axisymmetric Self-Propelled Body.
Journal of Marine Science and Engineering,
Vol. 6,
Issue. 2,
p.
46.
Shi, Beiji
Yang, Xiaolei
Jin, Guodong
He, Guowei
and
Wang, Shizhao
2019.
Wall-modeling for large-eddy simulation of flows around an axisymmetric body using the diffuse-interface immersed boundary method.
Applied Mathematics and Mechanics,
Vol. 40,
Issue. 3,
p.
305.
Beratlis, Nikolaos
Balaras, Elias
and
Squires, Kyle
2019.
On the origin of the drag force on dimpled spheres.
Journal of Fluid Mechanics,
Vol. 879,
Issue. ,
p.
147.
Ma, Ming
Huang, Wei-Xi
and
Xu, Chun-Xiao
2019.
A dynamic wall model for large eddy simulation of turbulent flow over complex/moving boundaries based on the immersed boundary method.
Physics of Fluids,
Vol. 31,
Issue. 11,
Huang, Wei-Xi
and
Tian, Fang-Bao
2019.
Recent trends and progress in the immersed boundary method.
Proceedings of the Institution of Mechanical Engineers, Part C: Journal of Mechanical Engineering Science,
Vol. 233,
Issue. 23-24,
p.
7617.
Józsa, T. I.
Balaras, E.
Kashtalyan, M.
Borthwick, A. G. L.
and
Viola, I. M.
2019.
Active and passive in-plane wall fluctuations in turbulent channel flows.
Journal of Fluid Mechanics,
Vol. 866,
Issue. ,
p.
689.
Posa, Antonio
2019.
Wake characterization of coupled configurations of vertical axis wind turbines using Large Eddy Simulation.
International Journal of Heat and Fluid Flow,
Vol. 75,
Issue. ,
p.
27.
Posa, Antonio
Broglia, Riccardo
Felli, Mario
Falchi, Massimo
and
Balaras, Elias
2019.
Characterization of the wake of a submarine propeller via Large-Eddy simulation.
Computers & Fluids,
Vol. 184,
Issue. ,
p.
138.
Posa, Antonio
and
Lippolis, Antonio
2019.
Effect of working conditions and diffuser setting angle on pressure fluctuations within a centrifugal pump.
International Journal of Heat and Fluid Flow,
Vol. 75,
Issue. ,
p.
44.
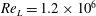 $\mathit{Re}_{L}=1.2\times 10^{6}$ (based on the free-stream velocity and the length of the body). The computational grid, composed of approximately 3 billion nodes, is designed to capture all essential flow features, including the turbulent boundary layers on the surface of the body. Our results are in good agreement with measurements available in the literature. It is shown that the wake of the body is affected mainly by the shear layer from the trailing edge of the fins and the turbulent boundary layer growing along the stern, while the influence of the wake of the sail is minimal. In agreement with the reference experiments, a bimodal behaviour for the turbulent stresses is observed in the wake. This is due to the displacement of the maximum of turbulent kinetic energy away from the wall along the surface of the stern, where the boundary layer is subjected to strong adverse pressure gradients. The junction flows, produced by the interaction of the boundary layer with the leading edge of the fins, enhance this bimodal pattern, feeding additional turbulence in the boundary layer and the downstream wake. The evolution of the wake towards self-similarity is also investigated up to nine diameters downstream of the tail. We found the mean flow approaches this condition, while its development is delayed by the wake of the appendages, especially by the flow coming from the tip of the fins. However, the width of the wake and its maximum momentum deficit follow the expected power-law behaviour on the side away from the sail. The second-order statistics, on the other hand, are still far from self-similarity, which is consistent with experimental observations in the literature.
$\mathit{Re}_{L}=1.2\times 10^{6}$ (based on the free-stream velocity and the length of the body). The computational grid, composed of approximately 3 billion nodes, is designed to capture all essential flow features, including the turbulent boundary layers on the surface of the body. Our results are in good agreement with measurements available in the literature. It is shown that the wake of the body is affected mainly by the shear layer from the trailing edge of the fins and the turbulent boundary layer growing along the stern, while the influence of the wake of the sail is minimal. In agreement with the reference experiments, a bimodal behaviour for the turbulent stresses is observed in the wake. This is due to the displacement of the maximum of turbulent kinetic energy away from the wall along the surface of the stern, where the boundary layer is subjected to strong adverse pressure gradients. The junction flows, produced by the interaction of the boundary layer with the leading edge of the fins, enhance this bimodal pattern, feeding additional turbulence in the boundary layer and the downstream wake. The evolution of the wake towards self-similarity is also investigated up to nine diameters downstream of the tail. We found the mean flow approaches this condition, while its development is delayed by the wake of the appendages, especially by the flow coming from the tip of the fins. However, the width of the wake and its maximum momentum deficit follow the expected power-law behaviour on the side away from the sail. The second-order statistics, on the other hand, are still far from self-similarity, which is consistent with experimental observations in the literature.