Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Shaqfeh, Eric S.G.
and
Khomami, Bamin
2021.
The Oldroyd-B fluid in elastic instabilities, turbulence and particle suspensions.
Journal of Non-Newtonian Fluid Mechanics,
Vol. 298,
Issue. ,
p.
104672.
Lopez, Jose M.
2022.
Vortex merging and splitting events in viscoelastic Taylor–Couette flow.
Journal of Fluid Mechanics,
Vol. 946,
Issue. ,
Song, Jiaxing
Liu, Nansheng
Lu, Xi-Yun
and
Khomami, Bamin
2022.
Direct numerical simulation of elastic turbulence in the Taylor–Couette flow: transition pathway and mechanistic insight.
Journal of Fluid Mechanics,
Vol. 949,
Issue. ,
Castillo Sánchez, Hugo A.
Jovanović, Mihailo R.
Kumar, Satish
Morozov, Alexander
Shankar, V.
Subramanian, Ganesh
and
Wilson, Helen J.
2022.
Understanding viscoelastic flow instabilities: Oldroyd-B and beyond.
Journal of Non-Newtonian Fluid Mechanics,
Vol. 302,
Issue. ,
p.
104742.
Song, Jiaxing
Lin, Fenghui
Zhu, Yabiao
Wan, Zhen-Hua
Liu, Nansheng
Lu, Xi-Yun
and
Khomami, Bamin
2023.
Self-sustaining cycle of purely elastic turbulence.
Physical Review Fluids,
Vol. 8,
Issue. 1,
Zhu, Yabiao
Wan, Zhenhua
Lin, Fenghui
Liu, Nansheng
Lu, Xiyun
and
Khomami, Bamin
2023.
Maximum drag enhancement asymptote in spanwise-rotating viscoelastic plane Couette flow of dilute polymeric solutions.
Journal of Fluid Mechanics,
Vol. 958,
Issue. ,
Lin, Fenghui
Song, Jiaxing
Zhao, Zhiye
Liu, Nansheng
Lu, Xi-Yun
and
Khomami, Bamin
2023.
A novel transition route to elastically dominated turbulence in viscoelastic Taylor–Couette flow.
Journal of Non-Newtonian Fluid Mechanics,
Vol. 312,
Issue. ,
p.
104968.
Song, Jiaxing
Zhu, Yabiao
Lin, Fenghui
Liu, Nansheng
and
Khomami, Bamin
2023.
Turbulent Taylor–Couette flow of dilute polymeric solutions: a 10-year retrospective.
Philosophical Transactions of the Royal Society A: Mathematical, Physical and Engineering Sciences,
Vol. 381,
Issue. 2243,
Boulafentis, T.
Lacassagne, T.
Cagney, N.
and
Balabani, S.
2023.
Experimental insights into elasto-inertial transitions in Taylor–Couette flows.
Philosophical Transactions of the Royal Society A: Mathematical, Physical and Engineering Sciences,
Vol. 381,
Issue. 2243,
Lopez, Jose M.
and
Altmeyer, Sebastian A.
2023.
Arrow-shaped elasto-inertial rotating waves.
Philosophical Transactions of the Royal Society A: Mathematical, Physical and Engineering Sciences,
Vol. 381,
Issue. 2246,
Hayani Choujaa, Mohamed
Riahi, Mehdi
and
Aniss, Saïd
2024.
Tricritical state and quasi-periodicity triggered by the non-linear elasticity in an Upper Convected Maxwell fluid confined between two co-oscillating cylinders about zero-mean.
Journal of Non-Newtonian Fluid Mechanics,
Vol. 325,
Issue. ,
p.
105202.
Lin, Fenghui
Song, Jiaxing
Liu, Nansheng
Wan, Zhenhua
Lu, Xi-Yun
and
Khomami, Bamin
2024.
Maximum drag enhancement asymptote in turbulent Taylor–Couette flow of dilute polymeric solutions.
Journal of Non-Newtonian Fluid Mechanics,
Vol. 323,
Issue. ,
p.
105172.
Boulafentis, T.
Lacassagne, T.
Cagney, N.
and
Balabani, S.
2024.
Coherent structures of elastoinertial instabilities in Taylor–Couette flows.
Journal of Fluid Mechanics,
Vol. 986,
Issue. ,
Carré, Charles
Moazzen, Masoud
Lacassagne, Tom
and
Bahrani, S. Amir
2024.
Elasto-inertial dissipation in particle-laden viscoelastic Taylor–Couette flow.
Journal of Fluid Mechanics,
Vol. 997,
Issue. ,
Zhang, Yi-Bao
Fan, Yaning
Su, Jinghong
Xi, Heng-Dong
and
Sun, Chao
2025.
Global drag reduction and local flow statistics in Taylor–Couette turbulence with dilute polymer additives.
Journal of Fluid Mechanics,
Vol. 1002,
Issue. ,
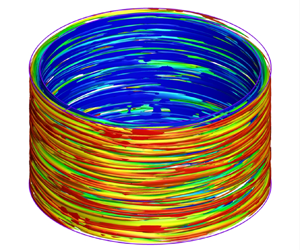
 $-3.5$ scaling law of the energy spectrum reminiscent of elastic turbulence. The flow states involved are distinctly different to those observed in the reverse transition route from inertial turbulence via a relaminarization of the flow to elasto-inertial turbulence in parallel shear flows, underscoring the importance of polymer-induced hoop stresses in realizing EDT that are absent in parallel shear flows.
$-3.5$ scaling law of the energy spectrum reminiscent of elastic turbulence. The flow states involved are distinctly different to those observed in the reverse transition route from inertial turbulence via a relaminarization of the flow to elasto-inertial turbulence in parallel shear flows, underscoring the importance of polymer-induced hoop stresses in realizing EDT that are absent in parallel shear flows.