Hostname: page-component-586b7cd67f-vdxz6 Total loading time: 0 Render date: 2024-11-29T02:36:31.077Z Has data issue: false hasContentIssue false
  • English
  • Français

Short Notes: New Data on the Thermal Conductivity of Natural Snow

Published online by Cambridge University Press:  30 January 2017

Gunter E. Weller  and
Peter Schwkkdtfecjer
Gunter E. Weller
Affiliation:
Geophysical Institute. University of Alaska, College, Alaska 99735, U.S.A.
Peter Schwkkdtfecjer
Affiliation:
Meteorology Department, University of Melbourne, Parkville, Victoria 3052, Australia
Rights & Permissions [Opens in a new window]

Abstract

The thermal conductivity and diffusivity of natural snow computed from Fourier-type analyses of annual snow temperature variations are shown to be strongly temperature dependent. The computed temperature coefficients of-0.007 and -0.012 deg-1 respectively, agree well with older laboratory experiments carried out on polycrystalline ice.

Résumé

Résumé

D’après les analyses par séries de Fourier des variations annuelles de la température de la neige, on montre que la conductivité et la diffusivité thermique de la neige naturelle dépendent beaucoup de la température. Les coefficients thermiques calculés égaux respectivement à 0,007 et -0,012 deg-1, sont en bon accord avec des résultats de laboratoire plus anciens obtenus sur de la glace polycristalline.

Zusammenfassung

Zusammenfassung

Die Wärmeleitfähigkeit und—durchlässigkeit natürlichen Schnees—berechnet aus Fourier-Analysen von jährlichen Änderungen der Schneetemperatur—erweisen sich als stark temperaturabhängig. Die errechneten Tcmperaturkoeflizienten von 0,007 bzw. 0,012 deg-1 stimmen mit älteren Laborergebnissen, die an polykristallinem Eis durchgeführt wurden, gut überein.

Type
Research Article
Information
Journal of Glaciology , Volume 10 , Issue 59 , 1971 , pp. 309 - 311
Copyright
Copyright © International Glaciological Society 1971

Tue thermal conductivity of pure polycrystalline ice at 0°C is approximately four times that of water at that temperature. The international critical tables contain two values for ice at 0°C which differ by 5%. Reference Sehofield, Hall and WashburnSchofield and Hall (1927) selected a value of 2.20 W m-1 deg-1, whereas Reference Dusen and WashburnVan Dusen (1929) gave a value of 2.09 W -1mdeg-1. Subsequent measurements as listed by Reference PowellPowell (1958) have confirmed that the I.C.T. values are approximately correct and have suggested that crystal anisotropy could possibly account for the observed small differences. The position is much less satisfactory at lower temperatures where large differences exist between the results of Reference LeesLees (1905) and Reference Jakob and ErkJakob and Erk (1929). The latter’s results appear to be more reliable, since Reference Dillard and TimmerhausDillard and Timmerhaus (1966) have reproduced these values experimentally and Reference RatcliffeRatcliffe’s (1962) values also agree to within about 12% at -120°C with those of Jakob and Erk. All these values are for polycrystalline ice. Moreover, the thermal conductivity of many crystalline materials has been found to be proportional to the reciprocal of the absolute temperature within a certain temperature range (Reference EukenEuken, 1911). Such a relation down to 100 K is more nearly satisfied by the data of Jakob and Erk than those of Lees, and also fits the data of Dillard and Timmerhaus and of Ratcliffe. Recently, Reference FletcherFletcher (1970) has described a “hump" effect in the temperature relationship at low temperatures, which has also been accurately measured by Reference Klinger and NeumaÏerKlinger and Neumaier (1969).

In snow, numerous measurements of the thermal conductivity exist close to the melting point. Summaries of these (Reference MantisMantis, 1951; Reference MellorMellor, 1964) show considerable divergence of the results owing partly to the difficulties in measuring low snow densities correctly and in neglecting structural differences in snow samples, and convective and radiative heat transfer processes in the snow. Theoretical attempts to formulate the thermal conductivity of snow as a function of density include a recent paper by Reference SchwerdtfegerSchwerdtfeger (1963). No data on the temperature coefficients are available.

The re-analysis of earlier measurements (Reference SehyttSchytt, 1958) of subsurface temperatures at Maudheim, Antarctica has provided a new value of the thermal conductivity of ice of density 0.57 Mg m-1 (Reference Dalrymple, Dalrymple, Lettau, Wollaston and RubinDalrymple and others, 1966). Similarly, a recent analysis of annual sub-surface temperatures at “Plateau" station on the central Antarctic plateau has given a new value of the thermal conductivity of snow of density 0.42 Mg m-3 (Reference Weller and SchwerddcgerWeller and Schwerdtfeger, 1968). These two new values were determined from Fourier-type analyses at mean annual snow temperatures of - 17°C and 60°C respectively, at depths where non-conductive processes of heat transfer were shown to be negligible. By comparing these results, listed in the table below, with mean weighted values of a series of measurements close to the freezing point, taken from Reference MellorMellor (1964), the temperature coefficients of the thermal conductivities and diffusivities could be deduced. They are assumed to be linear over the temperature range considered, as shown approximately by the laboratory results of Reference Jakob and ErkJakob and Erk (1929), Reference Dillard and TimmerhausDillard and Timmerhaus (1966), and Reference RatcliffeRatcliffe (1962). Changes of specific heat of the ice with temperature were taken from Reference DorseyDorsey (1940).

Table I. Tiif.rmal propiïkîiks of tcf.

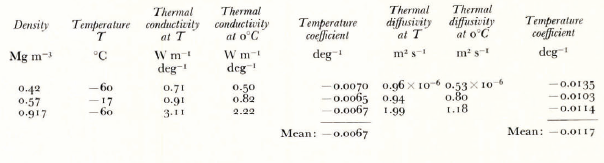
There is reasonable agreement between the temperature coefficients at different densities, and this further confirms the values obtained by Jakob and Erk.

The temperature dependence of the thermal properties can be seen to be anything but negligible, even for small temperature changes near the freezing point. This is usually not considered in heat-flux computations in ice and snow and may lead to considerable errors.

References

Dalrymple, P.C. 1966 South Pole micrometeorology program: data analysis, [by] Dalrymple, P.C.,Lettau, H.H,Wollaston, S.H.. (In Rubin, M.J., ed. Studies in Antarctic meteorology. Washington D.C., American Geophysical Union p. 13–57. (Antarctic Research Series, Vol. 9.)) Google Scholar
Dillard, S. Timmerhaus, K.D. 1966 Low temperature thermal conductivity of solidified H2O and D2O. Pure and Applied Cryogenics, Vol. 4, p. 35–44. Google Scholar
Dorsey, N.E. 1940 Properties of ordinary water–substance in all its phases: water=vapor, water and all the ices New York, Reinhold Publishing Corporation. (American Chemical Society. Monograph Series, No. 81.) Google Scholar
Euken, A. 1911 Über die Temperaturabhängigkeit der Wärmeleitfähigkeit fester Nichtmetalle. Annalen der Physik, Vierte Folge, Bd. 34, Ht. 2, p. 185–221. CrossRefGoogle Scholar
Fletcher, N.H. 1970 The chemical physics of ice. Cambridge, University Press. (Cambridge Monographs on Physics.) CrossRefGoogle Scholar
Jakob, M. Erk, S. 1928 Wärmedehnung des Eises zwischen o und –253°. Zeitschrift für die gesamte Kälteindustrie, Bd. 35, p. 125–30. Google Scholar
Klinger, J. NeumaÏer, K. 1969 Conductibilité thermique de la glace. Comptes Readies Hebdomadaires des Séances de l’Académie des Sciences (Paris), Sér. B, Tom. 269, No. 19, p. 945–48. Google Scholar
Lees, C.H. 1905 Effect of temperature on thermal conductivities of electrical insulators. Philosophical Transactions of the Royal Society, Ser. A, Vol. 204, Art. 12, p. 433–66. Google Scholar
Mantis, H.T. 1951 Review of I he properties of snow and ice. U.S. Sno, Ice and Permafrost Research Establishment. Report 4.Google Scholar
Mellor, M. 1964 Properties ôf snow, U.S. Cold Regions Research and Engineering Laboratory. Cold regions science and engineering. Hanover N.H., Pt. III, Sect. At. Google Scholar
Powell, R.W. 1958 Thermal conductivities and expansion coefficients of water and ice. Advances m Physics, Vol. 7, No. 26, p. 276–97. CrossRefGoogle Scholar
Ratcliffe, E.H. 1962 The thermal conductivity of ice: new data on the temperature coefficient. Philosophical Magazine, Eighth Ser., Vol. 7, No. 79, p. 1197–203. CrossRefGoogle Scholar
Sehofield, F.H. Hall, J.A. 1927 Thermal insulating materials for moderate and low temperatures. (In Washburn, E.W. ed. International critical tables of numerical data, physics, chemistry and technology. New York and London, McGraw–Hill. Vol. 2, p. 312–16.) Google Scholar
Schwerdtfeger, P. 1963 Theoretical derivation of the thermal conductivity and diffusivity of snow. Union Géodésique et Géophysique Internationale. Association Internationale d’Hydrologie Scientifique. Assemblée générale de Berkeley, 19–8–31–8 1963 Commission des. Neiges et des Glaces, p. 75–81. Google Scholar
Sehytt, V. 1960 Glaciology. II. Snow and ice temperatures in Dronning Maud Land. Norwegian–British–Swedish Antarctic Expedition, 1949–52. Scientific Results, Vol. 4D, p. 153–79. Google Scholar
Dusen, M.S. 1929 Thermal conductivity of non–metallic solids. (In Washburn, E. W., ed. International critical tables of numerical data, physics, chemistry and technology. New York and London. McGraw–Hill, Vol. 5, p. 216–17.) Google Scholar
Weller, G.E. Schwerddcger, P. 1970 Thermal properties and heat transfer processes of the snow of the central Antarctic plateau. Union Géodésique et Géophysique Internationale. Association Internationale d’Hydrologie Scientifique.] [International Council of Scientific Unions. Scientific Committee on Antarctic Research. International Association of Scientific Hydrology, Commission of Snow and Ice] International Symposium on Antarctic Glaciological Exploration (ISAGE), Hanover,. New Hampshire U.S.A., 3–7 September 1968, p. 284–98. Google Scholar
Figure 0

Table I. Tiif.rmal propiïkîiks of tcf.