Hostname: page-component-cd9895bd7-7cvxr Total loading time: 0 Render date: 2024-12-20T05:15:51.845Z Has data issue: false hasContentIssue false
  • English
  • Français

Modeling nanoindentation using the Material Point Method

Published online by Cambridge University Press:  25 May 2018

Chad C. Hammerquist  and
John A. Nairn Open the ORCID record for John A. Nairn [Opens in a new window]
Chad C. Hammerquist
Affiliation:
Wood Science and Engineering, Oregon State University, Corvallis, Oregon 97330, USA
John A. Nairn*
Affiliation:
Wood Science and Engineering, Oregon State University, Corvallis, Oregon 97330, USA
*
a)Address all correspondence to this author. e-mail: john.nairn@oregonstate.edu
Get access

Abstract

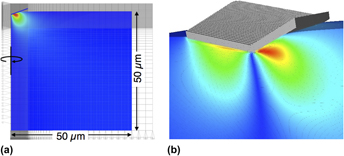
A numerical nanoindentation model was developed using the Material Point Method (MPM), which was chosen because it can handle both large deformations and dynamic contact under the indenter. Because of the importance of contact, prior MPM contact methods were enhanced to improve their accuracy for contact detection. Axisymmetric and full 3D simulations investigated the effects of hardening, strain-rate dependent yield properties, and local structure under the indenter. Convergence of load–displacement curves required small cells under the indenter. To reduce computation time, we used an effective nonregular grid, called a tartan grid and describe its implementation. Tartan grids reduced simulation times by an order of magnitude. A series of simulated load–displacement curves were analyzed as “virtual experiments” by standard Oliver–Pharr methods to extract effective modulus and hardness of the indented material. We found that standard analysis methods give results that are affected by hardening parameters and strain-rate dependence of plasticity. Because these parameters are not known during experiments, extracted properties will always have limited accuracy. We describe an approach for extracting more properties and more accurate properties by combining MPM simulations with inverse methods to fit simulation results to entire load–displacement curves.

Keywords

nanoindentationsimulationhardness
Type
Article
Information
Journal of Materials Research , Volume 33 , Issue 10 , 28 May 2018 , pp. 1369 - 1381
Copyright
Copyright © Materials Research Society 2018 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Oliver, W.C. and Pharr, G.M.: An improved technique for determining hardness and elastic modulus using load and displacement sensing indentation experiments. J. Mater. Res. 7, 1564–1583 (1992).CrossRefGoogle Scholar
Poon, B., Rittel, D., and Ravichandran, G.: An analysis of nanoindentation in elasto-plastic solids. Int. J. Solid Struct. 45, 6399–6415 (2008).Google Scholar
Poon, B., Rittel, D., and Ravichandran, G.: An analysis of nanoindentation in linearly elastic solids. Int. J. Solid Struct. 45, 6018–6033 (2008).Google Scholar
Chen, X., Ashcroft, I., Tuck, C., He, Y., Hague, R., and Wildman, R.: An investigation into the depth and time dependent behavior of UV cured 3D ink jet printed objects. J. Mater. Res. 32, 1407–1420 (2017).Google Scholar
Nardi, T., Hammerquist, C., Nairn, J.A., Karimi, A., Månson, J-A.E., and Leterrier, Y.: Nanoindentation of functionally graded polymer nanocomposites: Assessment of the strengthening parameters through experiments and modeling. Front. Mater. 2, 57 (2015).CrossRefGoogle Scholar
Ma, J., Liu, Y., Lu, H., and Komanduri, R.: Multiscale simulation of nanoindentation using the generalized interpolation material point (GIMP) method, dislocation dynamics (DD) and molecular dynamics (MD). Comput. Model. Eng. Sci. 16, 41–55 (2006).Google Scholar
Bardenhagen, S., Guilkey, J.E., Roessig, K., Brackbill, J., Witzel, W., and Foster, J.: An improved contact algorithm for the material point method and application to stress propagation in granular material. Comput. Model. Eng. Sci. 2, 509–522 (2001).Google Scholar
Nairn, J.A.: Modeling imperfect interfaces in the material point method using multimaterial methods. Comput. Model. Eng. Sci. 1, 1–15 (2013).Google Scholar
Nairn, J.A., Bardenhagen, S.G., and Smith, G.S.: Generalized contact and improved frictional heating in the material point method. Comput. Part. Mech. (2017). doi: 10.1007/s40571-017-0168-1.Google Scholar
Bardenhagen, S.G. and Kober, E.M.: The generalized interpolation material point method. Comput. Model. Eng. Sci. 5, 477–496 (2004).Google Scholar
Sulsky, D., Chen, Z., and Schreyer, H.L.: A particle method for history-dependent materials. Comput. Meth. Appl. Mech. Eng. 118, 179–186 (1994).CrossRefGoogle Scholar
Zhang, X., Chen, Z., and Liu, Y.: The Material Point Method: A Continuum-Based Particle Method for Extreme Loading Cases (Academic Press, Oxford, U.K., 2016).Google Scholar
Nairn, J.A.: Numerical simulations of transverse compression and densification in wood. Wood Fiber Sci. 38, 576–591 (2007).Google Scholar
Perré, P., Almeida, G., Ayouz, M., and Frank, X.: New modelling approaches to predict wood properties from its cellular structure: Image-based representation and meshless methods. Ann. For. Sci. 73, 147–162 (2016).CrossRefGoogle Scholar
Nairn, J.A.: Material point method simulations of transverse fracture in wood with realistic morphologies. Holzforschung 61, 375–381 (2007).Google Scholar
Guilkey, J.E., Hoying, J.B., and Weiss, J.A.: Computational modeling of multicellular constructs with the material point method. J. Biomech. 39, 2074–2086 (2006).Google Scholar
Ganpule, S., Daphalapurkar, N.P., Ramesh, K.T., Knutsen, A.K., Pham, D.L., Bayly, P.V., and Prince, J.L.: A three-dimensional computational human head model that captures live human brain dynamics. J. Neurotrauma 34, 2154–2166 (2017).Google Scholar
Daphalapurkar, N.P., Hanan, J.C., Phelps, N.B., Bale, H., and Lu, H.: Tomography and simulation of microstructure evolution of a closed-cell polymer foam in compression. Mech. Adv. Mater. Struct. 15, 594–611 (2008).CrossRefGoogle Scholar
Stomakhin, A., Schroeder, C., Chai, L., Teran, J., and Selle, A.: A material point method for snow simulation. ACM Trans. Graph. 32, 1–102 (2013).Google Scholar
Bardenhagen, S., Brackbill, J., and Sulsky, D.: The material-point method for granular materials. Comput. Meth. Appl. Mech. Eng. 187, 529–541 (2000).Google Scholar
Wang, Y., Beom, H., Sun, M., and Lin, S.: Numerical simulation of explosive welding using the material point method. Int. J. Impact Eng. 38, 51–60 (2011).Google Scholar
Ma, S. and Zhang, X.: Material point method for impact and explosion problems. In Proceedings of International Symposium on Computational Mechanics, Yao, Z., Yuan, M., eds. (Springer, Beijing, China, 2007); pp. 156–166.Google Scholar
Nairn, J.A.: Material point method calculations with explicit cracks. Comput. Model. Eng. Sci. 4, 649–664 (2003).Google Scholar
Bardenhagen, S.G., Nairn, J.A., and Lu, H.: Simulation of dynamic fracture with the material point method using a mixed J-integral and cohesive law approach. Int. J. Fract. 170, 49–66 (2011).Google Scholar
Ambati, R., Pan, X., Yuan, H., and Zhang, X.: Application of material point methods for cutting process simulations. Comput. Mater. Sci. 57, 102–110 (2012).Google Scholar
Nairn, J.A.: Numerical simulation of orthogonal cutting using the material point method. Eng. Fract. Mech. 149, 262–275 (2015).Google Scholar
Hu, P.G., Xue, L., Mao, S., Kamakoti, R., Zhao, H., Dittakavi, N., Wang, Z., Li, Q., Ni, K., and Brenner, M.: Material point method applied to fluid-structure interaction (FSI)/aeroelasticity problems. In 48th AIAA Aerospace Sciences Meeting Including the New Horizons Forum and Aerospace Exposition (Orlando, Florida, 2010); pp. 4–7.Google Scholar
Nairn, J.A.: Material point method (NairnMPM) and finite element analysis (NairnFEA) open-source software (2017). Available at: http://osupdocs.forestry.oregonstate.edu/index.php/Main_Page (accessed March 16, 2018).Google Scholar
Nairn, J.A. and Guilkey, J.E.: Axisymmetric form of the generalized interpolation material point method. Int. J. Numer. Meth. Eng. 101, 127–147 (2015).Google Scholar
Oliver, W. and Pharr, G.: Measurement of hardness and elastic modulus by instrumented indentation: Advances in understanding and refinements to methodology. J. Mater. Res. 19, 3–20 (2004).CrossRefGoogle Scholar
Clausner, A. and Richter, F.: Usage of the concept of the effectively shaped indenter for the determination of yield stress from berkovich nano-indentation experiments. Eur. J. Mech. Solid. 53, 294–302 (2015).Google Scholar
Bower, A.: Applied Mechanics of Solids (CRC Press, Boca Raton, Florida, 2009). ISBN: 9781439802489.Google Scholar
Chudoba, T. and Richter, F.: Investigation of creep behaviour under load during indentation experiments and its influence on hardness and modulus results. Surf. Coat. Technol. 148, 191–198 (2001).Google Scholar
Jakes, J.E., Frihart, C.R., Beecher, J.F., Moon, R.J., and Stone, D.: Experimental method to account for structural compliance in nanoindentation measurements. J. Mater. Res. 23, 1113–1127 (2008).Google Scholar
VanLandingham, M.R., Villarrubia, J.S., Guthrie, W.F., and Meyers, G.F.: Nanoindentation of polymers: An overview. In Macromolecular Symposia, Vol. 167 (Wiley-Blackwell, Hoboken, New Jersey, 2001); pp. 15–44.Google Scholar
Johnson, G.R. and Cook, W.H.: A constitutive model and data for metals subjected to large strains, high strain rates and high. In Proceedings of the 7th International Symposium on Ballistics (The Hague, The Netherlands, 1983); pp. 541–547.Google Scholar
Lemiale, V., Hurmane, A., and Nairn, J.A.: Material point method simulation of equal channel angular pressing involving large plastic strain and contact through sharp corners. Comput. Model. Eng. Sci. 70, 41–66 (2010).Google Scholar
Sadeghirad, A., Brannon, R.M., and Burghardt, J.: A convected particle domain interpolation technique to extend applicability of the material point method for problems involving massive deformations. Int. J. Numer. Meth. Eng. 86, 1435–1456 (2011).CrossRefGoogle Scholar
Hammerquist, C.C. and Nairn, J.A.: A new method for material point method particle updates that reduces noise and enhances stability. Comput. Meth. Appl. Mech. Eng. 318, 724–738 (2017).CrossRefGoogle Scholar
Mast, C.M., Arduino, P., Miller, G.R., and Mackenzie-Helnwein, P.: Avalanche and landslide simulation using the material point method: Flow dynamics and force interaction with structures. Comput. Geosci. 18, 817–830 (2014).CrossRefGoogle Scholar
Mao, S., Chen, Q., Li, D., and Feng, Z.: Modeling of free surface flows using improved material point method and dynamic adaptive mesh refinement. J. Eng. Mech. 142, 04015069 (2016).Google Scholar
Ma, J.: Multiscale simulation using the generalized interpolation material point method, discrete dislocations and molecular dynamics. Ph.D. thesis, Oklahoma State University, Stillwater, Oklahoma, 2006.Google Scholar
Larsson, P-L., Giannakopoulos, A., Söderlund, E., Rowcliffe, D., and Vestergaard, R.: Analysis of berkovich indentation. Int. J. Solid Struct. 33, 221–248 (1996).Google Scholar
R Core Team: R: A Language and Environment for Statistical Computing (R Foundation for Statistical Computing, Vienna, Austria, 2016). Available at: https://www.R-project.org/.Google Scholar
Troyon, M. and Lafaye, S.: About the importance of introducing a correction factor in the sneddon relationship for nanoindentation measurements. Philos. Mag. 86, 5299–5307 (2006).Google Scholar
Graf, J.: PID Control Fundamentals (Createspace Independent Publishing Platform, 2016). ISBN: 9781535358668.Google Scholar
Pharr, G.M. and Bolshakov, A.: Understanding nanoindentation unloading curves. J. Mater. Res. 17, 2660–2671 (2002).Google Scholar
Fu, G. and Cao, L.: On the effective indenter shape used in the analysis of nanoindentation unloading curves. J. Mater. Sci. 40, 2683–2684 (2005).Google Scholar
Chen, X., Ogasawara, N., Zhao, M., and Chiba, N.: On the uniqueness of measuring elastoplastic properties from indentation: The indistinguishable mystical materials. J. Mech. Phys. Solid. 55, 1618–1660 (2007).CrossRefGoogle Scholar
Supplementary material: PDF

Hammerquist and Nairn supplementary material

Hammerquist and Nairn supplementary material

Download Hammerquist and Nairn supplementary material(PDF)
PDF 711 KB