Hostname: page-component-78c5997874-xbtfd Total loading time: 0 Render date: 2024-11-18T14:16:30.117Z Has data issue: false hasContentIssue false
  • English
  • Français

Electron skin depth in low density plasmas

Published online by Cambridge University Press:  13 January 2020

F. E. M. Silveira Open the ORCID record for F. E. M. Silveira [Opens in a new window]  and
B. P. Siqueira
F. E. M. Silveira*
Affiliation:
Centro de Ciências Naturais e Humanas, Universidade Federal do ABC, Rua Santa Adélia, 166, Bairro Bangu, CEP 09210-170, Santo André, SP, Brazil
B. P. Siqueira
Affiliation:
Centro de Ciências Naturais e Humanas, Universidade Federal do ABC, Rua Santa Adélia, 166, Bairro Bangu, CEP 09210-170, Santo André, SP, Brazil
*
†Email address for correspondence: francisco.silveira@ufabc.edu.br
Get access Rights & Permissions [Opens in a new window]

Abstract

We rescale the generalized Ohm’s law and consider the limits that imply the electric field which moves with an ideal (inviscid and perfectly conducting) plasma be proportional to the time rate of change of the current density. Therefore, we show that those limits are satisfied by a sufficiently low electron number density, $n_{\text{e}}$. We also show that the electron–ion collision frequency, 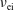$\unicode[STIX]{x1D708}_{\text{ei}}$, is much smaller than the ion cyclotron frequency, 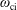$\unicode[STIX]{x1D714}_{\text{ci}}$. The combination of that condition with the Lawson criterion for a typical deuterium–tritium fusion in ITER reveals a lower bound for the geometric mean of the confinement time, 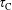$\unicode[STIX]{x1D70F}_{\text{C}}$, and collision interval, 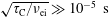$\sqrt{\unicode[STIX]{x1D70F}_{\text{C}}/\unicode[STIX]{x1D708}_{\text{ei}}}\gg 10^{-5}~\text{s}$. For that reaction, we estimate that 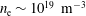$n_{\text{e}}\sim 10^{19}~\text{m}^{-3}$, and contrast typical parameters of our fully ionized gas with those of warm, hot and thermonuclear plasmas. When, in addition, $n_{\text{e}}$ varies slowly in time and weakly in space, we generalize Alfvén’s theorem, by showing that the frozen-in condition holds true for an effective magnetic field, which depends on a finite electron skin depth. We perform a (divergenceless) helical perturbation on an axisymmetric equilibrium, to derive a dispersion relation in the cylindrical tokamak limit, and, subsequently, apply our analytical formulation to the peaked model, which assumes a logarithmic derivative profile for the poloidal component of the equilibrium magnetic field. In that formulation, the definition of the safety factor in terms of the effective field yields a shift in the magnetic surfaces. We find that the instability peak may triple that predicted on neglect of a finite electron mass. We also find that inertial effects may centuple the radius of the stable cylindrical column.

Keywords

plasma instabilities
Type
Research Article
Information
Journal of Plasma Physics , Volume 86 , Issue 1 , February 2020 , 905860104
Copyright
© Cambridge University Press 2020

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Alfvén, H. 1942a On the existence of electromagnetic-hydrodynamic waves. Arkiv för matematik, astronomi och fysik 29, B1.Google Scholar
Alfvén, H. 1942b Existence of electromagnetic-hydrodynamic waves. Nature 150, 405.CrossRefGoogle Scholar
Bellan, P. M. 2000 Spheromaks: A Practical Application of Magnetohydrodynamic Dynamos and Plasma Self-organization. Imperial College Press.CrossRefGoogle Scholar
Bellan, P. M. 2012 Fundamentals of Plasma Physics. Cambridge University Press.Google Scholar
Bennett, A. 2006 Lagrangian Fluid Dynamics. Cambridge University Press.CrossRefGoogle Scholar
Bernstein, I. B., Frieman, E. A., Kruskal, M. D. & Kulsrud, R. M. 1958 An energy principle for hydromagnetic stability problems. Proc. R. Soc. Lond. A 244, 17.Google Scholar
Bittencourt, J. A. 2004 Fundamentals of Plasma Physics, 3rd edn.Springer.CrossRefGoogle Scholar
Bonanomi, N., Mantica, P., Szepesi, G., Hawkes, N., Lerche, E., Migliano, P., Peeters, A., Sozzi, C., Tsalas, M., Van Eester, D.& Jet Contributors 2015 Trapped electron mode driven electron heat transport in JET: experimental investigation and gyro-kinetic theory validation. Nucl. Fusion 55, 113016.CrossRefGoogle Scholar
Buneman, O. 1958 Instability, turbulence, and conductivity in current-carrying plasma. Phys. Rev. Lett. 1, 8.CrossRefGoogle Scholar
Buneman, O. 1963 Excitation of field aligned sound waves by electron streams. Phys. Rev. Lett. 10, 285.CrossRefGoogle Scholar
Bustos, A., Castejón, F., Fernández, L. A., García, J., Martin-Mayor, V., Reynolds, J. M., Seki, R. & Velasco, J. L. 2010 Impact of 3D features on ion collisional transport in ITER. Nucl. Fusion 50, 125007.CrossRefGoogle Scholar
Chatziantonaki, I., Tsironis, C. H., Isliker, H. & Vlahos, L. 2013 Self-consistent modeling of the dynamic evolution of magnetic island growth in the presence of stabilizing electron-cyclotron current drive. Plasma Phys. Control. Fusion 55, 115012.CrossRefGoogle Scholar
Cohen, R. S., Spitzer, L. & Routly, P. M. C. R. 1950 The electrical conductivity of an ionized gas. Phys. Rev. 80, 230.CrossRefGoogle Scholar
Ďuran, I., Entler, S., Grover, O., Bolshakova, I., Výborný, K., Kolčan, M., Jirman, T., Vayakis, G., Vasyliev, O., Radishevskyi, M. et al. 2019 Status of steady-state magnetic diagnostic for ITER and outlook for possible materials of Hall sensors for DEMO. Fusion Engng Des. 146, 2397.CrossRefGoogle Scholar
Farley, D. T. 1963 Two-stream plasma instability as a source of irregularities in the ionosphere. Phys. Rev. Lett. 10, 279.CrossRefGoogle Scholar
Freidberg, J. P. 2014 Ideal MHD. Cambridge University Press.CrossRefGoogle Scholar
Furth, H. P., Killeen, J. & Rosenbluth, M. N. 1963 Finite-resistivity instabilities of a sheet pinch. Phys. Fluids 6, 459.CrossRefGoogle Scholar
Furth, H. P., Rutherford, P. H. & Selberg, H. 1973 Tearing mode in the cylindrical tokamak. Phys. Fluids 16, 1054.CrossRefGoogle Scholar
Gambetta, G., Agostinetti, P., Sonato, P., Fedele, L., Bobbo, S. & Cabaleiro, D. 2019 Numerical analyses and tests for optimized and enhanced heat transfer solutions in DEMO. Fusion Engng Des. 146, 2692.CrossRefGoogle Scholar
Goedbloed, J. P. 1973 Generalization of Suydam’s criterion. Phys. Fluids 16, 1927.CrossRefGoogle Scholar
Gupta, S., Callen, J. D. & Hegna, C. C. 2002 Violating Suydam criterion produces feeble instabilities. Phys. Plasmas 9, 3395.CrossRefGoogle Scholar
Horton, W., Kim, J.-H., Asp, E., Hoang, T., Watanabe, T.-H. & Sugama, H. 2008 Drift wave turbulence. AIP Conf. Proc. 1013, 1.CrossRefGoogle Scholar
Horvath, A. & Rachlew, E. 2016 Nuclear power in the 21st century: challenges and possibilities. Ambio 45 (1), S38.CrossRefGoogle ScholarPubMed
ITER Physics Basis Editors et al. 1999a Chapter 1: overview and summary. Nucl. Fusion 39, 2137.CrossRefGoogle Scholar
ITER Physics Expert Group on Confinement and Transport et al. 1999b Chapter 2: plasma confinement and transport. Nucl. Fusion 39, 2175.CrossRefGoogle Scholar
Jorge, R., Ricci, P. & Loureiro, N. F. 2017 A drift-kinetic analytical model for scrape-off layer plasma dynamics at arbitrary collisionality. J. Plasma Phys. 83, 905830606.CrossRefGoogle Scholar
Jorge, R., Ricci, P. & Loureiro, N. F. 2018 Theory of the drift-wave instability at arbitrary collisionality. Phys. Rev. Lett. 121, 165001.CrossRefGoogle ScholarPubMed
Jorge, R., Ricci, P., Brunner, S., Gamba, S., Konovets, V., Loureiro, N. F., Perrone, L. M. & Teixeira, N. 2019 Linear theory of electron-plasma waves at arbitrary collisionality. J. Plasma Phys. 85, 905850211.CrossRefGoogle Scholar
Kadomtsev, B. 1966 Hydromagnetic stability of a plasma. Rev. Plasma Phys. 2, 153.Google Scholar
Klimontovich, Yu. L. 1967 The Statistical Theory of Non-equilibrium Processes in a Plasma. Pergamon.Google Scholar
Lawson, J. D.1955 Some criteria for a useful thermonuclear reaction. Tech. Rep., Atomic Energy Research Establishment, Harwell, Berkshire: UK.Google Scholar
Lawson, J. D. 1957 Some criteria for a power producing thermonuclear reactor. Proc. Phys. Soc. B 70, 6.CrossRefGoogle Scholar
Lundquist, S. 1952 Studies in magneto-hydrodynamics. Arkiv Mat. Astr. Fys. 5, 297.Google Scholar
Miyamoto, K. 2016 Plasma Physics for Controlled Fusion, 2nd edn.Springer.CrossRefGoogle Scholar
Moseev, D., Salewski, M., Garcia-Muñoz, M., Geiger, B. & Nocente, M. 2018 Recent progress in fast-ion diagnostics for magnetically confined plasmas. Rev. Mod. Plasma Phys. 2, 7.CrossRefGoogle Scholar
Nishikawa, K. & Wakatani, M. 2000 Plasma Physics: Basic Theory with Fusion Applications, 3rd edn.Springer.CrossRefGoogle Scholar
Ongena, J., Koch, R., Wolf, R. & Zohm, H. 2016 Magnetic-confinement fusion. Nat. Phys. 12, 398.CrossRefGoogle Scholar
Park, H. K. 2019 Newly uncovered physics of MHD instabilities using 2-D electron cyclotron emission imaging system in toroidal plasmas. Adv. Phys. X 4, 1633956.Google Scholar
Piel, A. 2017 Plasma Physics: An Introduction to Laboratory, Space, and Fusion Plasmas, 2nd edn.Springer.CrossRefGoogle Scholar
Pogutse, O. P. 1968 Magnetic drift instability in a collisionless plasma. Plasma Phys. 10, 649.CrossRefGoogle Scholar
Prǎvǎlie, R. & Bandoc, G. 2018 Nuclear energy: between global electricity demand, worldwide decarbonisation imperativeness, and planetary environmental implications. J. Environ. Manage. 209, 81.Google ScholarPubMed
Pushkarev, A., Bos, W. J. T. & Nazarenko, S. 2013 Zonal flow generation and its feedback on turbulence production in drift wave turbulence. Phys. Plasmas 20, 042304.CrossRefGoogle Scholar
Richardson, A. S. 2019 Naval Research Laboratory Plasma Formulary. The Office of Naval Research.Google Scholar
Rutherford, P. H. 1968 Drift instabilities in general magnetic field configurations. Phys. Fluids 11, 569.CrossRefGoogle Scholar
Silveira, F. E. M. & Orlandi, H. I. 2016 Rayleigh–Taylor instability with finite current relaxation. Phys. Plasmas 23, 042111.CrossRefGoogle Scholar
Silveira, F. E. M. & Orlandi, H. I. 2017 Viscous-resistive layer in Rayleigh–Taylor instability. Phys. Plasmas 24, 032112.CrossRefGoogle Scholar
Somov, B. V. 2013 Plasma Astrophysics, Part I: Fundamentals and Practice, 2nd edn.Springer.CrossRefGoogle Scholar
Spitzer, L. 1958 The stellarator concept. Phys. Fluids 1, 253.CrossRefGoogle Scholar
Spitzer, L. 2006 Physics of Fully Ionized Gases, 2nd edn.Dover.Google Scholar
Spitzer, L. & Härm, R. 1953 Transport phenomena in a completely ionized gas. Phys. Rev. 89, 977.CrossRefGoogle Scholar
Strauss, H., Joffrin, E., Riccardo, V., Breslau, J., Paccagnella, R.& Jet Contributors 2017 Comparison of JET AVDE disruption data with M3D simulations and implications for ITER. Phys. Plasmas 24, 102512.CrossRefGoogle Scholar
Suydam, B. R. 1958 Stability of a linear pinch. In Proceedings of the Second International Conference on the Peaceful Uses of Atomic Energy, vol. 31, p. 157. United Nations.Google Scholar
Sykes, A., Akers, R., Appel, L., Carolan, P. G., Conway, N. J., Cox, M., Field, A. R., Gates, D. A., Gee, S., Gryaznevich, M. et al. 1997 High-$\unicode[STIX]{x1D6FD}$ performance of the START spherical tokamak. Plasma Phys. Control. Fusion 39, B247.CrossRefGoogle Scholar
Wagner, F. 2013 Physics of magnetic confinement fusion. EPJ Web Conf. 54, 01007.CrossRefGoogle Scholar
Webb, G. 2018 Manetohydrodynamics and Fluid Dynamics: Action Principles and Conservation Laws. Springer.CrossRefGoogle Scholar
Wesson, J. 2004 Tokamaks, 3rd edn.Oxford Science Publications.Google Scholar
White, R. B. 2014 The Theory of Toroidally Confined Plasmas, 3rd edn.Imperial College Press.CrossRefGoogle Scholar
Zhao, L. & Diamond, P. H. 2012 Collisionless inter-species energy transfer and turbulent heating in driftwave turbulence. Phys. Plasmas 19, 082309.CrossRefGoogle Scholar