Hostname: page-component-78c5997874-v9fdk Total loading time: 0 Render date: 2024-11-17T04:20:19.071Z Has data issue: false hasContentIssue false
  • English
  • Français

On the accuracy of the binary-collision algorithm in particle-in-cell simulations of magnetically confined fusion plasmas

Part of: Focus on Fusion

Published online by Cambridge University Press:  19 April 2021

Timo P. Kiviniemi Open the ORCID record for Timo P. Kiviniemi [Opens in a new window] ,
Eero Hirvijoki Open the ORCID record for Eero Hirvijoki [Opens in a new window]  and
Antti J. Virtanen
Timo P. Kiviniemi*
Affiliation:
Department of Applied Physics, Aalto University, P.O. Box 11100, 00076Aalto, Finland
Eero Hirvijoki
Affiliation:
Department of Applied Physics, Aalto University, P.O. Box 11100, 00076Aalto, Finland
Antti J. Virtanen
Affiliation:
Department of Applied Physics, Aalto University, P.O. Box 11100, 00076Aalto, Finland
*
†Email address for correspondence: timo.kiviniemi@aalto.fi
Get access Rights & Permissions [Opens in a new window]

Abstract

Ideally, binary-collision algorithms conserve kinetic momentum and energy. In practice, the finite size of collision cells and the finite difference in the particle locations affect the conservation properties. In the present work, we investigate numerically how the accuracy of these algorithms is affected when the size of collision cells is large compared with gradient scale length of the background plasma, a parameter essential in full-$f$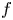 fusion plasma simulations. Additionally, we discuss implications for the conserved quantities in drift-kinetic formulations when fluctuating magnetic and electric fields are present: we suggest how the accuracy of the algorithms could potentially be improved with minor modifications.

Keywords

fusion plasmaplasma instabilitiesplasma simulation
Type
Research Article
Information
Journal of Plasma Physics , Volume 87 , Issue 2 , April 2021 , 905870219
Check for updates
Copyright
Copyright © The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Burby, J. W. & Brizard, A. J. 2019 Gauge-free electromagnetic gyrokinetic theory. Phys. Lett. A 383 (18), 2172–2175.CrossRefGoogle Scholar
Dimits, A. M., Wang, C., Caflisch, R., Cohen, B. I. & Huang, Y. 2009 Understanding the accuracy of Nanbu's numerical Coulomb collision operator. J. Comput. Phys. 228 (13), 4881–4892.CrossRefGoogle Scholar
Hager, R. & Chang, C. S. 2016 Gyrokinetic neoclassical study of the bootstrap current in the tokamak edge pedestal with fully non-linear Coulomb collisions. Phys. Plasmas 23 (4), 042503.CrossRefGoogle Scholar
Heikkinen, J. A., Korpilo, T., Janhunen, S. J., Kiviniemi, T. P., Leerink, S. & Ogando, F. 2012 Interpolat ion for momentum conservation in 3D toroidal gyrokinetic particle simulation of plasmas. Comput. Phys. Commun. 183 (8), 1719–1727.CrossRefGoogle Scholar
Hirvijoki, E., Burby, J. W., Pfefferlé, D. & Brizard, A. J. 2020 Energy and momentum conservation in the Euler-Poincaré formulation of local Vlasov-Maxwell-type systems. J. Phys. A: Math. Gen. 53 (23), 235204.CrossRefGoogle Scholar
Kiviniemi, T. P., Heikkinen, J. A. & Peeters, A. G. 2000 Test particle simulation of nonambipolar ion diffusion in tokamaks. Nucl. Fusion 40 (9), 1587–1596.CrossRefGoogle Scholar
Kiviniemi, T. P., Heikkinen, J. A. & Peeters, A. G. 2002 Neoclassical radial electric field and ion heat flux in the presence of the transport barrier. Contrib. Plasma Phys. 42 (2–4), 236–240.3.0.CO;2-X>CrossRefGoogle Scholar
Kiviniemi, T. P., Leerink, S., Niskala, P., Heikkinen, J. A., Korpilo, T. & Janhunen, S. 2014 Comparison of gyrokinetic simulations of parallel plasma conductivity with analytical models. Plasma Phys. Control. Fusion 56 (7), 075009.CrossRefGoogle Scholar
Korpilo, T., Gurchenko, A. D., Gusakov, E. Z., Heikkinen, J. A., Janhunen, S. J., Kiviniemi, T. P., Leerink, S., Niskala, P. & Perevalov, A. A. 2016 Gyrokinetic full-torus simulations of ohmic tokamak plasmas in circular limiter configuration. Comput. Phys. Commun. 203, 128–137.CrossRefGoogle Scholar
Nanbu, K. 1997 Theory of cumulative small-angle collisions in plasmas. Phys. Rev. E 55 (4), 4642–4652.CrossRefGoogle Scholar
Sauter, O., Angioni, C. & Lin-Liu, Y. R. 1999 Neoclassical conductivity and bootstrap current formulas for general axisymmetric equilibria and arbitrary collisionality regime. Phys. Plasmas 6 (7), 2834–2839.CrossRefGoogle Scholar
Takizuka, T. & Abe, H. 1977 A binary collision model for plasma simulation with a particle code. J. Comput. Phys. 25 (3), 205–219.Google Scholar
Wang, C., Lin, T., Caflisch, R., Cohen, B. I. & Dimits, A. M. 2008 Particle simulation of Coulomb collisions: comparing the methods of Takizuka & Abe and Nanbu. J. Comput. Phys. 227 (9), 4308–4329.CrossRefGoogle Scholar