Hostname: page-component-586b7cd67f-rcrh6 Total loading time: 0 Render date: 2024-11-23T03:04:31.905Z Has data issue: false hasContentIssue false
  • English
  • Français

Iterates of the core model

Published online by Cambridge University Press:  12 March 2014

Ralf Schindler
Ralf Schindler*
Affiliation:
Institut für Mathematische logik und Grundlagenforschung, Universität Münster, Einsteinstr. 62, 48149 Münster, Germany. E-mail: rds@math.uni-muenster.de, URL: http://wwwmath.uni-muenster.de/math/inst/logik/org/staff/rds
Get access Rights & Permissions [Opens in a new window]

Abstract

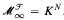Let N be a transitive model of ZFC such that “N ⊂ N and P(ℝ) ⊂ N. Assume that both V and N satisfy “the core model K exists.” Then KN is an iterate of K, i.e., there exists an iteration tree F on K such that F has successor length and . Moreover, if there exists an elementary embedding π: V → N then the iteration map associated to the main branch of F equals π յ K. (This answers a question of W. H. Woodin, M. Gitik, and others.) The hypothesis that P(ℝ) ⊂ N is not needed if there does not exist a transitive model of ZFC with infinitely many Woodin cardinals.

Keywords

set theorycore modelslarge cardinals
Type
Research Article
Information
The Journal of Symbolic Logic , Volume 71 , Issue 1 , March 2006 , pp. 241 - 251
Copyright
Copyright © Association for Symbolic Logic 2006

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Dodd, T., The core model, Lecture Note Series, no. 61, London Mathematical Society, Cambridge, 1982.CrossRefGoogle Scholar
[2]Jensen, R., The core model for non-overlapping extender sequences, handwritten notes, Oxford 1991.Google Scholar
[3]Jensen, R., On some problems of Mitchell, Welch, and Vickers, handwritten notes.Google Scholar
[4]Martin, T. and Steel, J., Iteration trees, Journal of American Mathematical Society, vol. 7 (1994). pp. 1–73.CrossRefGoogle Scholar
[5]Mitchell, W., Schimmerling, E., and Steel, J., The covering lemma up to a Woodin cardinal, Annals of Pure and Applied Logic, vol. 84 (1997), pp. 219–255.CrossRefGoogle Scholar
[6]Mitchell, W. and Steel, J., Fine structure and iteration trees, Lecture Notes in Logic, no. 3, Springer-Verlag, 1994.CrossRefGoogle Scholar
[7]Neeman, I., Inner models in the region of a Woodin limit of Woodin cardinals, Annals of Pure and Applied Logic, vol. 116 (2002), pp. 67–156.CrossRefGoogle Scholar
[8]Schindler, R.-D., The core model for almost linear iterations, Annals of Pure and Applied Logic, vol. 116 (2002), pp. 207–274.CrossRefGoogle Scholar
[9]Schindler, R.-D., Mutual stationarity in the core model, Logic Colloquium '01 (Baaz, M., Friedman, S., and Krajiček, J., editors), Lecture Notes in Logic, vol. 20, ASL and AK Peters, 2005, pp. 386–401.CrossRefGoogle Scholar
[10]Steel, J., The core model iterability problem, Lecture Notes in Logic, no. 8, Springer Verlag, 1996.CrossRefGoogle Scholar
[11]Steel, J., Core models with more Woodin cardinals, this Journal, vol. 67 (2002), pp. 1197–1226.Google Scholar