Hostname: page-component-586b7cd67f-tf8b9 Total loading time: 0 Render date: 2024-11-23T06:35:55.250Z Has data issue: false hasContentIssue false
  • English
  • Français

Pure denumerable Łukasiewiczian implication

Published online by Cambridge University Press:  12 March 2014

R. K. Meyer
R. K. Meyer*
Affiliation:
West Virginia University
Get access Rights & Permissions [Opens in a new window]

Extract

In [1], McCall and I offered an axiomatization of the implicational fragment of the 3-valued Łukasiewiczian system defined in [2]. The following axiom schemata sufficed, together with the rule of modus ponens.1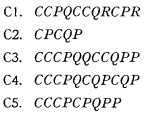

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 31 , Issue 4 , December 1966 , pp. 575 - 580
Copyright
Copyright © Association for Symbolic Logic 1997

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

[1]McCall, S. and Meyer, R. K., Pure three-valued Łukasiewiczian implication, to appear in this Journal, vol. 31 (1966), no. 2.Google Scholar
[2]Łukasiewicz, J. and Tarski, A., Untersuchungen über den Aussagenkalkul, Comptes Rendus des séances de la Société des Sciences et des Lettres de Varsovie, Classe III, vol. 23, 1930, pp. 1–21; English translation in A. Tarski, Logic, semantics, metamathematics, translated by J. H. Woodger, Oxford (Clarendon), 1956.Google Scholar
[3]Meredith, C. A., The dependence of an axiom of Łukasiewicz, Transactions of the American Mathematical Society 87 (1958), p. 54.Google Scholar
[4]Rose, Alan and Rosser, J. B., Fragments of many-valued statement calculi, Transactions of the American Mathematical Society, 87 (1958), pp. 1–53.CrossRefGoogle Scholar
[5]Chang, C. C., Proof of an axiom of Łukasiewicz, Transactions of the American Mathematical Society, 87 (1958), p. 55f.Google Scholar
[6]Turquette, Atwell R., Independent axioms for infinite-valued logic, this Journal, vol. 28 (1963), pp. 217–221.Google Scholar