Hostname: page-component-cd9895bd7-lnqnp Total loading time: 0 Render date: 2024-12-23T00:47:58.389Z Has data issue: false hasContentIssue false
  • English
  • Français

Combinatorial and recursive aspects of the automorphism group of the countable atomless Boolean algebra

Published online by Cambridge University Press:  12 March 2014

E. W. Madison  and
B. Zimmermann-Huisgen
E. W. Madison
Affiliation:
Division of Mathematical Sciences, University of Iowa, Iowa City, Iowa 52242
B. Zimmermann-Huisgen
Affiliation:
Division of Mathematical Sciences, University of Iowa, Iowa City, Iowa 52242
Get access Rights & Permissions [Opens in a new window]

Abstract

Given an admissible indexing φ of the countable atomless Boolean algebra ℬ, an automorphism F of ℬ is said to be recursively presented (relative to φ) if there exists a recursive function p ϵ Sym(ω) such that F ∘ φ = φ ∘ p. Our key result on recursiveness: Both the subset of Aut(ℬ) consisting of all those automorphisms which are recursively presented relative to some indexing, and its complement, the set of all “totally nonrecursive” automorphisms, are uncountable.

This arises as a consequence of the following combinatorial investigations: (1) A comparison of the cycle structures of ƒ and 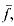, where ƒ is a permutation of some free basis of ℬ and 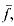is the automorphism of ℬ induced by ƒ.(2) An explicit description of the permutations of ω whose conjugacy classes in Sym(ω) are (a) uncountable, (b) countably infinite, and (c) finite.

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 51 , Issue 2 , June 1986 , pp. 292 - 301
Copyright
Copyright © Association for Symbolic Logic 1986

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1] Alton, D. A. and Madison, E. W., Computability of Boolean algebras and their extensions, Annals of Mathematical Logic, vol. 6 (1973), pp. 95–128.CrossRefGoogle Scholar
[2] Anderson, R. D., The algebraic simplicity of certain groups of homeomorphisms, American Journal of Mathematics, vol. 80 (1958), pp. 955–963.CrossRefGoogle Scholar
[3] Birkhoff, G. and Bartee, T. C., Modern applied algebra, McGraw-Hill, New York, 1966.Google Scholar
[4] Koppelberg, S., Homogeneous Boolean algebras may have nonsimple automorphism groups, Preprint No. 151, 05 1983.Google Scholar
[5] Madison, E. W., The existence of totally nonconstructive extensions of the countable atomless Boolean algebras, this Journal, vol. 48 (1983), pp. 167–170.Google Scholar
[6] Rabin, M. O., Computable algebras, Transactions of the American Mathematical Society, vol. 95 (1960), pp. 341–360.Google Scholar
[7] Rogers, H., Theory of recursive functions and effective computability, McGraw-Hill, New York, 1967.Google Scholar
[8] Schreier, J. and Ulam, S., Eine Bemerkung über die Gruppe der topologischen Abbildungen der Kreislinie aufsich selbst, Studia Mathematica, vol. 5 (1955), pp. 155–159.CrossRefGoogle Scholar
[9] Sikorski, R., Boolean algebras, 3rd ed., Springer-Verlag, Berlin, 1969.CrossRefGoogle Scholar