Published online by Cambridge University Press: 09 April 2009
Completely simple semigroups form a variety, 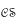 , of algebras with the operations of multiplication and inversion. It is known that the mapping
, of algebras with the operations of multiplication and inversion. It is known that the mapping 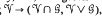 , where
, where 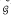 is the variety of all groups, is an isomorphism of the lattice
is the variety of all groups, is an isomorphism of the lattice 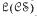 of all subvarieties of
of all subvarieties of 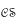 onto a subdirect product of the lattice of subvarieties of
onto a subdirect product of the lattice of subvarieties of 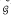 and the interval
and the interval 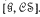 . We consider embeddings of
. We consider embeddings of 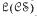 into certain direct products on the above pattern with rectangular bands, rectangular groups and central completely simple semigroups in place of groups.
into certain direct products on the above pattern with rectangular bands, rectangular groups and central completely simple semigroups in place of groups.