The rectangle ABCD is supposed to lie in a vertical plane, with AB horizontal. P is any point in the horizontal plane through CD. Prove that ∠APB < ∠CPD.

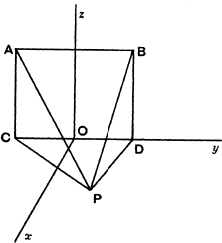
Provided that the perpendicular from P on to CD meets CD between C and D, this theorem is true and easily proved by comparing the angles into which a plane through P perpendicular to the rectangle divides the two angles considered. The sine of each of the angles into which ∠APB is divided is less than the sine of the corresponding angle in ∠CPD.