No CrossRef data available.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
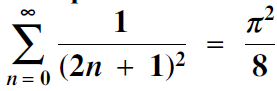$\sum\limits_{n = 0}^\infty {{1 \over {{{(2n + 1)}^2}}} = {{{\pi ^2}} \over 8}} $Published online by Cambridge University Press: 24 February 2022
Euler’s striking proof of the fact that the infinite sum
continues to charm all who become acquainted with it as well as to motivate the search for new proofs. With the introduction of the zeta function 
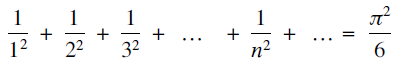${1 \over {{1^2}}}\, + \,{1 \over {{2^2}}}\, + \,{1 \over {{3^2}}} + \,...\, + \,{1 \over {{n^2}}} + \,...\, = {{{\pi ^2}} \over 6}$
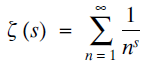$\zeta (s)\zeta = \sum\limits_{n = 1}^\infty {{1 \over {{n^s}}}} $
, the above sum is rewritten as 
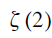$\zeta (2)$
and the problem of its evaluation in closed form is often named after Basel - the city of Euler’s birth. Many solutions to the Basel problem exist today, with several original ones appearing on the pages of The Mathematical Gazette. The purpose of this Note is to present two short proofs, the first of which is a simplification of one in the literature and the second is believed to be new, of the equivalent fact that
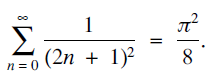$\sum\limits_{n = 0}^\infty {{1 \over {{{(2n + 1)}^2}}} = {{{\pi ^2}} \over 8}} .$