Published online by Cambridge University Press: 22 June 2010
We obtain a number of new bounds for exponential sums of the type S(χ, f) = ∑x = 1p−1 χ(x) ep(f(x)), with p a prime, f(x) = ∑i = 1raixki, ai, ki ∈ ℤ, 1 ≤ i ≤ r and χ a multiplicative character (mod p). The bounds refine earlier Mordell-type estimates and are particularly effective for polynomials in which a certain number of the ki have a large gcd with p − 1. For instance, if f(x) = ∑i = 1maixki + g(xd) with d|(p − 1) then 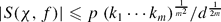 . If f(x) = axk + h(xd) with d|(p − 1) and (k, p − 1) = 1 then
. If f(x) = axk + h(xd) with d|(p − 1) and (k, p − 1) = 1 then  , and if f(x) = axk + bx−k + h(xd) with d|(p − 1) and (k, p − 1) = 1 then
, and if f(x) = axk + bx−k + h(xd) with d|(p − 1) and (k, p − 1) = 1 then 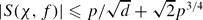 .
.