Hostname: page-component-586b7cd67f-r5fsc Total loading time: 0 Render date: 2024-11-29T22:00:58.103Z Has data issue: false hasContentIssue false
  • English
  • Français

The Cauchy property of the generalised Perron intergrals

Published online by Cambridge University Press:  24 October 2008

M. E. Grimshaw
M. E. Grimshaw
Affiliation:
Newnham College
Get access Rights & Permissions [Opens in a new window]

Extract

1. Saks calls an integral complete if it satisfies two conditions, of which one, the condition (C), is as follows.

If f(x) is defined in (a, b) and is integrable in each interval (a + ε, b − η), where a < a + ε < b − η < b, and

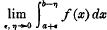
exists, then f(x) is integrable in (a, b) and the integral over (a, b) is equal to the above limit.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 30 , Issue 1 , January 1934 , pp. 15 - 18
Copyright
Copyright © Cambridge Philosophical Society 1934

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

* Saks, S., Fundamenta Math. 15 (1930), 242–262 (251).CrossRefGoogle Scholar

† Alexandroff, P., Math. Zeitschrift, 20 (1924), 213–222.CrossRefGoogle Scholar

‡ Burkill, J. C., Math. Zeitschrift, 34 (1931), 270–278CrossRefGoogle Scholar; Proc. London Math. Soc. (2), 34 (1932), 314–322.Google Scholar

* Compare Burkill, J. C., Math. Zeitschrift 34 (1931), 277.Google Scholar