Published online by Cambridge University Press: 24 October 2008
If H(ξ, η) is a harmonic function which is defined and positive in η > 0, then there is a non-negative number D and a bounded non-decreasing function G(x) such that
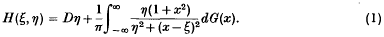
(For a proof, see Loomis and Widder, Duke Math. J. 9 (1942), 643–5.) If we write
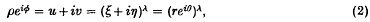
where λ > 1, then the equation
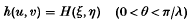
defines a harmonic function h which is positive in υ > 0. Hence there is a non-negative number d and a bounded non-decreasing function g(x) such that
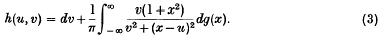
The problem of finding the connexion between the functions G(x) and g(x) has been mentioned by Loomis (Trans. American Math. Soc. 53 (1943), 239–50, 244).