‘On the global attractivity ina generalized delay-logistic differential equation’

In the proof of Theorem 3·1, the function space and its topology are incorrect, The correct function space and its topology with the related modifications are given below, which will replace the contents of the last line of page 188 and the first eight lines of page 189 of the above article.

Let B denote the real linear space of all continuous functions defined on [0, ∞)topologized by a separating family of seminorms Pn, where

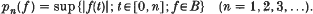
Since p1 ≤ p2 ≤ p3 ≤ …, the sets

form a convex local base for the topology of B. The topology generated by {Vn} is also compatible with that of the metric d, where

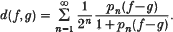
Thus B becomes a metrizable, Hausdorif, locally convex linear topological space. The strong version of the Tychonoff fixed-point theorem of S. A. Morris and E. S. Noussair (Mat. Čs. 25 (1975), 165–172) states ‘If T is a continuous mapping of a convex subset A of a Hausdorff locally convex linear topological space B into a compact subset of A then T has a fixed point’. In the following, the compactness of the image T(A) ⊂ A will follow from an application of the generalized version of Arzela's theorem found in J. R. Munkres (Topology, A first course, Prentice-Hall, 1975, p. 292). Note that the set A is not closed in B but is convex; we will show below that the image set T(A) is contained in A and the members of T(A) are pointwise bounded by (3·16) and pointwise equicontinuous, from which the sequential compactness of T(A) in the topology of compact convergence will follow by the generalized Arzela's theorem; since the real line is metrizable, such a sequential compactness implies sequential compactness in the compact-open topology of T(A); but the set T(A) is itself metrizable by d with a topology compatible with the compact-open topology. Thus the sequential compactness of T(A) in the compact-open topology implies the compactness of the set T(A) in the compact-open topology. Thus it is sufficient to show the pointwise boundedness and equicontinuity of elements of T(A).