Article contents
On the exponential sum 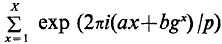
Published online by Cambridge University Press: 26 February 2010
Extract
In a conversation with Professor R. G. Stoneham, he mentioned the estimate which he had found for a new type of exponential sum, namely
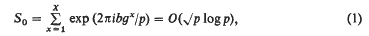
where p is a prime, g a primitive root mod p, pp × b, and l ≤ X ≤ p − 2. I found a proof and sent it to him. In correspondence with him and Professor D. A. Burgess,‡ I learnt that the estimate arose in Stoneham's work on normal numbers, in which they had been interested for some time. A proof of (1) will appear as a lemma in a paper by Stoneham [1] which has been submitted for publication. In fact, each of them has found several proofs of (1), and in particular, Burgess had found quite a simple one which is practically the same as my proof. However, my presentation led to an interesting generalization.
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © University College London 1972
References
- 3
- Cited by

