Published online by Cambridge University Press: 18 November 2020
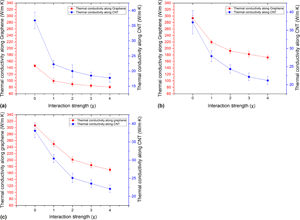
Thermal transport of pillared-graphene structure (PGS) supported on a copper substrate was investigated using equilibrium molecular dynamics. The results show that thermal conductivity along the graphene sheet in Cu-supported PGS ranges between 96.12 and 247.16 W/m K for systems with different dimensions at an interaction strength χ = 1. Thermal conductivity along carbon nanotube was found to range between 22.43 and 30.83 W/m K. The increase of interaction strength between Cu and carbon leads to a general decrease in thermal conductivity of PGS. The simulation results suggest that the thermal conductivity in Cu-supported PGS systems is governed by system geometry and phonon transport.