Hostname: page-component-cd9895bd7-gxg78 Total loading time: 0 Render date: 2024-12-22T01:32:03.386Z Has data issue: false hasContentIssue false
  • English
  • Français

CLUSTER CATEGORIES FROM GRASSMANNIANS AND ROOT COMBINATORICS

Part of: Algebraic combinatorics Lie algebras and Lie superalgebras Representation theory of rings and algebras

Published online by Cambridge University Press:  03 June 2019

KARIN BAUR Open the ORCID record for KARIN BAUR [Opens in a new window] ,
DUSKO BOGDANIC Open the ORCID record for DUSKO BOGDANIC [Opens in a new window]  and
ANA GARCIA ELSENER Open the ORCID record for ANA GARCIA ELSENER [Opens in a new window]
KARIN BAUR
Affiliation:
Karl-Franzens-Universitat Graz, Mathematics, Heinrichstraße 36, Graz 8010, Austria School of Mathematics, University of Leeds, Leeds, LS2 9JT, UK email ka.baur@me.com
DUSKO BOGDANIC
Affiliation:
Karl-Franzens-Universitat Graz, Mathematics, Heinrichstraße 36, Graz 8010, Austria email dusko.bogdanic@gmail.com
ANA GARCIA ELSENER
Affiliation:
Karl-Franzens-Universitat Graz, Mathematics, Heinrichstraße 36, Graz 8010, Austria email anaelsener@gmail.com
Get access Rights & Permissions [Opens in a new window]

Abstract

The category of Cohen–Macaulay modules of an algebra $B_{k,n}$ is used in Jensen et al. (A categorification of Grassmannian cluster algebras, Proc. Lond. Math. Soc. (3) 113(2) (2016), 185–212) to give an additive categorification of the cluster algebra structure on the homogeneous coordinate ring of the Grassmannian of 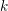$k$-planes in $n$-space. In this paper, we find canonical Auslander–Reiten sequences and study the Auslander–Reiten translation periodicity for this category. Furthermore, we give an explicit construction of Cohen–Macaulay modules of arbitrary rank. We then use our results to establish a correspondence between rigid indecomposable modules of rank 2 and real roots of degree 2 for the associated Kac–Moody algebra in the tame cases.

MSC classification

Primary: 05E10: Combinatorial aspects of representation theory 16G50: Cohen-Macaulay modules 17B22: Root systems
Type
Article
Information
Nagoya Mathematical Journal , Volume 240 , December 2020 , pp. 322 - 354
Check for updates
Copyright
© 2019 Foundation Nagoya Mathematical Journal

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Amiot, C., Cluster categories for algebras of global dimension 2 and quivers with potential, Ann. Inst. Fourier (Grenoble) 59(6) (2009), 2525–2590.10.5802/aif.2499Google Scholar
Assem, I., Simson, D. and Skowroński, A., Elements of the Representation Theory of Associative Algebras. Vol. 1, London Mathematical Society Student Texts 65, Cambridge University Press, Cambridge, 2006, Techniques of representation theory.10.1017/CBO9780511614309Google Scholar
Barot, M., Kussin, D. and Lenzing, H., The cluster category of a canonical algebra, Trans. Amer. Math. Soc. 362(8) (2010), 4313–4330.10.1090/S0002-9947-10-04998-6Google Scholar
Baur, K. and Bogdanic, D., Extensions between Cohen–Macaulay modules of Grassmannian cluster categories, J. Algebraic Combin. 4 (2016), 1–36.Google Scholar
Baur, K., King, A. D. and Marsh, R. J., Dimer models and cluster categories of Grassmannians, Proc. Lond. Math. Soc. (3) 113(2) (2016), 213–260.10.1112/plms/pdw029Google Scholar
Buchweitz, R. O., Maximal Cohen–Macaulay modules and Tate-cohomology over Gorenstein rings, University of Hannover, 1986. https://tspace.library.utoronto.ca/bitstream/1807/16682/1/maximal_cohen-macaulay_modules_1986.pdf.Google Scholar
Demonet, L. and Luo, X., Ice quivers with potential associated with triangulations and Cohen–Macaulay modules over orders, Trans. Amer. Math. Soc. 368(6) (2016), 4257–4293.10.1090/tran/6463Google Scholar
Derksen, H. and Weyman, J., An Introduction to Quiver Representations, Graduate Studies in Mathematics 184, American Mathematical Society, Providence, RI, 2017.10.1090/gsm/184Google Scholar
Derksen, H., Weyman, J. and Zelevinsky, A., Quivers with potentials and their representations. I. Mutations, Selecta Math. (N.S.) 14(1) (2008), 59–119.10.1007/s00029-008-0057-9Google Scholar
Geiß, C. and González-Silva, R., Tubular Jacobian algebras, Algebr. Represent. Theory 18(1) (2015), 161–181.10.1007/s10468-014-9486-7Google Scholar
Geiß, C., Labardini-Fragoso, D. and Schröer, J., The representation type of Jacobian algebras, Adv. Math. 290 (2016), 364–452.10.1016/j.aim.2015.09.038Google Scholar
Geiß, C., Leclerc, B. and Schröer, J., Rigid modules over preprojective algebras, Invent. Math. 165(3) (2006), 589–632.10.1007/s00222-006-0507-yGoogle Scholar
Geiss, C., Leclerc, B. and Schröer, J., Partial flag varieties and preprojective algebras, Ann. Inst. Fourier (Grenoble) 58(3) (2008), 825–876.10.5802/aif.2371Google Scholar
Happel, D., Triangulated Categories in the Representation Theory of Finite-Dimensional Algebras, London Mathematical Society Lecture Note Series 119, Cambridge University Press, Cambridge, 1988.10.1017/CBO9780511629228Google Scholar
Jensen, B. T., King, A. D. and Su, X., A categorification of Grassmannian cluster algebras, Proc. Lond. Math. Soc. (3) 113(2) (2016), 185–212.10.1112/plms/pdw028Google Scholar
Kac, V. G., Infinite-Dimensional Lie Algebras, 3rd ed. Cambridge University Press, Cambridge, 1990.10.1017/CBO9780511626234Google Scholar
Keller, B., The periodicity conjecture for pairs of Dynkin diagrams, Ann. of Math. (2) 177(1) (2013), 111–170.10.4007/annals.2013.177.1.3Google Scholar
Keller, B. and Reiten, I., Cluster-tilted algebras are Gorenstein and stably Calabi-Yau, Adv. Math. 211(1) (2007), 123–151.10.1016/j.aim.2006.07.013Google Scholar
Knutson, A., https://plus.google.com/+AllenKnutson/posts/TWsWhCakCQg. Accessed: 2018-04-28.Google Scholar
Postnikov, A., Total positivity, Grassmannians, and networks, preprint, 2006, arXiv:math/0609764.Google Scholar
Reiten, I. and Van den Bergh, M., Noetherian hereditary abelian categories satisfying Serre duality, J. Amer. Math. Soc. 15(2) (2002), 295–366.10.1090/S0894-0347-02-00387-9Google Scholar
Ringel, C. M., Tame Algebras and Integral Quadratic Forms, Lecture Notes in Mathematics, 1099, Springer, Berlin, 1984.10.1007/BFb0072870Google Scholar
Scott, J. S., Grassmannians and cluster algebras, Proc. Lond. Math. Soc. (3) 92(2) (2006), 345–380.10.1112/S0024611505015571Google Scholar
Simson, D., Linear Representations of Partially Ordered Sets and Vector Space Categories, Vol. 4, Gordon and Breach Science Publishers, Brooklyn, NY, 1992, 499 pp.Google Scholar
Simson, D., “Cohen–Macaulay modules over classical orders”, in Interactions Between Ring Theory and Representations of Algebras (Murcia), Lecture Notes in Pure and Appl. Math. 210, Dekker, New York, 2000, 345–382.Google Scholar
Simson, D. and Skowroński, A., Elements of the Representation Theory of Associative Algebras, Vol. 3, London Mathematical Society Student Texts 72, Cambridge University Press, Cambridge, 2007, Representation-infinite tilted algebras.Google Scholar
Yoshino, Y., Cohen–Macaulay Modules over Cohen–Macaulay Rings, London Mathematical Society Lecture Note Series 146, Cambridge University Press, Cambridge, 1990.10.1017/CBO9780511600685Google Scholar
Zelevinsky, A., Private communication, Zürich, 2012.Google Scholar