Introduction
Botswana supports the largest population of African elephant Loxodonta africana in any country (Cumming & Jones, Reference Cumming and Jones2005), and numbers are apparently increasing (Gibson et al., Reference Gibson, Craig and Masogo1998; Spinage, Reference Spinage1990; Blanc et al., Reference Blanc, Thouless, Hart, Dublin, Douglas-Hamilton, Craig and Barnes2003; Cumming & Jones, Reference Cumming and Jones2005). This has generated concern about potential adverse effects on vegetation and on co-occurring species (Sommerlatte, Reference Sommerlatte1976; Colegrave et al., Reference Colegrave, Lungu and Muwezwa1992; Ben-Shahar, Reference Ben-Shahar1997; Skarpe et al., Reference Skarpe, Aarrestad, Andreassen, Dhillion, Dimakatso and Du Toit2004) and the likely increase in human conflict (Bengis, Reference Bengis1996). In such cases population management is often mooted as a precaution.
There is a general assumption that elephant numbers and impact are directly related (van Aarde et al., Reference Van Aarde, Jackson and Ferreira2006; van Aarde & Jackson, Reference Van Aarde and Jackson2007). This may not necessarily be the case because density and, more specifically, the intensity of land use, may dictate impact. For instance, elephants in areas with a high density of water sources have smaller home ranges than those in areas with a low density of water sources (Grainger et al., Reference Grainger, van Aarde and Whyte2005). In small home ranges elephants may use specific parts of their ranges more intensely than in large home ranges and therefore impact may be more intense. Thus, it may be more appropriate to define elephant impact in terms of range utilization functions or densities rather than population numbers per se. This is particularly important for open populations where movement is not restricted by fences. In such cases, elephant movements may complicate the interpretation of trends in population size because an increase in number may not equate to an increase in density if the population expands its range. The northern Botswana elephant population may represent such a case.
Growth rates based on time series data may serve as a first estimate of population trends but the interpretation of these trends may be constrained by methodological aspects. For instance, an expansion of survey area over time could return an increase in number while density remains the same. Differences in the rates of change in numbers and densities may have different management implications and it is therefore important to address temporal trends in both.
Here we collated information on elephant population estimates and survey areas for northern Botswana, from which we calculated densities and intrinsic growth rates. We compare changes in these parameters over 1973–2004 to clarify temporal trends. Identification of any trends may guide future management actions to control the assumed impact that elephants may have on other species and on the livelihoods of people that live in areas onto which elephants are apparently expanding (Chafota & Owen-Smith, Reference Chafota and Owen-Smith1996).
Methods
As most of Botswana's elephants occur in the northern parts of the country (Gibson et al., Reference Gibson, Craig and Masogo1998), we extracted population estimates and survey details for elephants in northern Botswana from published (Melton, Reference Melton1985; Gibson et al., Reference Gibson, Craig and Masogo1998) and unpublished (Sommerlatte, Reference Sommerlatte1976; DWNP, 1996, 1999a,b, 2001, 2002, 2003, 2004) sources. For all surveys, Method II of Jolly (Reference Jolly1969) provided population estimates from fixed-width transects of unequal size sampled without replacement. Surveys were conducted during both dry and wet seasons. We excluded a 1985 survey (Spinage, Reference Spinage1990) for which the methodology was unknown. We also omitted estimates based on partial surveys conducted in 1983, 1984 and 1995 (Gibson et al., Reference Gibson, Craig and Masogo1998) and the 2005 survey conducted by the Department of Wildlife and National Parks (DWNP). In each case the survey area was that area for which the authors estimated population size. We calculated crude density (Gaston et al., Reference Gaston, Blackburn and Gregory1999) as the number of elephants per km2 of survey area.
From 1996 to 2004 surveys were country-wide, used standardized methods, and covered areas of 425,694-578,364 km2. For these surveys the DWNP divided the population estimate by the total area covered represented by all transects, irrespective of whether elephants occurred there or not. Because much of the survey area stretched beyond the known elephant range in northern Botswana, we opted to calculate ecological densities (Gaston et al., Reference Gaston, Blackburn and Gregory1999) for each of these years by dividing the population estimate by the sum of transect areas along which elephants were counted. The 1994 survey covered all of Botswana but we excluded these data from our analysis because Gibson et al. (Reference Gibson, Craig and Masogo1998) did not provide information that could be used to calculate the area over which elephants were encountered.
Following our filtering, the database represented two time periods: the first (1973–1993) comprised population estimates and crude densities and the second (1996–2004) population estimates and ecological densities. We used least squares regression analysis to test whether the natural logarithm of population estimates (expressed as elephant numbers) and elephant densities increased with time during each of these periods. The slopes and variances yielded estimates of exponential growth and their variances (Caughley, Reference Caughley1977). To accommodate the variances of population estimates in our calculation of population growth rates, we used Monte Carlo simulations (Manly, Reference Manly1991). This allowed us to estimate growth rates and their variance alternatively. We randomly drew population sizes from normal distributions defined for each population estimate and then recalculated exponential growth as the slope of the linear regression. We repeated this to find 2,000 estimates of population growth from which we calculated variance (Legendre & Legendre, Reference Legendre and Legendre1998). From these we could define standard errors for both methods of estimating population growth rate. We also used regression analysis to examine temporal trends in survey areas during each of the time periods.
In our final analyses we fitted two models to the complete time series of population estimates. We fitted an equilibrium model (Boltzman sigmoidal model ![]() , where a = lower asymptote, b = equilibrium population size or density, v 50 = the population estimate halfway between the lower asymptote and equilibrium, and c = growth when population size or densities are near a), and a non-equilibrium model (exponential model,
, where a = lower asymptote, b = equilibrium population size or density, v 50 = the population estimate halfway between the lower asymptote and equilibrium, and c = growth when population size or densities are near a), and a non-equilibrium model (exponential model, ![]() , were a = population size at time zero and b = the growth rate) using GraphPad Prism v. 3 (GraphPad Software, San Diego, USA). We relied on the F-test in GraphPad Prism to choose the best model.
, were a = population size at time zero and b = the growth rate) using GraphPad Prism v. 3 (GraphPad Software, San Diego, USA). We relied on the F-test in GraphPad Prism to choose the best model.
Results
Differences in sampling procedures that affected density estimates required us to analyse the data for the two time periods separately. The first period included eight estimates for dry and wet seasons but not all estimates were for the same years. As we had only one wet season estimate for the second time period, we excluded this period from the analysis of wet season data (Table 1).
Table 1 Population estimates (with 95% confidence limits where available), size of the areas for which the estimates were extrapolated, and elephant densities (with 95% confidence limits) for both wet and dry seasons, and the source reference. All densities are rounded to the second decimal place.

1 Country-wide surveys; survey area is the entire area over which the survey was conducted.
2 Country-wide surveys; survey area is the area over which elephants were encountered.
Seasonal differences in estimates were not consistent (paired t-test t = 0.39, df = 7, P = 0.71). From 1973 to 1993 elephant numbers and densities were 8,542–79,033 and 0.34–0.98 km−2, respectively. From 1996 to 2004 elephant numbers were 100,538–151,000 and densities 0.73–1.06 km−2. Variances of population estimates for 1973–1993 differed for both the dry (Fmax = 168.55, df = 5, P <0.05) and wet seasons (Fmax = 8.76, df = 4, P <0.05). However, variances for population estimates over 1996–2004 were similar (Fmax = 1.61, df = 4, P = 0.15).
Population growth rates calculated by regression analysis from population estimates for 1973–1993 were 11.2 ± SE 0.53% and 9.6 ± SE 1.11% (Fig. 1a,b; Table 2) for the dry and wet seasons, respectively. Monte Carlo simulations predicted growth rates of 11.1 ± SE 0.51% during the dry and 9.5 ± SE 0.54% during the wet season (Table 2). In contrast, growth rates in elephant densities for the same time period did not differ significantly from zero (Fig. 1a,b; Table 2). Growth rates for population estimates and densities differed significantly (Fdry = 34.0, df = 1,6, Pdry <0.0001; Fwet = 60.52, df = 1,6, Pwet <0.0001).

Fig. 1 Linear regressions (with 95% confidence limits) of the natural logarithm of (a) dry season and (b) wet season elephant numbers (open squares) and densities (solid squares) for 1973–1993, and (c) dry season elephant numbers and densities for 1996–2004. The regression line of density for 1996–2004 had wide confidence limits and is not shown. The slopes of the linear regressions represent intrinsic annual growth rates (r). Solid and stippled regression lines indicate significant and non-significant slopes, respectively. Note the different scaling of the vertical axis.
Table 2 Linear regression analysis and Monte Carlo simulations used to calculate intrinsic growth rates (r), expressed as a percentage. The slopes of the regression lines represent r. Growth rates in elephant numbers and densities are calculated separately for wet and dry season and for 1973–1993 and 1996–2004. Both numbers and densities were loge transformed for the linear regression analyses. Significant regressions are in bold.

From 1996 to 2004 neither elephant numbers nor densities changed significantly (Fig. 1c; Table 2). Estimated population size averaged 120,292 ± SE 13,990 and mean elephant density was 0.91 ± SE 0.06 km−2.
From 1973 to 1993 the size of the survey area increased significantly over time during both the dry and wet seasons (Fdry = 15.10, df = 1,6, Pdry <0.01; Fwet = 205.30, df = 1,6, Pwet <0.0001; Fig. 2a,b). However, since 1996 the size of the area over which elephants were encountered during surveys (averaging 134,800 ± SE 9,513 km2) did not change significantly (F = 4.94, df = 1,4, P = 0.09; Fig. 2c). However, statistical power for this regression is relatively low (1-β = 0.37), resulting in an increased probability of making a Type 2 error, i.e. falsely accepting that the size of the area over which elephants were encountered during surveys did not change.

Fig. 2 Linear regressions (with 95% confidence limits) of (a) dry and (b) wet season survey area sizes for 1973–1993, and (c) dry season survey area sizes for 1996–2004. Solid and stippled regression lines indicate significant and non-significant slopes, respectively.
The time series combining dry season elephant numbers from both periods were best described by an equilibrium model (Boltzman sigmoidal; F = 4.50, df = 11, P <0.05, R 2 = 0.97; Fig. 3). This suggests that, as elephant numbers increased over time, population growth rate declined until it did not differ significantly from zero.
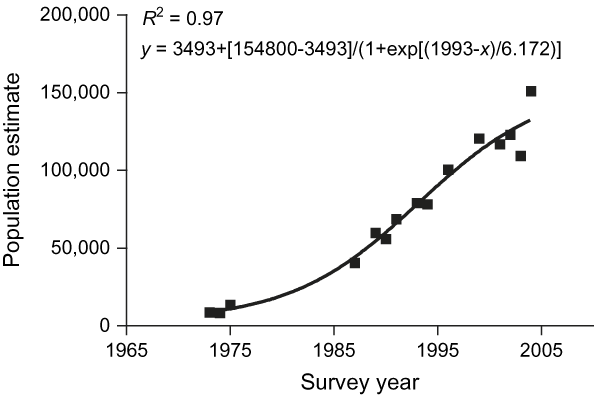
Fig. 3 Dry season elephant numbers for 1973–2004. The data converged best to a Boltzman sigmoidal curve (see text for details), suggesting that numbers are currently stabilizing.
Discussion
Between 200,000 and 400,000 elephants may have lived in Botswana at the beginning of the 19th century (Campbell, Reference Campbell, Hancock, Cantrell and Hughes1990), mostly in the north. In the 80 years that followed, uncontrolled commercial hunting for ivory exterminated elephants from southern Botswana and reduced their population to a mere remnant in the far north (Campbell, Reference Campbell, Hancock, Cantrell and Hughes1990). The reinvasion of the region by the tsetse fly, the subsequent collapse of the cattle population, and improved protection (Melton, Reference Melton1985) caused elephants to reappear along the Chobe River by the late 1940s (Sommerlatte, Reference Sommerlatte1976). Hearsay, suggesting that numbers increased, was supported by spoor and direct ground surveys carried out over 1963–1970 (Sommerlatte, Reference Sommerlatte1976; Campbell, Reference Campbell, Hancock, Cantrell and Hughes1990).
The first aerial counts in 1973–1975 were motivated by concerns that elephants may become overabundant in this region (Sommerlatte, Reference Sommerlatte1976). Since then, elephants in northern Botswana have been counted repeatedly, albeit at varying time intervals and survey intensities (Melton, Reference Melton1985; Gibson et al., Reference Gibson, Craig and Masogo1998, and sources therein, including KCS, 1984, 1985; Work, 1986; Gavor, 1987; Calef, Reference Calef1988, 1990; Craig, 1991, 1996; Bonifica, 1992; DWNP, 1993, 1995; ULG, 1993, 1994). However, survey methods were standardized in the mid 1990s (DWNP, 1996, 1999a,b, 2001, 2002, 2003, 2004).
The census data from 1973 to 1993 revealed a significant increase in elephant numbers in northern Botswana. During this period mean annual growth rate exceeded the maximum 7% estimated for elephants (Calef, Reference Calef1988). This may have been because of elephants dispersing from Zimbabwe, Zambia, Angola and Namibia (Campbell, Reference Campbell, Hancock, Cantrell and Hughes1990; Gibson et al., Reference Gibson, Craig and Masogo1998). In contrast, the growth rate for elephant densities during the same time did not differ from zero. How can this anomaly be explained?
A key constraint in the analysis of these temporal trends is that the surveys were carried out in areas that differ in size (surveyed areas increased from 1973 to 1993 but remained relatively constant afterwards). There are two possible explanations for the different trends in numbers and densities recorded before 1993. The first is that both the range of the population and the population size were stable over time and that we recorded an increase in numbers while densities remained constant; the initial surveys focused on only a fraction of the area in which elephants occur, and later survey areas increased until the entire range of the population was included (Fig. 4a). The second explanation is that both the range of the population and elephant numbers increased over time and surveys focused on those areas in which elephants were relatively abundant. Surveys thus covered larger areas over time in response to the expansion of elephant range and, as a result, more elephants were counted in larger areas, resulting in an increase in estimates of elephant numbers while densities remained relatively stable (Fig. 4b). We cannot unequivocally distinguish between the two explanations. However, given the historical accounts of the distribution of elephants in Botswana (Sommerlatte, Reference Sommerlatte1976; Campbell, Reference Campbell, Hancock, Cantrell and Hughes1990) it seems likely that this population increased and expanded its range from 1973 to 1993, i.e. in recovery following a precipitous decline.

Fig. 4 Simplified illustration of the two possible explanations for the trends in numbers and densities over time observed for the northern Botswana elephant population. Solid dots depict individuals and grey shading survey areas. The explanations differ in that the first (a) proposes a stable range and population size over time, whereas the second (b) suggests that the population increased in size and expanded its range over time (see text for further details).
Changes in surveyed areas do not constrain the trends recorded from 1996 to 2004 because the DWNP conducted country-wide surveys that included the entire range of Botswana's elephants. Elephant numbers for this period were therefore comparable between years, and neither the number of elephants nor densities changed significantly. This is in contrast to some earlier reports and deductions that implied a continuing increase of the northern Botswana population (Blanc et al., Reference Blanc, Thouless, Hart, Dublin, Douglas-Hamilton, Craig and Barnes2003, Reference Blanc, Barnes, Craig, Douglas-Hamilton, Dublin, Hart and Thouless2005; Cumming & Jones, Reference Cumming and Jones2005).
If the first explanation is correct, then the stabilization of numbers could be the result of surveys having reached the periphery of the range of the population. However, if the second explanation is correct then the onset of density-dependence (Sinclair, Reference Sinclair2003; Owen-Smith et al., Reference Owen-Smith, Kerley, Page, Slotow and van Aarde2006; Chamaillé-Jammes et al., Reference Chamaillé-Jammes, Valeix and Hervé2007) could be responsible for the apparent stabilization in numbers. The underlying mechanisms for any such stabilization are not yet clear but may result from density-dependent dispersal. Dispersal may also explain the abrupt increase in numbers from 2003 to 2004 (Fig. 3; Table 1). During this period surveys used standardized methods, yielding estimates with similar levels of precision. Therefore, the differences in population size may be the result of movements by elephants across national boundaries rather than variation in census error or population increase through reproduction. These matters need further investigation, most importantly by making use of synchronized counts across countries and population boundaries. Density-dependent stabilization, if it occurs, would be of particular importance for conservation management. For instance, should the levelling off in population size be induced by density, a reduction in numbers would merely be followed by an increase in growth rate.
Irrespective of which one of the two explanations is correct, it appears that elephant numbers in northern Botswana have begun to stabilize despite a high growth rate noted previously (Gibson et al., Reference Gibson, Craig and Masogo1998). Our results support this notion. An equilibrium model best described the trend in dry season elephant numbers over time, suggesting that population growth decreased with an increase in population size. Analyses of changes in elephant distribution and seasonal variability in densities calculated from survey data may identify areas where elephant impact and conflict is most intense. In addition, analyses that compare count-based growth rates and demographically derived growth rates may clarify the contribution of emigration and immigration to local population sizes.
Trends aside, the expansion of the elephant population into its traditional distributional range (Campbell, Reference Campbell, Hancock, Cantrell and Hughes1990; Gibson et al., Reference Gibson, Craig and Masogo1998), now inhabited by people, is a matter of concern because the livelihoods of people are influenced by the presence of elephants (Jackson et al., Reference Van Aarde and Jackson2008). However, the expansion of the range has the benefit of ameliorating impact on vegetation by allowing seasonal changes in habitat utilization through the restoration of traditional migratory patterns (van Aarde et al., Reference Van Aarde, Jackson and Ferreira2006), and also helps maintain metapopulation dynamics and caters for local instabilities (van Aarde & Jackson, Reference Van Aarde and Jackson2007). The regional management of landscapes and spatial utilization could therefore replace the need for the local management of numbers. The DWNP has expressed concern about the possible impact that elephants may have on biodiversity and included this as a criterion for management action in Botswana's Elephant Management Plan (DWNP, 1991, in Herremans, Reference Herremans1995). However, no culling of elephants has taken place in Botswana to date and the management plan is currently under review. Based on our recent satellite tracking studies and on the work of Verlinden & Gavor (Reference Verlinden and Gavor1998) we know that northern Botswana's elephants are part of a much larger regional population. Any efforts to reduce Botswana's elephants to ameliorate local impacts may therefore have regional effects on dispersal and hence on apparent local population trends, as has been illustrated for elephants in the Kruger National Park (van Aarde et al., Reference Van Aarde, Whyte and Pimm1999). This may nullify efforts to lower impact on local vegetation and other species.
Acknowledgements
We would like to thank the Department of Wildlife and National Parks, Botswana, for supporting our initiatives and for providing us with unpublished census data. Our research in Botswana is financially supported by the University of Pretoria, the Peace Parks Foundation, the International Fund for Animal Welfare, and the African Wilderness Programme of Conservation International.
Biographical sketches
Jessica Junker's research focuses on large mammal temporal population dynamics and their conservation. Rudi J. van Aarde, Director of the Conservation Ecology Research Unit (CERU), focuses on the restoration of populations and communities as a contribution to conservation. At present, CERU's research on elephants covers populations in Botswana, Malawi, Mozambique, Namibia, South Africa, Zambia and Zimbabwe. Sam M. Ferreira's research focuses on mammal and bird conservation biology with an emphasis on temporal dynamics and the factors influencing these. He also has a keen interest in restoration ecology and the application of theoretical ecology to address ecological problems. He currently coordinates aspects of the Elephant Programme at CERU.








