Hostname: page-component-84b7d79bbc-g78kv Total loading time: 0 Render date: 2024-07-31T04:29:17.832Z Has data issue: false hasContentIssue false
  • English
  • Français

An extension of a Hardy-Littlewood-Pólya inequality

Published online by Cambridge University Press:  20 January 2009

A. Erdélyi
A. Erdélyi
Affiliation:
Department of Mathematics, University of Edinburgh.
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

The Hardy-Littlewood-Pólya inequality in question can be written in the form

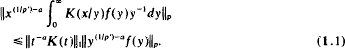
Here and throughout, all functions are assumed to be locally integrable on ]0,∞[, 1≤p≤∞,p-1+(p′)-1=1 (with similar conventions for q,r,s), is the usual norm on Lp(0,∞), and if the right hand side is finite, then (1.1) is understood to mean that

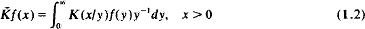
defines a locally integrable function Kf for which (1.1) holds.

Type
Research Article
Information
Proceedings of the Edinburgh Mathematical Society , Volume 21 , Issue 1 , March 1978 , pp. 11 - 15
Copyright
Copyright © Edinburgh Mathematical Society 1978

References

REFERENCES

(1) Erdélyi, A., On fractional integration and its application to the theory of Hankel transforms, Quart. J. Math. (Oxford) 11 (1940), 293–303.CrossRefGoogle Scholar
(2) Hardy, G. H., Littlewood, J. E., Pólya, G., Inequalities (Cambridge 1934).Google Scholar
(3) Kober, H., On fractional integrals and derivatives, Quart. J. Math. (Oxford) 11 (1940), 193–211.CrossRefGoogle Scholar
(4) Love, E. R., Two index laws for fractional integrals and derivatives, j. Austral. Math. Soc. 14 (1972), 385–410.CrossRefGoogle Scholar
(5) Love, E. R., A hypergeometric integral equation, Fractional Calculus and its Ap- plications (Springer Lecture Notes in Mathematics No. 457, 1975 ed. Ross, B.), 272–288.Google Scholar