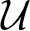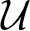1. Introduction
A linear operator on a Fréchet space X is said to be hypercyclic if there is a vector ![]() $x\in X$ such that for every non-empty open set
$x\in X$ such that for every non-empty open set ![]() $U\subset X$ the set
$U\subset X$ the set ![]() $N(x,U):=\{n\in\mathbb{N}:T^n x\in U\}$ is infinite, where
$N(x,U):=\{n\in\mathbb{N}:T^n x\in U\}$ is infinite, where ![]() $(T^n)$ is the sequence of iterates of T. In this situation, x is called a hypercyclic vector. Further there are more precise and stringent notions that allow to quantify how often a hypercyclic vector visits a non-empty open set. A linear operator on a Fréchet space X is said to be frequently hypercyclic (resp.
$(T^n)$ is the sequence of iterates of T. In this situation, x is called a hypercyclic vector. Further there are more precise and stringent notions that allow to quantify how often a hypercyclic vector visits a non-empty open set. A linear operator on a Fréchet space X is said to be frequently hypercyclic (resp. ![]() $\mathcal{U}$-frequently hypercyclic) if there is a vector
$\mathcal{U}$-frequently hypercyclic) if there is a vector ![]() $x\in X$ such that for every non-empty open set
$x\in X$ such that for every non-empty open set ![]() $U\subset X$ the set
$U\subset X$ the set ![]() $N(x,U)$ has positive lower (resp. upper) density, where the lower and upper densities of a subset
$N(x,U)$ has positive lower (resp. upper) density, where the lower and upper densities of a subset ![]() $A\subset \mathbb{N}$ are defined respectively as follows:
$A\subset \mathbb{N}$ are defined respectively as follows:
 \begin{equation*}\underline{d}(A)=\liminf_{n\rightarrow +\infty}\frac{\#A\cap\{1,\dots,n\}}{n}\ \hbox{ and }\
\overline{d}(A)=\limsup_{n\rightarrow +\infty}\frac{\#A\cap\{1,\dots,n\}}{n}.\end{equation*}
\begin{equation*}\underline{d}(A)=\liminf_{n\rightarrow +\infty}\frac{\#A\cap\{1,\dots,n\}}{n}\ \hbox{ and }\
\overline{d}(A)=\limsup_{n\rightarrow +\infty}\frac{\#A\cap\{1,\dots,n\}}{n}.\end{equation*} These notions were introduced by Bayart and Grivaux [Reference Bayart and Grivaux1] and Shkarin [Reference Shkarin26]. The dynamics of linear operators is a very active branch of research: we refer the reader to [Reference Bayart and Matheron2, Reference Grosse-Erdmann and Peris21] and the references therein for background in linear dynamics. Clearly a frequently hypercyclic vector is ![]() $\mathcal{U}$-frequently hypercyclic and a
$\mathcal{U}$-frequently hypercyclic and a ![]() $\mathcal{U}$-frequently hypercyclic vector is hypercyclic. Classical examples of frequently or
$\mathcal{U}$-frequently hypercyclic vector is hypercyclic. Classical examples of frequently or ![]() $\mathcal{U}$-frequently hypercyclic operators are given by suitable weighted shifts. As usual we denote by
$\mathcal{U}$-frequently hypercyclic operators are given by suitable weighted shifts. As usual we denote by ![]() $\mathbb{D}$ the open unit disc
$\mathbb{D}$ the open unit disc ![]() $\{z\in\mathbb{C}:\vert z\vert \lt 1\}$ of the complex plane and by
$\{z\in\mathbb{C}:\vert z\vert \lt 1\}$ of the complex plane and by ![]() $H(\mathbb{D})$ the set of analytic functions in
$H(\mathbb{D})$ the set of analytic functions in ![]() $\mathbb{D}$. It is well known that
$\mathbb{D}$. It is well known that ![]() $H(\mathbb{D})$ endowed with the topology of uniform convergence on compact subsets is a Fréchet space. For
$H(\mathbb{D})$ endowed with the topology of uniform convergence on compact subsets is a Fréchet space. For ![]() $\alpha\in\mathbb{R}$, let
$\alpha\in\mathbb{R}$, let ![]() $w(\alpha)=(w_n(\alpha))$ be the weighted sequence of non-zero complex numbers given by, for all
$w(\alpha)=(w_n(\alpha))$ be the weighted sequence of non-zero complex numbers given by, for all ![]() $n\geq 1$,
$n\geq 1$,
 \begin{equation*}w_n(\alpha)=\left(1+\frac{1}{n}\right)^{\alpha}.\end{equation*}
\begin{equation*}w_n(\alpha)=\left(1+\frac{1}{n}\right)^{\alpha}.\end{equation*}In the present paper, we consider the associated weighted Taylor shift:
 \begin{equation*}T_{\alpha}:H(\mathbb{D})\rightarrow H(\mathbb{D})\hbox{ given by }T_{\alpha}(\sum_{k\geq 0}a_k z^k)=\sum_{k\geq 0}a_{k+1}w_{k+1}(\alpha) z^k.\end{equation*}
\begin{equation*}T_{\alpha}:H(\mathbb{D})\rightarrow H(\mathbb{D})\hbox{ given by }T_{\alpha}(\sum_{k\geq 0}a_k z^k)=\sum_{k\geq 0}a_{k+1}w_{k+1}(\alpha) z^k.\end{equation*} For α = 0, T 0 is the classical Taylor shift operator. It is easy to check that for every real number α, Tα is a frequently hypercyclic operator. For instance, we refer the reader to [Reference Bernal-González4, Reference Grosse-Erdmann20, Reference Mouze and Munnier25]. The problem of determining possible rates of growth of frequently hypercyclic functions for Tα in terms of Lp averages was studied in [Reference Mouze and Munnier25] (see [Reference Mouze and Munnier24] for the case α = 0 too). For ![]() $0 \lt r \lt 1$ and
$0 \lt r \lt 1$ and ![]() $f\in H(\mathbb{D})$, we consider the classical integral means:
$f\in H(\mathbb{D})$, we consider the classical integral means:
 \begin{equation*}M_p(f,r)=\left(\frac{1}{2\pi}\int_{0}^{2\pi}\vert f(r\mathrm{e}^{\mathrm{i}\theta})\vert^{p}\,\mathrm{d}\theta\right)^{1/p} (1\leq p \lt \infty)\hbox{ and }
M_{\infty}(f,r)=\sup_{0\leq t\leq 2\pi}\vert f(r\mathrm{e}^{\mathrm{i}t})\vert.\end{equation*}
\begin{equation*}M_p(f,r)=\left(\frac{1}{2\pi}\int_{0}^{2\pi}\vert f(r\mathrm{e}^{\mathrm{i}\theta})\vert^{p}\,\mathrm{d}\theta\right)^{1/p} (1\leq p \lt \infty)\hbox{ and }
M_{\infty}(f,r)=\sup_{0\leq t\leq 2\pi}\vert f(r\mathrm{e}^{\mathrm{i}t})\vert.\end{equation*} In the same way, for any holomorphic polynomial P let us define, for ![]() $p\geq 1,$
$p\geq 1,$
 \begin{equation*}\Vert P\Vert_p=\left(\frac{1}{2\pi}\int_{0}^{2\pi}\vert P(\mathrm{e}^{\mathrm{i}\theta})\vert^{p}\,\mathrm{d}\theta\right)^{1/p}\hbox{ and }
\Vert P\Vert_{\infty}=\sup_{0\leq t\leq 2\pi}\vert P(\mathrm{e}^{\mathrm{i}t})\vert.\end{equation*}
\begin{equation*}\Vert P\Vert_p=\left(\frac{1}{2\pi}\int_{0}^{2\pi}\vert P(\mathrm{e}^{\mathrm{i}\theta})\vert^{p}\,\mathrm{d}\theta\right)^{1/p}\hbox{ and }
\Vert P\Vert_{\infty}=\sup_{0\leq t\leq 2\pi}\vert P(\mathrm{e}^{\mathrm{i}t})\vert.\end{equation*} In the following, for all p > 1, q will stand for the exponent conjugate to p, i.e.  $\frac{1}{p}+\frac{1}{q}=1$ and we will adopt the convention
$\frac{1}{p}+\frac{1}{q}=1$ and we will adopt the convention ![]() $q=\infty$ if p = 1. For
$q=\infty$ if p = 1. For ![]() $1\leq p\leq\infty$, the authors recently highlighted a critical exponent, i.e. a value of the parameter α from which the Lp-growth of a frequently hypercyclic function for Tα no longer has the same behaviour. In the case of frequent hypercyclicity for Tα, the critical exponent is equal to
$1\leq p\leq\infty$, the authors recently highlighted a critical exponent, i.e. a value of the parameter α from which the Lp-growth of a frequently hypercyclic function for Tα no longer has the same behaviour. In the case of frequent hypercyclicity for Tα, the critical exponent is equal to  $\alpha=\frac{1}{\max(2,q)}$. Indeed the authors obtained the following statements. First for p > 1 they proved the following result.
$\alpha=\frac{1}{\max(2,q)}$. Indeed the authors obtained the following statements. First for p > 1 they proved the following result.
Theorem 1.1. ([Reference Mouze and Munnier25, Theorem 1.2]) Let ![]() $\alpha\in\mathbb{R}.$ The following assertions hold
$\alpha\in\mathbb{R}.$ The following assertions hold
(1) For any
 $1 \lt p \lt +\infty$ there is a frequently hypercyclic function f in
$1 \lt p \lt +\infty$ there is a frequently hypercyclic function f in  $H(\mathbb{D})$ for Tα satisfying the following estimates: there exists C > 0 such that for every
$H(\mathbb{D})$ for Tα satisfying the following estimates: there exists C > 0 such that for every  $0 \lt r \lt 1$
$0 \lt r \lt 1$
 \begin{equation*}M_{p}(f,r)\leq \left\{\begin{array}{ll}
C(1-r)^{\alpha-\frac{1}{\max(2,q)}}& \mbox{ if } \alpha \lt \frac{1}{\max(2,q)},\\
C \vert \log(1-r) \vert ^{\frac{1}{p}}& \mbox{ if } \alpha=\frac{1}{\max(2,q)},\\
C &\mbox{ if } \alpha \gt \frac{1}{\max(2,q)}.
\end{array}\right.\end{equation*}
\begin{equation*}M_{p}(f,r)\leq \left\{\begin{array}{ll}
C(1-r)^{\alpha-\frac{1}{\max(2,q)}}& \mbox{ if } \alpha \lt \frac{1}{\max(2,q)},\\
C \vert \log(1-r) \vert ^{\frac{1}{p}}& \mbox{ if } \alpha=\frac{1}{\max(2,q)},\\
C &\mbox{ if } \alpha \gt \frac{1}{\max(2,q)}.
\end{array}\right.\end{equation*}These estimates are optimal: every frequently hypercyclic function f in
 $H(\mathbb{D})$ for Tα is bounded from below by the corresponding previous estimate depending on
$H(\mathbb{D})$ for Tα is bounded from below by the corresponding previous estimate depending on  $\alpha.$
$\alpha.$(2) There is a frequently hypercyclic function f in
 $H(\mathbb{D})$ for Tα satisfying the following estimates: there exists C > 0 such that for every
$H(\mathbb{D})$ for Tα satisfying the following estimates: there exists C > 0 such that for every  $0 \lt r \lt 1$
$0 \lt r \lt 1$
 \begin{equation*}M_{\infty}(f,r)\leq \left\{\begin{array}{ll}
C(1-r)^{\alpha-\frac{1}{2}}& \mbox{ if } \alpha \lt 1/2,\\
C \vert\log(1-r)\vert& \mbox{ if } \alpha=1/2,\\
C &\mbox{ if } \alpha \gt 1/2.
\end{array}\right.\end{equation*}
\begin{equation*}M_{\infty}(f,r)\leq \left\{\begin{array}{ll}
C(1-r)^{\alpha-\frac{1}{2}}& \mbox{ if } \alpha \lt 1/2,\\
C \vert\log(1-r)\vert& \mbox{ if } \alpha=1/2,\\
C &\mbox{ if } \alpha \gt 1/2.
\end{array}\right.\end{equation*}For
 $\alpha\ne 1/2,$ these estimates are optimal: every frequently hypercyclic function f in
$\alpha\ne 1/2,$ these estimates are optimal: every frequently hypercyclic function f in  $H(\mathbb{D})$ for Tα is bounded from below by the corresponding previous estimate depending on
$H(\mathbb{D})$ for Tα is bounded from below by the corresponding previous estimate depending on  $\alpha.$
$\alpha.$
For p = 1, the following result holds. For any positive integer ![]() $\ell\geq 1$,
$\ell\geq 1$, ![]() $\log_{\ell}$ stands for
$\log_{\ell}$ stands for ![]() $\log\circ\dots\circ\log$ where log appears
$\log\circ\dots\circ\log$ where log appears ![]() $\ell$ times.
$\ell$ times.
Theorem 1.2. ([Reference Mouze and Munnier25, Proposition 4.1 and Theorem 4.4])
For any ![]() $\ell\geq 1$, there is a frequently hypercyclic function f in
$\ell\geq 1$, there is a frequently hypercyclic function f in ![]() $H(\mathbb{D})$ for Tα satisfying the following estimates: there exists C > 0 such that for every
$H(\mathbb{D})$ for Tα satisfying the following estimates: there exists C > 0 such that for every ![]() $0 \lt r \lt 1$ sufficiently large
$0 \lt r \lt 1$ sufficiently large
 \begin{equation*}M_{1}(f,r)\leq \left\{\begin{array}{ll}
C(1-r)^{\alpha}\log_\ell(-\log(1-r))& \mbox{ if } \alpha \lt 0,\\
C \vert \log(1-r) \vert \log_\ell(-\log(1-r))& \mbox{ if } \alpha=0,\\
C &\mbox{ if } \alpha \gt 0.
\end{array}\right.\end{equation*}
\begin{equation*}M_{1}(f,r)\leq \left\{\begin{array}{ll}
C(1-r)^{\alpha}\log_\ell(-\log(1-r))& \mbox{ if } \alpha \lt 0,\\
C \vert \log(1-r) \vert \log_\ell(-\log(1-r))& \mbox{ if } \alpha=0,\\
C &\mbox{ if } \alpha \gt 0.
\end{array}\right.\end{equation*} Moreover every frequently hypercyclic function f in ![]() $H(\mathbb{D})$ for Tα satisfies the following estimates:
$H(\mathbb{D})$ for Tα satisfies the following estimates:
 \begin{equation*}\liminf_{r\rightarrow 1^{-}}\left[M_{1}(f,r)(1-r)^{-\alpha}\right] \gt 0 \mbox{ if } \alpha \lt 0,\quad
\displaystyle\liminf_{r\rightarrow 1^{-}}\left[\frac{M_{1}(f,r)}{-\log(1-r)}\right] \gt 0 \mbox{ if } \alpha=0, \end{equation*}
\begin{equation*}\liminf_{r\rightarrow 1^{-}}\left[M_{1}(f,r)(1-r)^{-\alpha}\right] \gt 0 \mbox{ if } \alpha \lt 0,\quad
\displaystyle\liminf_{r\rightarrow 1^{-}}\left[\frac{M_{1}(f,r)}{-\log(1-r)}\right] \gt 0 \mbox{ if } \alpha=0, \end{equation*} \begin{equation*}\displaystyle\liminf_{r\rightarrow 1^{-}}\left[M_{1}(f,r)\right] \gt 0\mbox{ if } \alpha \gt 0.\end{equation*}
\begin{equation*}\displaystyle\liminf_{r\rightarrow 1^{-}}\left[M_{1}(f,r)\right] \gt 0\mbox{ if } \alpha \gt 0.\end{equation*} It should be noted that the study of the growth of hypercyclic or frequently hypercyclic functions started with those related to the differentiation operator on ![]() $H(\mathbb{C})$ (see for instance [Reference Blasco, Bonilla and Grosse-Erdmann6, Reference Drasin and Saksman8, Reference Grosse-Erdmann18, Reference Grosse-Erdmann19]) but was also recently extended to the partial differentiation operator [Reference Gilmore, Saksman and Tylli16] or the Dunkl operator [Reference Bernal-González and Bonilla3]. Here, as a first step, we obtain sharp results on the permissible rates of Lp-growth of hypercyclic and
$H(\mathbb{C})$ (see for instance [Reference Blasco, Bonilla and Grosse-Erdmann6, Reference Drasin and Saksman8, Reference Grosse-Erdmann18, Reference Grosse-Erdmann19]) but was also recently extended to the partial differentiation operator [Reference Gilmore, Saksman and Tylli16] or the Dunkl operator [Reference Bernal-González and Bonilla3]. Here, as a first step, we obtain sharp results on the permissible rates of Lp-growth of hypercyclic and ![]() $\mathcal{U}$-frequently hypercyclic functions for Tα. On one hand, for hypercyclicity, for any
$\mathcal{U}$-frequently hypercyclic functions for Tα. On one hand, for hypercyclicity, for any ![]() $1\leq p\leq \infty$ we find that the rate of growth
$1\leq p\leq \infty$ we find that the rate of growth ![]() $(1-r)^{\min(\alpha,0)}$ turns out to be critical and hence, for any
$(1-r)^{\min(\alpha,0)}$ turns out to be critical and hence, for any ![]() $1\leq p\leq \infty$, α = 0 is the critical exponent. Observe that in this case the critical exponent does not depend on p. We refer to Theorem 2.2. In particular this result states that for
$1\leq p\leq \infty$, α = 0 is the critical exponent. Observe that in this case the critical exponent does not depend on p. We refer to Theorem 2.2. In particular this result states that for ![]() $1\leq p\leq\infty$ there is no hypercyclic function f for Tα satisfying
$1\leq p\leq\infty$ there is no hypercyclic function f for Tα satisfying ![]() $\limsup_{r\rightarrow 1^{-}}((1-r)^{-\alpha}M_p(f,r)) \lt +\infty$ if
$\limsup_{r\rightarrow 1^{-}}((1-r)^{-\alpha}M_p(f,r)) \lt +\infty$ if ![]() $\alpha\leq 0$ while if α > 0 there exist hypercyclic functions f for Tα such that the average
$\alpha\leq 0$ while if α > 0 there exist hypercyclic functions f for Tα such that the average ![]() $M_p(f,r)$ is bounded. In passing Theorem 2.2 gives a negative answer to a question of [Reference Mouze and Munnier25] which asked if for α < 0 there is a frequently hypercyclic function gα for Tα such that
$M_p(f,r)$ is bounded. In passing Theorem 2.2 gives a negative answer to a question of [Reference Mouze and Munnier25] which asked if for α < 0 there is a frequently hypercyclic function gα for Tα such that ![]() $\limsup_{r\rightarrow 1^-}( (1-r)^{-\alpha}M_1(g_{\alpha},r)) \lt +\infty$. On the other hand, for
$\limsup_{r\rightarrow 1^-}( (1-r)^{-\alpha}M_1(g_{\alpha},r)) \lt +\infty$. On the other hand, for ![]() $\mathcal{U}$-frequent hypercyclicity, we find the same critical exponent
$\mathcal{U}$-frequent hypercyclicity, we find the same critical exponent  $\alpha=\frac{1}{\max(2,q)}$ as for frequent hypercyclicity. Therefore contrary to the previous case this exponent depends on p. Moreover we show that the
$\alpha=\frac{1}{\max(2,q)}$ as for frequent hypercyclicity. Therefore contrary to the previous case this exponent depends on p. Moreover we show that the ![]() $\mathcal{U}$-frequently hypercyclic functions and the frequently hypercyclic functions for Tα share the same admissible (and optimal) Lp-growth when α is different from the critical exponent, i.e.
$\mathcal{U}$-frequently hypercyclic functions and the frequently hypercyclic functions for Tα share the same admissible (and optimal) Lp-growth when α is different from the critical exponent, i.e.  $\alpha\ne \frac{1}{\max(2,q)}$. Concerning the case
$\alpha\ne \frac{1}{\max(2,q)}$. Concerning the case  $\alpha= \frac{1}{\max(2,q)}$ with
$\alpha= \frac{1}{\max(2,q)}$ with ![]() $1\leq p\leq\infty$, we prove that every
$1\leq p\leq\infty$, we prove that every ![]() $\mathcal{U}$-frequently hypercyclic vector f for Tα satisfies
$\mathcal{U}$-frequently hypercyclic vector f for Tα satisfies ![]() $\limsup_{r\rightarrow 1^{-}}M_p(f,r)=+\infty$, without a priori additional information on the growth of the function. We refer to Theorems 3.3 and 3.5. Nevertheless several questions remain and need to be addressed. The first question that comes to mind is the following: what is the optimal boundary growth of
$\limsup_{r\rightarrow 1^{-}}M_p(f,r)=+\infty$, without a priori additional information on the growth of the function. We refer to Theorems 3.3 and 3.5. Nevertheless several questions remain and need to be addressed. The first question that comes to mind is the following: what is the optimal boundary growth of ![]() $\mathcal{U}$-frequently hypercyclic functions for Tα when α is the critical exponent? Further if we go back to what was just said, we see that for p = 1 the critical exponent is always equal to 0 for the hypercyclic case, the
$\mathcal{U}$-frequently hypercyclic functions for Tα when α is the critical exponent? Further if we go back to what was just said, we see that for p = 1 the critical exponent is always equal to 0 for the hypercyclic case, the ![]() $\mathcal{U}$-frequently case and the frequently hypercyclic case. But surprisingly for p > 1 this critical exponent is equal to
$\mathcal{U}$-frequently case and the frequently hypercyclic case. But surprisingly for p > 1 this critical exponent is equal to  $\frac{1}{\max(2,q)}$ for the
$\frac{1}{\max(2,q)}$ for the ![]() $\mathcal{U}$-frequently or frequently hypercyclic cases and is equal to zero for the hypercyclic case. Thus, as a second question, we can wonder about what happens between
$\mathcal{U}$-frequently or frequently hypercyclic cases and is equal to zero for the hypercyclic case. Thus, as a second question, we can wonder about what happens between ![]() $\mathcal{U}$-frequent hypercyclicity and hypercyclicity. Why does the critical exponent go from
$\mathcal{U}$-frequent hypercyclicity and hypercyclicity. Why does the critical exponent go from  $\frac{1}{\max(2,q)}$ to zero? In order to understand this phenomenon, we introduce intermediate notions of linear dynamics between
$\frac{1}{\max(2,q)}$ to zero? In order to understand this phenomenon, we introduce intermediate notions of linear dynamics between ![]() $\mathcal{U}$-frequent hypercyclicity and hypercyclicity:
$\mathcal{U}$-frequent hypercyclicity and hypercyclicity: ![]() $\mathcal{U}_{\beta^{\gamma}}$-frequent hypercyclicity related to notions of upper weighted densities
$\mathcal{U}_{\beta^{\gamma}}$-frequent hypercyclicity related to notions of upper weighted densities ![]() $\overline{d}_{\beta^{\gamma}}$, with
$\overline{d}_{\beta^{\gamma}}$, with ![]() $0\leq \gamma\leq 1$ a continuous parameter, where we replace in the definition of
$0\leq \gamma\leq 1$ a continuous parameter, where we replace in the definition of ![]() $\mathcal{U}$-frequent hypercyclicity the natural upper density
$\mathcal{U}$-frequent hypercyclicity the natural upper density ![]() $\overline{d}$ by
$\overline{d}$ by ![]() $\overline{d}_{\beta^{\gamma}}$. Moreover for γ = 0
$\overline{d}_{\beta^{\gamma}}$. Moreover for γ = 0 ![]() $\mathcal{U}_{\beta^{0}}$-frequent hypercyclicity will coincide with frequent hypercyclicity and for γ = 1
$\mathcal{U}_{\beta^{0}}$-frequent hypercyclicity will coincide with frequent hypercyclicity and for γ = 1 ![]() $\mathcal{U}_{\beta^{1}}$-frequent hypercyclicity will coincide with hypercyclicity. Further for any
$\mathcal{U}_{\beta^{1}}$-frequent hypercyclicity will coincide with hypercyclicity. Further for any ![]() $0\leq \gamma\leq\gamma'\leq 1$ and for any subset
$0\leq \gamma\leq\gamma'\leq 1$ and for any subset ![]() $E\subset \mathbb{N}$, the following chain of inequalities
$E\subset \mathbb{N}$, the following chain of inequalities  $\overline{d}(E)\leq \overline{d}_{\beta^{\gamma}}(E)\leq\overline{d}_{\beta^{\gamma'}}(E)\leq\overline{d}_{\beta^{1}}(E)$ will show that the notions of
$\overline{d}(E)\leq \overline{d}_{\beta^{\gamma}}(E)\leq\overline{d}_{\beta^{\gamma'}}(E)\leq\overline{d}_{\beta^{1}}(E)$ will show that the notions of ![]() $\mathcal{U}_{\beta^{\gamma}}$-frequent hypercyclicity for
$\mathcal{U}_{\beta^{\gamma}}$-frequent hypercyclicity for ![]() $\gamma\in(0,1)$ furnish refined notions of linear dynamics between
$\gamma\in(0,1)$ furnish refined notions of linear dynamics between ![]() $\mathcal{U}$-frequent hypercyclicity and hypercyclicity. We refer the reader to the beginning of § 4 for the main definitions and properties. Similar notions of weaker densities have been recently studied in the context of linear dynamics (see for instance [Reference Bès, Menet, Peris and Puig5, Reference Bonilla and Grosse-Erdmann7, Reference Ernst, Esser and Menet11–Reference Ernst and Mouze13, Reference Menet23] and the references therein). In the present paper, we investigate the growth in terms of Lp-averages of
$\mathcal{U}$-frequent hypercyclicity and hypercyclicity. We refer the reader to the beginning of § 4 for the main definitions and properties. Similar notions of weaker densities have been recently studied in the context of linear dynamics (see for instance [Reference Bès, Menet, Peris and Puig5, Reference Bonilla and Grosse-Erdmann7, Reference Ernst, Esser and Menet11–Reference Ernst and Mouze13, Reference Menet23] and the references therein). In the present paper, we investigate the growth in terms of Lp-averages of ![]() $\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic functions for Tα. In particular, for
$\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic functions for Tα. In particular, for ![]() $0 \lt \gamma \lt 1$, and for p > 1 we find that the critical exponent is given by
$0 \lt \gamma \lt 1$, and for p > 1 we find that the critical exponent is given by  $\alpha =\frac{1-\gamma}{\max(2,q)}$. Hence let us observe that this critical exponent:
$\alpha =\frac{1-\gamma}{\max(2,q)}$. Hence let us observe that this critical exponent:
• tends to
 $\frac{1}{\max(2,q)}$ as γ tends to zero, i.e. tends to the critical exponent for
$\frac{1}{\max(2,q)}$ as γ tends to zero, i.e. tends to the critical exponent for  $\mathcal{U}$-frequent hypercyclicity;
$\mathcal{U}$-frequent hypercyclicity;• tends to 0 as γ tends to 1, i.e. tends to the critical exponent for hypercyclicity.
These estimates thus allow to highlight a continuous path between the rate of growth of hypercyclic and ![]() $\mathcal{U}$-frequently hypercyclic functions: the growth (in terms of Lp-averages) of a hypercyclic function for Tα continuously depends on the frequency of visits (measured by the densities
$\mathcal{U}$-frequently hypercyclic functions: the growth (in terms of Lp-averages) of a hypercyclic function for Tα continuously depends on the frequency of visits (measured by the densities ![]() $\overline{d}_{\beta^\gamma}$,
$\overline{d}_{\beta^\gamma}$, ![]() $0\leq\gamma\leq 1$) of non-empty open subsets by its orbit under the action of Tα. We also show that the estimates on the growth of
$0\leq\gamma\leq 1$) of non-empty open subsets by its orbit under the action of Tα. We also show that the estimates on the growth of ![]() $\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic functions that we obtained are optimal. To do this, we apply a method based on the use of Rudin–Shapiro polynomials and inspired by a construction of frequently hypercyclic functions with optimal growth for differentiation operator on
$\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic functions that we obtained are optimal. To do this, we apply a method based on the use of Rudin–Shapiro polynomials and inspired by a construction of frequently hypercyclic functions with optimal growth for differentiation operator on ![]() $H(\mathbb{C})$ due to Drasin and Saksman [Reference Drasin and Saksman8] and that has also been adapted for the proofs of Theorems 1.1 and 1.2 in [Reference Mouze and Munnier24, Reference Mouze and Munnier25]. For all these results, we refer the reader to Theorems 4.5, 4.10 and 4.12. Finally let us return to the first question mentioned above. In the last section, we answer it by showing that the optimal growth of
$H(\mathbb{C})$ due to Drasin and Saksman [Reference Drasin and Saksman8] and that has also been adapted for the proofs of Theorems 1.1 and 1.2 in [Reference Mouze and Munnier24, Reference Mouze and Munnier25]. For all these results, we refer the reader to Theorems 4.5, 4.10 and 4.12. Finally let us return to the first question mentioned above. In the last section, we answer it by showing that the optimal growth of ![]() $\mathcal{U}$-frequently and
$\mathcal{U}$-frequently and ![]() $\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic functions for Tα coincides whenever α is the critical exponent: actually the Lp-growth can be arbitrarily slow as in the hypercyclic case. We refer to Theorem 5.12.
$\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic functions for Tα coincides whenever α is the critical exponent: actually the Lp-growth can be arbitrarily slow as in the hypercyclic case. We refer to Theorem 5.12.
The paper is organized as follows. In § 2 and 3, we establish the boundary behaviour of hypercyclic functions and ![]() $\mathcal{U}$-frequently hypercyclic functions for Tα respectively. In § 4, we deal with the
$\mathcal{U}$-frequently hypercyclic functions for Tα respectively. In § 4, we deal with the ![]() $\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic functions for Tα. In § 5, we turn our attention to the specific case of critical exponent.
$\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic functions for Tα. In § 5, we turn our attention to the specific case of critical exponent.
Throughout the paper, whenever A and B depend on some parameters, we will use the notation ![]() $A\lesssim B$ (resp.
$A\lesssim B$ (resp. ![]() $A \gt rsim B$) to mean
$A \gt rsim B$) to mean ![]() $A\leq CB$ (resp.
$A\leq CB$ (resp. ![]() $A\geq C B$) for some constant C > 0 that does not depend on the involved parameters.
$A\geq C B$) for some constant C > 0 that does not depend on the involved parameters.
2. Growth of hypercyclic functions
In this section, we are going to establish the rate of growth of hypercyclic functions with respect to the weighted Taylor shift operator Tα. To do this, inspired by the proofs of [Reference Grosse-Erdmann18, Theorem (A)] and [Reference Bernal-González and Bonilla3, Theorem 3], where the authors are interested in the rate of growth of hypercyclic functions with respect to the Mac-Lane operator or the Dunkl operator respectively, we need an important tool in linear dynamics: the Universality Criterion. Indeed a natural extension of the notion of hypercyclicity is the concept of universality. A sequence of continuous linear mappings ![]() $L_n:X\rightarrow Y$ between topological vector spaces
$L_n:X\rightarrow Y$ between topological vector spaces ![]() $X,Y$ is said to be universal whenever there exists a vector
$X,Y$ is said to be universal whenever there exists a vector ![]() $x\in X$ such that the set
$x\in X$ such that the set ![]() $\{L_nx\ ;\ n\in\mathbb{N}\}$ is dense in Y. Such a vector x is called a universal vector for
$\{L_nx\ ;\ n\in\mathbb{N}\}$ is dense in Y. Such a vector x is called a universal vector for ![]() $(L_n)$. Observe that an operator
$(L_n)$. Observe that an operator ![]() $T:X\rightarrow X$ is hypercyclic if and only if the sequence
$T:X\rightarrow X$ is hypercyclic if and only if the sequence ![]() $(T^n)$ is universal. The following result which is known as the Universality Criterion furnishes a sufficient condition for universality [Reference Grosse-Erdmann17]. It is a refined version of the hypercyclicity criterion [Reference Gethner and Shapiro15, Reference Kitai22].
$(T^n)$ is universal. The following result which is known as the Universality Criterion furnishes a sufficient condition for universality [Reference Grosse-Erdmann17]. It is a refined version of the hypercyclicity criterion [Reference Gethner and Shapiro15, Reference Kitai22].
Theorem 2.1. (Universality Criterion) Assume that X and Y are topological vector spaces, such that X is a Baire space and Y is separable and metrizable. Let ![]() $L_j:X\rightarrow Y$ be a sequence of continuous linear mappings. Suppose that there are dense subsets X 0 of X and Y 0 of Y and mappings
$L_j:X\rightarrow Y$ be a sequence of continuous linear mappings. Suppose that there are dense subsets X 0 of X and Y 0 of Y and mappings ![]() $S_j:Y_0\rightarrow X$ such that
$S_j:Y_0\rightarrow X$ such that
(i) for every
 $x\in X_0$,
$x\in X_0$,  $L_jx\rightarrow 0$,
$L_jx\rightarrow 0$,(ii) for every
 $y\in Y_0$,
$y\in Y_0$,  $S_jy\rightarrow 0$,
$S_jy\rightarrow 0$,(iii) for every
 $y\in Y_0$,
$y\in Y_0$,  $(L_jS_j)y\rightarrow y$.
$(L_jS_j)y\rightarrow y$.
Then ![]() $(L_j)$ is universal and the set of universal vectors for
$(L_j)$ is universal and the set of universal vectors for ![]() $(L_j)$ is residual in X.
$(L_j)$ is residual in X.
Now we are ready to obtain the critical rate of growth for hypercyclic functions with respect to the weighted Taylor shift operator Tα. The following statement holds.
Theorem 2.2. Let ![]() $1\leq p\leq\infty$.
$1\leq p\leq\infty$.
(1) Let
 $\alpha\leq 0.$
$\alpha\leq 0.$(a) For any function
 $\varphi:[0,1)\rightarrow\mathbb{R}_+$ with
$\varphi:[0,1)\rightarrow\mathbb{R}_+$ with  $\varphi(r)\rightarrow \infty$ as
$\varphi(r)\rightarrow \infty$ as  $r\rightarrow 1^{-}$ there is a hypercyclic function f for Tα with
$r\rightarrow 1^{-}$ there is a hypercyclic function f for Tα with
 \begin{equation*}M_p(f,r)\lesssim\varphi(r) (1-r)^{\alpha}\quad\hbox{for }0 \lt r \lt 1\hbox{ sufficiently close to }1.\end{equation*}
\begin{equation*}M_p(f,r)\lesssim\varphi(r) (1-r)^{\alpha}\quad\hbox{for }0 \lt r \lt 1\hbox{ sufficiently close to }1.\end{equation*}(b) There is no hypercyclic function f for Tα that satisfies, for
 $0 \lt r \lt 1$
$0 \lt r \lt 1$
 \begin{equation*}M_p(f,r)\leq C (1-r)^{\alpha},\end{equation*}
\begin{equation*}M_p(f,r)\leq C (1-r)^{\alpha},\end{equation*}where C > 0.
(2) Let α > 0.
(a) There is a hypercyclic function f for Tα with
 \begin{equation*}M_p(f,r)\leq C\end{equation*}
\begin{equation*}M_p(f,r)\leq C\end{equation*}for some C > 0.
(b) For any function
 $\varphi:[0,1)\rightarrow\mathbb{R}_+$ with
$\varphi:[0,1)\rightarrow\mathbb{R}_+$ with  $\varphi(r)\rightarrow 0$ as
$\varphi(r)\rightarrow 0$ as  $r\rightarrow 1^{-}$, there is no hypercyclic function f for Tα that satisfies, for
$r\rightarrow 1^{-}$, there is no hypercyclic function f for Tα that satisfies, for  $0 \lt r \lt 1$
$0 \lt r \lt 1$
 \begin{equation*}M_p(f,r)\leq \varphi(r).\end{equation*}
\begin{equation*}M_p(f,r)\leq \varphi(r).\end{equation*}
Proof. Since we have for ![]() $1\leq p\leq l$
$1\leq p\leq l$
it suffices to prove assertions (1a) and (2a) for ![]() $M_{\infty}(f,r)$ and assertions (1b) and (2b) for
$M_{\infty}(f,r)$ and assertions (1b) and (2b) for ![]() $M_1(f,r)$.
$M_1(f,r)$.
(1) We begin by the case
 $\alpha\leq 0$.
$\alpha\leq 0$.First we can assume without loss of generality that the function φ is increasing and continuous with
 $\varphi(0) \gt 0$. Let us consider the space X of all functions f in
$\varphi(0) \gt 0$. Let us consider the space X of all functions f in  $H(\mathbb{D})$ with
$H(\mathbb{D})$ with  $f(z)=\sum\limits_{k\geq 0}a_k z^k$ satisfying for any
$f(z)=\sum\limits_{k\geq 0}a_k z^k$ satisfying for any  $n\geq 0$,
$n\geq 0$,  $\rho_n(f) \lt +\infty$ and
$\rho_n(f) \lt +\infty$ and  $\rho_n(f)\rightarrow 0$ as
$\rho_n(f)\rightarrow 0$ as  $n\rightarrow +\infty$, where
$n\rightarrow +\infty$, where
 \begin{equation*}\rho_n(f)=\sup_{\vert z\vert \lt 1}\left\{\left\vert\sum_{k=n}^{+\infty}a_k z^k\right\vert (1-\vert z\vert)^{-\alpha}[\varphi(\vert z\vert)]^{-1}\right\}.\end{equation*}
\begin{equation*}\rho_n(f)=\sup_{\vert z\vert \lt 1}\left\{\left\vert\sum_{k=n}^{+\infty}a_k z^k\right\vert (1-\vert z\vert)^{-\alpha}[\varphi(\vert z\vert)]^{-1}\right\}.\end{equation*}It is easy to check that X endowed with the norm
 $\Vert .\Vert=\sup_{n} \rho_n(.)$ is a Banach space. Therefore
$\Vert .\Vert=\sup_{n} \rho_n(.)$ is a Banach space. Therefore  $(X,\Vert .\Vert)$ is a Baire space. For all integer j, let
$(X,\Vert .\Vert)$ is a Baire space. For all integer j, let  $L_j:X\rightarrow H(\mathbb{D})$ be the operator given by
$L_j:X\rightarrow H(\mathbb{D})$ be the operator given by  $L_jf=T^jf$. Clearly
$L_jf=T^jf$. Clearly  $(L_j)$ is a sequence of continuous linear operators. We choose
$(L_j)$ is a sequence of continuous linear operators. We choose  $X_0=Y_0=\mathcal{P}$ the set of polynomials. The set
$X_0=Y_0=\mathcal{P}$ the set of polynomials. The set  $\mathcal{P}$ is dense in
$\mathcal{P}$ is dense in  $H(\mathbb{D})$. Moreover, setting the polynomial
$H(\mathbb{D})$. Moreover, setting the polynomial  $s_N(f)=\sum_{k=0}^N a_k z^k$ we get
$s_N(f)=\sum_{k=0}^N a_k z^k$ we get
 \begin{equation*}\rho_n(f-s_N(f))=\left\{\begin{array}{ll}\rho_n(f)& \hbox{ for }n\geq N+1\\
\rho_{N+1}(f)& \hbox{ for }n\leq N\end{array}\right.\end{equation*}
\begin{equation*}\rho_n(f-s_N(f))=\left\{\begin{array}{ll}\rho_n(f)& \hbox{ for }n\geq N+1\\
\rho_{N+1}(f)& \hbox{ for }n\leq N\end{array}\right.\end{equation*}which implies
 $\Vert f-s_N(f)\Vert=\sup_{n\geq N+1}\rho_n(f)\rightarrow 0$ as N tends to infinity. Hence
$\Vert f-s_N(f)\Vert=\sup_{n\geq N+1}\rho_n(f)\rightarrow 0$ as N tends to infinity. Hence  $\mathcal{P}$ is dense in X. Then we define the operators Sj as follows
$\mathcal{P}$ is dense in X. Then we define the operators Sj as follows
 \begin{equation*}S_j:\mathcal{P}\rightarrow X,\quad S_j(\sum_{k=0}^n a_k z^k)=\sum_{k=0}^n a_k\frac{(k+1)^{\alpha}}{(k+j+1)^{\alpha}} z^{k+j}.\end{equation*}
\begin{equation*}S_j:\mathcal{P}\rightarrow X,\quad S_j(\sum_{k=0}^n a_k z^k)=\sum_{k=0}^n a_k\frac{(k+1)^{\alpha}}{(k+j+1)^{\alpha}} z^{k+j}.\end{equation*}Clearly we have, for all
 $P\in \mathcal{P}$,
$P\in \mathcal{P}$,
 \begin{equation*}L_j(P)\rightarrow 0,\hbox{ as }j\rightarrow +\infty,\quad \hbox{ and } L_jS_j(P)=P.\end{equation*}
\begin{equation*}L_j(P)\rightarrow 0,\hbox{ as }j\rightarrow +\infty,\quad \hbox{ and } L_jS_j(P)=P.\end{equation*}Now we prove that, for all
 $P\in \mathcal{P},$
$P\in \mathcal{P},$  $S_j(P) \rightarrow 0$, as
$S_j(P) \rightarrow 0$, as  $j\rightarrow +\infty$. Since
$j\rightarrow +\infty$. Since  $S_j(z^k)=(k+1)^{\alpha}S_{j+k}(1)$, it suffices to show that
$S_j(z^k)=(k+1)^{\alpha}S_{j+k}(1)$, it suffices to show that  $S_j(1)\rightarrow 0$, as
$S_j(1)\rightarrow 0$, as  $j\rightarrow +\infty$. To do this, observe that
$j\rightarrow +\infty$. To do this, observe that
 \begin{equation*}\Vert S_j(1)\Vert=\sup_{0 \lt r \lt 1}\frac{r^j(1-r)^{-\alpha}}{(j+1)^{\alpha}\varphi(r)}.\end{equation*}
\begin{equation*}\Vert S_j(1)\Vert=\sup_{0 \lt r \lt 1}\frac{r^j(1-r)^{-\alpha}}{(j+1)^{\alpha}\varphi(r)}.\end{equation*}Let us define
 $h_j:[0,1)\rightarrow \mathbb{R}_+$ given by
$h_j:[0,1)\rightarrow \mathbb{R}_+$ given by  $h_j(r)=\frac{r^j(1-r)^{-\alpha}}{\varphi(r)}$. We have
$h_j(r)=\frac{r^j(1-r)^{-\alpha}}{\varphi(r)}$. We have  $h_j(0)=0=\lim\limits_{r\rightarrow 1^{-}} h_j(r)$. Let
$h_j(0)=0=\lim\limits_{r\rightarrow 1^{-}} h_j(r)$. Let  $0 \lt r_j \lt 1$ with
$0 \lt r_j \lt 1$ with  $h_j(r_j)=\sup_{0 \lt r \lt 1}\frac{r^j(1-r)^{-\alpha}}{\varphi(r)}$. If
$h_j(r_j)=\sup_{0 \lt r \lt 1}\frac{r^j(1-r)^{-\alpha}}{\varphi(r)}$. If  $r_{j+1} \lt r_j,$ we get
$r_{j+1} \lt r_j,$ we get
 \begin{equation*}h_{j+1}(r_{j+1})=r_{j+1}h_j(r_{j+1}) \lt r_j h_j(r_{j+1})\leq r_j h_j(r_j)=h_{j+1}(r_j)\end{equation*}
\begin{equation*}h_{j+1}(r_{j+1})=r_{j+1}h_j(r_{j+1}) \lt r_j h_j(r_{j+1})\leq r_j h_j(r_j)=h_{j+1}(r_j)\end{equation*}which gives a contradiction. Hence the sequence
 $(r_j)$ is increasing. If
$(r_j)$ is increasing. If  $r_j\rightarrow \gamma$ with γ < 1, then
$r_j\rightarrow \gamma$ with γ < 1, then
 \begin{equation*}\Vert S_j(1)\Vert=(j+1)^{-\alpha}h_j(r_j)\leq (j+1)^{-\alpha}\frac{\gamma^j}{\varphi(0)}\rightarrow 0,\hbox{ as }j\rightarrow +\infty.\end{equation*}
\begin{equation*}\Vert S_j(1)\Vert=(j+1)^{-\alpha}h_j(r_j)\leq (j+1)^{-\alpha}\frac{\gamma^j}{\varphi(0)}\rightarrow 0,\hbox{ as }j\rightarrow +\infty.\end{equation*}Otherwise
 $r_j\rightarrow 1$ and
$r_j\rightarrow 1$ and
 \begin{equation*}\Vert S_j(1)\Vert\leq \frac{(j+1)^{-\alpha}}{\varphi(r_j)}\left(\frac{j}{j-\alpha}\right)^j
\left(1-\frac{j}{j-\alpha}\right)^{-\alpha}\rightarrow 0,\hbox{ as }j\rightarrow +\infty.\end{equation*}
\begin{equation*}\Vert S_j(1)\Vert\leq \frac{(j+1)^{-\alpha}}{\varphi(r_j)}\left(\frac{j}{j-\alpha}\right)^j
\left(1-\frac{j}{j-\alpha}\right)^{-\alpha}\rightarrow 0,\hbox{ as }j\rightarrow +\infty.\end{equation*}Thus we have
 $\Vert S_j(1)\Vert\rightarrow 0$ as j tends to infinity. We apply the universality criterion to obtain universal elements for the sequence
$\Vert S_j(1)\Vert\rightarrow 0$ as j tends to infinity. We apply the universality criterion to obtain universal elements for the sequence  $(L_j)$ that are hypercyclic functions for Tα satisfying the growth condition required.
$(L_j)$ that are hypercyclic functions for Tα satisfying the growth condition required.For assertion (1b), assume that
 $f=\sum\limits_{k\geq 0}a_kz^k$ is a function in
$f=\sum\limits_{k\geq 0}a_kz^k$ is a function in  $H(\mathbb{D})$ with, for all
$H(\mathbb{D})$ with, for all  $0 \lt r \lt 1$,
$0 \lt r \lt 1$,  $M_1(f,r)\leq C (1-r)^{\alpha}$, for some C > 0. By Cauchy estimates we get
$M_1(f,r)\leq C (1-r)^{\alpha}$, for some C > 0. By Cauchy estimates we get
 \begin{equation*}\vert a_n\vert\leq \frac{M_1(f,r)}{r^n}.\end{equation*}
\begin{equation*}\vert a_n\vert\leq \frac{M_1(f,r)}{r^n}.\end{equation*}We obtain, for all
 $n\geq 0$ and all
$n\geq 0$ and all  $0 \lt r \lt 1$,
$0 \lt r \lt 1$,
 \begin{equation*}\vert a_n w_1(\alpha)\dots w_n(\alpha)\vert\leq C \frac{\vert w_1(\alpha)\dots w_n(\alpha)\vert}{r^n}(1-r)^{\alpha}.\end{equation*}
\begin{equation*}\vert a_n w_1(\alpha)\dots w_n(\alpha)\vert\leq C \frac{\vert w_1(\alpha)\dots w_n(\alpha)\vert}{r^n}(1-r)^{\alpha}.\end{equation*}Hence we get for all
 $n\geq 0$,
$n\geq 0$,
 \begin{equation*}\vert a_n (n+1)^{\alpha}\vert\leq C \frac{(n+1)^{\alpha}}{e^{-n/(n+1)}}(1-e^{-1/(n+1)})^{\alpha}\end{equation*}
\begin{equation*}\vert a_n (n+1)^{\alpha}\vert\leq C \frac{(n+1)^{\alpha}}{e^{-n/(n+1)}}(1-e^{-1/(n+1)})^{\alpha}\end{equation*}which is bounded. Hence f cannot be hypercyclic for Tα.
(2) Now let us consider the case α > 0.
First we can assume without loss of generality that the function φ is increasing and continuous with
 $\varphi(0) \gt 0$. Let us consider the Banach space
$\varphi(0) \gt 0$. Let us consider the Banach space  $(H^{\infty}(\mathbb{D}),\Vert .\Vert)$
$(H^{\infty}(\mathbb{D}),\Vert .\Vert)$
 \begin{equation*}H^{\infty}(\mathbb{D})=\left\{f\in H(\mathbb{D})\ ;\ \Vert f\Vert:=\sup_{0 \lt r \lt 1}M_{\infty}(f,r) \lt \infty \right\}\end{equation*}
\begin{equation*}H^{\infty}(\mathbb{D})=\left\{f\in H(\mathbb{D})\ ;\ \Vert f\Vert:=\sup_{0 \lt r \lt 1}M_{\infty}(f,r) \lt \infty \right\}\end{equation*}which is continuously embedded in
 $H(\mathbb{D})$. We set X the closure of the polynomials in
$H(\mathbb{D})$. We set X the closure of the polynomials in  $H^{\infty}(\mathbb{D})$. Let us define the sequences
$H^{\infty}(\mathbb{D})$. Let us define the sequences  $(L_j)$ and
$(L_j)$ and  $(S_j)$ of linear operators as in the previous case. Clearly we have, for all
$(S_j)$ of linear operators as in the previous case. Clearly we have, for all  $P\in \mathcal{P}$, where
$P\in \mathcal{P}$, where  $\mathcal{P}$ is the set of polynomials,
$\mathcal{P}$ is the set of polynomials,
 \begin{equation*}L_j(P)\rightarrow 0,\hbox{ as }j\rightarrow +\infty,\quad \hbox{ and } L_jS_j(P)=P.\end{equation*}
\begin{equation*}L_j(P)\rightarrow 0,\hbox{ as }j\rightarrow +\infty,\quad \hbox{ and } L_jS_j(P)=P.\end{equation*}Now we prove that, for all
 $P\in \mathcal{P},$
$P\in \mathcal{P},$  $S_j(P) \rightarrow 0$, as
$S_j(P) \rightarrow 0$, as  $j\rightarrow +\infty$. To do this, it suffices to show that
$j\rightarrow +\infty$. To do this, it suffices to show that  $S_j(1)\rightarrow 0$, as
$S_j(1)\rightarrow 0$, as  $j\rightarrow +\infty$. Since
$j\rightarrow +\infty$. Since
 \begin{equation*}\Vert S_j(1)\Vert\leq\frac{1}{(j+1)^{\alpha}}\end{equation*}
\begin{equation*}\Vert S_j(1)\Vert\leq\frac{1}{(j+1)^{\alpha}}\end{equation*}and α > 0 we have
 $\Vert S_j(1)\Vert\rightarrow 0$ as j tends to infinity. We apply the universality criterion to obtain universal elements for the sequence
$\Vert S_j(1)\Vert\rightarrow 0$ as j tends to infinity. We apply the universality criterion to obtain universal elements for the sequence  $(L_j)$. These universal vectors are clearly hypercyclic functions for Tα satisfying the growth condition required.
$(L_j)$. These universal vectors are clearly hypercyclic functions for Tα satisfying the growth condition required.For assertion (2b), assume that
 $f=\sum\limits_{k\geq 0}a_kz^k$ is a function in
$f=\sum\limits_{k\geq 0}a_kz^k$ is a function in  $H(\mathbb{D})$ with, for all
$H(\mathbb{D})$ with, for all  $0 \lt r \lt 1$,
$0 \lt r \lt 1$,  $M_1(f,r)\leq \varphi(r)$, where
$M_1(f,r)\leq \varphi(r)$, where  $\varphi:[0,1)\rightarrow\mathbb{R}_+$ is a function such that
$\varphi:[0,1)\rightarrow\mathbb{R}_+$ is a function such that  $\varphi(r)\rightarrow 0$ as
$\varphi(r)\rightarrow 0$ as  $r\rightarrow 1^{-}$. We obviously may assume that φ is continuous and decreasing. By Cauchy estimates, we get
$r\rightarrow 1^{-}$. We obviously may assume that φ is continuous and decreasing. By Cauchy estimates, we get
 \begin{equation*}\vert a_n\vert\leq \frac{\varphi(r)}{r^n}.\end{equation*}
\begin{equation*}\vert a_n\vert\leq \frac{\varphi(r)}{r^n}.\end{equation*}We obtain, for all
 $n\geq 0$ and all
$n\geq 0$ and all  $0 \lt r \lt 1$,
$0 \lt r \lt 1$,
 \begin{equation*}\vert a_n (n+1)^{\alpha}\vert\leq \frac{(n+1)^{\alpha}\varphi(r)}{r^n}.\end{equation*}
\begin{equation*}\vert a_n (n+1)^{\alpha}\vert\leq \frac{(n+1)^{\alpha}\varphi(r)}{r^n}.\end{equation*}Let us choose a sequence
 $(r_n)$ such that
$(r_n)$ such that  $r_n\geq \max(1-1/n,\varphi^{-1}\left((n+1)^{-\alpha}\right))$. Hence we get, for all
$r_n\geq \max(1-1/n,\varphi^{-1}\left((n+1)^{-\alpha}\right))$. Hence we get, for all  $n\in\mathbb{N}$,
$n\in\mathbb{N}$,
 \begin{equation*}\vert a_n (n+1)^{\alpha}\vert\leq \frac{(n+1)^{\alpha}\varphi(r_n)}{r_n^n}\leq \mathrm{e}^{-n\log(1-1/n)}\end{equation*}
\begin{equation*}\vert a_n (n+1)^{\alpha}\vert\leq \frac{(n+1)^{\alpha}\varphi(r_n)}{r_n^n}\leq \mathrm{e}^{-n\log(1-1/n)}\end{equation*}which is bounded. Hence f cannot be hypercyclic for Tα.
Remark 2.3. For α < 0, Theorem 2.2 ensures that every hypercyclic function f for Tα satisfies
 \begin{equation*}\displaystyle\limsup_{r\rightarrow 1^{-}}\left[(1-r)^{-\alpha}M_1(f,r)\right]=+\infty.\end{equation*}
\begin{equation*}\displaystyle\limsup_{r\rightarrow 1^{-}}\left[(1-r)^{-\alpha}M_1(f,r)\right]=+\infty.\end{equation*} Since a frequently hypercyclic function is necessarily hypercyclic, this observation gives a negative answer to the first part of the question from Remark 4.5 of [Reference Mouze and Munnier25] which asked if for α < 0 there is a frequently hypercyclic function gα for Tα such that  $\limsup\limits_{r\rightarrow 1^-}( (1-r)^{-\alpha}M_1(g_{\alpha},r)) \lt +\infty$.
$\limsup\limits_{r\rightarrow 1^-}( (1-r)^{-\alpha}M_1(g_{\alpha},r)) \lt +\infty$.
3. Growth of  $\mathcal{U}$-frequently hypercyclic functions
$\mathcal{U}$-frequently hypercyclic functions
In this section, we are interested in the growth of ![]() $\mathcal{U}$-frequently hypercyclic functions for Tα. First of all, in the sequel, we will need the following easy lemmas.
$\mathcal{U}$-frequently hypercyclic functions for Tα. First of all, in the sequel, we will need the following easy lemmas.
Lemma 3.1. Let ![]() $N\in\mathbb{N}$. Let AN be a subset of
$N\in\mathbb{N}$. Let AN be a subset of ![]() $\{1,\dots,N\}$. For all
$\{1,\dots,N\}$. For all ![]() $\gamma\in\mathbb{R}\setminus\{-1\}$ the following estimate holds
$\gamma\in\mathbb{R}\setminus\{-1\}$ the following estimate holds
 \begin{equation*}\sum_{k\in A_N} (k+1)^{\gamma}\geq\left\{\begin{array}{ll}
\displaystyle\frac{(\#A_N+1)^{\gamma +1}-1}{\gamma+1}&\hbox{ if }\gamma \geq 0,\\
\displaystyle\frac{(N+2)^{\gamma+1}}{\gamma+1}\left(1-\left(1-\frac{\#A_N}{N+2}\right)^{\gamma+1}\right)&\hbox{ if }\gamma \lt 0,\ \gamma\ne -1.\end{array}\right.
\end{equation*}
\begin{equation*}\sum_{k\in A_N} (k+1)^{\gamma}\geq\left\{\begin{array}{ll}
\displaystyle\frac{(\#A_N+1)^{\gamma +1}-1}{\gamma+1}&\hbox{ if }\gamma \geq 0,\\
\displaystyle\frac{(N+2)^{\gamma+1}}{\gamma+1}\left(1-\left(1-\frac{\#A_N}{N+2}\right)^{\gamma+1}\right)&\hbox{ if }\gamma \lt 0,\ \gamma\ne -1.\end{array}\right.
\end{equation*}Proof. We begin by the case ![]() $\gamma \geq 0$. We write
$\gamma \geq 0$. We write
 \begin{equation*}\sum_{k\in A_N} (k+1)^{\gamma}\geq \sum_{k=1}^{\#A_N} (k+1)^{\gamma}\geq \int_0^{\#A_N}(t+1)^{\gamma}\,\mathrm{d}t,\end{equation*}
\begin{equation*}\sum_{k\in A_N} (k+1)^{\gamma}\geq \sum_{k=1}^{\#A_N} (k+1)^{\gamma}\geq \int_0^{\#A_N}(t+1)^{\gamma}\,\mathrm{d}t,\end{equation*}which gives the announced result.
For γ < 0 with ![]() $\gamma\ne -1$, we obtain in an analogue way
$\gamma\ne -1$, we obtain in an analogue way
 \begin{equation*}\sum_{k\in A_N} (k+1)^{\gamma}\geq \sum_{k=N-\#A_N+1}^{N} (k+1)^{\gamma}\geq \int_{N-\#A_N+1}^{N+1}(t+1)^{\gamma}\,\mathrm{d}t,\end{equation*}
\begin{equation*}\sum_{k\in A_N} (k+1)^{\gamma}\geq \sum_{k=N-\#A_N+1}^{N} (k+1)^{\gamma}\geq \int_{N-\#A_N+1}^{N+1}(t+1)^{\gamma}\,\mathrm{d}t,\end{equation*}which allows to finish the proof.
Lemma 3.2. Let ![]() $(u_k)$ and
$(u_k)$ and ![]() $(v_k)$ be two sequences of non-negative real numbers. Assume that
$(v_k)$ be two sequences of non-negative real numbers. Assume that ![]() $(v_k)$ is decreasing. For any increasing sub-sequence
$(v_k)$ is decreasing. For any increasing sub-sequence ![]() $(N_j)\subset\mathbb{N}$, the following inequality holds, for all
$(N_j)\subset\mathbb{N}$, the following inequality holds, for all ![]() $l\geq 1$:
$l\geq 1$:
 \begin{equation*}\sum_{k=1+N_0}^{N_l}u_kv_k\geq S_{N_l}v_{N_l}-S_{N_0}v_{N_0}+\sum_{j=1}^l S_{N_{j-1}}(v_{N_{j-1}}-v_{N_j}),\end{equation*}
\begin{equation*}\sum_{k=1+N_0}^{N_l}u_kv_k\geq S_{N_l}v_{N_l}-S_{N_0}v_{N_0}+\sum_{j=1}^l S_{N_{j-1}}(v_{N_{j-1}}-v_{N_j}),\end{equation*} with  $S_N=\sum\limits_{k=1}^N u_k$.
$S_N=\sum\limits_{k=1}^N u_k$.
Proof. Observe that ![]() $(S_N)$ is increasing and
$(S_N)$ is increasing and ![]() $(v_k)$ is decreasing. Thus by using a summation by parts we derive
$(v_k)$ is decreasing. Thus by using a summation by parts we derive
 \begin{align*}\displaystyle\sum_{k=1+N_0}^{N_l}u_kv_k & =\sum_{j=1}^l\sum_{k=1+N_{j-1}}^{N_j}u_kv_k \\
&=\displaystyle\sum_{j=1}^l\left(S_{N_j}v_{N_j}-S_{N_{j-1}}v_{N_{j-1}} +\sum_{k=N_{j-1}}^{N_j-1}S_k(v_k-v_{k+1})\right)\\
&= \displaystyle S_{N_l}v_{N_l}-S_{N_0}v_{N_0}+\sum_{j=1}^l\sum_{k=N_{j-1}}^{N_j-1}S_k(v_k-v_{k+1})\\
& \geq \displaystyle S_{N_l}v_{N_l}-S_{N_0}v_{N_0}+\sum_{j=1}^l S_{N_{j-1}}(v_{N_{j-1}}-v_{N_j}).\end{align*}
\begin{align*}\displaystyle\sum_{k=1+N_0}^{N_l}u_kv_k & =\sum_{j=1}^l\sum_{k=1+N_{j-1}}^{N_j}u_kv_k \\
&=\displaystyle\sum_{j=1}^l\left(S_{N_j}v_{N_j}-S_{N_{j-1}}v_{N_{j-1}} +\sum_{k=N_{j-1}}^{N_j-1}S_k(v_k-v_{k+1})\right)\\
&= \displaystyle S_{N_l}v_{N_l}-S_{N_0}v_{N_0}+\sum_{j=1}^l\sum_{k=N_{j-1}}^{N_j-1}S_k(v_k-v_{k+1})\\
& \geq \displaystyle S_{N_l}v_{N_l}-S_{N_0}v_{N_0}+\sum_{j=1}^l S_{N_{j-1}}(v_{N_{j-1}}-v_{N_j}).\end{align*} We are ready to establish the boundary behaviour of ![]() $\mathcal{U}$-frequently hypercyclic functions for Tα. Actually we are going to prove that these functions share the same optimal growth as frequently hypercyclic functions except in the case where α is the critical exponent, for which we will show in § 5 that the growth can be arbitrarily slow. We begin by the case p > 1.
$\mathcal{U}$-frequently hypercyclic functions for Tα. Actually we are going to prove that these functions share the same optimal growth as frequently hypercyclic functions except in the case where α is the critical exponent, for which we will show in § 5 that the growth can be arbitrarily slow. We begin by the case p > 1.
Theorem 3.3. Let f be a ![]() $\mathcal{U}$-frequently hypercyclic function for the operator Tα and
$\mathcal{U}$-frequently hypercyclic function for the operator Tα and ![]() $1 \lt p\leq\infty$. Then the following estimates hold
$1 \lt p\leq\infty$. Then the following estimates hold
 \begin{align*}&\limsup_{r\rightarrow 1^{-}}\left((1-r)^{\frac{1}{\max(2,q)}-\alpha}M_p(f,r)\right) \gt 0,\quad\hbox{ if }\alpha \lt \frac{1}{\max(2,q)},\\
&\limsup_{r\rightarrow 1^{-}}\left(M_p(f,r)\right)=+\infty,\quad\hbox{ if }\alpha=\frac{1}{\max(2,q)},\\
&\limsup_{r\rightarrow 1^{-}}M_p(f,r) \gt 0,\quad\hbox{ if }\alpha \gt \frac{1}{\max(2,q)}.
\end{align*}
\begin{align*}&\limsup_{r\rightarrow 1^{-}}\left((1-r)^{\frac{1}{\max(2,q)}-\alpha}M_p(f,r)\right) \gt 0,\quad\hbox{ if }\alpha \lt \frac{1}{\max(2,q)},\\
&\limsup_{r\rightarrow 1^{-}}\left(M_p(f,r)\right)=+\infty,\quad\hbox{ if }\alpha=\frac{1}{\max(2,q)},\\
&\limsup_{r\rightarrow 1^{-}}M_p(f,r) \gt 0,\quad\hbox{ if }\alpha \gt \frac{1}{\max(2,q)}.
\end{align*} For  $\alpha\ne\frac{1}{\max(2,q)}$, these results are optimal in the following sense: for all p > 1, there exists a
$\alpha\ne\frac{1}{\max(2,q)}$, these results are optimal in the following sense: for all p > 1, there exists a ![]() $\mathcal{U}$-frequently hypercyclic function for Tα such that, for every
$\mathcal{U}$-frequently hypercyclic function for Tα such that, for every ![]() $0 \lt r \lt 1$,
$0 \lt r \lt 1$,
 \begin{equation*}M_p(f,r)\lesssim\left\{\begin{array}{ll} (1-r)^{\alpha-\frac{1}{\max(2,q)}}& \hbox{ if } \alpha \lt \frac{1}{\max(2,q)}\\1&
\hbox{ if } \alpha \gt \frac{1}{\max(2,q)}.\end{array}\right.\end{equation*}
\begin{equation*}M_p(f,r)\lesssim\left\{\begin{array}{ll} (1-r)^{\alpha-\frac{1}{\max(2,q)}}& \hbox{ if } \alpha \lt \frac{1}{\max(2,q)}\\1&
\hbox{ if } \alpha \gt \frac{1}{\max(2,q)}.\end{array}\right.\end{equation*}Remark 3.4. For the critical value  $\alpha=\frac{1}{\max(2,q)}$, the optimality of the rate of growth in this theorem will be obtained in Theorem 5.7 further in the paper.
$\alpha=\frac{1}{\max(2,q)}$, the optimality of the rate of growth in this theorem will be obtained in Theorem 5.7 further in the paper.
Proof. We write  $f=\sum\limits_{k\geq 0}\frac{a_k}{(k+1)^{\alpha}}z^k.$ Since f is
$f=\sum\limits_{k\geq 0}\frac{a_k}{(k+1)^{\alpha}}z^k.$ Since f is ![]() $\mathcal{U}$-frequently hypercyclic there exists an increasing sub-sequence
$\mathcal{U}$-frequently hypercyclic there exists an increasing sub-sequence ![]() $(n_k)\subset\mathbb{N}$ with positive upper density such that for all
$(n_k)\subset\mathbb{N}$ with positive upper density such that for all ![]() $k\geq 1$
$k\geq 1$
 \begin{equation*}\vert T_{\alpha}^{n_k}f(0)-3/2\vert=\vert a_{n_k}-3/2\vert \lt 1/2.\end{equation*}
\begin{equation*}\vert T_{\alpha}^{n_k}f(0)-3/2\vert=\vert a_{n_k}-3/2\vert \lt 1/2.\end{equation*} We get, for all ![]() $k\geq 1$,
$k\geq 1$, ![]() $\vert a_{n_k}\vert \geq 1$. Set
$\vert a_{n_k}\vert \geq 1$. Set ![]() $I=\{n_k\ :\ k\geq 1\}$ and for all
$I=\{n_k\ :\ k\geq 1\}$ and for all ![]() $N\geq 1$,
$N\geq 1$, ![]() $I_N=I\cap\{1,\dots,N\}$. The hypothesis
$I_N=I\cap\{1,\dots,N\}$. The hypothesis ![]() $\overline{d}(I) \gt 0$ ensures that there exist
$\overline{d}(I) \gt 0$ ensures that there exist ![]() $0 \lt C \lt 1$ and an increasing sequence
$0 \lt C \lt 1$ and an increasing sequence ![]() $(N_l)$ of positive integers such that
$(N_l)$ of positive integers such that
Up to take a sub-sequence, we can also assume that
Let us consider, for all ![]() $l\geq 1$,
$l\geq 1$,  $1-\frac{1}{N_l-1}\leq r_l \lt 1-\frac{1}{N_l}$. Thus, we derive
$1-\frac{1}{N_l-1}\leq r_l \lt 1-\frac{1}{N_l}$. Thus, we derive
 \begin{equation}
N_l-1\leq \frac{1}{1-r_l} \lt N_l.
\end{equation}
\begin{equation}
N_l-1\leq \frac{1}{1-r_l} \lt N_l.
\end{equation}(1) Case
 $2\leq p\leq\infty$:
$2\leq p\leq\infty$:Jensen’s inequality and Parseval’s Theorem give
 \begin{equation*}[M_p(f,r_l)]^2\geq [M_2(f,r_l)]^2=\sum_{k\geq 0}\frac{\vert a_k\vert^2}{(k+1)^{2\alpha}}r_l^{2k}\geq \sum_{k=1}^{N_l}\frac{\vert a_k\vert^2}{(k+1)^{2\alpha}}r_l^{2k}.\end{equation*}
\begin{equation*}[M_p(f,r_l)]^2\geq [M_2(f,r_l)]^2=\sum_{k\geq 0}\frac{\vert a_k\vert^2}{(k+1)^{2\alpha}}r_l^{2k}\geq \sum_{k=1}^{N_l}\frac{\vert a_k\vert^2}{(k+1)^{2\alpha}}r_l^{2k}.\end{equation*}Thus, we deduce
(4) \begin{equation}
[M_p(f,r_l)]^2\geq \displaystyle\left(1-\frac{1}{N_l-1}\right)^{2N_l}\sum_{k=1}^{N_l}\frac{\vert a_k\vert^2}{(k+1)^{2\alpha}} \gt rsim \sum_{k=1}^{N_l}\frac{\vert a_k\vert^2}{(k+1)^{2\alpha}}
\end{equation}
\begin{equation}
[M_p(f,r_l)]^2\geq \displaystyle\left(1-\frac{1}{N_l-1}\right)^{2N_l}\sum_{k=1}^{N_l}\frac{\vert a_k\vert^2}{(k+1)^{2\alpha}} \gt rsim \sum_{k=1}^{N_l}\frac{\vert a_k\vert^2}{(k+1)^{2\alpha}}
\end{equation}and using the inequality
 $\vert a_k\vert\geq 1$ for
$\vert a_k\vert\geq 1$ for  $k\in I_{N_l}$
(5)
$k\in I_{N_l}$
(5) \begin{equation}[M_p(f,r_l)]^2 \gt rsim \sum_{k\in I_{N_l}}\frac{1}{(k+1)^{2\alpha}}.
\end{equation}
\begin{equation}[M_p(f,r_l)]^2 \gt rsim \sum_{k\in I_{N_l}}\frac{1}{(k+1)^{2\alpha}}.
\end{equation}(a) Case
 $\alpha \leq 0$: Combining Lemma 3.1 with (1), (3) and (5) we get
$\alpha \leq 0$: Combining Lemma 3.1 with (1), (3) and (5) we get
 \begin{equation*}[M_p(f,r_l)]^2 \gt rsim N_l^{-2\alpha +1}\geq (1-r_l)^{2\alpha-1}.\end{equation*}
\begin{equation*}[M_p(f,r_l)]^2 \gt rsim N_l^{-2\alpha +1}\geq (1-r_l)^{2\alpha-1}.\end{equation*}(b) Case
 $0 \lt \alpha \lt 1/2$: using (5) and Lemma 3.1 again, we get
$0 \lt \alpha \lt 1/2$: using (5) and Lemma 3.1 again, we get
 \begin{equation*}[M_p(f,r_l)]^2 \geq \frac{(2+N_l)^{-2\alpha+1}}{-2\alpha+1}\left(1-\left( 1-\frac{\#I_{N_l}}{2+N_l}\right)^{-2\alpha+1}\right).\end{equation*}
\begin{equation*}[M_p(f,r_l)]^2 \geq \frac{(2+N_l)^{-2\alpha+1}}{-2\alpha+1}\left(1-\left( 1-\frac{\#I_{N_l}}{2+N_l}\right)^{-2\alpha+1}\right).\end{equation*}The inequality (1) ensures
 $\left( 1-\frac{\#I_{N_l}}{2+N_l}\right)^{-2\alpha+1}\leq\left( 1-\frac{CN_l}{2+N_l}\right)^{-2\alpha+1}$. We deduce by using (1) and (3) again
$\left( 1-\frac{\#I_{N_l}}{2+N_l}\right)^{-2\alpha+1}\leq\left( 1-\frac{CN_l}{2+N_l}\right)^{-2\alpha+1}$. We deduce by using (1) and (3) again
 \begin{equation*}[M_p(f,r_l)]^2 \gt rsim N_l^{-2\alpha +1}\geq (1-r_l)^{2\alpha-1}.\end{equation*}
\begin{equation*}[M_p(f,r_l)]^2 \gt rsim N_l^{-2\alpha +1}\geq (1-r_l)^{2\alpha-1}.\end{equation*}Hence we conclude
 \begin{equation*}\limsup_{r\rightarrow 1^{-}}\left((1-r)^{\frac{1}{2}-\alpha}M_p(f,r)\right) \gt 0.\end{equation*}
\begin{equation*}\limsup_{r\rightarrow 1^{-}}\left((1-r)^{\frac{1}{2}-\alpha}M_p(f,r)\right) \gt 0.\end{equation*}(c) Case
 $\alpha=\frac{1}{2}$: using (4), we know
$\alpha=\frac{1}{2}$: using (4), we know
 \begin{equation*} [M_p(f,r_l)]^2 \gt rsim \sum_{j=1}^{l}\sum_{k=1+N_{j-1}}^{N_j}\frac{\vert a_k\vert^2}{k+1}.\end{equation*}
\begin{equation*} [M_p(f,r_l)]^2 \gt rsim \sum_{j=1}^{l}\sum_{k=1+N_{j-1}}^{N_j}\frac{\vert a_k\vert^2}{k+1}.\end{equation*}Hence applying Lemma 3.2 with
 $u_k=\vert a_k\vert^2$ and
$u_k=\vert a_k\vert^2$ and  $v_k=1/(k+1)$, we get, for all
$v_k=1/(k+1)$, we get, for all  $l\geq 1$,
$l\geq 1$,
 \begin{equation*}[M_p(f,r_l)]^2 \gt rsim \frac{S_{N_l}}{N_l+1}-\frac{S_{N_0}}{N_0+1}+\sum_{j=1}^l S_{N_{j-1}}\left(\frac{1}{N_{j-1}+1}-\frac{1}{N_j+1}\right).\end{equation*}
\begin{equation*}[M_p(f,r_l)]^2 \gt rsim \frac{S_{N_l}}{N_l+1}-\frac{S_{N_0}}{N_0+1}+\sum_{j=1}^l S_{N_{j-1}}\left(\frac{1}{N_{j-1}+1}-\frac{1}{N_j+1}\right).\end{equation*}By construction
 $S_{N_{k-1}} \gt rsim N_{k-1}+1$. Thus taking into account (2) we derive, for all
$S_{N_{k-1}} \gt rsim N_{k-1}+1$. Thus taking into account (2) we derive, for all  $l\geq 1$, the inequality
$l\geq 1$, the inequality
 \begin{equation*} [M_p(f,r_l)]^2 \gt rsim \sum_{j=1}^l\left(1-\frac{N_{j-1}+1}{N_j+1}\right) \gt rsim l,\end{equation*}
\begin{equation*} [M_p(f,r_l)]^2 \gt rsim \sum_{j=1}^l\left(1-\frac{N_{j-1}+1}{N_j+1}\right) \gt rsim l,\end{equation*}that allows to obtain
 $\displaystyle\limsup_{r\rightarrow 1^{-}}M_p(f,r)=+\infty$.
$\displaystyle\limsup_{r\rightarrow 1^{-}}M_p(f,r)=+\infty$.
(2) Case
 $1 \lt p \lt 2$:
$1 \lt p \lt 2$:From Hausdorff–Young inequality (see [Reference Duren9]) we get
 \begin{equation*}[M_p(f,r_l)]^q\geq \sum_{k\geq 0}\frac{\vert a_k\vert^q}{(k+1)^{q\alpha}}r_l^{qk}
\geq \sum_{k=1}^{N_l}\frac{\vert a_k\vert^q}{(k+1)^{q\alpha}}r_l^{qk}.\end{equation*}
\begin{equation*}[M_p(f,r_l)]^q\geq \sum_{k\geq 0}\frac{\vert a_k\vert^q}{(k+1)^{q\alpha}}r_l^{qk}
\geq \sum_{k=1}^{N_l}\frac{\vert a_k\vert^q}{(k+1)^{q\alpha}}r_l^{qk}.\end{equation*}Thus, we deduce
 \begin{equation*}[M_p(f,r_l)]^q\geq \displaystyle\left(1-\frac{1}{N_l-1}\right)^{qN_l}\sum_{k=1}^{N_l}\frac{\vert a_k\vert^q}{(k+1)^{q\alpha}} \gt rsim \sum_{k=1}^{N_l}\frac{\vert a_k\vert^q}{(k+1)^{q\alpha}}.\end{equation*}
\begin{equation*}[M_p(f,r_l)]^q\geq \displaystyle\left(1-\frac{1}{N_l-1}\right)^{qN_l}\sum_{k=1}^{N_l}\frac{\vert a_k\vert^q}{(k+1)^{q\alpha}} \gt rsim \sum_{k=1}^{N_l}\frac{\vert a_k\vert^q}{(k+1)^{q\alpha}}.\end{equation*}Using the same strategy as in the case
 $2\leq p\leq\infty$, we obtain,
$2\leq p\leq\infty$, we obtain,
 \begin{align*}&\limsup_{r\rightarrow 1^{-}}\left((1-r)^{\frac{1}{q}-\alpha}M_p(f,r)\right) \gt 0,\quad \hbox{ for } \alpha \lt 1/q,\\
&\limsup_{r\rightarrow 1^{-}}\left(M_p(f,r)\right)=+\infty,\quad \hbox{ for } \alpha=1/q.
\end{align*}
\begin{align*}&\limsup_{r\rightarrow 1^{-}}\left((1-r)^{\frac{1}{q}-\alpha}M_p(f,r)\right) \gt 0,\quad \hbox{ for } \alpha \lt 1/q,\\
&\limsup_{r\rightarrow 1^{-}}\left(M_p(f,r)\right)=+\infty,\quad \hbox{ for } \alpha=1/q.
\end{align*}
Moreover, since a ![]() $\mathcal{U}$-frequently hypercyclic function is necessarily hypercyclic, the assertion for the case
$\mathcal{U}$-frequently hypercyclic function is necessarily hypercyclic, the assertion for the case  $\alpha \gt \frac{1}{\max(2,q)}$ of the statement is given by the assertion (2b) of Theorem 2.2.
$\alpha \gt \frac{1}{\max(2,q)}$ of the statement is given by the assertion (2b) of Theorem 2.2.
Finally, since a frequently hypercyclic function is necessarily ![]() $\mathcal{U}$-frequently hypercyclic, Theorem 1.1 ensures that the estimates we have proved are optimal when
$\mathcal{U}$-frequently hypercyclic, Theorem 1.1 ensures that the estimates we have proved are optimal when  $\alpha\ne\frac{1}{\max(2,q)}$.
$\alpha\ne\frac{1}{\max(2,q)}$.
Now we deal with the case p = 1.
Theorem 3.5. Let f be a ![]() $\mathcal{U}$-frequently hypercyclic function for the operator Tα. Then, the following estimates hold
$\mathcal{U}$-frequently hypercyclic function for the operator Tα. Then, the following estimates hold
 \begin{align*}&\limsup_{r\rightarrow 1^{-}}\left((1-r)^{-\alpha}M_1(f,r)\right)=+\infty ,\quad\hbox{ if }\alpha\leq 0,\\
&\limsup_{r\rightarrow 1^{-}}M_1(f,r) \gt 0,\quad\hbox{ if }\alpha \gt 0.
\end{align*}
\begin{align*}&\limsup_{r\rightarrow 1^{-}}\left((1-r)^{-\alpha}M_1(f,r)\right)=+\infty ,\quad\hbox{ if }\alpha\leq 0,\\
&\limsup_{r\rightarrow 1^{-}}M_1(f,r) \gt 0,\quad\hbox{ if }\alpha \gt 0.
\end{align*} For ![]() $\alpha\ne 0$, these results are optimal in the following sense: for any positive integer
$\alpha\ne 0$, these results are optimal in the following sense: for any positive integer ![]() $l\geq 1$, there exists a
$l\geq 1$, there exists a ![]() $\mathcal{U}$-frequently hypercyclic function for the operator Tα such that for every
$\mathcal{U}$-frequently hypercyclic function for the operator Tα such that for every ![]() $0 \lt r \lt 1$ sufficiently large
$0 \lt r \lt 1$ sufficiently large
 \begin{equation*}M_1(f,r)\lesssim\left\{\begin{array}{ll} (1-r)^{\alpha}\log_l(-\log(1-r))& \hbox{ if } \alpha \lt 0\\1&
\hbox{ if } \alpha \gt 0.\end{array}\right.\end{equation*}
\begin{equation*}M_1(f,r)\lesssim\left\{\begin{array}{ll} (1-r)^{\alpha}\log_l(-\log(1-r))& \hbox{ if } \alpha \lt 0\\1&
\hbox{ if } \alpha \gt 0.\end{array}\right.\end{equation*}Proof. First since a ![]() $\mathcal{U}$-frequently hypercyclic function is necessarily hypercyclic, the assertions (1b) and (2b) of Theorem 2.2 ensure that,
$\mathcal{U}$-frequently hypercyclic function is necessarily hypercyclic, the assertions (1b) and (2b) of Theorem 2.2 ensure that,
 \begin{equation*}\limsup_{r\rightarrow 1^{-}}\left((1-r)^{-\alpha}M_1(f,r)\right)=+\infty,\hbox{ if }\alpha\leq 0,\quad\hbox{ and }\quad\limsup_{r\rightarrow 1^{-}}M_1(f,r) \gt 0,\hbox{ if }\alpha \gt 0.\end{equation*}
\begin{equation*}\limsup_{r\rightarrow 1^{-}}\left((1-r)^{-\alpha}M_1(f,r)\right)=+\infty,\hbox{ if }\alpha\leq 0,\quad\hbox{ and }\quad\limsup_{r\rightarrow 1^{-}}M_1(f,r) \gt 0,\hbox{ if }\alpha \gt 0.\end{equation*} Moreover, since a frequently hypercyclic function is necessarily ![]() $\mathcal{U}$-frequently hypercyclic, Theorem 1.2 shows that the previous estimates are optimal when
$\mathcal{U}$-frequently hypercyclic, Theorem 1.2 shows that the previous estimates are optimal when ![]() $\alpha\ne 0$.
$\alpha\ne 0$.
4. Between  $\mathcal{U}$-frequent hypercyclicity and hypercyclicity
$\mathcal{U}$-frequent hypercyclicity and hypercyclicity
Let ![]() $1\leq p\leq\infty$. In view of Theorems 1.1, 1.2, 3.3 and 3.5, the critical exponent related to the Lp growth of frequently hypercyclic functions for Tα is the same as that related to the Lp growth of
$1\leq p\leq\infty$. In view of Theorems 1.1, 1.2, 3.3 and 3.5, the critical exponent related to the Lp growth of frequently hypercyclic functions for Tα is the same as that related to the Lp growth of ![]() $\mathcal{U}$-frequently hypercyclic functions. It is equal to
$\mathcal{U}$-frequently hypercyclic functions. It is equal to  $\frac{1}{\max(2,q)}$. Nevertheless, this critical exponent is always equal to 0 in the case of hypercyclic functions, and hence it does not depend on p. In this section, we are interested in what happens between
$\frac{1}{\max(2,q)}$. Nevertheless, this critical exponent is always equal to 0 in the case of hypercyclic functions, and hence it does not depend on p. In this section, we are interested in what happens between ![]() $\mathcal{U}$-frequent hypercyclicity and hypercyclicity. In particular, when p > 1, we will try to understand why and how the critical exponent goes from
$\mathcal{U}$-frequent hypercyclicity and hypercyclicity. In particular, when p > 1, we will try to understand why and how the critical exponent goes from  $\frac{1}{\max(2,q)}$ in the case of Lp-norm of
$\frac{1}{\max(2,q)}$ in the case of Lp-norm of ![]() $\mathcal{U}$-frequently hypercyclic functions to 0 for the Lp-norm of hypercyclic functions. To do this, we introduce intermediate notions of linear dynamics that link
$\mathcal{U}$-frequently hypercyclic functions to 0 for the Lp-norm of hypercyclic functions. To do this, we introduce intermediate notions of linear dynamics that link ![]() $\mathcal{U}$-frequent hypercyclicity and hypercyclicity. First of all, we need some definitions and results.
$\mathcal{U}$-frequent hypercyclicity and hypercyclicity. First of all, we need some definitions and results.
4.1. Some weighted densities
First, we introduce a refined notion of upper densities.
Definition 4.1. Let ![]() $\beta=(\beta_n)$ be a non-decreasing sequence of positive real numbers tending to infinity. For a subset
$\beta=(\beta_n)$ be a non-decreasing sequence of positive real numbers tending to infinity. For a subset ![]() $E\subset \mathbb{N}$, its upper β-density is given by
$E\subset \mathbb{N}$, its upper β-density is given by
 \begin{equation*}\overline{d}_{\beta}(E)=\limsup_{n\rightarrow +\infty}\frac{\sum_{k=1; k\in E}^n \beta_k}{\sum_{k=1}^n \beta_k}.\end{equation*}
\begin{equation*}\overline{d}_{\beta}(E)=\limsup_{n\rightarrow +\infty}\frac{\sum_{k=1; k\in E}^n \beta_k}{\sum_{k=1}^n \beta_k}.\end{equation*} These quantities enjoy all the classical properties of densities (see [Reference Ernst and Mouze12, Reference Freedman and Sember14]) and allow to define dynamical notions of the same nature as hypercyclicity or ![]() $\mathcal {U}$-frequent hypercyclicity.
$\mathcal {U}$-frequent hypercyclicity.
Definition 4.2. Let ![]() $\beta=(\beta_n)$ be a non-decreasing sequence of positive real numbers tending to infinity and let E be a subset of
$\beta=(\beta_n)$ be a non-decreasing sequence of positive real numbers tending to infinity and let E be a subset of ![]() $\mathbb{N}$. An operator
$\mathbb{N}$. An operator ![]() $T:X\rightarrow X$, where X is a Fréchet space, is said to be
$T:X\rightarrow X$, where X is a Fréchet space, is said to be ![]() $\mathcal{U}_{\beta}$-frequently hypercyclic if there exists
$\mathcal{U}_{\beta}$-frequently hypercyclic if there exists ![]() $x\in X$ such that for every non-empty open subset
$x\in X$ such that for every non-empty open subset ![]() $U\subset X$,
$U\subset X$,
In the sequel, we are interested in densities given by the weighted sequence denoted by β γ and defined by  $\beta^{\gamma}=(e^{n^{\gamma}})$, where γ is a parameter with
$\beta^{\gamma}=(e^{n^{\gamma}})$, where γ is a parameter with ![]() $0\leq \gamma\leq 1.$ First of all, let us notice that:
$0\leq \gamma\leq 1.$ First of all, let us notice that:
(i) the density
 $\overline{d}_{\beta^{0}}$ coincides with the upper natural density
$\overline{d}_{\beta^{0}}$ coincides with the upper natural density  $\overline{d}$,
$\overline{d}$,(ii) for any subset
 $E\subset\mathbb{N}$,
$E\subset\mathbb{N}$,  $\overline{d}_{\beta^{1}}(E) \gt 0$ if and only if E is infinite.
$\overline{d}_{\beta^{1}}(E) \gt 0$ if and only if E is infinite.
Moreover for ![]() $0 \lt \gamma \lt 1$ an integral comparison test leads to the estimate
$0 \lt \gamma \lt 1$ an integral comparison test leads to the estimate
 \begin{equation*}\displaystyle \sum_{k=1}^n e^{k^{\gamma}}\sim \frac{n^{1-\gamma}}{\gamma}e^{n^{\gamma}},\quad\hbox{ as }n\hbox{ tends to infinity,}\end{equation*}
\begin{equation*}\displaystyle \sum_{k=1}^n e^{k^{\gamma}}\sim \frac{n^{1-\gamma}}{\gamma}e^{n^{\gamma}},\quad\hbox{ as }n\hbox{ tends to infinity,}\end{equation*} that we will use regularly in the rest of the paper. In addition, according to Lemma 2.8 of [Reference Ernst and Mouze12], the following inequalities hold. For the sake of clarity, let us mention that the density ![]() $\overline{d}_{\beta^{\gamma}}$ is denoted by
$\overline{d}_{\beta^{\gamma}}$ is denoted by  $\overline{d}_{A_{\gamma}}$ in [Reference Ernst and Mouze12].
$\overline{d}_{A_{\gamma}}$ in [Reference Ernst and Mouze12].
Lemma 4.3. For any ![]() $0\leq \gamma_1\leq \gamma_2\leq 1$ and for any subset E of
$0\leq \gamma_1\leq \gamma_2\leq 1$ and for any subset E of ![]() $\mathbb{N}$, we have
$\mathbb{N}$, we have
 \begin{equation*}\overline{d}(E)\leq \overline{d}_{\beta^{\gamma_1}}(E)\leq \overline{d}_{\beta^{\gamma_2}}(E)\leq \overline{d}_{\beta^{1}}(E).\end{equation*}
\begin{equation*}\overline{d}(E)\leq \overline{d}_{\beta^{\gamma_1}}(E)\leq \overline{d}_{\beta^{\gamma_2}}(E)\leq \overline{d}_{\beta^{1}}(E).\end{equation*} Therefore, the densities ![]() $\overline{d}_{\beta^{\gamma}}$ can give very different notions of dynamics that are intermediate between
$\overline{d}_{\beta^{\gamma}}$ can give very different notions of dynamics that are intermediate between ![]() $\mathcal{U}$-frequent hypercyclicity and hypercyclicity. In particular, the following lemma holds.
$\mathcal{U}$-frequent hypercyclicity and hypercyclicity. In particular, the following lemma holds.
Lemma 4.4. Let ![]() $0 \lt \gamma\leq 1.$ There exists a subset
$0 \lt \gamma\leq 1.$ There exists a subset ![]() $E_{\gamma}\subset\mathbb{N}$ such that, for any
$E_{\gamma}\subset\mathbb{N}$ such that, for any ![]() $0\leq \gamma' \lt \gamma\leq 1$,
$0\leq \gamma' \lt \gamma\leq 1$, ![]() $\overline{d}_{\beta^{\gamma}}(E) \gt 0$ and
$\overline{d}_{\beta^{\gamma}}(E) \gt 0$ and  $\overline{d}_{\beta^{\gamma'}}(E)=0$.
$\overline{d}_{\beta^{\gamma'}}(E)=0$.
Proof. First observe that, for all ![]() $0 \lt t \lt 1$,
$0 \lt t \lt 1$,
 \begin{equation}
\frac{\sum_{k=1}^{2^{n-1}}\mathrm{e}^{k^{t}}}{\sum_{k=1}^{2^{n}}\mathrm{e}^{k^{t}}}\sim 2^{-(1-t)}\mathrm{e}^{-2^{nt}(1-2^{-t})}\rightarrow 0\hbox{ as }n\rightarrow +\infty.
\end{equation}
\begin{equation}
\frac{\sum_{k=1}^{2^{n-1}}\mathrm{e}^{k^{t}}}{\sum_{k=1}^{2^{n}}\mathrm{e}^{k^{t}}}\sim 2^{-(1-t)}\mathrm{e}^{-2^{nt}(1-2^{-t})}\rightarrow 0\hbox{ as }n\rightarrow +\infty.
\end{equation} Let ![]() $\gamma\ne 1$. Set
$\gamma\ne 1$. Set  $E_{\gamma}=\mathbb{N}\bigcap\left(\bigcup_{n\geq \lfloor \frac{1}{\gamma}\rfloor +1}\left[2^{n}-\lfloor2^{n(1-\gamma)}\rfloor;2^n\right]\right)$. Clearly, for all n large enough, we have
$E_{\gamma}=\mathbb{N}\bigcap\left(\bigcup_{n\geq \lfloor \frac{1}{\gamma}\rfloor +1}\left[2^{n}-\lfloor2^{n(1-\gamma)}\rfloor;2^n\right]\right)$. Clearly, for all n large enough, we have
 \begin{equation}
\sum_{k=1;k\in E_{\gamma}}^{2^n}\mathrm{e}^{k^{\gamma}}\geq \sum_{k=2^n-\lfloor2^{n(1-\gamma)}\rfloor +1}^{2^{n}}\mathrm{e}^{k^{\gamma}}.
\end{equation}
\begin{equation}
\sum_{k=1;k\in E_{\gamma}}^{2^n}\mathrm{e}^{k^{\gamma}}\geq \sum_{k=2^n-\lfloor2^{n(1-\gamma)}\rfloor +1}^{2^{n}}\mathrm{e}^{k^{\gamma}}.
\end{equation}Moreover, we get
 \begin{equation*}\frac{\sum_{k=2^n-\lfloor2^{n(1-\gamma)}\rfloor +1}^{2^{n}}\mathrm{e}^{k^{\gamma}}}{\sum_{k=1}^{2^{n}}\mathrm{e}^{k^{\gamma}}}=1-\frac{\sum_{k=1}^{2^n-\lfloor2^{n(1-\gamma)}\rfloor}\mathrm{e}^{k^{\gamma}}}{\sum_{k=1}^{2^{n}}\mathrm{e}^{k^{\gamma}}}.\end{equation*}
\begin{equation*}\frac{\sum_{k=2^n-\lfloor2^{n(1-\gamma)}\rfloor +1}^{2^{n}}\mathrm{e}^{k^{\gamma}}}{\sum_{k=1}^{2^{n}}\mathrm{e}^{k^{\gamma}}}=1-\frac{\sum_{k=1}^{2^n-\lfloor2^{n(1-\gamma)}\rfloor}\mathrm{e}^{k^{\gamma}}}{\sum_{k=1}^{2^{n}}\mathrm{e}^{k^{\gamma}}}.\end{equation*}But we compute
 \begin{equation*}\frac{\sum_{k=1}^{2^n-\lfloor2^{n(1-\gamma)}\rfloor}\mathrm{e}^{k^{\gamma}}}{\sum_{k=1}^{2^{n}}\mathrm{e}^{k^{\gamma}}}\sim \left(1-\frac{\lfloor 2^{n(1-\gamma)}\rfloor}{2^n}\right)^{1-\gamma}\mathrm{e}^{2^{n\gamma}((1-2^{-n}\lfloor 2^{n(1-\gamma)}\rfloor)^{\gamma}-1)}\rightarrow \mathrm{e}^{-\gamma}\hbox{ as }n\rightarrow +\infty.\end{equation*}
\begin{equation*}\frac{\sum_{k=1}^{2^n-\lfloor2^{n(1-\gamma)}\rfloor}\mathrm{e}^{k^{\gamma}}}{\sum_{k=1}^{2^{n}}\mathrm{e}^{k^{\gamma}}}\sim \left(1-\frac{\lfloor 2^{n(1-\gamma)}\rfloor}{2^n}\right)^{1-\gamma}\mathrm{e}^{2^{n\gamma}((1-2^{-n}\lfloor 2^{n(1-\gamma)}\rfloor)^{\gamma}-1)}\rightarrow \mathrm{e}^{-\gamma}\hbox{ as }n\rightarrow +\infty.\end{equation*}Taking into account (6) and (7), we deduce
Now let ![]() $0\leq \gamma' \lt \gamma\leq 1$. Clearly keeping in mind that
$0\leq \gamma' \lt \gamma\leq 1$. Clearly keeping in mind that
 \begin{equation*}\sum_{k=1;k\in E_{\gamma}}^{2^n}\mathrm{e}^{k^{\gamma'}}\leq \sum_{k=1}^{2^{n-1}}\mathrm{e}^{k^{\gamma'}} + \sum_{k=2^n-\lfloor2^{n(1-\gamma)}\rfloor}^{2^{n}}\mathrm{e}^{k^{\gamma'}}\end{equation*}
\begin{equation*}\sum_{k=1;k\in E_{\gamma}}^{2^n}\mathrm{e}^{k^{\gamma'}}\leq \sum_{k=1}^{2^{n-1}}\mathrm{e}^{k^{\gamma'}} + \sum_{k=2^n-\lfloor2^{n(1-\gamma)}\rfloor}^{2^{n}}\mathrm{e}^{k^{\gamma'}}\end{equation*} and by using both ![]() $\gamma'-\gamma \lt 0$, the estimate
$\gamma'-\gamma \lt 0$, the estimate
 \begin{equation*}\frac{\sum_{k=1}^{2^n-\lfloor2^{n(1-\gamma)}\rfloor}\mathrm{e}^{k^{\gamma'}}}{\sum_{k=1}^{2^{n}}\mathrm{e}^{k^{\gamma'}}}\sim \left(1-\frac{\lfloor 2^{n(1-\gamma)}\rfloor}{2^n}\right)^{1-\gamma'}\mathrm{e}^{2^{n\gamma'}((1-2^{-n}\lfloor 2^{n(1-\gamma)}\rfloor)^{\gamma'}-1)}\rightarrow 1\hbox{ as }n\rightarrow +\infty,\end{equation*}
\begin{equation*}\frac{\sum_{k=1}^{2^n-\lfloor2^{n(1-\gamma)}\rfloor}\mathrm{e}^{k^{\gamma'}}}{\sum_{k=1}^{2^{n}}\mathrm{e}^{k^{\gamma'}}}\sim \left(1-\frac{\lfloor 2^{n(1-\gamma)}\rfloor}{2^n}\right)^{1-\gamma'}\mathrm{e}^{2^{n\gamma'}((1-2^{-n}\lfloor 2^{n(1-\gamma)}\rfloor)^{\gamma'}-1)}\rightarrow 1\hbox{ as }n\rightarrow +\infty,\end{equation*}and (6) we derive
 \begin{equation*}\overline{d}_{\beta^{\gamma'}}(E_{\gamma})=0.\end{equation*}
\begin{equation*}\overline{d}_{\beta^{\gamma'}}(E_{\gamma})=0.\end{equation*} Finally, for γ = 1, the lemma is easy to establish since a subset ![]() $E\subset\mathbb{N}$ satisfies
$E\subset\mathbb{N}$ satisfies ![]() $d_{\beta^1}(E) \gt 0$ if and only if E is infinite. This finishes the proof.
$d_{\beta^1}(E) \gt 0$ if and only if E is infinite. This finishes the proof.
In some sense, the densities ![]() $\underline{d}_{\beta^\gamma}$,
$\underline{d}_{\beta^\gamma}$, ![]() $0\leq\gamma\leq 1$, will us allow to interpolate the behaviour of hypercyclic vectors between
$0\leq\gamma\leq 1$, will us allow to interpolate the behaviour of hypercyclic vectors between ![]() $\mathcal{U}$-frequent hypercyclicity and hypercyclicity.
$\mathcal{U}$-frequent hypercyclicity and hypercyclicity.
4.2. Rate of growth of  $\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic functions
$\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic functions
First, we deal with the case p > 1. We will discuss the case p = 1 at the end of the section. We are ready to state the result that highlights both the continuous variation of the critical exponent and that of the growth (in term of Lp averages) of a hypercyclic function for Tα according to the frequency of visits of non-empty open subsets by its orbit under the action of Tα.
Theorem 4.5. Let ![]() $0 \lt \gamma \lt 1$ and
$0 \lt \gamma \lt 1$ and ![]() $1 \lt p\leq\infty$. Let f be a
$1 \lt p\leq\infty$. Let f be a ![]() $\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic function for the operator Tα. Then, the following hold
$\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic function for the operator Tα. Then, the following hold
 \begin{align*}
&\hbox{ if } \alpha \lt \frac{1-\gamma}{\max(2,q)},\quad \limsup_{r\rightarrow 1^{-}}\left(\left[1-r\right]^{\frac{1-\gamma}{\max(2,q)}-\alpha}M_p(f,r)\right) \gt 0,\\
&\hbox{ if } \alpha=\frac{1-\gamma}{\max(2,q)} ,\quad \limsup_{r\rightarrow 1^{-}}\left(M_p(f,r)\right)=+\infty,\\
&\hbox{ if } \alpha \gt \frac{1-\gamma}{\max(2,q)} ,\quad \limsup_{r\rightarrow 1^{-}}\left(M_p(f,r)\right) \gt 0.
\end{align*}
\begin{align*}
&\hbox{ if } \alpha \lt \frac{1-\gamma}{\max(2,q)},\quad \limsup_{r\rightarrow 1^{-}}\left(\left[1-r\right]^{\frac{1-\gamma}{\max(2,q)}-\alpha}M_p(f,r)\right) \gt 0,\\
&\hbox{ if } \alpha=\frac{1-\gamma}{\max(2,q)} ,\quad \limsup_{r\rightarrow 1^{-}}\left(M_p(f,r)\right)=+\infty,\\
&\hbox{ if } \alpha \gt \frac{1-\gamma}{\max(2,q)} ,\quad \limsup_{r\rightarrow 1^{-}}\left(M_p(f,r)\right) \gt 0.
\end{align*}Proof. Let f be a ![]() $\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic function for Tα. We write
$\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic function for Tα. We write  $f=\sum\limits_{k\geq 0}\frac{a_k}{(k+1)^{\alpha}}z^k.$ Since f is
$f=\sum\limits_{k\geq 0}\frac{a_k}{(k+1)^{\alpha}}z^k.$ Since f is ![]() $\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic, there exists an increasing sub-sequence
$\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic, there exists an increasing sub-sequence ![]() $(n_k)\subset\mathbb{N}$ with positive upper β γ-density such that, for all
$(n_k)\subset\mathbb{N}$ with positive upper β γ-density such that, for all ![]() $k\geq 1$,
$k\geq 1$,
 \begin{equation*}\vert T_{\alpha}^{n_k}f(0)-3/2\vert=\vert a_{n_k}-3/2\vert \lt 1/2.\end{equation*}
\begin{equation*}\vert T_{\alpha}^{n_k}f(0)-3/2\vert=\vert a_{n_k}-3/2\vert \lt 1/2.\end{equation*} We get, for all ![]() $k\geq 1$,
$k\geq 1$, ![]() $\vert a_{n_k}\vert \geq 1$. Set
$\vert a_{n_k}\vert \geq 1$. Set ![]() $I=\{n_k\ :\ k\geq 1\}$ and for all
$I=\{n_k\ :\ k\geq 1\}$ and for all ![]() $N\geq 1$,
$N\geq 1$, ![]() $I_N=I\cap\{1,\dots,N\}$. The hypothesis
$I_N=I\cap\{1,\dots,N\}$. The hypothesis ![]() $\overline{d}_{\beta^{\gamma}}(I) \gt 0$ ensures that there exist
$\overline{d}_{\beta^{\gamma}}(I) \gt 0$ ensures that there exist ![]() $0 \lt C \lt 1$ and an increasing sequence
$0 \lt C \lt 1$ and an increasing sequence ![]() $(N_l)$ of positive integers such that
$(N_l)$ of positive integers such that
 \begin{equation}
\sum_{k\in I_{N_l}}\mathrm{e}^{k^{\gamma}} \geq C \frac{N_l^{1-\gamma}}{\gamma}\mathrm{e}^{N_l^{\gamma}}.
\end{equation}
\begin{equation}
\sum_{k\in I_{N_l}}\mathrm{e}^{k^{\gamma}} \geq C \frac{N_l^{1-\gamma}}{\gamma}\mathrm{e}^{N_l^{\gamma}}.
\end{equation}Up to take a sub-sequence, we can suppose that
Let us consider, for all ![]() $l\geq 1$, a sequence
$l\geq 1$, a sequence ![]() $(r_l)$ with
$(r_l)$ with  $1-\frac{1}{N_l-1}\leq r_l \lt 1-\frac{1}{N_l}$. Observe that
$1-\frac{1}{N_l-1}\leq r_l \lt 1-\frac{1}{N_l}$. Observe that
 \begin{equation}
N_l-1\leq \frac{1}{1-r_l} \lt N_l.
\end{equation}
\begin{equation}
N_l-1\leq \frac{1}{1-r_l} \lt N_l.
\end{equation}(1) Case
 $2\leq p\leq\infty$:
$2\leq p\leq\infty$:Jensen’s inequality and Parseval’s Theorem give
(11) \begin{equation}
[M_p(f,r_l)]^2\geq [M_2(f,r_l)]^2=\sum_{k\geq 0}\frac{\vert a_k\vert^2}{(k+1)^{2\alpha}}r_l^{2k}
\geq \sum_{k=1}^{N_l}\frac{\vert a_k\vert^2}{(k+1)^{2\alpha}}r_l^{2k} \gt rsim \sum_{k=1}^{N_l}\frac{\vert a_k\vert^2}{(k+1)^{2\alpha}}.
\end{equation}
\begin{equation}
[M_p(f,r_l)]^2\geq [M_2(f,r_l)]^2=\sum_{k\geq 0}\frac{\vert a_k\vert^2}{(k+1)^{2\alpha}}r_l^{2k}
\geq \sum_{k=1}^{N_l}\frac{\vert a_k\vert^2}{(k+1)^{2\alpha}}r_l^{2k} \gt rsim \sum_{k=1}^{N_l}\frac{\vert a_k\vert^2}{(k+1)^{2\alpha}}.
\end{equation}Let us choose
 $j_0\in\mathbb{N}$ such that the function
$j_0\in\mathbb{N}$ such that the function  $t\mapsto (t+1)^{-2\alpha}e^{-t^{\gamma}}$ is decreasing for
$t\mapsto (t+1)^{-2\alpha}e^{-t^{\gamma}}$ is decreasing for  $t\geq N_{j_0}$. Thus, we can write, for all
$t\geq N_{j_0}$. Thus, we can write, for all  $l\geq j_0+1$,
$l\geq j_0+1$,
 \begin{equation*}[M_p(f,r_l)]^2 \gt rsim \sum_{j=1+j_0}^l\sum_{k=1+N_{j-1}}^{N_j}\frac{\vert a_k\vert^2}{(k+1)^{2\alpha}}\mathrm{e}^{k^{\gamma}}\mathrm{e}^{-k^{\gamma}}.\end{equation*}
\begin{equation*}[M_p(f,r_l)]^2 \gt rsim \sum_{j=1+j_0}^l\sum_{k=1+N_{j-1}}^{N_j}\frac{\vert a_k\vert^2}{(k+1)^{2\alpha}}\mathrm{e}^{k^{\gamma}}\mathrm{e}^{-k^{\gamma}}.\end{equation*}Then applying Lemma 3.2 with
 $u_k=\vert a_k\vert^2 \mathrm{e}^{k^{\gamma}}$, we get(12)
$u_k=\vert a_k\vert^2 \mathrm{e}^{k^{\gamma}}$, we get(12) \begin{equation}
\begin{array}{ll}\displaystyle[M_p(f,r_l)]^2 \gt rsim &\displaystyle S_{N_l}(N_l+1)^{-2\alpha}\mathrm{e}^{-N_{l}^{\gamma}}-\displaystyle S_{N_{j_0}}(N_{j_0}+1)^{-2\alpha}\mathrm{e}^{-N_{j_0}^{\gamma}}\\ &+\displaystyle\sum_{j=1+j_0}^l S_{N_{j-1}}\left((N_{j-1}+1)^{-2\alpha}\mathrm{e}^{-N_{j-1}^{\gamma}}-(N_{j}+1)^{-2\alpha}\mathrm{e}^{-N_{j}^{\gamma}}\right).\end{array}
\end{equation}
\begin{equation}
\begin{array}{ll}\displaystyle[M_p(f,r_l)]^2 \gt rsim &\displaystyle S_{N_l}(N_l+1)^{-2\alpha}\mathrm{e}^{-N_{l}^{\gamma}}-\displaystyle S_{N_{j_0}}(N_{j_0}+1)^{-2\alpha}\mathrm{e}^{-N_{j_0}^{\gamma}}\\ &+\displaystyle\sum_{j=1+j_0}^l S_{N_{j-1}}\left((N_{j-1}+1)^{-2\alpha}\mathrm{e}^{-N_{j-1}^{\gamma}}-(N_{j}+1)^{-2\alpha}\mathrm{e}^{-N_{j}^{\gamma}}\right).\end{array}
\end{equation}Since
 $S_{N_i}=\sum\limits_{k\leq N_i}\vert a_k\vert^2 \mathrm{e}^{k^{\gamma}}$, by construction and by (8), we get, for all
$S_{N_i}=\sum\limits_{k\leq N_i}\vert a_k\vert^2 \mathrm{e}^{k^{\gamma}}$, by construction and by (8), we get, for all  $i\geq 1$,(13)
$i\geq 1$,(13) \begin{equation}
S_{N_i}\geq \sum_{k\in I_{N_i}}\mathrm{e}^{k^{\gamma}} \gt rsim N_{i}^{1-\gamma}\mathrm{e}^{N_{i}^{\gamma}} \gt rsim (N_{i}^{1-\gamma}+1)\mathrm{e}^{N_{i}^{\gamma}}.
\end{equation}(14)
\begin{equation}
S_{N_i}\geq \sum_{k\in I_{N_i}}\mathrm{e}^{k^{\gamma}} \gt rsim N_{i}^{1-\gamma}\mathrm{e}^{N_{i}^{\gamma}} \gt rsim (N_{i}^{1-\gamma}+1)\mathrm{e}^{N_{i}^{\gamma}}.
\end{equation}(14) \begin{align}
\displaystyle[M_p(f,r_l)]^2 \gt rsim &\displaystyle (N_l+1)^{(1-\gamma)-2\alpha}\nonumber\\
&+\displaystyle\sum_{j=1+j_0}^l (N_{j-1}+1)^{1-\gamma}\mathrm{e}^{N_{j-1}^{\gamma}}\left((N_{j-1}+1)^{-2\alpha}\mathrm{e}^{-N_{j-1}^{\gamma}}\right.\nonumber\\
& \qquad \left.-(N_{j}+1)^{-2\alpha}\mathrm{e}^{-N_{j}^{\gamma}}\right).\end{align}
\begin{align}
\displaystyle[M_p(f,r_l)]^2 \gt rsim &\displaystyle (N_l+1)^{(1-\gamma)-2\alpha}\nonumber\\
&+\displaystyle\sum_{j=1+j_0}^l (N_{j-1}+1)^{1-\gamma}\mathrm{e}^{N_{j-1}^{\gamma}}\left((N_{j-1}+1)^{-2\alpha}\mathrm{e}^{-N_{j-1}^{\gamma}}\right.\nonumber\\
& \qquad \left.-(N_{j}+1)^{-2\alpha}\mathrm{e}^{-N_{j}^{\gamma}}\right).\end{align}(a) Case
 $\alpha \lt \frac{1-\gamma}{2}$: From (14), we get, for l large enough,
(15)
$\alpha \lt \frac{1-\gamma}{2}$: From (14), we get, for l large enough,
(15) \begin{equation}[M_p(f,r_l)]^2 \gt rsim \displaystyle (N_l+1)^{(1-\gamma)-2\alpha}.
\end{equation}
\begin{equation}[M_p(f,r_l)]^2 \gt rsim \displaystyle (N_l+1)^{(1-\gamma)-2\alpha}.
\end{equation}Thanks to (10) and (15), we deduce
 \begin{equation*}[M_p(f,r_l)]^2 \gt rsim (1-r_l)^{2\alpha-(1-\gamma)}.\end{equation*}
\begin{equation*}[M_p(f,r_l)]^2 \gt rsim (1-r_l)^{2\alpha-(1-\gamma)}.\end{equation*}Hence we conclude
 \begin{equation*}\limsup_{r\rightarrow 1^{-}}\left[(1-r)^{-\alpha+\frac{1-\gamma}{2}}M_p(f,r)\right] \gt 0.\end{equation*}
\begin{equation*}\limsup_{r\rightarrow 1^{-}}\left[(1-r)^{-\alpha+\frac{1-\gamma}{2}}M_p(f,r)\right] \gt 0.\end{equation*}(b) Case
 $\alpha = \frac{1-\gamma}{2}$: taking into consideration (14), we can write, for all
$\alpha = \frac{1-\gamma}{2}$: taking into consideration (14), we can write, for all  $l\geq 1+j_0$,
$l\geq 1+j_0$,
 \begin{equation*}\displaystyle[M_p(f,r_l)]^2 \gt rsim\displaystyle \sum_{j=1}^l \left(1-\left(\frac{N_{j-1}+1}{N_{j}+1}\right)^{1-\gamma}\mathrm{e}^{N_{j-1}^{\gamma}-N_{j}^{\gamma}}\right).\end{equation*}
\begin{equation*}\displaystyle[M_p(f,r_l)]^2 \gt rsim\displaystyle \sum_{j=1}^l \left(1-\left(\frac{N_{j-1}+1}{N_{j}+1}\right)^{1-\gamma}\mathrm{e}^{N_{j-1}^{\gamma}-N_{j}^{\gamma}}\right).\end{equation*}Thus taking into account (9), we derive, for all
 $l\geq 1+j_0$,
$l\geq 1+j_0$,  $[M_p(f,r_l)]^2 \gt rsim l$, which allows to obtain
$[M_p(f,r_l)]^2 \gt rsim l$, which allows to obtain
 \begin{equation*}\displaystyle\limsup_{r\rightarrow 1^{-}}M_p(f,r)=+\infty.\end{equation*}
\begin{equation*}\displaystyle\limsup_{r\rightarrow 1^{-}}M_p(f,r)=+\infty.\end{equation*}(c) Case
 $\alpha \gt \frac{1-\gamma}{2}$: since f is hypercyclic, the conclusion is given by Theorem 2.2.
$\alpha \gt \frac{1-\gamma}{2}$: since f is hypercyclic, the conclusion is given by Theorem 2.2.
(2) Case
 $1 \lt p \lt 2$:
$1 \lt p \lt 2$:It suffices to combine the arguments of the proof of the preceding case with those of the proof of (2) of Theorem 3.3 to obtain the desired conclusions.
4.3. Optimal growth of  $\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic functions: a constructive proof
$\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic functions: a constructive proof
In this subsection, we intend to prove that the estimates given by Theorem 4.5 whenever α is different from the critical exponent, i.e.  $\alpha\ne \frac{1-\gamma}{\max(2,q)}$, are optimal. The case
$\alpha\ne \frac{1-\gamma}{\max(2,q)}$, are optimal. The case  $\alpha = \frac{1-\gamma}{\max(2,q)}$ will be treated separately in § 5. Thus for all
$\alpha = \frac{1-\gamma}{\max(2,q)}$ will be treated separately in § 5. Thus for all ![]() $0 \lt \gamma \lt 1$ and for
$0 \lt \gamma \lt 1$ and for  $\alpha\ne \frac{1-\gamma}{\max(2,q)}$, we propose to build
$\alpha\ne \frac{1-\gamma}{\max(2,q)}$, we propose to build ![]() $\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic functions for Tα that have the required Lp growth and no more. To do this, we follow the construction of frequently hypercyclic functions for Tα given in [Reference Mouze and Munnier25] which itself was partly inspired by [Reference Drasin and Saksman8]. In particular, we will need the so-called Rudin–Shapiro polynomials (combined with the de la Vallée–Poussin polynomials), which have coefficients ±1 (or bounded by 1) and an optimal growth of Lp-norm. Let us recall the associated result in the form of Lemma 2.1 of [Reference Drasin and Saksman8] that summarized the result of Rudin–Shapiro [Reference Rudin27].
$\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic functions for Tα that have the required Lp growth and no more. To do this, we follow the construction of frequently hypercyclic functions for Tα given in [Reference Mouze and Munnier25] which itself was partly inspired by [Reference Drasin and Saksman8]. In particular, we will need the so-called Rudin–Shapiro polynomials (combined with the de la Vallée–Poussin polynomials), which have coefficients ±1 (or bounded by 1) and an optimal growth of Lp-norm. Let us recall the associated result in the form of Lemma 2.1 of [Reference Drasin and Saksman8] that summarized the result of Rudin–Shapiro [Reference Rudin27].
(1) For each
 $N\geq 1$, there is a trigonometric polynomial
$N\geq 1$, there is a trigonometric polynomial  $p_N=\sum_{k=0}^{N-1}\varepsilon_{N,k}\mathrm{e}^{\mathrm{i}k\theta}$ where
$p_N=\sum_{k=0}^{N-1}\varepsilon_{N,k}\mathrm{e}^{\mathrm{i}k\theta}$ where  $\varepsilon_{N,k}=\pm 1$ for all
$\varepsilon_{N,k}=\pm 1$ for all  $0\leq k\leq N-1$ with at least half of the coefficients being +1 and with
$0\leq k\leq N-1$ with at least half of the coefficients being +1 and with
 \begin{equation*}\Vert p_{N}\Vert_{p}\leq 5\sqrt{N}\hbox{ for }p\in [2,+\infty].\end{equation*}
\begin{equation*}\Vert p_{N}\Vert_{p}\leq 5\sqrt{N}\hbox{ for }p\in [2,+\infty].\end{equation*}(2) For each
 $N\geq 1$, there is a trigonometric polynomial
$N\geq 1$, there is a trigonometric polynomial  $p_N^*=\sum_{k=0}^{N-1}a_{N,k}\mathrm{e}^{\mathrm{i}k\theta}$ where
$p_N^*=\sum_{k=0}^{N-1}a_{N,k}\mathrm{e}^{\mathrm{i}k\theta}$ where  $\vert a_{N,k}\vert\leq 1$ for all
$\vert a_{N,k}\vert\leq 1$ for all  $0\leq k\leq N-1$ with at least
$0\leq k\leq N-1$ with at least  $\lfloor \frac{N}{4} \rfloor$ coefficients being +1 and with
$\lfloor \frac{N}{4} \rfloor$ coefficients being +1 and with
 \begin{equation*}\|p^{*}_{N}\|_{p}\leq 3N^{1/q}\hbox{ for }p\in [1,2].\end{equation*}
\begin{equation*}\|p^{*}_{N}\|_{p}\leq 3N^{1/q}\hbox{ for }p\in [1,2].\end{equation*}
For any given polynomial q with  $q(z)=\sum_{j=0}^d b_j z^j$ with
$q(z)=\sum_{j=0}^d b_j z^j$ with ![]() $b_d\ne 0$, we denote
$b_d\ne 0$, we denote ![]() $d=\hbox{deg}(q)$ and
$d=\hbox{deg}(q)$ and  $\displaystyle \Vert q\Vert_{\ell^1}=\sum_{j=0}^d\vert b_j\vert$. We set
$\displaystyle \Vert q\Vert_{\ell^1}=\sum_{j=0}^d\vert b_j\vert$. We set  $\displaystyle 2\mathbb{N}=\bigcup_{k\geq 1}\mathcal{A}_{k}$ where for any
$\displaystyle 2\mathbb{N}=\bigcup_{k\geq 1}\mathcal{A}_{k}$ where for any ![]() $k\geq 1,$
$k\geq 1,$  $\displaystyle \mathcal{A}_{k}=\left\{2^{k}(2j-1);j\in\mathbb{N} \right\}.$ Denote by
$\displaystyle \mathcal{A}_{k}=\left\{2^{k}(2j-1);j\in\mathbb{N} \right\}.$ Denote by ![]() $\mathcal{P}$ the countable set of polynomials with rational coefficients and let us also consider pairs (q, l) with
$\mathcal{P}$ the countable set of polynomials with rational coefficients and let us also consider pairs (q, l) with ![]() $q\in\mathcal{P}$ and
$q\in\mathcal{P}$ and ![]() $l\in \mathbb{N}$ satisfying
$l\in \mathbb{N}$ satisfying ![]() $\Vert q\Vert_{\ell^1}\leq l$, displayed as a single sequence
$\Vert q\Vert_{\ell^1}\leq l$, displayed as a single sequence ![]() $(q_k,l_k)$. Clearly,
$(q_k,l_k)$. Clearly, ![]() $(q_k)$ is a dense set in
$(q_k)$ is a dense set in ![]() $H(\mathbb{D})$. Hence, for any
$H(\mathbb{D})$. Hence, for any ![]() $k\geq 1,$ we set
$k\geq 1,$ we set ![]() $d_k=\deg (q_k)$ and we have
$d_k=\deg (q_k)$ and we have
For any ![]() $\alpha\in\mathbb{R},$ for any positive integer
$\alpha\in\mathbb{R},$ for any positive integer ![]() $k\geq 1,$ we set
$k\geq 1,$ we set  $\displaystyle \tilde{q_{k}}(z)=\sum_{j=0}^{d_{k}}(j+1)^\alpha b_{j}^{(k)}z^{j}.$
$\displaystyle \tilde{q_{k}}(z)=\sum_{j=0}^{d_{k}}(j+1)^\alpha b_{j}^{(k)}z^{j}.$
Let α be a real number and ![]() $p\in (1,\infty].$ For all integer
$p\in (1,\infty].$ For all integer ![]() $n\geq 0,$ we set
$n\geq 0,$ we set ![]() $I_{n}=\{2^{n},\ldots,2^{n+1}-1\}.$ Next, for
$I_{n}=\{2^{n},\ldots,2^{n+1}-1\}.$ Next, for ![]() $k\geq 1,$ let us define the integers
$k\geq 1,$ let us define the integers
 \begin{equation*}\alpha_{k}=1+
\left\lfloor \max\left(l_{k}^{2}(1+d_{k})^{2\max(\alpha, 0)},d_{k}+
\max(3,3+\alpha)l_{k}^2+\max(\alpha, 0)l_{k}\log(1+d_{k})\right)\right\rfloor\end{equation*}
\begin{equation*}\alpha_{k}=1+
\left\lfloor \max\left(l_{k}^{2}(1+d_{k})^{2\max(\alpha, 0)},d_{k}+
\max(3,3+\alpha)l_{k}^2+\max(\alpha, 0)l_{k}\log(1+d_{k})\right)\right\rfloor\end{equation*}and
 \begin{equation*}\alpha^{*}_{k}=1+
\left\lfloor \max\left(l_{k}^{q}(1+d_{k})^{q\max(\alpha, 0)},d_{k}+\max(3,3+\alpha)l_{k}^2+\max(\alpha, 0)l_{k}\log(1+d_{k})
\right)\right\rfloor.\end{equation*}
\begin{equation*}\alpha^{*}_{k}=1+
\left\lfloor \max\left(l_{k}^{q}(1+d_{k})^{q\max(\alpha, 0)},d_{k}+\max(3,3+\alpha)l_{k}^2+\max(\alpha, 0)l_{k}\log(1+d_{k})
\right)\right\rfloor.\end{equation*} We set  $\displaystyle f_{\alpha}=\sum\limits_{n\geq 0}P_{n,\alpha}$ where the blocks
$\displaystyle f_{\alpha}=\sum\limits_{n\geq 0}P_{n,\alpha}$ where the blocks ![]() $(P_{n,\alpha})$ are polynomials defined as follows, using Rudin–Shapiro polynomials given by Lemma 4.6,
$(P_{n,\alpha})$ are polynomials defined as follows, using Rudin–Shapiro polynomials given by Lemma 4.6,
 \begin{equation}
P_{n,\alpha}(z) = \left\{\begin{array}{l}
0 \hbox{ if } n \mbox{ is odd }\\
0 \hbox{ if } n\in \mathcal{A}_{k}\hbox{ and } 2^{n-1} \lt \alpha_{k}\\
z^{2^{n}}Q_{n}(z) \hbox{ if } n\in \mathcal{A}_{k}\hbox{ and }2^{n-1}\geq \alpha_{k}
\end{array}\right.
\end{equation}
\begin{equation}
P_{n,\alpha}(z) = \left\{\begin{array}{l}
0 \hbox{ if } n \mbox{ is odd }\\
0 \hbox{ if } n\in \mathcal{A}_{k}\hbox{ and } 2^{n-1} \lt \alpha_{k}\\
z^{2^{n}}Q_{n}(z) \hbox{ if } n\in \mathcal{A}_{k}\hbox{ and }2^{n-1}\geq \alpha_{k}
\end{array}\right.
\end{equation} with for ![]() $n\in \mathcal{A}_{k}$,
$n\in \mathcal{A}_{k}$,
 \begin{equation*}Q_{n}(z)=\sum\limits_{j\in I_{n}}(j+1)^{-\alpha}c_{j-2^{n}}^{(k)}z^{j-2^{n}}\end{equation*}
\begin{equation*}Q_{n}(z)=\sum\limits_{j\in I_{n}}(j+1)^{-\alpha}c_{j-2^{n}}^{(k)}z^{j-2^{n}}\end{equation*} where the sequence  $(c_{j}^{(k)})$ denotes the sequence of the coefficients of the polynomial
$(c_{j}^{(k)})$ denotes the sequence of the coefficients of the polynomial  $\displaystyle p_{\lfloor \frac{2^{n(1-\gamma)}}{\alpha_{k}} \rfloor}(z^{\alpha_k})\tilde{q_{k}}(z)$.
$\displaystyle p_{\lfloor \frac{2^{n(1-\gamma)}}{\alpha_{k}} \rfloor}(z^{\alpha_k})\tilde{q_{k}}(z)$.
We also set  $\displaystyle f^{*}_{\alpha}=\sum\limits_{n\geq 0}P^{*}_{n,\alpha}$ where the blocks
$\displaystyle f^{*}_{\alpha}=\sum\limits_{n\geq 0}P^{*}_{n,\alpha}$ where the blocks ![]() $(P^{*}_{n,\alpha})$ are polynomials defined as follows, using the de la Vallée–Poussin polynomials given by Lemma 4.6,
$(P^{*}_{n,\alpha})$ are polynomials defined as follows, using the de la Vallée–Poussin polynomials given by Lemma 4.6,
 \begin{equation}
P^{*}_{n,\alpha}(z) = \left\{\begin{array}{l}
0 \hbox{ if } n \hbox{ is odd }\\
0 \hbox{ if } n\in \mathcal{A}_{k}\hbox{ and } 2^{n-1} \lt \alpha^{*}_{k}\\
\displaystyle z^{2^{n}}Q_{n}^{*}(z)\hbox{ if } n\in \mathcal{A}_{k}\hbox{ and } 2^{n-1}\geq\alpha^{*}_{k},
\end{array}\right.
\end{equation}
\begin{equation}
P^{*}_{n,\alpha}(z) = \left\{\begin{array}{l}
0 \hbox{ if } n \hbox{ is odd }\\
0 \hbox{ if } n\in \mathcal{A}_{k}\hbox{ and } 2^{n-1} \lt \alpha^{*}_{k}\\
\displaystyle z^{2^{n}}Q_{n}^{*}(z)\hbox{ if } n\in \mathcal{A}_{k}\hbox{ and } 2^{n-1}\geq\alpha^{*}_{k},
\end{array}\right.
\end{equation} with, for ![]() $n\in \mathcal{A}_{k}$,
$n\in \mathcal{A}_{k}$,
 \begin{equation*}Q_{n}^{*}(z)=\sum\limits_{j\in I_{n}}(j+1)^{-\alpha}c_{j-2^{n}}^{(k)}z^{j-2^{n}}\end{equation*}
\begin{equation*}Q_{n}^{*}(z)=\sum\limits_{j\in I_{n}}(j+1)^{-\alpha}c_{j-2^{n}}^{(k)}z^{j-2^{n}}\end{equation*} where the sequence  $(c_{j}^{(k)})$ denotes the sequence of the coefficients of the polynomial
$(c_{j}^{(k)})$ denotes the sequence of the coefficients of the polynomial  $\displaystyle p^{*}_{\lfloor \frac{2^{n(1-\gamma)}}{\alpha^{*}_{k}} \rfloor}(z^{\alpha^{*}_{k}})\tilde{q_{k}}(z)$.
$\displaystyle p^{*}_{\lfloor \frac{2^{n(1-\gamma)}}{\alpha^{*}_{k}} \rfloor}(z^{\alpha^{*}_{k}})\tilde{q_{k}}(z)$.
A combination of Lemma 4.7 below with the triangle inequality shows that the function fα (resp. ![]() $f_{\alpha}^*$) belongs to
$f_{\alpha}^*$) belongs to ![]() $H(\mathbb{D})$. Observe that, if we denote the polynomial
$H(\mathbb{D})$. Observe that, if we denote the polynomial  $z\mapsto p_{\lfloor \frac{2^{n-1}}{\alpha_{k}} \rfloor}(z^{\alpha_k})$ (resp.
$z\mapsto p_{\lfloor \frac{2^{n-1}}{\alpha_{k}} \rfloor}(z^{\alpha_k})$ (resp.  $z\mapsto p_{\lfloor \frac{2^{n-1}}{\alpha_{k}} \rfloor}^*(z^{\alpha_k^*})$) by gk (resp.
$z\mapsto p_{\lfloor \frac{2^{n-1}}{\alpha_{k}} \rfloor}^*(z^{\alpha_k^*})$) by gk (resp. ![]() $g_k^*$), we have, for all
$g_k^*$), we have, for all ![]() $1\leq p\leq +\infty,$
$1\leq p\leq +\infty,$  $\Vert g_k \Vert_p=
\Vert p_{\lfloor \frac{2^{n-1}}{\alpha_{k}} \rfloor}\Vert_p$ (resp.
$\Vert g_k \Vert_p=
\Vert p_{\lfloor \frac{2^{n-1}}{\alpha_{k}} \rfloor}\Vert_p$ (resp.  $\Vert g_k^* \Vert_p=\Vert p_{\lfloor \frac{2^{n-1}}{\alpha_{k}^*} \rfloor}^*\Vert_p$). Finally for any integer n, let us denote
$\Vert g_k^* \Vert_p=\Vert p_{\lfloor \frac{2^{n-1}}{\alpha_{k}^*} \rfloor}^*\Vert_p$). Finally for any integer n, let us denote ![]() $(\phi_n(k))$ the sequence defined as follows
$(\phi_n(k))$ the sequence defined as follows
 \begin{equation*}\phi_{n}(k)=\left\{\begin{array}{ll}(k+1)^{-\alpha}&\hbox{ if }k\in I_n\\0&\hbox{ otherwise.}
\end{array}\right.\end{equation*}
\begin{equation*}\phi_{n}(k)=\left\{\begin{array}{ll}(k+1)^{-\alpha}&\hbox{ if }k\in I_n\\0&\hbox{ otherwise.}
\end{array}\right.\end{equation*}Lemma 4.7. Let ![]() $\alpha\in\mathbb{R}$. The following estimates hold.
$\alpha\in\mathbb{R}$. The following estimates hold.
(i) For any
 $2\leq p\leq +\infty,$ any
$2\leq p\leq +\infty,$ any  $0 \lt r \lt 1$ and any
$0 \lt r \lt 1$ and any  $n\in\mathbb{N},$ we have
$n\in\mathbb{N},$ we have
 \begin{equation*}M_p(P_{n,\alpha},r)\lesssim 2^{n(\frac{1-\gamma}{2}-\alpha)}r^{2^{n}}.\end{equation*}
\begin{equation*}M_p(P_{n,\alpha},r)\lesssim 2^{n(\frac{1-\gamma}{2}-\alpha)}r^{2^{n}}.\end{equation*}(ii) For any
 $1 \lt p \lt 2,$ any
$1 \lt p \lt 2,$ any  $0 \lt r \lt 1$ and any
$0 \lt r \lt 1$ and any  $n\in\mathbb{N},$ we have
$n\in\mathbb{N},$ we have
 \begin{equation*}M_p(P^{*}_{n,\alpha},r)\lesssim 2^{n(\frac{1-\gamma}{q}-\alpha)}r^{2^{n}}.\end{equation*}
\begin{equation*}M_p(P^{*}_{n,\alpha},r)\lesssim 2^{n(\frac{1-\gamma}{q}-\alpha)}r^{2^{n}}.\end{equation*}
(i) On one hand, we deal with the case
 $2\leq p \lt +\infty.$ Let n be a positive integer. Without loss of generality, we can assume that n belongs to the set
$2\leq p \lt +\infty.$ Let n be a positive integer. Without loss of generality, we can assume that n belongs to the set  $\mathcal{A}_{k}$ for some
$\mathcal{A}_{k}$ for some  $k\geq 1$. Let r be in
$k\geq 1$. Let r be in  $(0,1).$ Since
$(0,1).$ Since  $\displaystyle r \mapsto M_p(f,.)$ is increasing, we get
$\displaystyle r \mapsto M_p(f,.)$ is increasing, we get
 \begin{equation*}M_p(P_{n,\alpha},r)\leq r^{2^{n}}\|Q_{n}\|_{p}.\end{equation*}
\begin{equation*}M_p(P_{n,\alpha},r)\leq r^{2^{n}}\|Q_{n}\|_{p}.\end{equation*}Then, the polynomial Qn can be viewed as a trigonometric polynomial obtained by an abstract convolution operator on
 $\mathbb{T},$ given by
$\mathbb{T},$ given by  $(c_{k})_{k\geq 0} \mapsto (\phi_{n}(j)c_{j-2^{n}}^{(k)})_{j\geq 0}$ (where
$(c_{k})_{k\geq 0} \mapsto (\phi_{n}(j)c_{j-2^{n}}^{(k)})_{j\geq 0}$ (where  $(c_{j}^{(k)})$ denotes the sequence of the coefficients of the polynomial
$(c_{j}^{(k)})$ denotes the sequence of the coefficients of the polynomial  $\displaystyle p_{\lfloor \frac{2^{n-1}}{\alpha_{k}} \rfloor}\tilde{q_{k}}$). Now, we are going to apply the Marcinkiewicz Multiplier Theorem [Reference Edwards and Gaudry10, Theorem 8.2 p.148]. To do this, observe that we have, for any
$\displaystyle p_{\lfloor \frac{2^{n-1}}{\alpha_{k}} \rfloor}\tilde{q_{k}}$). Now, we are going to apply the Marcinkiewicz Multiplier Theorem [Reference Edwards and Gaudry10, Theorem 8.2 p.148]. To do this, observe that we have, for any  $l\geq 1,$
$l\geq 1,$
 \begin{equation*}\sup_{j\in I_{l}}\vert \phi_{n}(j) \vert \leq\sup_{j\in I_{n}}\vert \phi_{n}(j) \vert \lesssim 2^{-n\alpha}\end{equation*}
\begin{equation*}\sup_{j\in I_{l}}\vert \phi_{n}(j) \vert \leq\sup_{j\in I_{n}}\vert \phi_{n}(j) \vert \lesssim 2^{-n\alpha}\end{equation*}and
 \begin{equation*}\sup_{l}\sum_{j\in I_{l}}\vert \phi_{n}(j+1)-\phi_{n}(j)\vert \leq \sum_{j\in I_{n}}\vert \phi_{n}(j+1)-\phi_{n}(j)\vert \lesssim 2^{-n\alpha}.\end{equation*}
\begin{equation*}\sup_{l}\sum_{j\in I_{l}}\vert \phi_{n}(j+1)-\phi_{n}(j)\vert \leq \sum_{j\in I_{n}}\vert \phi_{n}(j+1)-\phi_{n}(j)\vert \lesssim 2^{-n\alpha}.\end{equation*}Hence, taking into account the choice of αk and Lemma 4.6, we get
 \begin{equation*}\begin{array}{rl}
\|Q_{n}\|_{p} & \lesssim 2^{-n\alpha} \Vert p_{\lfloor \frac{2^{n(1-\gamma)}}{\alpha_{k}}\rfloor}\Vert_{p} \|\tilde{q_{k}}\|_{\infty}\\
& \lesssim 2^{-n\alpha} \sqrt{\frac{2^{n(1-\gamma)}}{\alpha_{k}}}\ l_{k}(1+d_{k})^{\max(\alpha,0)}\\
& \lesssim 2^{n(\frac{1-\gamma}{2}-\alpha)}.\end{array}\end{equation*}
\begin{equation*}\begin{array}{rl}
\|Q_{n}\|_{p} & \lesssim 2^{-n\alpha} \Vert p_{\lfloor \frac{2^{n(1-\gamma)}}{\alpha_{k}}\rfloor}\Vert_{p} \|\tilde{q_{k}}\|_{\infty}\\
& \lesssim 2^{-n\alpha} \sqrt{\frac{2^{n(1-\gamma)}}{\alpha_{k}}}\ l_{k}(1+d_{k})^{\max(\alpha,0)}\\
& \lesssim 2^{n(\frac{1-\gamma}{2}-\alpha)}.\end{array}\end{equation*}Finally, we obtain the desired estimate
 \begin{equation*}M_p(P_{n,\alpha},r)\lesssim 2^{n(\frac{1-\gamma}{2}-\alpha)}r^{2^{n}}.\end{equation*}
\begin{equation*}M_p(P_{n,\alpha},r)\lesssim 2^{n(\frac{1-\gamma}{2}-\alpha)}r^{2^{n}}.\end{equation*}On the other hand, we deal with the case
 $p=\infty$. Let us recall that
$p=\infty$. Let us recall that  $P_{n,\alpha}(z)=0$ or
$P_{n,\alpha}(z)=0$ or  $z^{2^{n}} Q_{n}(z)$ with
$z^{2^{n}} Q_{n}(z)$ with  $Q_{n}(z)=\sum\limits_{j\in I_{n}}(j+1)^{-\alpha}c_{j-2^{n}}^{(k)}z^{j-2^{n}}$ where
$Q_{n}(z)=\sum\limits_{j\in I_{n}}(j+1)^{-\alpha}c_{j-2^{n}}^{(k)}z^{j-2^{n}}$ where  $(c_{j}^{(k)})$ denotes the sequence of the coefficients of the polynomial
$(c_{j}^{(k)})$ denotes the sequence of the coefficients of the polynomial  $p_{\lfloor \frac{2^{n(1-\gamma)}}{\alpha_{k}} \rfloor}(z^{\alpha_k})\tilde{q_{k}}(z)$. First, assume that
$p_{\lfloor \frac{2^{n(1-\gamma)}}{\alpha_{k}} \rfloor}(z^{\alpha_k})\tilde{q_{k}}(z)$. First, assume that  $\alpha\leq 0$. We write
$\alpha\leq 0$. We write
 \begin{equation*} M_{\infty}(P_{n,\alpha},r)\lesssim r^{2^n} \Vert Q_{n} \Vert_{\infty}.\end{equation*}
\begin{equation*} M_{\infty}(P_{n,\alpha},r)\lesssim r^{2^n} \Vert Q_{n} \Vert_{\infty}.\end{equation*}Using the form of Qn, as in the proof of Lemma 3.6 of [Reference Mouze and Munnier25], we apply a fractional Bernstein’s inequality to obtain, taking into consideration Lemma 4.6,
 \begin{equation*} M_{\infty}(P_{n,\alpha},r)\lesssim r^{2^n} 2^{-n\alpha}\Vert Q_{n} \Vert_{\infty}
\lesssim 2^{-n\alpha}\Vert p_{\lfloor \frac{2^{n(1-\gamma)}}{\alpha_{k}} \rfloor}\Vert_{\infty}
\Vert \tilde{q_{k}} \Vert_{\infty}\lesssim 2^{-n\alpha}\sqrt{\lfloor \frac{2^{n(1-\gamma)}}{\alpha_{k}} \rfloor} l_k.\end{equation*}
\begin{equation*} M_{\infty}(P_{n,\alpha},r)\lesssim r^{2^n} 2^{-n\alpha}\Vert Q_{n} \Vert_{\infty}
\lesssim 2^{-n\alpha}\Vert p_{\lfloor \frac{2^{n(1-\gamma)}}{\alpha_{k}} \rfloor}\Vert_{\infty}
\Vert \tilde{q_{k}} \Vert_{\infty}\lesssim 2^{-n\alpha}\sqrt{\lfloor \frac{2^{n(1-\gamma)}}{\alpha_{k}} \rfloor} l_k.\end{equation*}Thanks to the choice of αk, we have, for
 $\alpha\leq 0$,
$\alpha\leq 0$,
 \begin{equation*}M_{\infty}(P_{n,\alpha},r)\lesssim 2^{n(\frac{1-\gamma}{2}-\alpha)}.\end{equation*}
\begin{equation*}M_{\infty}(P_{n,\alpha},r)\lesssim 2^{n(\frac{1-\gamma}{2}-\alpha)}.\end{equation*}To conclude, it suffices to mimic the induction of the proof of Lemma 3.7 of [Reference Mouze and Munnier25].
(ii) The proof is similar as that of the case
 $2\leq p \lt +\infty$ by applying Lemma 4.6 for
$2\leq p \lt +\infty$ by applying Lemma 4.6 for  $1 \lt p \lt 2$.
$1 \lt p \lt 2$.
Now we are ready to obtain the rate of growth of the aforementioned functions fα and ![]() $f^*_{\alpha}.$ We refer to Lemmas 3.4, 3.5 and 3.8 of [Reference Mouze and Munnier25] with obvious modifications.
$f^*_{\alpha}.$ We refer to Lemmas 3.4, 3.5 and 3.8 of [Reference Mouze and Munnier25] with obvious modifications.
(1) Let
 $2\leq p\leq+\infty.$ For all
$2\leq p\leq+\infty.$ For all  $0 \lt r \lt 1,$ the following estimates hold
$0 \lt r \lt 1,$ the following estimates hold
 \begin{equation*}M_p(f_{\alpha},r)\lesssim \left\{\begin{array}{l}(1-r)^{\alpha-\frac{1-\gamma}{2}}\hbox{ if }\alpha \lt \frac{1-\gamma}{2}.\\
1\hbox{ if }\alpha \gt \frac{1-\gamma}{2}\end{array}\right.\end{equation*}
\begin{equation*}M_p(f_{\alpha},r)\lesssim \left\{\begin{array}{l}(1-r)^{\alpha-\frac{1-\gamma}{2}}\hbox{ if }\alpha \lt \frac{1-\gamma}{2}.\\
1\hbox{ if }\alpha \gt \frac{1-\gamma}{2}\end{array}\right.\end{equation*}(2) Let
 $1 \lt p \lt 2$. For all
$1 \lt p \lt 2$. For all  $0 \lt r \lt 1,$ the following estimates hold
$0 \lt r \lt 1,$ the following estimates hold
 \begin{equation*}M_p(f_{\alpha}^{*},r)\lesssim\left\{\begin{array}{l}(1-r)^{\alpha-\frac{1-\gamma}{q}}\hbox{ if }\alpha \lt \frac{1-\gamma}{q}.\\
1\hbox{ if }\alpha \gt \frac{1-\gamma}{q}\end{array}\right.\end{equation*}
\begin{equation*}M_p(f_{\alpha}^{*},r)\lesssim\left\{\begin{array}{l}(1-r)^{\alpha-\frac{1-\gamma}{q}}\hbox{ if }\alpha \lt \frac{1-\gamma}{q}.\\
1\hbox{ if }\alpha \gt \frac{1-\gamma}{q}\end{array}\right.\end{equation*}
Now we are going to prove that the functions fα and ![]() $f^{*}_{\alpha}$ are
$f^{*}_{\alpha}$ are ![]() $U_{\beta^{\gamma}}$-frequently hypercyclic for Tα.
$U_{\beta^{\gamma}}$-frequently hypercyclic for Tα.
Proposition 4.9. For ![]() $p\geq 2$ (resp.
$p\geq 2$ (resp. ![]() $1 \lt p \lt 2$), the function fα (resp.
$1 \lt p \lt 2$), the function fα (resp. ![]() $f^{*}_{\alpha}$) is a
$f^{*}_{\alpha}$) is a ![]() $U_{\beta^{\gamma}}$-frequently hypercyclic vector for the operator Tα.
$U_{\beta^{\gamma}}$-frequently hypercyclic vector for the operator Tα.
Proof. We only prove that the vector fα is ![]() $U_{\beta^{\gamma}}$-frequently hypercyclic for the operator Tα. We do not repeat the details for
$U_{\beta^{\gamma}}$-frequently hypercyclic for the operator Tα. We do not repeat the details for ![]() $f^{*}_{\alpha}$: it will be enough to make the appropriate modifications.
$f^{*}_{\alpha}$: it will be enough to make the appropriate modifications.
Let k be a large enough integer. Let us consider ![]() $n\in\mathcal{A}_{k}$ such that
$n\in\mathcal{A}_{k}$ such that ![]() $2^{n-1}\geq \alpha_{k}.$ We consider
$2^{n-1}\geq \alpha_{k}.$ We consider ![]() $\mathcal{B}_{n}$ the set of s in In such that the coefficient of z s in the polynomial
$\mathcal{B}_{n}$ the set of s in In such that the coefficient of z s in the polynomial  $z^{2^{n}}p_{\lfloor \frac{2^{n(1-\gamma)}}{\alpha_{k}} \rfloor}(z^{\alpha_{k}})$ is equal to 1 and we denote by
$z^{2^{n}}p_{\lfloor \frac{2^{n(1-\gamma)}}{\alpha_{k}} \rfloor}(z^{\alpha_{k}})$ is equal to 1 and we denote by  $\displaystyle T_{k}=\left\{s: s\in \mathcal{B}_{n},\ n\in\mathcal{A}_{k}, \ 2^{n-1}\geq \alpha_{k}\right\}.$
$\displaystyle T_{k}=\left\{s: s\in \mathcal{B}_{n},\ n\in\mathcal{A}_{k}, \ 2^{n-1}\geq \alpha_{k}\right\}.$
Observe that ![]() $\max(\mathcal{B}_{n})\leq 2^n+\lfloor2^{n(1-\gamma)}\rfloor$ and since at least half of the coefficients of
$\max(\mathcal{B}_{n})\leq 2^n+\lfloor2^{n(1-\gamma)}\rfloor$ and since at least half of the coefficients of  $p_{\lfloor \frac{2^{n(1-\gamma)}}{\alpha_{k}} \rfloor}$ being +1, we get
$p_{\lfloor \frac{2^{n(1-\gamma)}}{\alpha_{k}} \rfloor}$ being +1, we get
 \begin{equation}\frac{\sum\limits_{j\leq \max(\mathcal{B}_{n});\atop j\in T_{k}}\mathrm{e}^{j^{\gamma}}}{\sum\limits_{j\leq \max(\mathcal{B}_{n})}\mathrm{e}^{j^{\gamma}}}\geq
\frac{\sum\limits_{j=2^n}^{2^n+2^{-1}\lfloor2^{n(1-\gamma)}\rfloor}\mathrm{e}^{j^{\gamma}}}{\sum\limits_{j=1}^{2^n+\lfloor2^{n(1-\gamma)}\rfloor}\mathrm{e}^{j^{\gamma}}}=
\frac{\sum\limits_{j=1}^{2^n+2^{-1}\lfloor2^{n(1-\gamma)}\rfloor}\mathrm{e}^{j^{\gamma}}}{\sum\limits_{j=1}^{2^n+\lfloor2^{n(1-\gamma)}\rfloor}\mathrm{e}^{j^{\gamma}}}-\frac{\sum\limits_{j=1}^{2^n-1}\mathrm{e}^{j^{\gamma}}}{\sum\limits_{j=1}^{2^n+\lfloor2^{n(1-\gamma)}\rfloor}\mathrm{e}^{j^{\gamma}}}.
\end{equation}
\begin{equation}\frac{\sum\limits_{j\leq \max(\mathcal{B}_{n});\atop j\in T_{k}}\mathrm{e}^{j^{\gamma}}}{\sum\limits_{j\leq \max(\mathcal{B}_{n})}\mathrm{e}^{j^{\gamma}}}\geq
\frac{\sum\limits_{j=2^n}^{2^n+2^{-1}\lfloor2^{n(1-\gamma)}\rfloor}\mathrm{e}^{j^{\gamma}}}{\sum\limits_{j=1}^{2^n+\lfloor2^{n(1-\gamma)}\rfloor}\mathrm{e}^{j^{\gamma}}}=
\frac{\sum\limits_{j=1}^{2^n+2^{-1}\lfloor2^{n(1-\gamma)}\rfloor}\mathrm{e}^{j^{\gamma}}}{\sum\limits_{j=1}^{2^n+\lfloor2^{n(1-\gamma)}\rfloor}\mathrm{e}^{j^{\gamma}}}-\frac{\sum\limits_{j=1}^{2^n-1}\mathrm{e}^{j^{\gamma}}}{\sum\limits_{j=1}^{2^n+\lfloor2^{n(1-\gamma)}\rfloor}\mathrm{e}^{j^{\gamma}}}.
\end{equation}Clearly, we have
 \begin{equation*}\left(2^n+\frac{\lfloor2^{n(1-\gamma)}\rfloor}{2}\right)^{\gamma}-\left(2^n+\lfloor2^{n(1-\gamma)}\rfloor\right)^{\gamma}\rightarrow -\frac{\gamma}{2}\end{equation*}
\begin{equation*}\left(2^n+\frac{\lfloor2^{n(1-\gamma)}\rfloor}{2}\right)^{\gamma}-\left(2^n+\lfloor2^{n(1-\gamma)}\rfloor\right)^{\gamma}\rightarrow -\frac{\gamma}{2}\end{equation*}and
 \begin{equation*}\left(2^n-1\right)^{\gamma}-\left(2^n+\lfloor2^{n(1-\gamma)}\rfloor\right)^{\gamma}\rightarrow -\gamma,\end{equation*}
\begin{equation*}\left(2^n-1\right)^{\gamma}-\left(2^n+\lfloor2^{n(1-\gamma)}\rfloor\right)^{\gamma}\rightarrow -\gamma,\end{equation*}which implies, using similar estimations as those of the proof of Lemma 4.4,
 \begin{equation*}\frac{\sum\limits_{j=1}^{2^n+2^{-1}\lfloor2^{n(1-\gamma)}\rfloor}\mathrm{e}^{j^{\gamma}}}{\sum\limits_{j=1}^{2^n+\lfloor2^{n(1-\gamma)}\rfloor}\mathrm{e}^{j^{\gamma}}}\rightarrow e^{-\gamma/2}\quad
\hbox{ and }\quad\frac{\sum\limits_{j=1}^{2^n-1}\mathrm{e}^{j^{\gamma}}}{\sum\limits_{j=1}^{2^n+\lfloor2^{n(1-\gamma)}\rfloor}\mathrm{e}^{j^{\gamma}}}\rightarrow \mathrm{e}^{-\gamma},\quad \hbox{ as }n\rightarrow +\infty.\end{equation*}
\begin{equation*}\frac{\sum\limits_{j=1}^{2^n+2^{-1}\lfloor2^{n(1-\gamma)}\rfloor}\mathrm{e}^{j^{\gamma}}}{\sum\limits_{j=1}^{2^n+\lfloor2^{n(1-\gamma)}\rfloor}\mathrm{e}^{j^{\gamma}}}\rightarrow e^{-\gamma/2}\quad
\hbox{ and }\quad\frac{\sum\limits_{j=1}^{2^n-1}\mathrm{e}^{j^{\gamma}}}{\sum\limits_{j=1}^{2^n+\lfloor2^{n(1-\gamma)}\rfloor}\mathrm{e}^{j^{\gamma}}}\rightarrow \mathrm{e}^{-\gamma},\quad \hbox{ as }n\rightarrow +\infty.\end{equation*}Hence the inequality (18) ensures that
Then let α be a real number and let ![]() $k\in\mathbb{N}$. Let us consider
$k\in\mathbb{N}$. Let us consider ![]() $s\in\mathcal{B}_{n}$ with
$s\in\mathcal{B}_{n}$ with ![]() $n\in\mathcal{A}_{k}$ satisfying
$n\in\mathcal{A}_{k}$ satisfying ![]() $2^{n-1}\geq\alpha_{k}.$ As in the proof of Lemma 3.9 of [Reference Mouze and Munnier25] with easy modifications, we can prove that
$2^{n-1}\geq\alpha_{k}.$ As in the proof of Lemma 3.9 of [Reference Mouze and Munnier25] with easy modifications, we can prove that
 \begin{equation*}\sup_{\vert z\vert = 1-\frac{1}{l_{k}}} \vert T_{\alpha}^{s}(f_{\alpha})(z)-q_{k}(z)\vert \lesssim \frac{1}{l_{k}},\end{equation*}
\begin{equation*}\sup_{\vert z\vert = 1-\frac{1}{l_{k}}} \vert T_{\alpha}^{s}(f_{\alpha})(z)-q_{k}(z)\vert \lesssim \frac{1}{l_{k}},\end{equation*} provided that k is chosen large enough. This allows to obtain ![]() $U_{\beta^{\gamma}}$-frequent hypercyclicity of fα.
$U_{\beta^{\gamma}}$-frequent hypercyclicity of fα.
In summary, Lemma 4.8 and Proposition 4.9 leads to the following result, which shows that the statement of Theorem 4.5 is optimal whenever α is not the critical exponent.
Theorem 4.10. Let ![]() $0 \lt \gamma \lt 1$ and
$0 \lt \gamma \lt 1$ and ![]() $1 \lt p\leq\infty$.
$1 \lt p\leq\infty$.
(1) for
 $\alpha \lt \frac{1-\gamma}{\max(2,q)}$, there exists a
$\alpha \lt \frac{1-\gamma}{\max(2,q)}$, there exists a  $\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic function for the operator Tα such that
$\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic function for the operator Tα such that
 \begin{equation*}M_p(f,r)\lesssim (1-r)^{\alpha-\frac{1-\gamma}{\max(2,q)}};\end{equation*}
\begin{equation*}M_p(f,r)\lesssim (1-r)^{\alpha-\frac{1-\gamma}{\max(2,q)}};\end{equation*}(2) for
 $\alpha \gt \frac{1-\gamma}{\max(2,q)}$, there exists a
$\alpha \gt \frac{1-\gamma}{\max(2,q)}$, there exists a  $\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic function for the operator Tα such that
$\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic function for the operator Tα such that
 \begin{equation*}M_p(f,r)\lesssim 1.\end{equation*}
\begin{equation*}M_p(f,r)\lesssim 1.\end{equation*}
(1) For the critical value
 $\alpha=\frac{1-\gamma}{\max(2,q)}$, the optimality of the rate of growth in this theorem will be obtained in Theorem 5.11.
$\alpha=\frac{1-\gamma}{\max(2,q)}$, the optimality of the rate of growth in this theorem will be obtained in Theorem 5.11.(2) Let
 $0 \lt \gamma \lt 1$. It seems important to note that the functions constructed for the proof of Theorem 4.10 are
$0 \lt \gamma \lt 1$. It seems important to note that the functions constructed for the proof of Theorem 4.10 are  $\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic for Tα but neither
$\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic for Tα but neither  $\mathcal{U}_{\beta^{\gamma'}}$-frequently hypercyclic for
$\mathcal{U}_{\beta^{\gamma'}}$-frequently hypercyclic for  $0 \lt \gamma' \lt \gamma$ nor
$0 \lt \gamma' \lt \gamma$ nor  $\mathcal{U}$-frequently hypercyclic, since they don’t satisfy the estimates given by Theorem 4.5 or Theorem 3.3.
$\mathcal{U}$-frequently hypercyclic, since they don’t satisfy the estimates given by Theorem 4.5 or Theorem 3.3.
Finally let us say some words for the case p = 1. As in the proof of Theorem 3.5, observe that a ![]() $\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic function is necessarily hypercyclic and a
$\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic function is necessarily hypercyclic and a ![]() $\mathcal{U}$-frequently hypercyclic function is necessarily
$\mathcal{U}$-frequently hypercyclic function is necessarily ![]() $\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic. This leads to the following statement.
$\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic. This leads to the following statement.
Theorem 4.12. Let ![]() $0 \lt \gamma \lt 1$. Let f be a
$0 \lt \gamma \lt 1$. Let f be a ![]() $\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic function for the operator Tα. Then, the following assertions hold
$\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic function for the operator Tα. Then, the following assertions hold
 \begin{align*}&\limsup_{r\rightarrow 1^{-}}\left((1-r)^{-\alpha}M_1(f,r)\right)=+\infty ,\quad\hbox{ if }\alpha\leq 0,\\
&\limsup_{r\rightarrow 1^{-}}M_1(f,r) \gt 0,\quad\hbox{ if }\alpha \gt 0.
\end{align*}
\begin{align*}&\limsup_{r\rightarrow 1^{-}}\left((1-r)^{-\alpha}M_1(f,r)\right)=+\infty ,\quad\hbox{ if }\alpha\leq 0,\\
&\limsup_{r\rightarrow 1^{-}}M_1(f,r) \gt 0,\quad\hbox{ if }\alpha \gt 0.
\end{align*} These results are optimal in the following sense: for any positive integer ![]() $l\geq 1$, there exists a
$l\geq 1$, there exists a ![]() $\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic function for the operator Tα such that for every
$\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic function for the operator Tα such that for every ![]() $0 \lt r \lt 1$ sufficiently large
$0 \lt r \lt 1$ sufficiently large
 \begin{equation*}M_1(f,r)\lesssim\left\{\begin{array}{ll} (1-r)^{\alpha}\log_l(-\log(1-r))& \hbox{ if } \alpha \lt 0\\1&
\hbox{ if } \alpha \gt 0.\end{array}\right.\end{equation*}
\begin{equation*}M_1(f,r)\lesssim\left\{\begin{array}{ll} (1-r)^{\alpha}\log_l(-\log(1-r))& \hbox{ if } \alpha \lt 0\\1&
\hbox{ if } \alpha \gt 0.\end{array}\right.\end{equation*}5. Optimal estimates: the case of the critical exponent
In this section, we are going to show that the growth of ![]() $\mathcal{U}$-frequently or
$\mathcal{U}$-frequently or ![]() $U_{\beta^{\gamma}}$-frequently hypercyclic functions for Tα can be arbitrarily slow when α is the critical exponent. The situation will therefore be similar to the hypercyclic case for which for all
$U_{\beta^{\gamma}}$-frequently hypercyclic functions for Tα can be arbitrarily slow when α is the critical exponent. The situation will therefore be similar to the hypercyclic case for which for all ![]() $1\leq p\leq\infty$ the critical exponent is α = 0 and, according Theorem 2.2, the two following properties hold: for all hypercyclic function f for Tα,
$1\leq p\leq\infty$ the critical exponent is α = 0 and, according Theorem 2.2, the two following properties hold: for all hypercyclic function f for Tα, ![]() $\limsup_{r\rightarrow 1^{-}}M_p(f,r)=+\infty$ and for any function
$\limsup_{r\rightarrow 1^{-}}M_p(f,r)=+\infty$ and for any function ![]() $\varphi:[0,1)\rightarrow \mathbb{R}_+$ tending to infinity as r tends to 1, there is a hypercyclic function f such that
$\varphi:[0,1)\rightarrow \mathbb{R}_+$ tending to infinity as r tends to 1, there is a hypercyclic function f such that ![]() $M_p(f,r)\leq \varphi(r)$. For this, we are going to adapt the constructive method used in § 4.3. Before we start, we establish a lemma that will be useful in the following.
$M_p(f,r)\leq \varphi(r)$. For this, we are going to adapt the constructive method used in § 4.3. Before we start, we establish a lemma that will be useful in the following.
Lemma 5.1. Let ![]() $(w_n)$ be an increasing sequence of positive integers such that
$(w_n)$ be an increasing sequence of positive integers such that  $\frac{w_{n+1}}{w_n}\rightarrow +\infty$ as n tends to infinity. Let
$\frac{w_{n+1}}{w_n}\rightarrow +\infty$ as n tends to infinity. Let ![]() $(a_n)$ be a bounded sequence of positive real numbers such that
$(a_n)$ be a bounded sequence of positive real numbers such that ![]() $\sum a_n=+\infty$. If we denote by
$\sum a_n=+\infty$. If we denote by ![]() $h:\mathbb{R}_+\rightarrow\mathbb{R}_+$ an increasing function with, for all
$h:\mathbb{R}_+\rightarrow\mathbb{R}_+$ an increasing function with, for all ![]() $n\in\mathbb{N}$,
$n\in\mathbb{N}$, ![]() $h(n)=w_n$, the following estimate holds
$h(n)=w_n$, the following estimate holds
 \begin{equation*}\sum_{n\geq 0} a_n \left( 1-\frac{1}{h(x)}\right)^{w_n}\sim \sum_{n\leq x}a_n,\quad\hbox{as }x\rightarrow\infty.\end{equation*}
\begin{equation*}\sum_{n\geq 0} a_n \left( 1-\frac{1}{h(x)}\right)^{w_n}\sim \sum_{n\leq x}a_n,\quad\hbox{as }x\rightarrow\infty.\end{equation*}Proof. Let ɛ > 0. Let ![]() $n\leq x \lt n+1$,
$n\leq x \lt n+1$, ![]() $n\geq 1$ so big that
$n\geq 1$ so big that ![]() $h(n) \gt 1$. Clearly, the following inequality holds
$h(n) \gt 1$. Clearly, the following inequality holds
 \begin{equation}
\left(1-\frac{1}{h(n)}\right)^{h(k)}\leq \left(1-\frac{1}{h(x)}\right)^{h(k)}\leq\left(1-\frac{1}{h(n+1)}\right)^{h(k)}.
\end{equation}
\begin{equation}
\left(1-\frac{1}{h(n)}\right)^{h(k)}\leq \left(1-\frac{1}{h(x)}\right)^{h(k)}\leq\left(1-\frac{1}{h(n+1)}\right)^{h(k)}.
\end{equation} From this, we get for ![]() $k\leq n-1$
$k\leq n-1$
 \begin{equation*}1\geq \left(1-\frac{1}{h(x)}\right)^{h(k)}\geq \left(1-\frac{1}{h(n)}\right)^{h(n-1)} =\left(\left(1-\frac{1}{w_n}\right)^{w_n}\right)^{\frac{w_ {n-1}}{w_n}}\rightarrow 1\end{equation*}
\begin{equation*}1\geq \left(1-\frac{1}{h(x)}\right)^{h(k)}\geq \left(1-\frac{1}{h(n)}\right)^{h(n-1)} =\left(\left(1-\frac{1}{w_n}\right)^{w_n}\right)^{\frac{w_ {n-1}}{w_n}}\rightarrow 1\end{equation*} as ![]() $n\rightarrow +\infty$ since
$n\rightarrow +\infty$ since  $\frac{w_{n+1}}{w_n}\rightarrow +\infty$. Thus, there is some N > 0 such that, whenever
$\frac{w_{n+1}}{w_n}\rightarrow +\infty$. Thus, there is some N > 0 such that, whenever ![]() $N\leq k\leq n-1$ and
$N\leq k\leq n-1$ and ![]() $n\leq x \lt n+1$,
$n\leq x \lt n+1$,
 \begin{equation}
1-\varepsilon\leq \left(1-\frac{1}{h(x)}\right)^{h(k)}\leq 1.
\end{equation}
\begin{equation}
1-\varepsilon\leq \left(1-\frac{1}{h(x)}\right)^{h(k)}\leq 1.
\end{equation} Next let ![]() $k\geq n+2$. From 19, we get
$k\geq n+2$. From 19, we get
 \begin{equation*}\left(1-\frac{1}{h(x)}\right)^{h(k)}\leq \left(1-\frac{1}{h(n+1)}\right)^{h(k)}=\left(\left(1-\frac{1}{w_{n+1}}\right)^{w_{n+1}}\right)^{\frac{w_ {k}}{w_{n+1}}}.\end{equation*}
\begin{equation*}\left(1-\frac{1}{h(x)}\right)^{h(k)}\leq \left(1-\frac{1}{h(n+1)}\right)^{h(k)}=\left(\left(1-\frac{1}{w_{n+1}}\right)^{w_{n+1}}\right)^{\frac{w_ {k}}{w_{n+1}}}.\end{equation*} Now there is some N ′ such that, for all ![]() $n\geq N'$,
$n\geq N'$,
 \begin{equation*}\left(1-\frac{1}{w_{n+1}}\right)^{w_{n+1}}\leq \frac{1}{2}\end{equation*}
\begin{equation*}\left(1-\frac{1}{w_{n+1}}\right)^{w_{n+1}}\leq \frac{1}{2}\end{equation*} and, for all ![]() $k\geq n+2\geq N^{\prime}$,
$k\geq n+2\geq N^{\prime}$,
 \begin{equation*}\frac{w_k}{w_{n+1}}=\prod_{j=0}^{k-n+2}\frac{w_{k-j}}{w_{k-j-1}}\geq 2^{k-(n+1)}\geq k-(n+1),\end{equation*}
\begin{equation*}\frac{w_k}{w_{n+1}}=\prod_{j=0}^{k-n+2}\frac{w_{k-j}}{w_{k-j-1}}\geq 2^{k-(n+1)}\geq k-(n+1),\end{equation*} so that, with ![]() $M=\sup_n a_n$,
$M=\sup_n a_n$,
 \begin{equation}
\sum_{k=n+2}^{+\infty}a_k \left(1-\frac{1}{h(x)}\right)^{h(k)}\leq M \sum_{k=n+2}^{+\infty}\frac{1}{2^{k-(n+1)}}=M.
\end{equation}
\begin{equation}
\sum_{k=n+2}^{+\infty}a_k \left(1-\frac{1}{h(x)}\right)^{h(k)}\leq M \sum_{k=n+2}^{+\infty}\frac{1}{2^{k-(n+1)}}=M.
\end{equation} Thus, for ![]() $n\geq \max(N,N')$ and
$n\geq \max(N,N')$ and ![]() $n\leq x \lt n+1$, let us define
$n\leq x \lt n+1$, let us define
 \begin{equation*}\begin{array}{rcl}\rho_n &:=&\displaystyle\frac{\sum\limits_{k\geq 0}a_k \left(1-\frac{1}{h(x)}\right)^{h(k)}}{\sum\limits_{k\leq x}a_k}\\
&=&\displaystyle\frac{\sum\limits_{k=0}^{N-1}u_k(x)+\sum\limits_{k=N}^{n-1}u_k(x)+\sum\limits_{k=n}^{n+1}u_k(x)+\sum\limits_{k\geq n+2}u_k(x)}{\sum\limits_{k=0}^{N-1}a_k+\sum\limits_{k=N}^{n-1}a_k +a_n},
\end{array}
\end{equation*}
\begin{equation*}\begin{array}{rcl}\rho_n &:=&\displaystyle\frac{\sum\limits_{k\geq 0}a_k \left(1-\frac{1}{h(x)}\right)^{h(k)}}{\sum\limits_{k\leq x}a_k}\\
&=&\displaystyle\frac{\sum\limits_{k=0}^{N-1}u_k(x)+\sum\limits_{k=N}^{n-1}u_k(x)+\sum\limits_{k=n}^{n+1}u_k(x)+\sum\limits_{k\geq n+2}u_k(x)}{\sum\limits_{k=0}^{N-1}a_k+\sum\limits_{k=N}^{n-1}a_k +a_n},
\end{array}
\end{equation*} with  $u_k(x)=a_k \left(1-\frac{1}{h(x)}\right)^{h(k)}$. Since
$u_k(x)=a_k \left(1-\frac{1}{h(x)}\right)^{h(k)}$. Since  $(1-\varepsilon)\sum\limits_{k=N}^{n-1}a_k\leq\sum\limits_{k=N}^{n-1}u_k(x)\leq \sum\limits_{k=N}^{n-1}a_k$ thanks to (20), we deduce, taking into account (21) and the fact that
$(1-\varepsilon)\sum\limits_{k=N}^{n-1}a_k\leq\sum\limits_{k=N}^{n-1}u_k(x)\leq \sum\limits_{k=N}^{n-1}a_k$ thanks to (20), we deduce, taking into account (21) and the fact that ![]() $(a_n)$ is a bounded sequence,
$(a_n)$ is a bounded sequence,
 \begin{equation*}1-\varepsilon\leq \liminf\limits_{n\rightarrow +\infty}\rho_n\leq\limsup\limits_{n\rightarrow +\infty}\rho_n\leq 1,\end{equation*}
\begin{equation*}1-\varepsilon\leq \liminf\limits_{n\rightarrow +\infty}\rho_n\leq\limsup\limits_{n\rightarrow +\infty}\rho_n\leq 1,\end{equation*}which implies the claim.
As a direct corollary of Lemma 5.1, we can state the following result.
Lemma 5.2. Let ![]() $(w_n)$ be an increasing sequence of positive integers such that
$(w_n)$ be an increasing sequence of positive integers such that  $\frac{w_{n+1}}{w_n}\rightarrow +\infty$ as n tends to infinity. Let
$\frac{w_{n+1}}{w_n}\rightarrow +\infty$ as n tends to infinity. Let ![]() $(a_n)$ be a bounded sequence of positive real numbers such that
$(a_n)$ be a bounded sequence of positive real numbers such that ![]() $\sum a_n=+\infty$. If we denote by
$\sum a_n=+\infty$. If we denote by ![]() $h:\mathbb{R}_+\rightarrow\mathbb{R}_+$ a continuous and strictly increasing function with, for all
$h:\mathbb{R}_+\rightarrow\mathbb{R}_+$ a continuous and strictly increasing function with, for all ![]() $n\in\mathbb{N}$,
$n\in\mathbb{N}$, ![]() $h(n)=w_n$, the following estimate holds
$h(n)=w_n$, the following estimate holds
 \begin{equation*}\sum_{n\geq 0} a_nr^{w_n}\sim (\theta_a\circ h^{-1})\left(\frac{1}{1-r}\right)\quad\hbox{ as }r\rightarrow 1^{-},\end{equation*}
\begin{equation*}\sum_{n\geq 0} a_nr^{w_n}\sim (\theta_a\circ h^{-1})\left(\frac{1}{1-r}\right)\quad\hbox{ as }r\rightarrow 1^{-},\end{equation*} where for all ![]() $x\in{\mathbb{R}_+}$,
$x\in{\mathbb{R}_+}$,  $\theta_a(x)=\sum\limits_{n\leq x} {a_n}$.
$\theta_a(x)=\sum\limits_{n\leq x} {a_n}$.
5.1. The  $\mathcal{U}$-frequently hypercyclic case
$\mathcal{U}$-frequently hypercyclic case
We keep the definitions and the notations of § 4.3. Let us also consider an increasing function ![]() $h:\mathbb{R}_+\rightarrow \mathbb{R}_+$ tending to infinity such that, for any
$h:\mathbb{R}_+\rightarrow \mathbb{R}_+$ tending to infinity such that, for any ![]() $n\in\mathbb{N}$,
$n\in\mathbb{N}$, ![]() $h(n):=u_n\in\mathbb{N}$ and
$h(n):=u_n\in\mathbb{N}$ and ![]() $u_{n+1}-u_n\rightarrow +\infty$ as n tends to infinity. Let α be a real number. For all integer
$u_{n+1}-u_n\rightarrow +\infty$ as n tends to infinity. Let α be a real number. For all integer ![]() $n\geq 0,$ we set
$n\geq 0,$ we set  $I_{n}^{(u)}=\{2^{u_n},\ldots,2^{u_{n+1}}-1\}.$ Next, for
$I_{n}^{(u)}=\{2^{u_n},\ldots,2^{u_{n+1}}-1\}.$ Next, for ![]() $k\geq 1,$ we keep the definition of integers αk and
$k\geq 1,$ we keep the definition of integers αk and ![]() $\alpha^{*}_{k}$ given in § 4.3. We set
$\alpha^{*}_{k}$ given in § 4.3. We set  $\displaystyle f_{\alpha}^{(u)}=\sum\limits_{n\geq 0}P_{n,\alpha}^{(u)}$ where the blocks
$\displaystyle f_{\alpha}^{(u)}=\sum\limits_{n\geq 0}P_{n,\alpha}^{(u)}$ where the blocks  $(P_{n,\alpha}^{(u)})$ are polynomials defined as follows, using Rudin–Shapiro polynomials given by Lemma 4.6,
$(P_{n,\alpha}^{(u)})$ are polynomials defined as follows, using Rudin–Shapiro polynomials given by Lemma 4.6,
 \begin{equation}
P_{n,\alpha}^{(u)}(z) = \left\{\begin{array}{l}
0 \hbox{ if } n \mbox{ is odd }\\
0 \hbox{ if } n\in \mathcal{A}_{k}\hbox{ and } 2^{u_{n-1}} \lt \alpha_{k}\\
z^{2^{u_n}}Q_{n}(z) \hbox{ if } n\in \mathcal{A}_{k}\hbox{ and }2^{u_{n-1}}\geq \alpha_{k}
\end{array}\right.
\end{equation}
\begin{equation}
P_{n,\alpha}^{(u)}(z) = \left\{\begin{array}{l}
0 \hbox{ if } n \mbox{ is odd }\\
0 \hbox{ if } n\in \mathcal{A}_{k}\hbox{ and } 2^{u_{n-1}} \lt \alpha_{k}\\
z^{2^{u_n}}Q_{n}(z) \hbox{ if } n\in \mathcal{A}_{k}\hbox{ and }2^{u_{n-1}}\geq \alpha_{k}
\end{array}\right.
\end{equation} with for ![]() $n\in \mathcal{A}_{k}$,
$n\in \mathcal{A}_{k}$,
 \begin{equation*}Q_{n}^{(u)}(z)=\sum\limits_{j\in I_{n}^{(u)}}(j+1)^{-\alpha}c_{j-2^{u_n}}^{(k)}z^{j-2^{u_n}}\end{equation*}
\begin{equation*}Q_{n}^{(u)}(z)=\sum\limits_{j\in I_{n}^{(u)}}(j+1)^{-\alpha}c_{j-2^{u_n}}^{(k)}z^{j-2^{u_n}}\end{equation*} where the sequence  $(c_{j}^{(k)})$ denotes the sequence of the coefficients of the polynomial
$(c_{j}^{(k)})$ denotes the sequence of the coefficients of the polynomial  $\displaystyle p_{\lfloor \frac{2^{u_n}}{\alpha_{k}} \rfloor}(z^{\alpha_k})\tilde{q_{k}}(z)$. We also set
$\displaystyle p_{\lfloor \frac{2^{u_n}}{\alpha_{k}} \rfloor}(z^{\alpha_k})\tilde{q_{k}}(z)$. We also set  $\displaystyle f^{*(u)}_{\alpha}=\sum\limits_{n\geq 0}P^{*(u)}_{n,\alpha}$ where the blocks
$\displaystyle f^{*(u)}_{\alpha}=\sum\limits_{n\geq 0}P^{*(u)}_{n,\alpha}$ where the blocks  $(P^{*(u)}_{n,\alpha})$ are polynomials defined as follows, using polynomials given by Lemma 4.6,
$(P^{*(u)}_{n,\alpha})$ are polynomials defined as follows, using polynomials given by Lemma 4.6,
 \begin{equation}
P^{*(u)}_{n,\alpha}(z) = \left\{\begin{array}{l}
0 \hbox{ if } n \hbox{ is odd }\\
0 \hbox{ if } n\in \mathcal{A}_{k}\hbox{ and } 2^{u_{n-1}} \lt \alpha^{*}_{k}\\
\displaystyle z^{2^{u_n}}Q_{n}^{*(u)}(z)\hbox{ if } n\in \mathcal{A}_{k}\hbox{ and } 2^{u_{n-1}}\geq\alpha^{*}_{k},
\end{array}\right.
\end{equation}
\begin{equation}
P^{*(u)}_{n,\alpha}(z) = \left\{\begin{array}{l}
0 \hbox{ if } n \hbox{ is odd }\\
0 \hbox{ if } n\in \mathcal{A}_{k}\hbox{ and } 2^{u_{n-1}} \lt \alpha^{*}_{k}\\
\displaystyle z^{2^{u_n}}Q_{n}^{*(u)}(z)\hbox{ if } n\in \mathcal{A}_{k}\hbox{ and } 2^{u_{n-1}}\geq\alpha^{*}_{k},
\end{array}\right.
\end{equation} with, for ![]() $n\in \mathcal{A}_{k}$,
$n\in \mathcal{A}_{k}$,
 \begin{equation*}Q_{n}^{*(u)}(z)=\sum\limits_{j\in I_{n}^{(u)}}(j+1)^{-\alpha}c_{j-2^{u_n}}^{(k)}z^{j-2^{u_n}}\end{equation*}
\begin{equation*}Q_{n}^{*(u)}(z)=\sum\limits_{j\in I_{n}^{(u)}}(j+1)^{-\alpha}c_{j-2^{u_n}}^{(k)}z^{j-2^{u_n}}\end{equation*} where the sequence  $(c_{j}^{(k)})$ denotes the sequence of the coefficients of the polynomial
$(c_{j}^{(k)})$ denotes the sequence of the coefficients of the polynomial  $\displaystyle p^{*}_{\lfloor \frac{2^{u_n}}{\alpha^{*}_{k}} \rfloor}(z^{\alpha^{*}_{k}})\tilde{q_{k}}(z)$.
$\displaystyle p^{*}_{\lfloor \frac{2^{u_n}}{\alpha^{*}_{k}} \rfloor}(z^{\alpha^{*}_{k}})\tilde{q_{k}}(z)$.
For ![]() $1\leq p\leq\infty$, we denote by αc the critical exponent
$1\leq p\leq\infty$, we denote by αc the critical exponent  $\alpha_c=\frac{1}{\max(2,q)}$.
$\alpha_c=\frac{1}{\max(2,q)}$.
Lemma 5.3. We have, for any ![]() $0 \lt r \lt 1$,
$0 \lt r \lt 1$,
 \begin{align*}M_p(P_{n,\alpha_c}^{(u)},r)\lesssim r^{2^{u_n}}&\hbox{ if } 2\leq p\leq\infty,\quad M_p(P^{*(u)}_{n,\alpha_c},r)\lesssim r^{2^{u_n}}\hbox{ if } 1 \lt p \lt 2,\\ &\hbox{ and }\quad\quad M_1(P^{*(u)}_{n,0},r)\lesssim r^{2^{u_n}}l_k.
\end{align*}
\begin{align*}M_p(P_{n,\alpha_c}^{(u)},r)\lesssim r^{2^{u_n}}&\hbox{ if } 2\leq p\leq\infty,\quad M_p(P^{*(u)}_{n,\alpha_c},r)\lesssim r^{2^{u_n}}\hbox{ if } 1 \lt p \lt 2,\\ &\hbox{ and }\quad\quad M_1(P^{*(u)}_{n,0},r)\lesssim r^{2^{u_n}}l_k.
\end{align*}Proof. For p > 1, it suffices to argue along the same lines as the proof of Lemma 4.7 replacing the sequence ![]() $(2^{n})$ by
$(2^{n})$ by ![]() $(2^{u_n})$.
$(2^{u_n})$.
Now let us consider the case p = 1 (hence ![]() $\alpha_c=0$). We can write, keeping in mind that
$\alpha_c=0$). We can write, keeping in mind that ![]() $q_k=\tilde{q}_k$ for α = 0,
$q_k=\tilde{q}_k$ for α = 0,
 \begin{equation*}\begin{array}{rcl}M_1(P^{*(u)}_{n,0},r)&\leq&\displaystyle\frac{r^{2^{u_n}}}{2\pi}\int_0^{2\pi}\left\vert Q_n^{*(u)}(r \mathrm{e}^{\mathrm{i}t})\right\vert\,\mathrm{d}t\\ &\leq&\displaystyle
\frac{r^{2^{u_n}}}{2\pi}\int_0^{2\pi}\vert \sum_{j\in I_n^{(u)}} c_{j-2^{u_n}}^{(k)}(r \mathrm{e}^{\mathrm{i}t})^{j-2^{u_n}}\vert\,\mathrm{d}t\\ &\leq&\displaystyle
\frac{r^{2^{u_n}}}{2\pi}\int_0^{2\pi}\left\vert p^{*}_{\lfloor \frac{2^{u_n}}{\alpha^{*}_{k}} \rfloor}((r\mathrm{e}^{\mathrm{i}t})^{\alpha^{*}_{k}})\tilde{q_{k}}(r\mathrm{e}^{\mathrm{i}t})\right\vert\,\mathrm{d}t\\ &\leq&\displaystyle
r^{2^{u_n}} \Vert p^{*}_{\lfloor \frac{2^{u_n}}{\alpha^{*}_{k}} \rfloor}\Vert_1
\Vert q_k\Vert_{\infty}\\ &\lesssim&\displaystyle r^{2^{u_n}}l_k.
\end{array}
\end{equation*}
\begin{equation*}\begin{array}{rcl}M_1(P^{*(u)}_{n,0},r)&\leq&\displaystyle\frac{r^{2^{u_n}}}{2\pi}\int_0^{2\pi}\left\vert Q_n^{*(u)}(r \mathrm{e}^{\mathrm{i}t})\right\vert\,\mathrm{d}t\\ &\leq&\displaystyle
\frac{r^{2^{u_n}}}{2\pi}\int_0^{2\pi}\vert \sum_{j\in I_n^{(u)}} c_{j-2^{u_n}}^{(k)}(r \mathrm{e}^{\mathrm{i}t})^{j-2^{u_n}}\vert\,\mathrm{d}t\\ &\leq&\displaystyle
\frac{r^{2^{u_n}}}{2\pi}\int_0^{2\pi}\left\vert p^{*}_{\lfloor \frac{2^{u_n}}{\alpha^{*}_{k}} \rfloor}((r\mathrm{e}^{\mathrm{i}t})^{\alpha^{*}_{k}})\tilde{q_{k}}(r\mathrm{e}^{\mathrm{i}t})\right\vert\,\mathrm{d}t\\ &\leq&\displaystyle
r^{2^{u_n}} \Vert p^{*}_{\lfloor \frac{2^{u_n}}{\alpha^{*}_{k}} \rfloor}\Vert_1
\Vert q_k\Vert_{\infty}\\ &\lesssim&\displaystyle r^{2^{u_n}}l_k.
\end{array}
\end{equation*} From Lemma 5.2 and 5.3, we deduce the rate of growth of the functions  $f_{\alpha_c}^{(u)}$ and
$f_{\alpha_c}^{(u)}$ and  $f^{*(u)}_{\alpha_c}.$ We begin by the case
$f^{*(u)}_{\alpha_c}.$ We begin by the case ![]() $p\ne 1$.
$p\ne 1$.
Lemma 5.4. Let ![]() $1 \lt p\leq\infty$. Under the preceding definitions and assumptions, the following estimates hold: for all
$1 \lt p\leq\infty$. Under the preceding definitions and assumptions, the following estimates hold: for all ![]() $0 \lt r \lt 1$,
$0 \lt r \lt 1$,
 \begin{align*}&\quad\quad\quad M_p(f_{\alpha_c}^{(u)},r)\lesssim h^{-1}\left(\frac{-\log(1-r)}{\log(2)}\right)\hbox{ if }2\leq p\leq\infty\\ &
\hbox{ and } \quad
M_p(f_{\alpha_c}^{*(u)},r)\lesssim h^{-1}\left(\frac{-\log(1-r)}{\log(2)}\right)\hbox{ if }1 \lt p \lt 2.
\end{align*}
\begin{align*}&\quad\quad\quad M_p(f_{\alpha_c}^{(u)},r)\lesssim h^{-1}\left(\frac{-\log(1-r)}{\log(2)}\right)\hbox{ if }2\leq p\leq\infty\\ &
\hbox{ and } \quad
M_p(f_{\alpha_c}^{*(u)},r)\lesssim h^{-1}\left(\frac{-\log(1-r)}{\log(2)}\right)\hbox{ if }1 \lt p \lt 2.
\end{align*}Proof. Let ![]() $2\leq p\leq\infty$. Combining Lemma 5.3 with triangle inequality, we get
$2\leq p\leq\infty$. Combining Lemma 5.3 with triangle inequality, we get
 \begin{equation*}\displaystyle M_p(f_{\alpha_c}^{(u)},r)\lesssim \sum_{n\geq 0}r^{2^{u_n}}.\end{equation*}
\begin{equation*}\displaystyle M_p(f_{\alpha_c}^{(u)},r)\lesssim \sum_{n\geq 0}r^{2^{u_n}}.\end{equation*} By hypothesis ![]() $2^{u_{n+1}-u_n}\rightarrow +\infty$ as n tends to infinity. We apply Lemma 5.2 with
$2^{u_{n+1}-u_n}\rightarrow +\infty$ as n tends to infinity. We apply Lemma 5.2 with ![]() $w_n=2^{u_n}$ and
$w_n=2^{u_n}$ and ![]() $a_n=1$ and we obtain, for
$a_n=1$ and we obtain, for ![]() $0 \lt r \lt 1$,
$0 \lt r \lt 1$,
 \begin{equation*} M_p(f_{\alpha_c}^{(u)},r)\lesssim h^{-1}\left(\frac{-\log(1-r)}{\log(2)}\right).\end{equation*}
\begin{equation*} M_p(f_{\alpha_c}^{(u)},r)\lesssim h^{-1}\left(\frac{-\log(1-r)}{\log(2)}\right).\end{equation*} For the case ![]() $1 \lt p \lt 2$, the proof works along the same lines.
$1 \lt p \lt 2$, the proof works along the same lines.
Now we are interested in the specific case p = 1.
Lemma 5.5. There is a function of the form  $f_{0}^{*(u)}$ such that
$f_{0}^{*(u)}$ such that
 \begin{equation*}M_1(f_{0}^{*(u)},r)\lesssim \left(h^{-1}\left(\frac{-\log(1-r)}{\log(2)}\right)\right)^2.\end{equation*}
\begin{equation*}M_1(f_{0}^{*(u)},r)\lesssim \left(h^{-1}\left(\frac{-\log(1-r)}{\log(2)}\right)\right)^2.\end{equation*}Proof. Without loss of generality we can assume that ![]() $\alpha_k^* \gt 1+\lfloor m_k\rfloor$ where mk is the least real number such that
$\alpha_k^* \gt 1+\lfloor m_k\rfloor$ where mk is the least real number such that ![]() $g(\log(\alpha_k^*)/\log(2)) \gt l_k$. Observe that, for all
$g(\log(\alpha_k^*)/\log(2)) \gt l_k$. Observe that, for all ![]() $k\geq 1$, for any
$k\geq 1$, for any ![]() $n\in\mathcal{A}_k$ with
$n\in\mathcal{A}_k$ with ![]() $2^{u_n-1}\geq \alpha_k^*$, we have
$2^{u_n-1}\geq \alpha_k^*$, we have ![]() $h^{-1}(u_n)\geq h^{-1}(u_{n-1})\geq h^{-1}(\log(\alpha_k^*)/\log(2))$. Taking into account Lemma 5.3 and the inequality
$h^{-1}(u_n)\geq h^{-1}(u_{n-1})\geq h^{-1}(\log(\alpha_k^*)/\log(2))$. Taking into account Lemma 5.3 and the inequality ![]() $1-t\leq \mathrm{e}^{-t}$, we get, for any
$1-t\leq \mathrm{e}^{-t}$, we get, for any  $1-\frac{1}{2^{u_j}}\leq r \lt 1-\frac{1}{2^{u_{j+1}}}$ (
$1-\frac{1}{2^{u_j}}\leq r \lt 1-\frac{1}{2^{u_{j+1}}}$ (![]() $j\geq 1$),
$j\geq 1$),
 \begin{equation*}\begin{array}{rcl}M_1(f_{0}^{*(u)},r)&\leq&\displaystyle \sum_{n\geq 1}M_1(P^{*(u)}_{n,0},r)\\ &\lesssim&
\displaystyle\sum_k\sum_{n\in\mathcal{A}_k; 2^{u_n-1}\geq \alpha_k^*}\left(1-\frac{1}{2^{u_{j+1}}}\right)^{2^{u_n}}l_k
\\ &\lesssim&\displaystyle
\sum_k\sum_{n\in\mathcal{A}_k; 2^{u_n-1}\geq \alpha_k^*} \mathrm{e}^{-2^{u_n-u_{j+1}}}l_k\frac{h^{-1}(u_{n})}{h^{-1}(\log(\alpha_k^*)/\log(2))}\\ &\lesssim&\displaystyle
\sum_{n=1}^{j+1} \mathrm{e}^{-2^{u_n-u_{j+1}}} h^{-1}(u_{n})\\ &\lesssim&\displaystyle
(j+1)h^{-1}(u_{j+1})=(j+1)^2.
\end{array}\end{equation*}
\begin{equation*}\begin{array}{rcl}M_1(f_{0}^{*(u)},r)&\leq&\displaystyle \sum_{n\geq 1}M_1(P^{*(u)}_{n,0},r)\\ &\lesssim&
\displaystyle\sum_k\sum_{n\in\mathcal{A}_k; 2^{u_n-1}\geq \alpha_k^*}\left(1-\frac{1}{2^{u_{j+1}}}\right)^{2^{u_n}}l_k
\\ &\lesssim&\displaystyle
\sum_k\sum_{n\in\mathcal{A}_k; 2^{u_n-1}\geq \alpha_k^*} \mathrm{e}^{-2^{u_n-u_{j+1}}}l_k\frac{h^{-1}(u_{n})}{h^{-1}(\log(\alpha_k^*)/\log(2))}\\ &\lesssim&\displaystyle
\sum_{n=1}^{j+1} \mathrm{e}^{-2^{u_n-u_{j+1}}} h^{-1}(u_{n})\\ &\lesssim&\displaystyle
(j+1)h^{-1}(u_{j+1})=(j+1)^2.
\end{array}\end{equation*} Since  $2^{u_j}\leq \frac{1}{1-r} \lt 2^{u_{j+1}}$, we find
$2^{u_j}\leq \frac{1}{1-r} \lt 2^{u_{j+1}}$, we find  $j\leq h^{-1}(\frac{-\log(1-r)}{\log(2)})$ and we get
$j\leq h^{-1}(\frac{-\log(1-r)}{\log(2)})$ and we get
 \begin{equation*}M_1(f_{0}^{*(u)},r)\lesssim \left(h^{-1}\left(\frac{-\log(1-r)}{\log(2)}\right)\right)^2.\end{equation*}
\begin{equation*}M_1(f_{0}^{*(u)},r)\lesssim \left(h^{-1}\left(\frac{-\log(1-r)}{\log(2)}\right)\right)^2.\end{equation*} Now we are going to prove that the functions  $f_{\alpha_c}^{(u)}$ and
$f_{\alpha_c}^{(u)}$ and  $f^{*(u)}_{\alpha_c}$ are
$f^{*(u)}_{\alpha_c}$ are ![]() $\mathcal{U}$-frequently hypercyclic for
$\mathcal{U}$-frequently hypercyclic for ![]() $T_{\alpha_c}$.
$T_{\alpha_c}$.
Proposition 5.6. For ![]() $p\geq 2$ (resp.
$p\geq 2$ (resp. ![]() $1\leq p \lt 2$), the function
$1\leq p \lt 2$), the function  $f_{\alpha_c}^{(u)}$ (resp.
$f_{\alpha_c}^{(u)}$ (resp.  $f^{*(u)}_{\alpha_c}$) is a
$f^{*(u)}_{\alpha_c}$) is a ![]() $\mathcal{U}$-frequently hypercyclic vector for the operator
$\mathcal{U}$-frequently hypercyclic vector for the operator ![]() $T_{\alpha_c}$.
$T_{\alpha_c}$.
Proof. We only prove that the vector  $f_{\alpha_c}^{(u)}$ is frequently hypercyclic for the operator
$f_{\alpha_c}^{(u)}$ is frequently hypercyclic for the operator ![]() $T_{\alpha_c}$. We do not repeat the details for
$T_{\alpha_c}$. We do not repeat the details for  $f^{*(u)}_{\alpha_c}$: it will be enough to make the appropriate modifications.
$f^{*(u)}_{\alpha_c}$: it will be enough to make the appropriate modifications.
Let k be a large enough integer. Let us consider ![]() $n\in\mathcal{A}_{k}$ such that
$n\in\mathcal{A}_{k}$ such that ![]() $2^{u_{n-1}}\geq \alpha_{k}.$ We consider
$2^{u_{n-1}}\geq \alpha_{k}.$ We consider ![]() $\mathcal{B}_{n}$ the set of s in
$\mathcal{B}_{n}$ the set of s in ![]() $I_n^{(u)}$ such that the coefficient z s in the polynomial
$I_n^{(u)}$ such that the coefficient z s in the polynomial  $z^{2^{u_n}}p_{\lfloor \frac{2^{u_n}}{\alpha_{k}} \rfloor}(z^{\alpha_{k}})$ is equal to 1 and we denote by
$z^{2^{u_n}}p_{\lfloor \frac{2^{u_n}}{\alpha_{k}} \rfloor}(z^{\alpha_{k}})$ is equal to 1 and we denote by ![]() $\displaystyle T_{k}=\left\{s: s\in \mathcal{B}_{n},\ n\in\mathcal{A}_{k}, \ 2^{u_{n-1}}\geq \alpha_{k}\right\}.$
$\displaystyle T_{k}=\left\{s: s\in \mathcal{B}_{n},\ n\in\mathcal{A}_{k}, \ 2^{u_{n-1}}\geq \alpha_{k}\right\}.$
Observe that ![]() $\max(\mathcal{B}_{n})\leq 2^{u_n+1}$ and since at least half of the coefficients of
$\max(\mathcal{B}_{n})\leq 2^{u_n+1}$ and since at least half of the coefficients of  $p_{\lfloor \frac{2^{u_n}}{\alpha_{k}} \rfloor}$ being +1, we get
$p_{\lfloor \frac{2^{u_n}}{\alpha_{k}} \rfloor}$ being +1, we get
 \begin{equation}\frac{\#\{j\leq \max(\mathcal{B}_{n});j\in T_{k}\}}{\max(\mathcal{B}_{n})}
\geq \frac{2^{u_n-1}}{2^{u_n+1}}=\frac{1}{4},
\end{equation}
\begin{equation}\frac{\#\{j\leq \max(\mathcal{B}_{n});j\in T_{k}\}}{\max(\mathcal{B}_{n})}
\geq \frac{2^{u_n-1}}{2^{u_n+1}}=\frac{1}{4},
\end{equation}which implies
Then let α be a real number and let k be in ![]() $\mathbb{N}$. Now let us consider
$\mathbb{N}$. Now let us consider ![]() $s\in\mathcal{B}_{n}$ with
$s\in\mathcal{B}_{n}$ with ![]() $n\in\mathcal{A}_{k}$ satisfying
$n\in\mathcal{A}_{k}$ satisfying ![]() $2^{u_{n-1}}\geq\alpha_{k}.$ As in Proposition 4.9, by construction we get
$2^{u_{n-1}}\geq\alpha_{k}.$ As in Proposition 4.9, by construction we get
 \begin{equation*}\sup_{\vert z\vert = 1-\frac{1}{l_{k}}} \vert T_{\alpha_c}^{s}(f_{\alpha_c}^{(u)})(z)-q_{k}(z)\vert \lesssim \frac{1}{l_{k}},\end{equation*}
\begin{equation*}\sup_{\vert z\vert = 1-\frac{1}{l_{k}}} \vert T_{\alpha_c}^{s}(f_{\alpha_c}^{(u)})(z)-q_{k}(z)\vert \lesssim \frac{1}{l_{k}},\end{equation*} provided that k is chosen large enough. This allows to obtain frequent hypercyclicity of  $f_{\alpha_c}^{(u)}$.
$f_{\alpha_c}^{(u)}$.
Combining Lemma 5.4 with Proposition 5.6, we obtain the following result.
Theorem 5.7. Let ![]() $1\leq p\leq \infty$ and
$1\leq p\leq \infty$ and  $\alpha_c=\frac{1}{\max(2,q)}$. Then, for any function
$\alpha_c=\frac{1}{\max(2,q)}$. Then, for any function ![]() $\varphi:[0,1)\rightarrow\mathbb{R}_+$ with
$\varphi:[0,1)\rightarrow\mathbb{R}_+$ with ![]() $\varphi(r)\rightarrow +\infty$ as
$\varphi(r)\rightarrow +\infty$ as ![]() $r\rightarrow 1^{-}$, there is a function f in
$r\rightarrow 1^{-}$, there is a function f in ![]() $H(\mathbb{D})$ with
$H(\mathbb{D})$ with
that is ![]() $\mathcal{U}$-frequently hypercyclic for
$\mathcal{U}$-frequently hypercyclic for ![]() $T_{\alpha_c}$.
$T_{\alpha_c}$.
Proof. We begin by the case p > 1. Without loss of generality, we can assume that the function φ is a continuous increasing function that can be written, for all ![]() $0 \lt r \lt 1$,
$0 \lt r \lt 1$,  $\varphi(r)=\psi \left(\frac{1}{1-r}\right)$ where ψ is a continuous increasing function with, for all
$\varphi(r)=\psi \left(\frac{1}{1-r}\right)$ where ψ is a continuous increasing function with, for all ![]() $n\in\mathbb{N}$,
$n\in\mathbb{N}$, ![]() $u_n:=\psi^{-1}(n)\in \mathbb{N}$ and
$u_n:=\psi^{-1}(n)\in \mathbb{N}$ and ![]() $u_{n+1}-u_{n}\rightarrow +\infty$. Thus Lemma 5.4 and Proposition 5.6 ensure that, for all
$u_{n+1}-u_{n}\rightarrow +\infty$. Thus Lemma 5.4 and Proposition 5.6 ensure that, for all ![]() $1 \lt p\leq\infty$, there is a function f in
$1 \lt p\leq\infty$, there is a function f in ![]() $H(\mathbb{D})$ with
$H(\mathbb{D})$ with
 \begin{equation*}M_p(f,r)\lesssim \psi \left(\frac{-\log(1-r)}{\log(2)}\right)\lesssim \psi\left(\frac{1}{1-r}\right)=\varphi(r)\end{equation*}
\begin{equation*}M_p(f,r)\lesssim \psi \left(\frac{-\log(1-r)}{\log(2)}\right)\lesssim \psi\left(\frac{1}{1-r}\right)=\varphi(r)\end{equation*} that is ![]() $\mathcal{U}$-frequently hypercyclic for
$\mathcal{U}$-frequently hypercyclic for ![]() $T_{\alpha_c}$.
$T_{\alpha_c}$.
Now we deal with the case p = 1. Without loss of generality, we can assume that φ is a continuous increasing function that can be written, for all ![]() $0 \lt r \lt 1$,
$0 \lt r \lt 1$,  $\varphi (r)=\left(\psi \left(\frac{1}{1-r}\right)\right)^2$ where ψ is a continuous and increasing function such that, for all
$\varphi (r)=\left(\psi \left(\frac{1}{1-r}\right)\right)^2$ where ψ is a continuous and increasing function such that, for all ![]() $n\in\mathbb{N}$,
$n\in\mathbb{N}$, ![]() $u_n:=\psi^{-1}(n)\in \mathbb{N}$ and
$u_n:=\psi^{-1}(n)\in \mathbb{N}$ and ![]() $u_{n+1}-u_{n}\rightarrow +\infty$. Applying Lemma 5.5 and Proposition 5.6, we find a function
$u_{n+1}-u_{n}\rightarrow +\infty$. Applying Lemma 5.5 and Proposition 5.6, we find a function ![]() $f\in H(\mathbb{D})$ with
$f\in H(\mathbb{D})$ with
 \begin{equation*}M_1(f,r)\lesssim \left(\psi \left(\frac{-\log(1-r)}{\log(2)}\right)\right)^2\lesssim \left(\psi\left(\frac{1}{1-r}\right)\right)^2=\varphi(r)\end{equation*}
\begin{equation*}M_1(f,r)\lesssim \left(\psi \left(\frac{-\log(1-r)}{\log(2)}\right)\right)^2\lesssim \left(\psi\left(\frac{1}{1-r}\right)\right)^2=\varphi(r)\end{equation*} that is ![]() $\mathcal{U}$-frequently hypercyclic for T 0.
$\mathcal{U}$-frequently hypercyclic for T 0.
The proof is complete.
The  $\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic case
$\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic case
We keep the definitions and the notations of § 5.1. We modify the definitions of polynomials  $P_{n,\alpha}^{(u)}$ and
$P_{n,\alpha}^{(u)}$ and  $P_{n,\alpha}^{*(u)}$ as follows:
$P_{n,\alpha}^{*(u)}$ as follows:
 \begin{equation}
P_{n,\alpha}^{(u)}(z) = \left\{\begin{array}{l}
0 \hbox{ if } n \mbox{ is odd }\\
0 \hbox{ if } n\in \mathcal{A}_{k}\hbox{ and } 2^{u_{n-1}} \lt \alpha_{k}\\
z^{2^{u_n}}Q_{n}(z) \hbox{ if } n\in \mathcal{A}_{k}\hbox{ and }2^{u_{n-1}}\geq \alpha_{k}
\end{array}\right.
\end{equation}
\begin{equation}
P_{n,\alpha}^{(u)}(z) = \left\{\begin{array}{l}
0 \hbox{ if } n \mbox{ is odd }\\
0 \hbox{ if } n\in \mathcal{A}_{k}\hbox{ and } 2^{u_{n-1}} \lt \alpha_{k}\\
z^{2^{u_n}}Q_{n}(z) \hbox{ if } n\in \mathcal{A}_{k}\hbox{ and }2^{u_{n-1}}\geq \alpha_{k}
\end{array}\right.
\end{equation} with for ![]() $n\in \mathcal{A}_{k}$,
$n\in \mathcal{A}_{k}$,
 \begin{equation*}Q_{n}^{(u)}(z)=\sum\limits_{j\in I_{n}^{(u)}}(j+1)^{-\alpha}c_{j-2^{u_n}}^{(k)}z^{j-2^{u_n}}\end{equation*}
\begin{equation*}Q_{n}^{(u)}(z)=\sum\limits_{j\in I_{n}^{(u)}}(j+1)^{-\alpha}c_{j-2^{u_n}}^{(k)}z^{j-2^{u_n}}\end{equation*} where the sequence  $(c_{j}^{(k)})$ denotes the sequence of the coefficients of the polynomial
$(c_{j}^{(k)})$ denotes the sequence of the coefficients of the polynomial  $ \displaystyle p_{\lfloor \frac{2^{u_n(1-\gamma)}}{\alpha_{k}} \rfloor}(z^{\alpha_k})\tilde{q_{k}}(z)$.
$ \displaystyle p_{\lfloor \frac{2^{u_n(1-\gamma)}}{\alpha_{k}} \rfloor}(z^{\alpha_k})\tilde{q_{k}}(z)$.
 \begin{equation}
P^{*(u)}_{n,\alpha}(z) = \left\{\begin{array}{l}
0 \hbox{ if } n \hbox{ is odd }\\
0 \hbox{ if } n\in \mathcal{A}_{k}\hbox{ and } 2^{u_{n-1}} \lt \alpha^{*}_{k}\\
\displaystyle z^{2^{u_n}}Q_{n}^{*(u)}(z)\hbox{ if } n\in \mathcal{A}_{k}\hbox{ and } 2^{u_{n-1}}\geq\alpha^{*}_{k},
\end{array}\right.
\end{equation}
\begin{equation}
P^{*(u)}_{n,\alpha}(z) = \left\{\begin{array}{l}
0 \hbox{ if } n \hbox{ is odd }\\
0 \hbox{ if } n\in \mathcal{A}_{k}\hbox{ and } 2^{u_{n-1}} \lt \alpha^{*}_{k}\\
\displaystyle z^{2^{u_n}}Q_{n}^{*(u)}(z)\hbox{ if } n\in \mathcal{A}_{k}\hbox{ and } 2^{u_{n-1}}\geq\alpha^{*}_{k},
\end{array}\right.
\end{equation} with, for ![]() $n\in \mathcal{A}_{k}$,
$n\in \mathcal{A}_{k}$,
 \begin{equation*}Q_{n}^{*(u)}(z)=\sum\limits_{j\in I_{n}^{(u)}}(j+1)^{-\alpha}c_{j-2^{u_n}}^{(k)}z^{j-2^{u_n}}\end{equation*}
\begin{equation*}Q_{n}^{*(u)}(z)=\sum\limits_{j\in I_{n}^{(u)}}(j+1)^{-\alpha}c_{j-2^{u_n}}^{(k)}z^{j-2^{u_n}}\end{equation*} where the sequence  $(c_{j}^{(k)})$ denotes the sequence of the coefficients of the polynomial
$(c_{j}^{(k)})$ denotes the sequence of the coefficients of the polynomial  $\displaystyle p^{*}_{\lfloor \frac{2^{u_n(1-\gamma)}}{\alpha^{*}_{k}} \rfloor}(z^{\alpha^{*}_{k}})\tilde{q_{k}}(z)$.
$\displaystyle p^{*}_{\lfloor \frac{2^{u_n(1-\gamma)}}{\alpha^{*}_{k}} \rfloor}(z^{\alpha^{*}_{k}})\tilde{q_{k}}(z)$.
Let ![]() $1 \lt p\leq\infty$. Set
$1 \lt p\leq\infty$. Set  $\alpha_c=\frac{1-\gamma}{\max(2,q)}$.
$\alpha_c=\frac{1-\gamma}{\max(2,q)}$.
Lemma 5.8. We have, for any ![]() $0 \lt r \lt 1$ and all
$0 \lt r \lt 1$ and all ![]() $n\in\mathbb{N}$,
$n\in\mathbb{N}$,
 \begin{equation*}M_p(P_{n,\alpha_c}^{(u)},r)\lesssim r^{2^{u_n}}\hbox{ if }2\leq p\leq\infty\quad\hbox{ and }\quad
M_p(P_{n,\alpha_c}^{*(u)},r)\lesssim r^{2^{u_n}}\hbox{ if }1 \lt p \lt 2.\end{equation*}
\begin{equation*}M_p(P_{n,\alpha_c}^{(u)},r)\lesssim r^{2^{u_n}}\hbox{ if }2\leq p\leq\infty\quad\hbox{ and }\quad
M_p(P_{n,\alpha_c}^{*(u)},r)\lesssim r^{2^{u_n}}\hbox{ if }1 \lt p \lt 2.\end{equation*}Proof. It suffices to argue along the same lines as the proof of Lemma 4.7 replacing the sequence ![]() $(2^{n})$ by
$(2^{n})$ by ![]() $(2^{u_n})$.
$(2^{u_n})$.
From Lemma 5.2 and 5.8, we deduce the rate of growth of the functions  $f_{\alpha_c}^{(u)}$ and
$f_{\alpha_c}^{(u)}$ and  $f^{*(u)}_{\alpha_c}.$
$f^{*(u)}_{\alpha_c}.$
Lemma 5.9. Let ![]() $1 \lt p\leq\infty$ and
$1 \lt p\leq\infty$ and  $\alpha_c=\frac{1-\gamma}{\max(2,q)}$. Then, for all
$\alpha_c=\frac{1-\gamma}{\max(2,q)}$. Then, for all ![]() $0 \lt r \lt 1$,
$0 \lt r \lt 1$,
 \begin{equation*}M_p(f_{\alpha_c}^{(u)},r)\lesssim h^{-1}\left(\frac{-\log(1-r)}{\log(2)}\right)\hbox{ if }2\leq p\leq\infty,\end{equation*}
\begin{equation*}M_p(f_{\alpha_c}^{(u)},r)\lesssim h^{-1}\left(\frac{-\log(1-r)}{\log(2)}\right)\hbox{ if }2\leq p\leq\infty,\end{equation*}and
 \begin{equation*}M_p(f_{\alpha_c}^{*(u)},r)\lesssim h^{-1}\left(\frac{-\log(1-r)}{\log(2)}\right)\hbox{ if }1 \lt p \lt 2.\end{equation*}
\begin{equation*}M_p(f_{\alpha_c}^{*(u)},r)\lesssim h^{-1}\left(\frac{-\log(1-r)}{\log(2)}\right)\hbox{ if }1 \lt p \lt 2.\end{equation*} Now we are going to prove that the functions  $f_{\alpha_c}^{(u)}$ and
$f_{\alpha_c}^{(u)}$ and  $f^{*(u)}_{\alpha_c}$ are
$f^{*(u)}_{\alpha_c}$ are ![]() $U_{\beta^{\gamma}}$-frequently hypercyclic for
$U_{\beta^{\gamma}}$-frequently hypercyclic for ![]() $T_{\alpha_c}$.
$T_{\alpha_c}$.
Proposition 5.10. For ![]() $p\geq 2$ (resp.
$p\geq 2$ (resp. ![]() $1 \lt p \lt 2$), the function
$1 \lt p \lt 2$), the function  $f_{\alpha_c}^{(u)}$ (resp.
$f_{\alpha_c}^{(u)}$ (resp.  $f^{*(u)}_{\alpha_c}$) is a
$f^{*(u)}_{\alpha_c}$) is a ![]() $U_{\beta^{\gamma}}$-frequently hypercyclic vector for the operator
$U_{\beta^{\gamma}}$-frequently hypercyclic vector for the operator ![]() $T_{\alpha_c}$.
$T_{\alpha_c}$.
Proof. We only prove that the vector  $f_{\alpha_c}^{(u)}$ is frequently hypercyclic for the operator
$f_{\alpha_c}^{(u)}$ is frequently hypercyclic for the operator ![]() $T_{\alpha_c}$. We do not repeat the details for
$T_{\alpha_c}$. We do not repeat the details for  $f^{*(u)}_{\alpha_c}$: it will be enough to make the appropriate modifications.
$f^{*(u)}_{\alpha_c}$: it will be enough to make the appropriate modifications.
Let k be a large enough integer. Let us consider ![]() $n\in\mathcal{A}_{k}$ such that
$n\in\mathcal{A}_{k}$ such that ![]() $2^{u_{n-1}}\geq \alpha_{k}.$ We consider
$2^{u_{n-1}}\geq \alpha_{k}.$ We consider ![]() $\mathcal{B}_{n}$ the set of s in
$\mathcal{B}_{n}$ the set of s in ![]() $I_n^{(u)}$ such that the coefficient z s in the polynomial
$I_n^{(u)}$ such that the coefficient z s in the polynomial  $z^{2^{u_n}}p_{\lfloor \frac{2^{u_n(1-\gamma)}}{\alpha_{k}} \rfloor}(z^{\alpha_{k}})$ is equal to 1 and we denote by
$z^{2^{u_n}}p_{\lfloor \frac{2^{u_n(1-\gamma)}}{\alpha_{k}} \rfloor}(z^{\alpha_{k}})$ is equal to 1 and we denote by ![]() $\displaystyle T_{k}=\left\{s: s\in \mathcal{B}_{n},\ n\in\mathcal{A}_{k}, \ 2^{u_{n-1}}\geq \alpha_{k}\right\}.$
$\displaystyle T_{k}=\left\{s: s\in \mathcal{B}_{n},\ n\in\mathcal{A}_{k}, \ 2^{u_{n-1}}\geq \alpha_{k}\right\}.$
Observe that ![]() $\max(\mathcal{B}_{n})\leq 2^{u_n}+\lfloor2^{u_n(1-\gamma)}\rfloor$ and since at least half of the coefficients of
$\max(\mathcal{B}_{n})\leq 2^{u_n}+\lfloor2^{u_n(1-\gamma)}\rfloor$ and since at least half of the coefficients of  $p_{\lfloor \frac{2^{u_n(1-\gamma)}}{\alpha_{k}} \rfloor}$ being +1, we get
$p_{\lfloor \frac{2^{u_n(1-\gamma)}}{\alpha_{k}} \rfloor}$ being +1, we get
 \begin{equation}\frac{\sum\limits_{j\leq \max(\mathcal{B}_{n});\atop j\in T_{k}}\mathrm{e}^{j^{\gamma}}}{\sum\limits_{j\leq \max(\mathcal{B}_{n})}\mathrm{e}^{j^{\gamma}}}\geq
\frac{\sum\limits_{j=2^{u_n}}^{2^{u_n}+2^{-1}\lfloor2^{u_n(1-\gamma)}\rfloor}\mathrm{e}^{j^{\gamma}}}{\sum\limits_{j=1}^{2^{u_n}+\lfloor2^{u_n(1-\gamma)}\rfloor}\mathrm{e}^{j^{\gamma}}}
=\frac{\sum\limits_{j=1}^{2^{u_n}+2^{-1}\lfloor2^{u_n(1-\gamma)}\rfloor}\mathrm{e}^{j^{\gamma}}}{\sum\limits_{j=1}^{2^{u_n}+\lfloor2^{u_n(1-\gamma)}\rfloor}\mathrm{e}^{j^{\gamma}}}-
\frac{\sum\limits_{j=1}^{2^{u_n}-1}\mathrm{e}^{j^{\gamma}}}{\sum\limits_{j=1}^{2^{u_n}+\lfloor2^{u_n(1-\gamma)}\rfloor}\mathrm{e}^{j^{\gamma}}}.
\end{equation}
\begin{equation}\frac{\sum\limits_{j\leq \max(\mathcal{B}_{n});\atop j\in T_{k}}\mathrm{e}^{j^{\gamma}}}{\sum\limits_{j\leq \max(\mathcal{B}_{n})}\mathrm{e}^{j^{\gamma}}}\geq
\frac{\sum\limits_{j=2^{u_n}}^{2^{u_n}+2^{-1}\lfloor2^{u_n(1-\gamma)}\rfloor}\mathrm{e}^{j^{\gamma}}}{\sum\limits_{j=1}^{2^{u_n}+\lfloor2^{u_n(1-\gamma)}\rfloor}\mathrm{e}^{j^{\gamma}}}
=\frac{\sum\limits_{j=1}^{2^{u_n}+2^{-1}\lfloor2^{u_n(1-\gamma)}\rfloor}\mathrm{e}^{j^{\gamma}}}{\sum\limits_{j=1}^{2^{u_n}+\lfloor2^{u_n(1-\gamma)}\rfloor}\mathrm{e}^{j^{\gamma}}}-
\frac{\sum\limits_{j=1}^{2^{u_n}-1}\mathrm{e}^{j^{\gamma}}}{\sum\limits_{j=1}^{2^{u_n}+\lfloor2^{u_n(1-\gamma)}\rfloor}\mathrm{e}^{j^{\gamma}}}.
\end{equation}Clearly, we have
 \begin{align*}&\left(2^{u_n}+\frac{\lfloor2^{u_n(1-\gamma)}\rfloor}{2}\right)^{\gamma}-\left(2^{u_n}+\lfloor2^{u_n(1-\gamma)}\rfloor\right)^{\gamma}\rightarrow -\frac{\gamma}{2},\\
&\left(2^{u_n}-1\right)^{\gamma}-\left(2^{u_n}+\lfloor2^{u_n(1-\gamma)}\rfloor\right)^{\gamma}\rightarrow -\gamma,
\end{align*}
\begin{align*}&\left(2^{u_n}+\frac{\lfloor2^{u_n(1-\gamma)}\rfloor}{2}\right)^{\gamma}-\left(2^{u_n}+\lfloor2^{u_n(1-\gamma)}\rfloor\right)^{\gamma}\rightarrow -\frac{\gamma}{2},\\
&\left(2^{u_n}-1\right)^{\gamma}-\left(2^{u_n}+\lfloor2^{u_n(1-\gamma)}\rfloor\right)^{\gamma}\rightarrow -\gamma,
\end{align*}which implies, using similar estimations as those of the proof of Lemma 4.4,
 \begin{equation*}\frac{\sum\limits_{j=1}^{2^{u_n}+2^{-1}\lfloor2^{u_n(1-\gamma)}\rfloor}\mathrm{e}^{j^{\gamma}}}{\sum\limits_{j=1}^{2^{u_n}+\lfloor2^{u_n(1-\gamma)}\rfloor}\mathrm{e}^{j^{\gamma}}}-
\frac{\sum\limits_{j=1}^{2^{u_n}-1}\mathrm{e}^{j^{\gamma}}}{\sum\limits_{j=1}^{2^{u_n}+\lfloor2^{u_n(1-\gamma)}\rfloor}\mathrm{e}^{j^{\gamma}}}\rightarrow \mathrm{e}^{-\gamma/2}(1-\mathrm{e}^{-\gamma/2}), \hbox{ as }n\rightarrow +\infty.\end{equation*}
\begin{equation*}\frac{\sum\limits_{j=1}^{2^{u_n}+2^{-1}\lfloor2^{u_n(1-\gamma)}\rfloor}\mathrm{e}^{j^{\gamma}}}{\sum\limits_{j=1}^{2^{u_n}+\lfloor2^{u_n(1-\gamma)}\rfloor}\mathrm{e}^{j^{\gamma}}}-
\frac{\sum\limits_{j=1}^{2^{u_n}-1}\mathrm{e}^{j^{\gamma}}}{\sum\limits_{j=1}^{2^{u_n}+\lfloor2^{u_n(1-\gamma)}\rfloor}\mathrm{e}^{j^{\gamma}}}\rightarrow \mathrm{e}^{-\gamma/2}(1-\mathrm{e}^{-\gamma/2}), \hbox{ as }n\rightarrow +\infty.\end{equation*}Hence the inequality (27) ensures that
Then let α be a real number and let k be in ![]() $\mathbb{N}$. Now for
$\mathbb{N}$. Now for ![]() $s\in\mathcal{B}_{n}$ with
$s\in\mathcal{B}_{n}$ with ![]() $n\in\mathcal{A}_{k}$ satisfying
$n\in\mathcal{A}_{k}$ satisfying ![]() $2^{u_{n-1}}\geq\alpha_{k}$, as in Proposition 4.9, by construction we get
$2^{u_{n-1}}\geq\alpha_{k}$, as in Proposition 4.9, by construction we get
 \begin{equation*}\sup_{\vert z\vert = 1-\frac{1}{l_{k}}} \vert T_{\alpha_c}^{s}(f_{\alpha_c}^{(u)})(z)-q_{k}(z)\vert \lesssim \frac{1}{l_{k}},\end{equation*}
\begin{equation*}\sup_{\vert z\vert = 1-\frac{1}{l_{k}}} \vert T_{\alpha_c}^{s}(f_{\alpha_c}^{(u)})(z)-q_{k}(z)\vert \lesssim \frac{1}{l_{k}},\end{equation*} provided that k is chosen large enough. This allows to obtain frequent hypercyclicity of  $f_{\alpha_c}^{(u)}$.
$f_{\alpha_c}^{(u)}$.
Combining Lemma 5.9 with Proposition 5.10 we obtain the following result.
Theorem 5.11. Let ![]() $0 \lt \gamma \lt 1$. Let
$0 \lt \gamma \lt 1$. Let ![]() $1\leq p\leq \infty$ and
$1\leq p\leq \infty$ and  $\alpha_c=\frac{1-\gamma}{\max(2,q)}$. Then, for any function
$\alpha_c=\frac{1-\gamma}{\max(2,q)}$. Then, for any function ![]() $\varphi:[0,1)\rightarrow\mathbb{R}_+$, with
$\varphi:[0,1)\rightarrow\mathbb{R}_+$, with ![]() $\varphi(r)\rightarrow +\infty$ as
$\varphi(r)\rightarrow +\infty$ as ![]() $r\rightarrow 1^{-}$, there is a function f in
$r\rightarrow 1^{-}$, there is a function f in ![]() $H(\mathbb{D})$ with
$H(\mathbb{D})$ with
that is ![]() $\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic for
$\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic for ![]() $T_{\alpha_c}$.
$T_{\alpha_c}$.
Proof. For p = 1, we have ![]() $\alpha_c=0$ and the result is given by Theorem 5.7. Now let p > 1. Without loss of generality, we can assume that the φ is a continuous increasing function such that, for all
$\alpha_c=0$ and the result is given by Theorem 5.7. Now let p > 1. Without loss of generality, we can assume that the φ is a continuous increasing function such that, for all ![]() $0 \lt r \lt 1$,
$0 \lt r \lt 1$,  $\varphi(r)=\psi \left(\frac{1}{1-r}\right)$ where ψ is continuous and increasing with, for all
$\varphi(r)=\psi \left(\frac{1}{1-r}\right)$ where ψ is continuous and increasing with, for all ![]() $n\in\mathbb{N}$,
$n\in\mathbb{N}$, ![]() $u_n:=\psi^{-1}(n)\in \mathbb{N}$ and
$u_n:=\psi^{-1}(n)\in \mathbb{N}$ and ![]() $u_{n+1}-u_{n}\rightarrow +\infty$. Thus, Lemma 5.9 and Proposition 5.10 ensure that, for all
$u_{n+1}-u_{n}\rightarrow +\infty$. Thus, Lemma 5.9 and Proposition 5.10 ensure that, for all ![]() $1 \lt p\leq\infty$, there is a function f in
$1 \lt p\leq\infty$, there is a function f in ![]() $H(\mathbb{D})$ with
$H(\mathbb{D})$ with
 \begin{equation*}M_p(f,r)\lesssim \psi \left(\frac{-\log(1-r)}{\log(2)}\right)\lesssim \varphi (r)\end{equation*}
\begin{equation*}M_p(f,r)\lesssim \psi \left(\frac{-\log(1-r)}{\log(2)}\right)\lesssim \varphi (r)\end{equation*} that is ![]() $\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic for
$\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic for ![]() $T_{\alpha_c}$. The proof is complete.
$T_{\alpha_c}$. The proof is complete.
From Theorems 2.2, 5.7 and 5.11, we can state the following result that unifies what happens in the critical case, given by the critical exponent, for the Lp growth of ![]() $\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic functions for Tα, when γ belongs to
$\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic functions for Tα, when γ belongs to ![]() $[0,1]$.
$[0,1]$.
Theorem 5.12. Let ![]() $0\leq \gamma \leq 1$ and
$0\leq \gamma \leq 1$ and ![]() $1\leq p\leq \infty$. Then, for any function
$1\leq p\leq \infty$. Then, for any function ![]() $\varphi:[0,1)\rightarrow\mathbb{R}_+$, with
$\varphi:[0,1)\rightarrow\mathbb{R}_+$, with ![]() $\varphi(r)\rightarrow +\infty$ as
$\varphi(r)\rightarrow +\infty$ as ![]() $r\rightarrow 1^{-}$, there is a function f in
$r\rightarrow 1^{-}$, there is a function f in ![]() $H(\mathbb{D})$ with
$H(\mathbb{D})$ with ![]() $M_p(f,r)\lesssim \varphi(r)$ that is
$M_p(f,r)\lesssim \varphi(r)$ that is ![]() $\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic for
$\mathcal{U}_{\beta^{\gamma}}$-frequently hypercyclic for ![]() $T_{\alpha_c}$ where
$T_{\alpha_c}$ where  $\alpha_c=\frac{1-\gamma}{\max(2,q)}$.
$\alpha_c=\frac{1-\gamma}{\max(2,q)}$.
Acknowledgements
The authors would like to thank the anonymous referee for her/his simplification of the proof of Lemma 5.2.